Volume and Capacity Worksheets Rectangular Prism

Worksheets are an excellent tool for practicing and reinforcing concepts related to volume and capacity, specifically focusing on rectangular prisms. These worksheets serve as a valuable resource for teachers and parents alike, offering structured practice that engages learners in a variety of problem-solving activities. By providing a range of exercises that target understanding the relationship between length, width, and height, these worksheets support the development of a solid foundation in this essential mathematical concept.

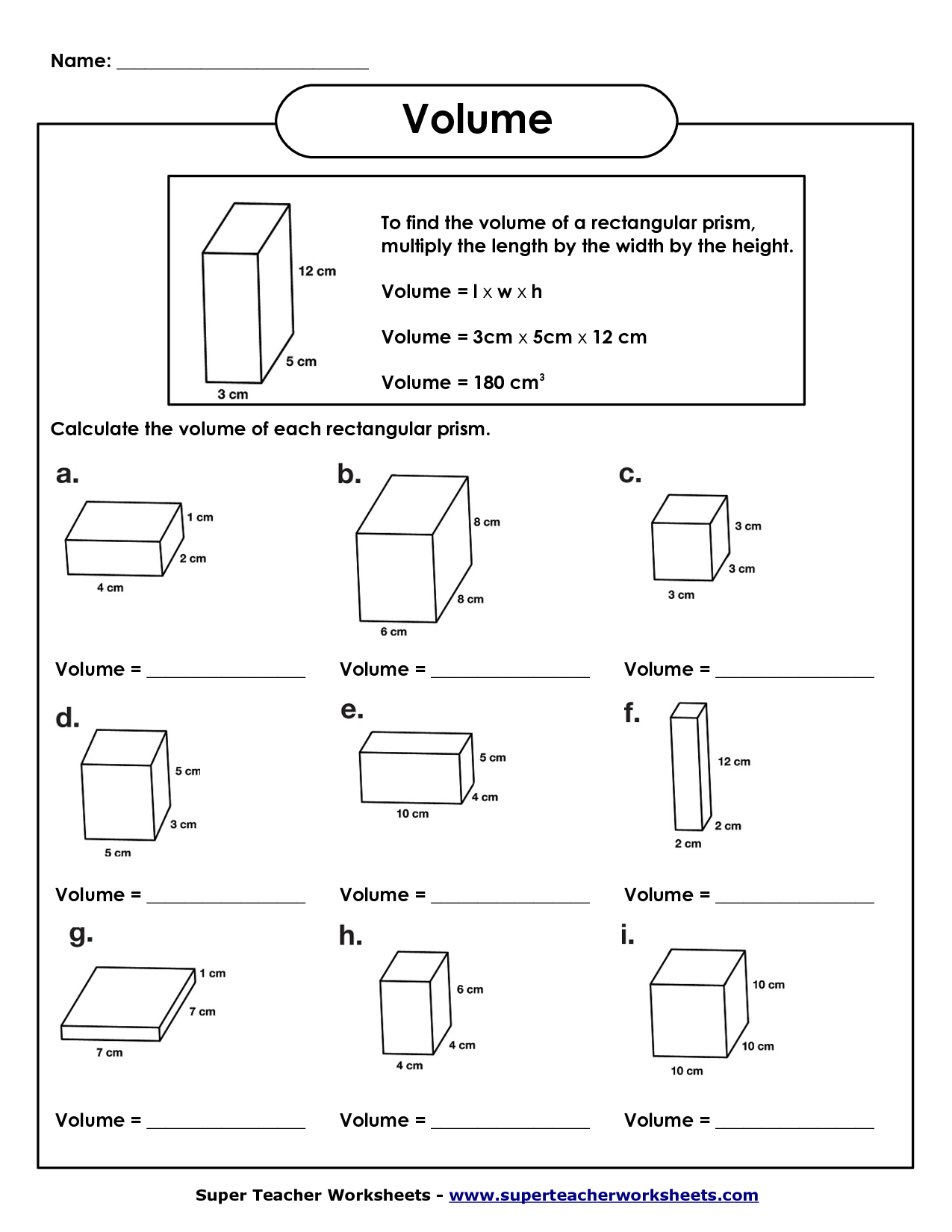
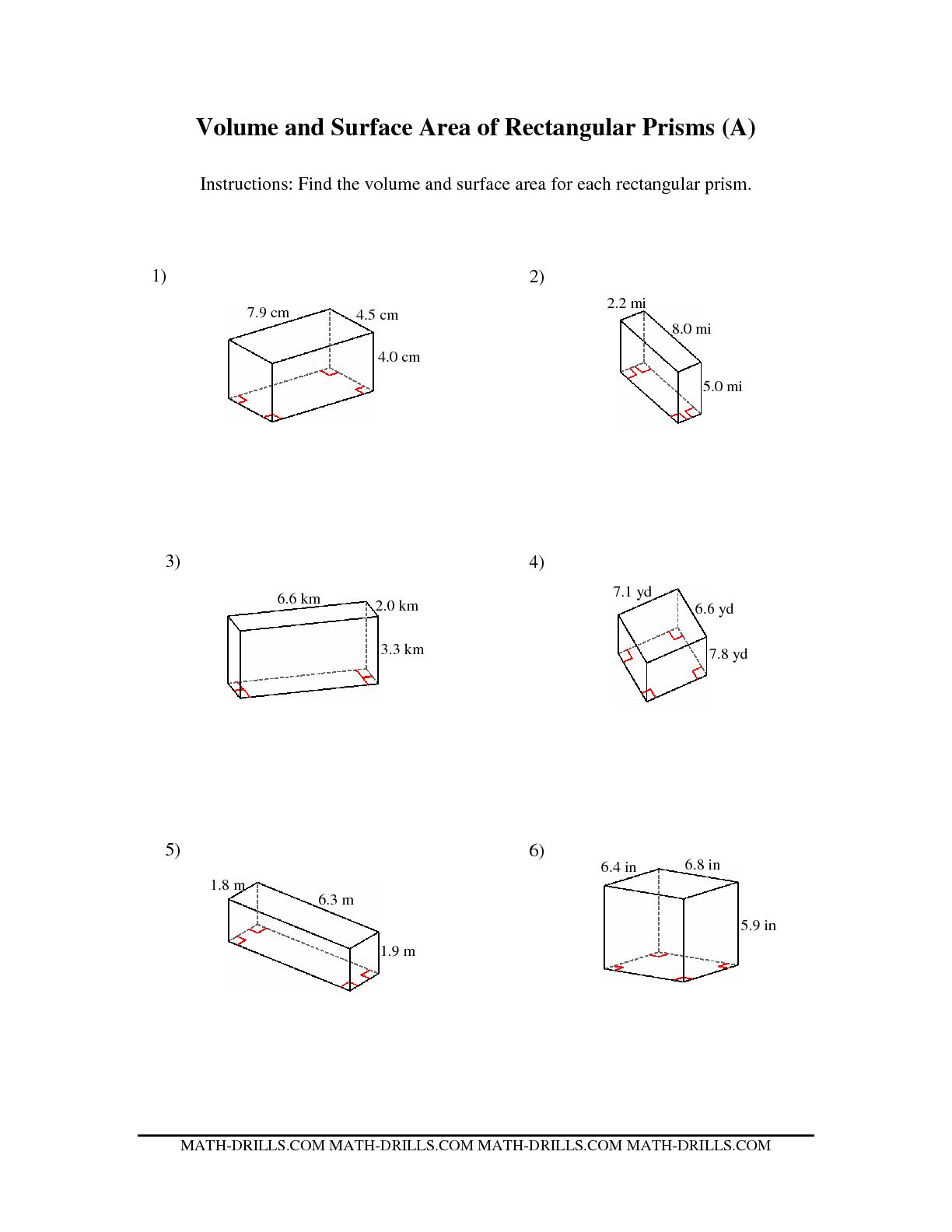
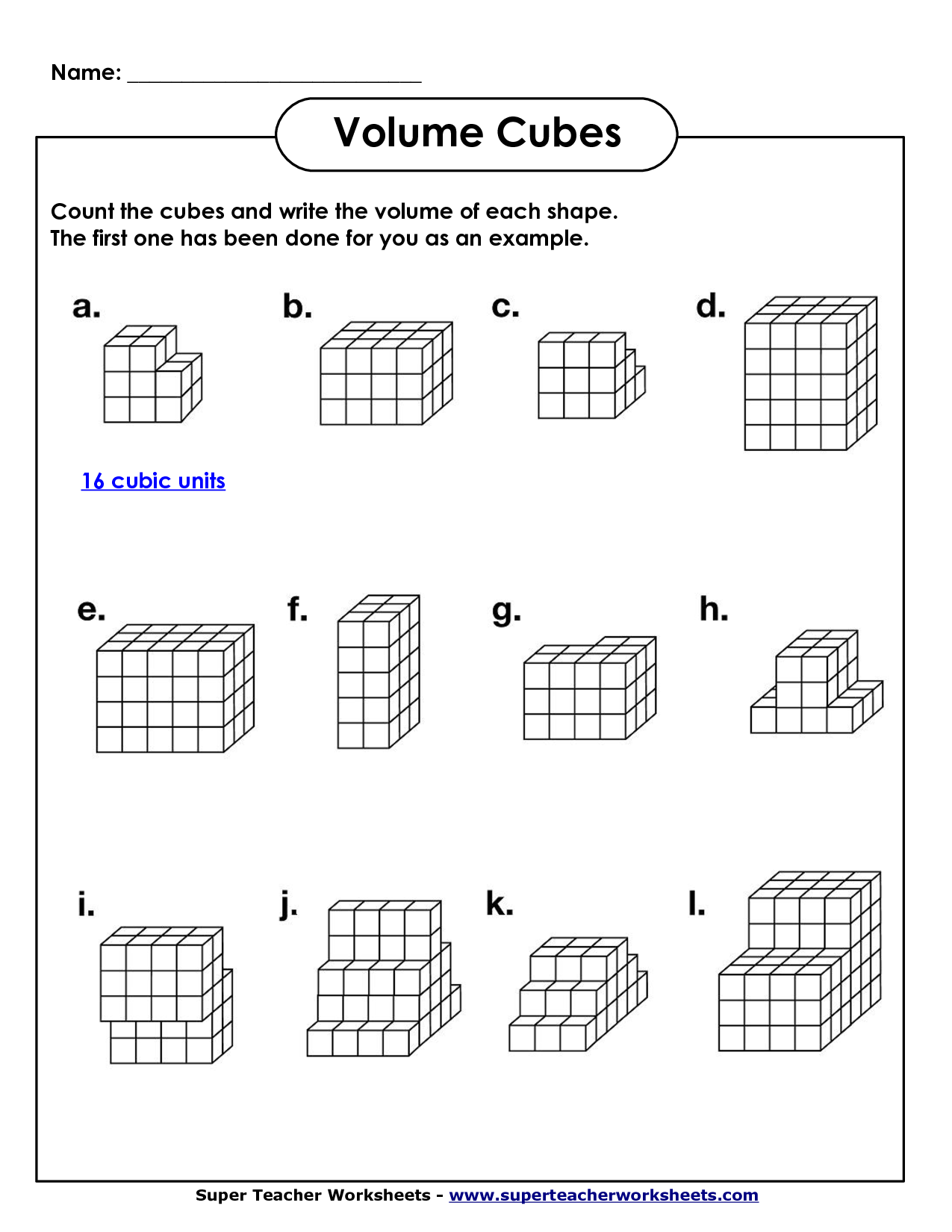
  Rectangular Prism Volume Worksheet

---

  Rectangular Prism Volume Worksheet

---

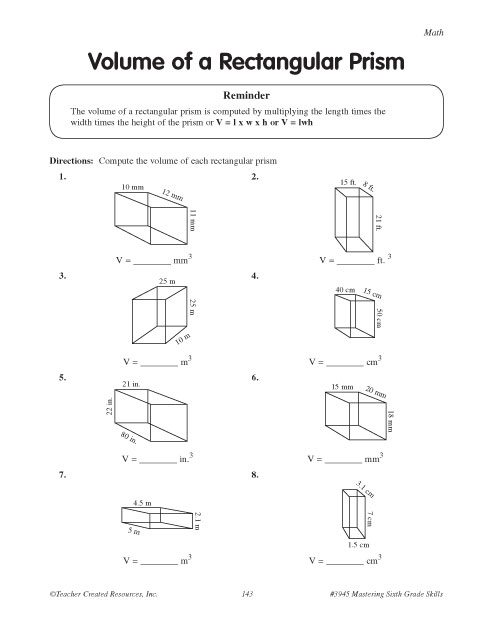
  Volume of Rectangular Prism Worksheets 5th Grade

---

  Volume Worksheets 5th Grade

---

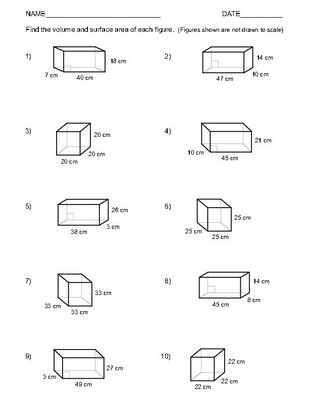
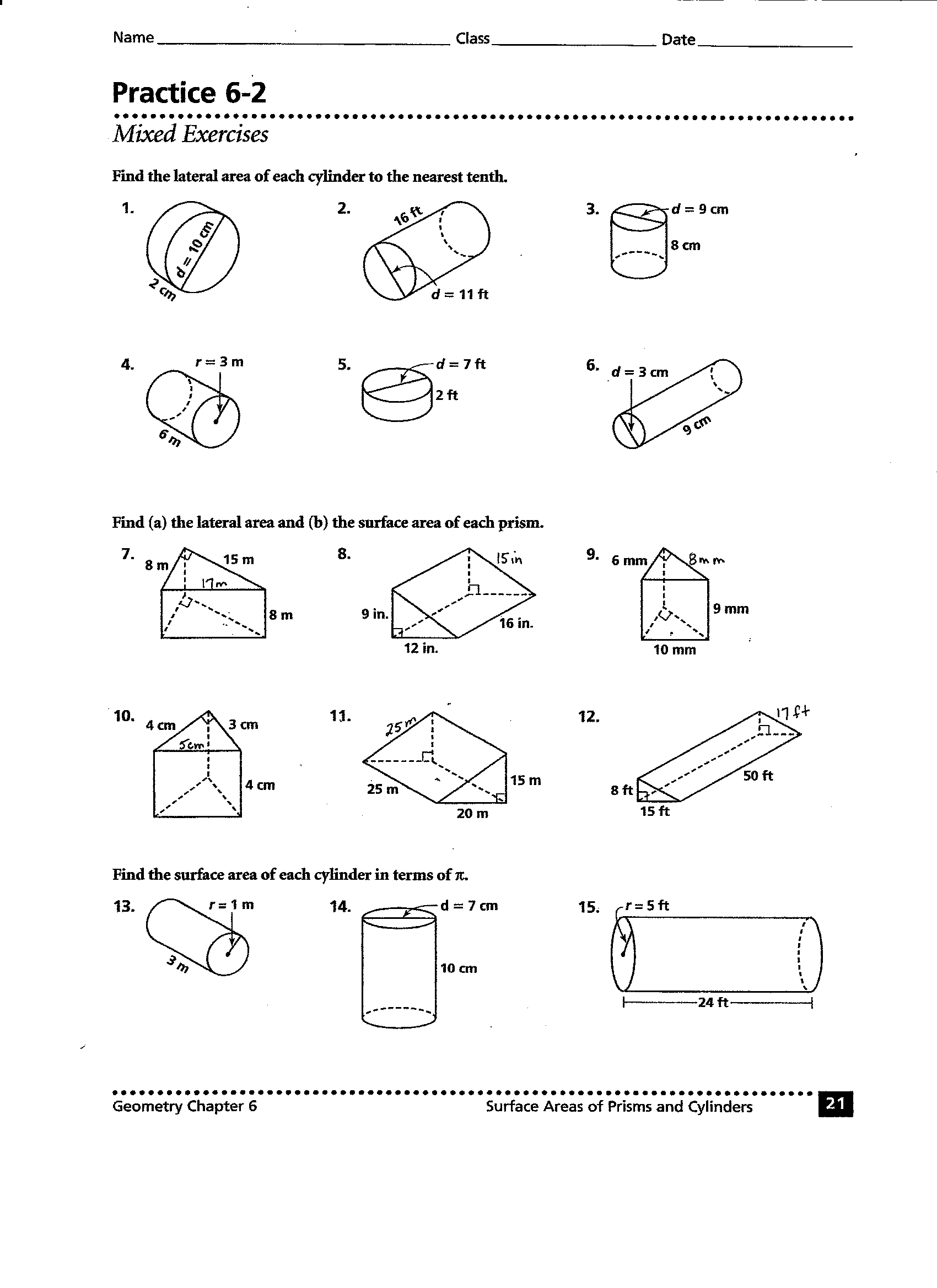
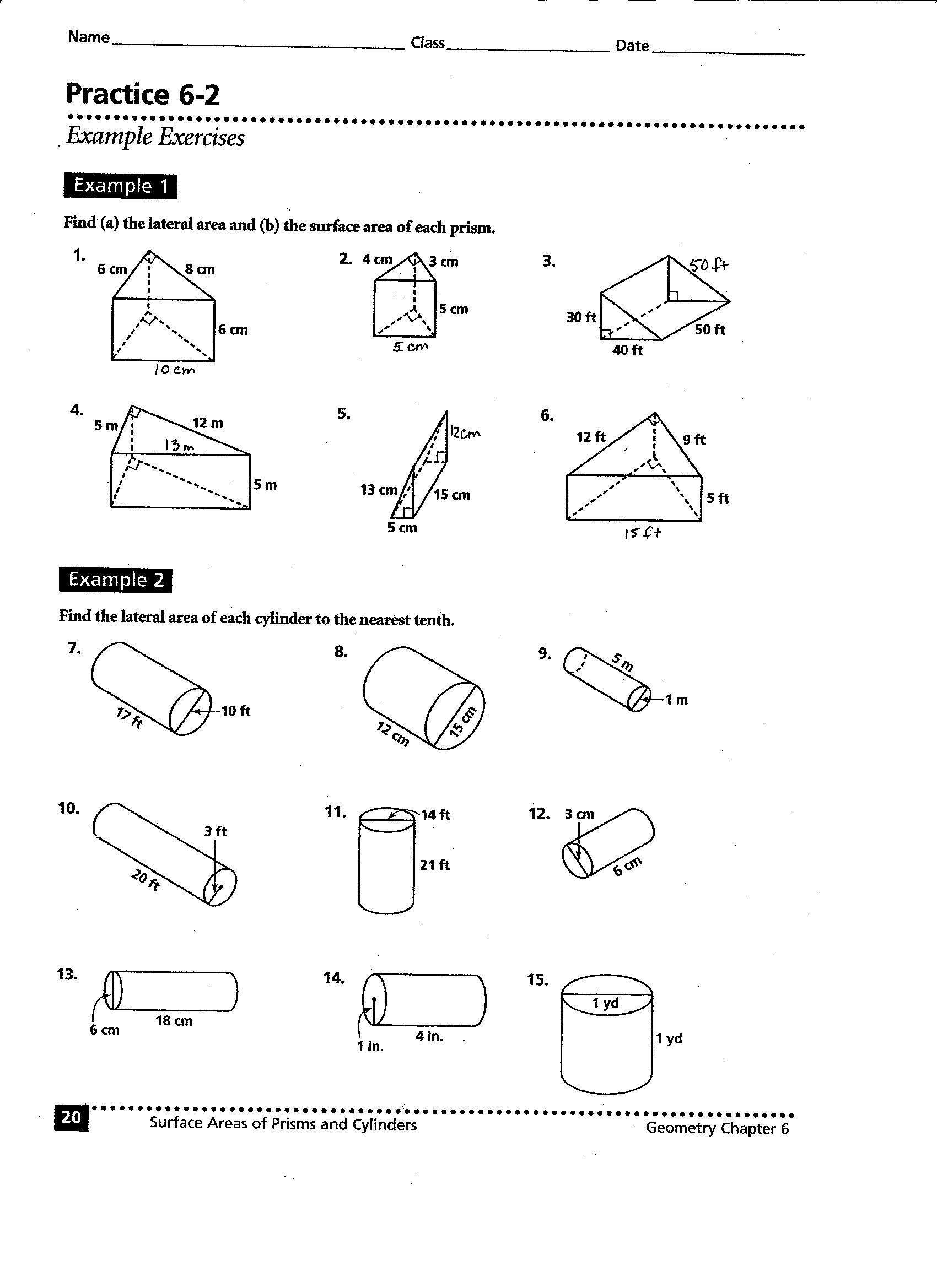
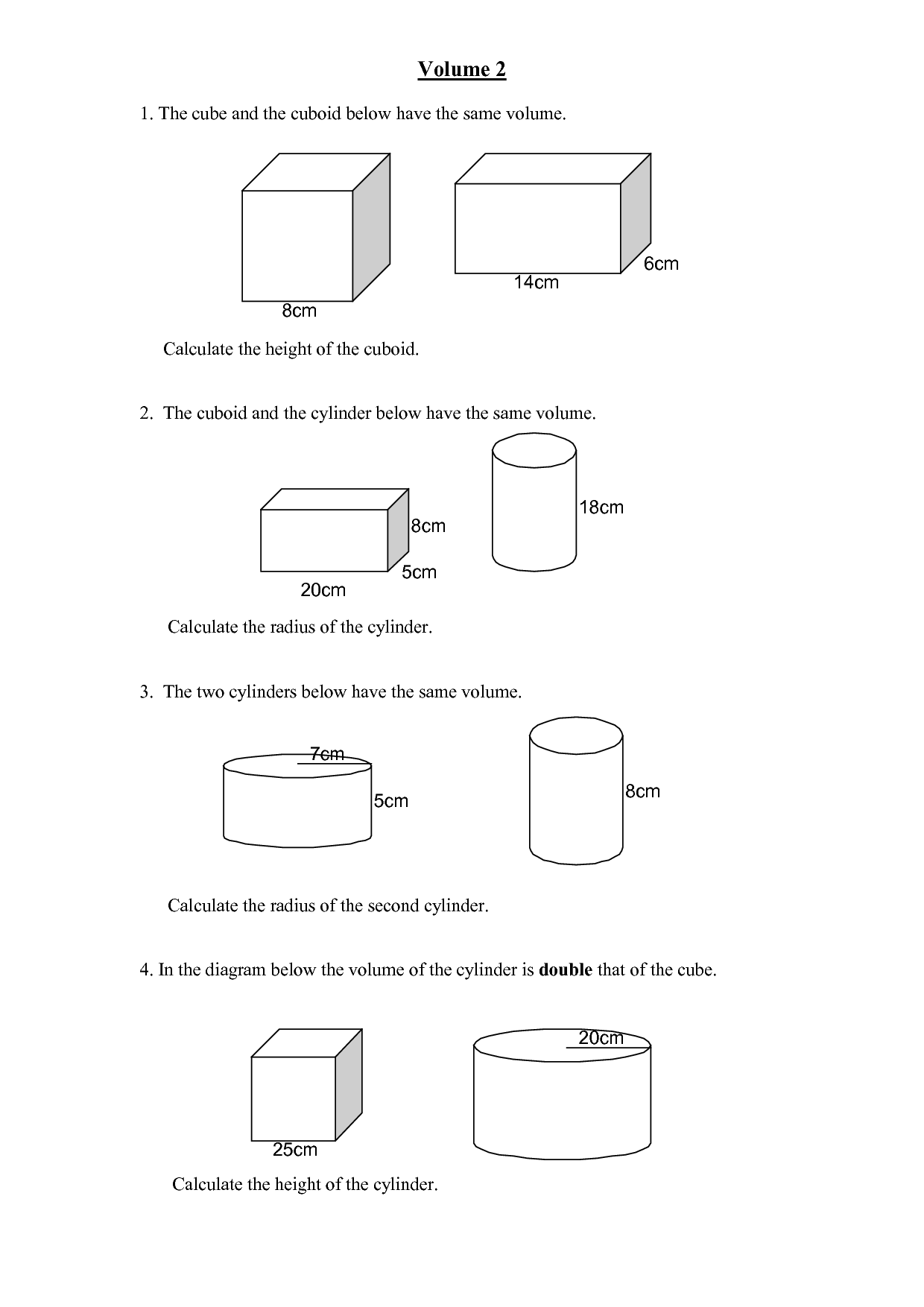
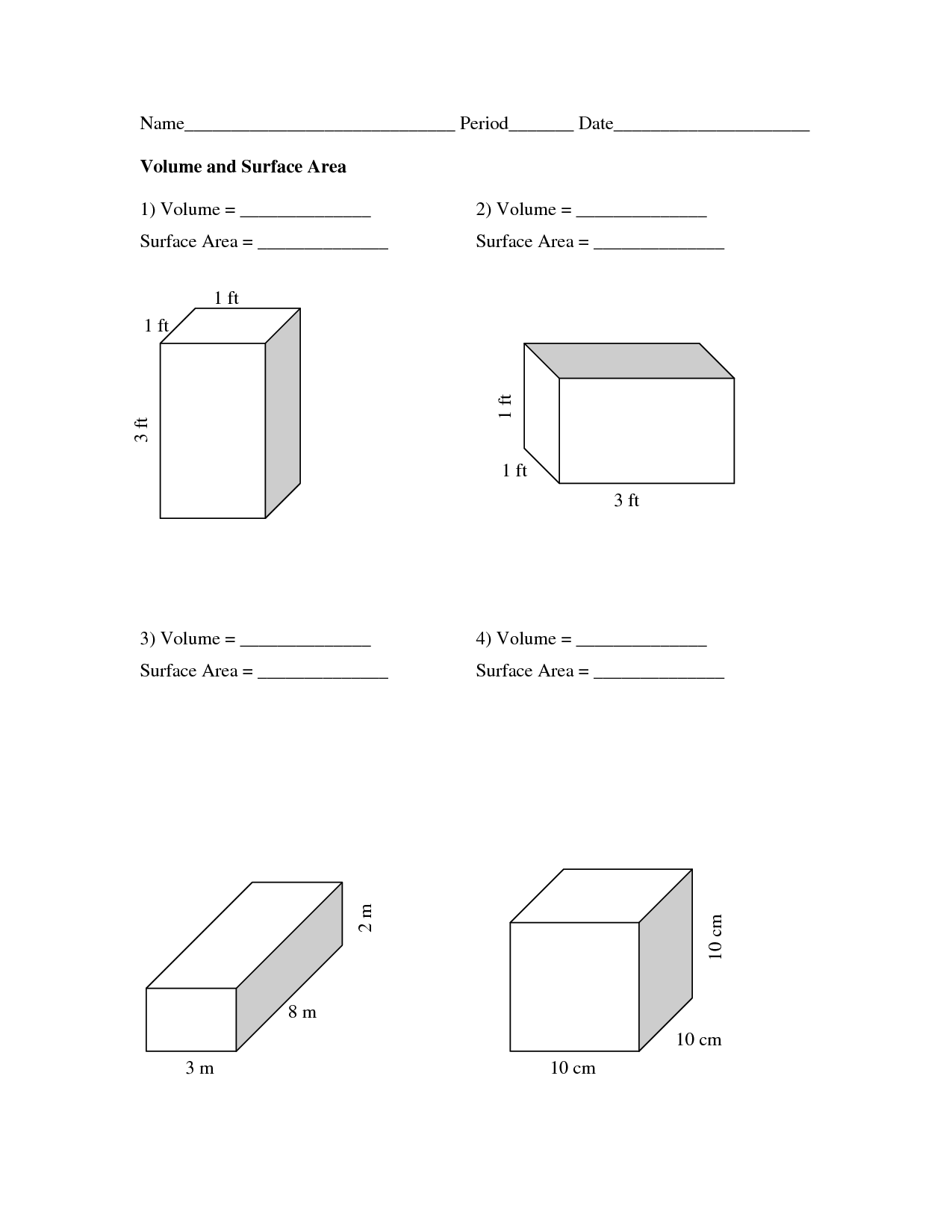
  Surface Area Rectangular Prism Volume Worksheet

---

  Rectangular Prism Volume Worksheet

---

  Rectangular Prism Volume Worksheet

---

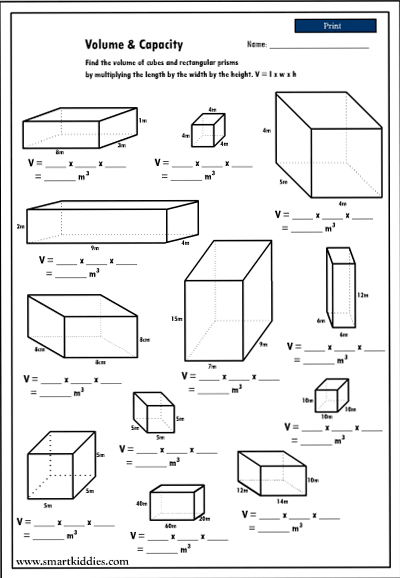
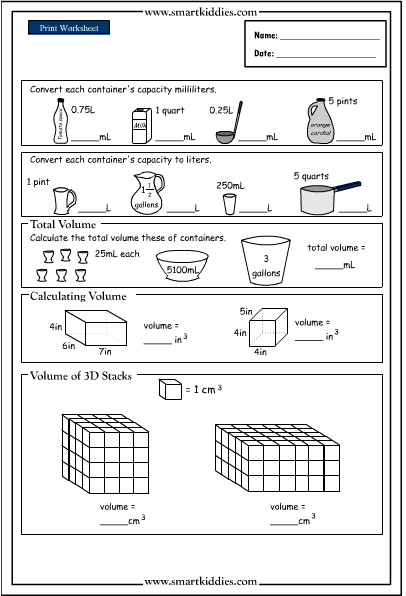
  Volume and Capacity Worksheet

---

  Surface Area of Right Rectangular Prisms

---

  Surface Area and Volume Worksheets

---

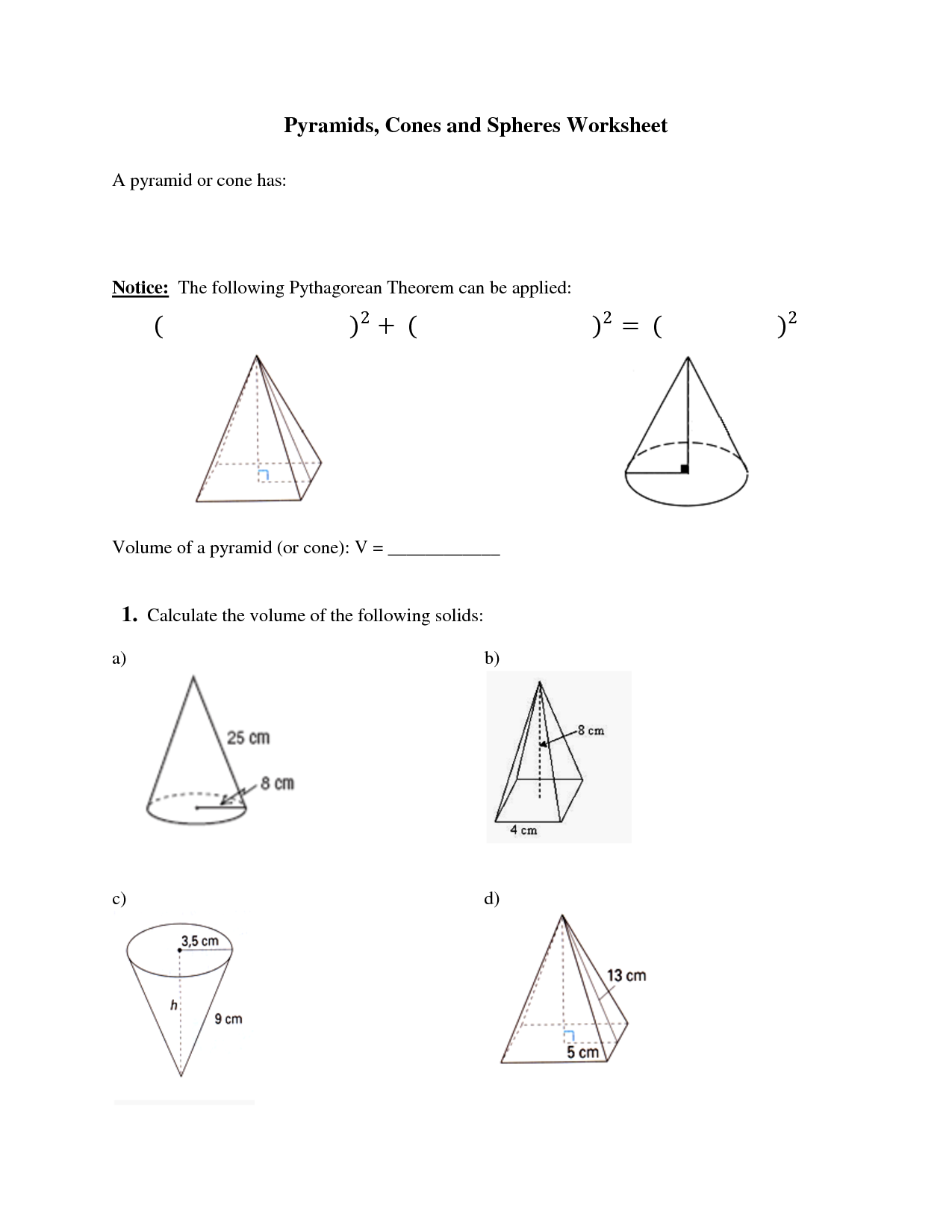
  Volume Pyramid Worksheet

---

  Cube Volume Worksheets 5th Grade Math

---

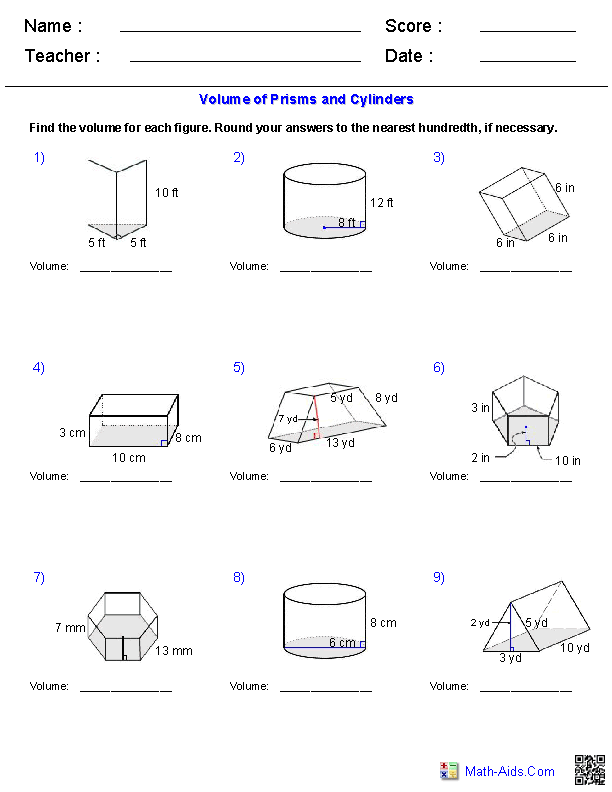
  Volume Prisms and Cylinders Worksheets

---

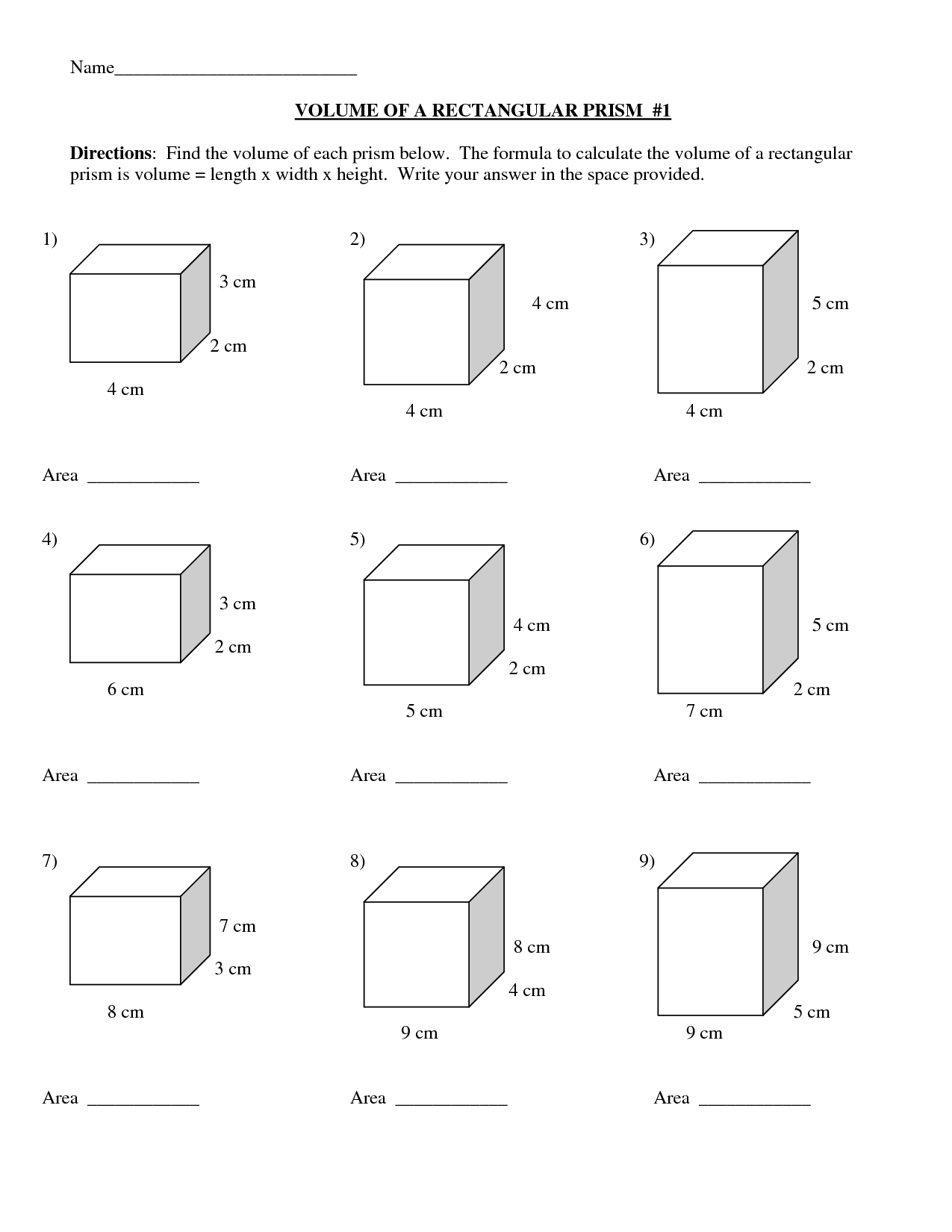
  Rectangular Prism Volume Worksheet

---

  Finding Volume Worksheets Rectangular Prism

---

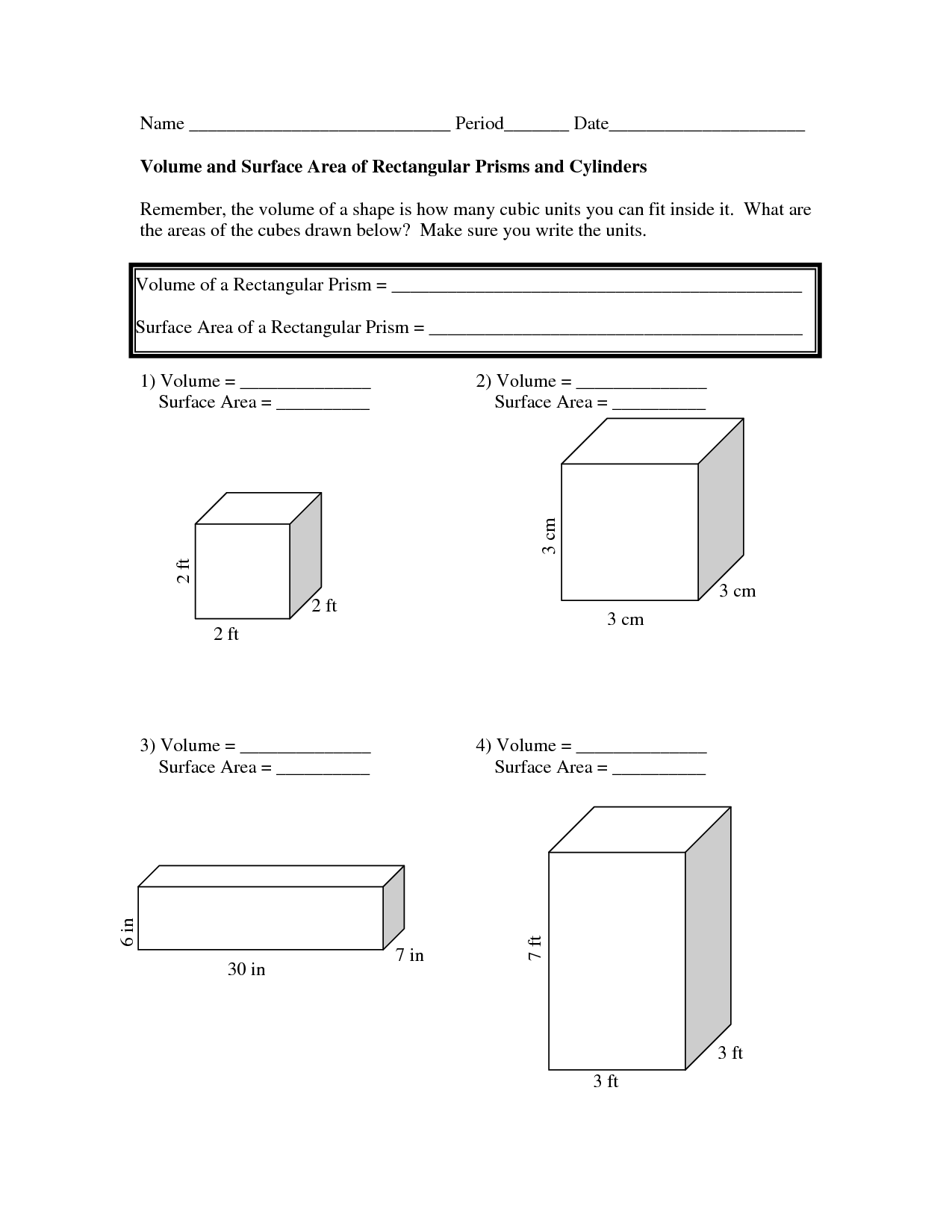
  Prism Surface Area and Volume Worksheet

---

  Surface Area and Volume Worksheets

---

  Volume Prisms and Cylinders Worksheets

---

  Surface Area and Volume of Cones Worksheets

---

What is volume?

Volume is the amount of space that an object or substance occupies. It is typically measured in cubic units, such as cubic meters or cubic centimeters, and can refer to the total amount of three-dimensional space enclosed by an object or container. Volume is an important concept in various fields, including physics, engineering, and mathematics.

How is volume measured?

Volume is typically measured using units like liters, milliliters, cubic centimeters, or gallons, depending on the size and scale of the object being measured. For liquids, volume is often measured by pouring the substance into a graduated cylinder or container with marked increments, and for irregularly shaped objects, it can be determined by measuring the displacement of water when the object is submerged. The volume of regular geometric shapes like cubes, spheres, or cylinders can be calculated using specific mathematical formulas.

What is the formula for calculating the volume of a rectangular prism?

The formula for calculating the volume of a rectangular prism is V = l × w × h, where V represents the volume, l is the length, w is the width, and h is the height of the prism.

How do you find the length, width, and height of a rectangular prism?

To find the length, width, and height of a rectangular prism, you would need to measure the three different dimensions using a ruler or a tape measure. The length is the longest side of the prism, the width is the shorter side, and the height is the distance between the top and bottom faces. Simply measure each of these dimensions accurately to determine the size of the rectangular prism.

How can you determine the volume of an irregularly shaped object using a rectangular prism?

One way to determine the volume of an irregularly shaped object using a rectangular prism is by submerging the object in a container filled with water and measuring the volume of water displaced by the object. The volume of water displaced is equal to the volume of the irregularly shaped object. By then placing the irregularly shaped object into a rectangular prism filled with water, you can measure the volume of water it displaces, which would give you an accurate measurement of the object's volume.

How does the volume of a rectangular prism change when its dimensions are doubled or tripled?

When the dimensions of a rectangular prism are doubled, its volume increases by a factor of 8, as the volume formula for a rectangular prism is volume = length x width x height. Likewise, when the dimensions are tripled, the volume increases by a factor of 27. This is because the volume of a rectangular prism is directly proportional to the product of its dimensions.

How does volume correspond to the capacity of a container?

Volume is directly proportional to the capacity of a container. The volume of a container represents the amount of space inside it that can be filled with a substance, and this directly relates to the capacity of the container in terms of how much liquid or material it can hold. The greater the volume of a container, the larger its capacity will be to accommodate a greater quantity of the substance it is designed to hold.

How can you convert volume from one unit of measurement to another?

To convert volume from one unit of measurement to another, you can use conversion factors specific to the units you are working with. You need to know the relationship between the two units (e.g., how many milliliters are in a liter) and then multiply or divide the original measurement by the appropriate conversion factor to get the desired unit of volume. Practice and familiarity with common conversion factors will make this process easier and faster.

What are some real-life examples of rectangular prisms and their volumes?

A common real-life example of a rectangular prism and its volume is a shipping box. For instance, a shipping box that measures 10 inches in length, 8 inches in width, and 6 inches in height forms a rectangular prism. The volume of this rectangular prism would be calculated by multiplying its three dimensions, resulting in a volume of 480 cubic inches. This demonstrates how rectangular prisms are used in various practical scenarios such as packaging and storage.

Can the volume of a rectangular prism ever be negative?

No, the volume of a rectangular prism cannot be negative. Volume is a measure of the amount of space occupied by an object, and as such, it is always a positive value because it represents a physical quantity that cannot exist in negative form.