Unit Rate Worksheets 6th Grade

Unit rate worksheets are a valuable resource for 6th grade students who are learning about ratios, proportions, and rates. These worksheets provide an opportunity for students to practice calculating unit rates in real-world scenarios, honing their mathematical skills and developing a deeper understanding of the subject matter.

  Unit Rates Worksheet 6th Grade Math

---

  6th Grade Ratio Word Problems Worksheets

---

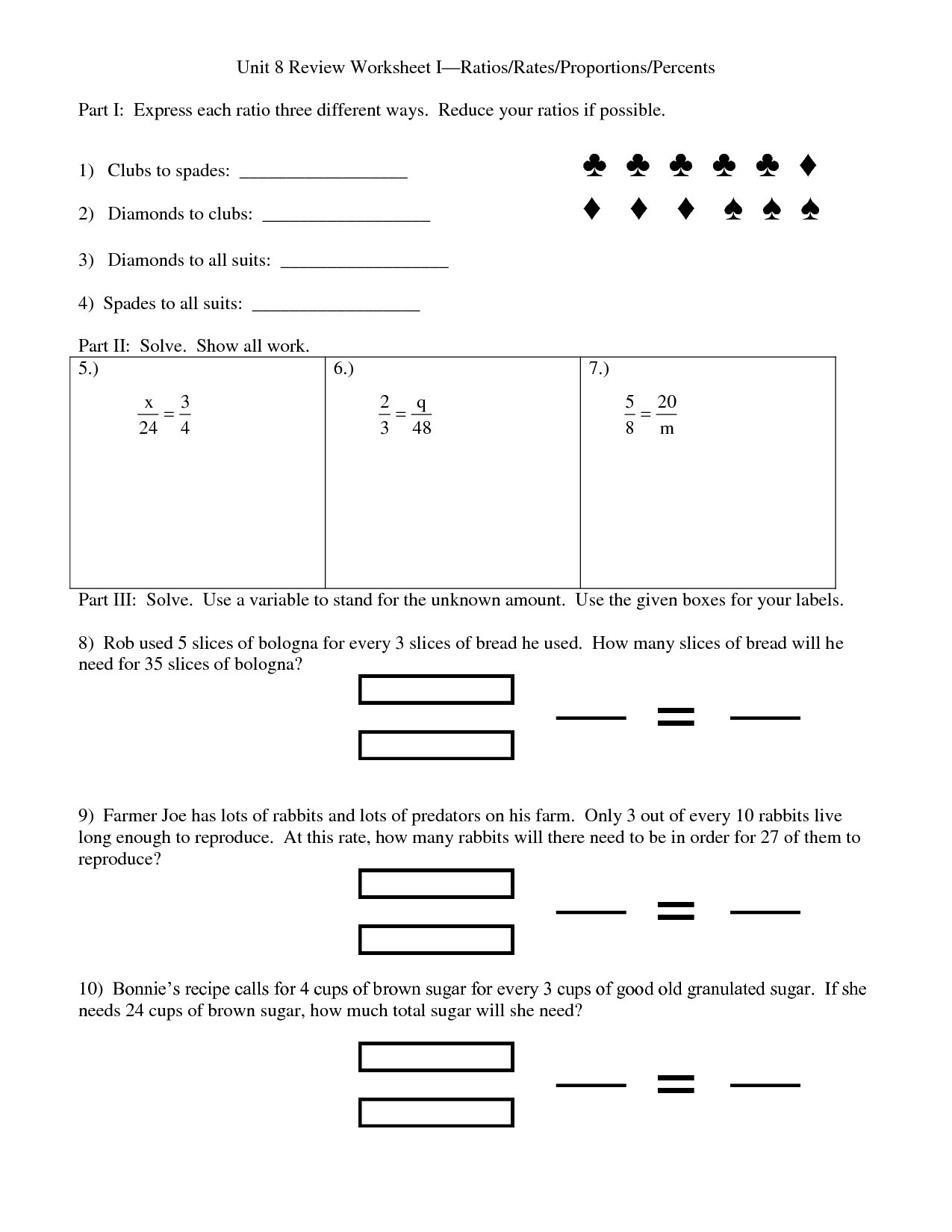
  Ratios and Unit Rates Worksheet 6th Grade

---

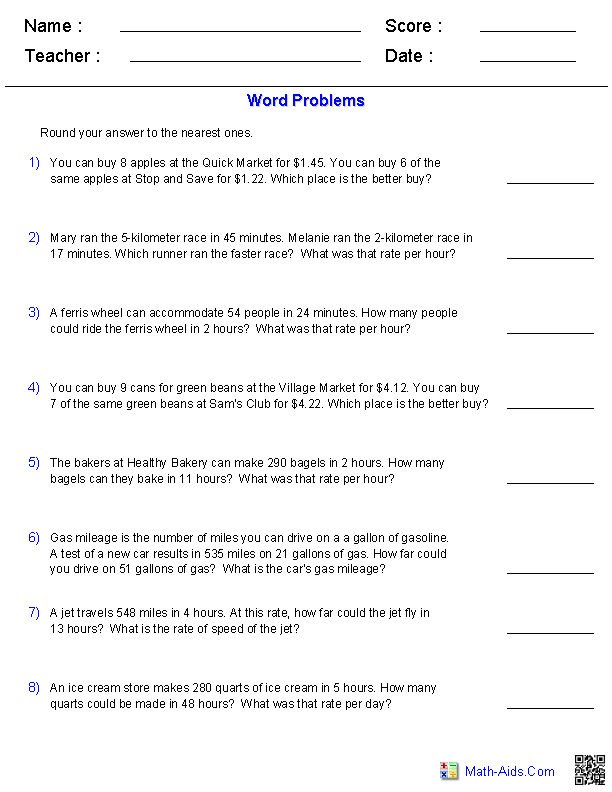
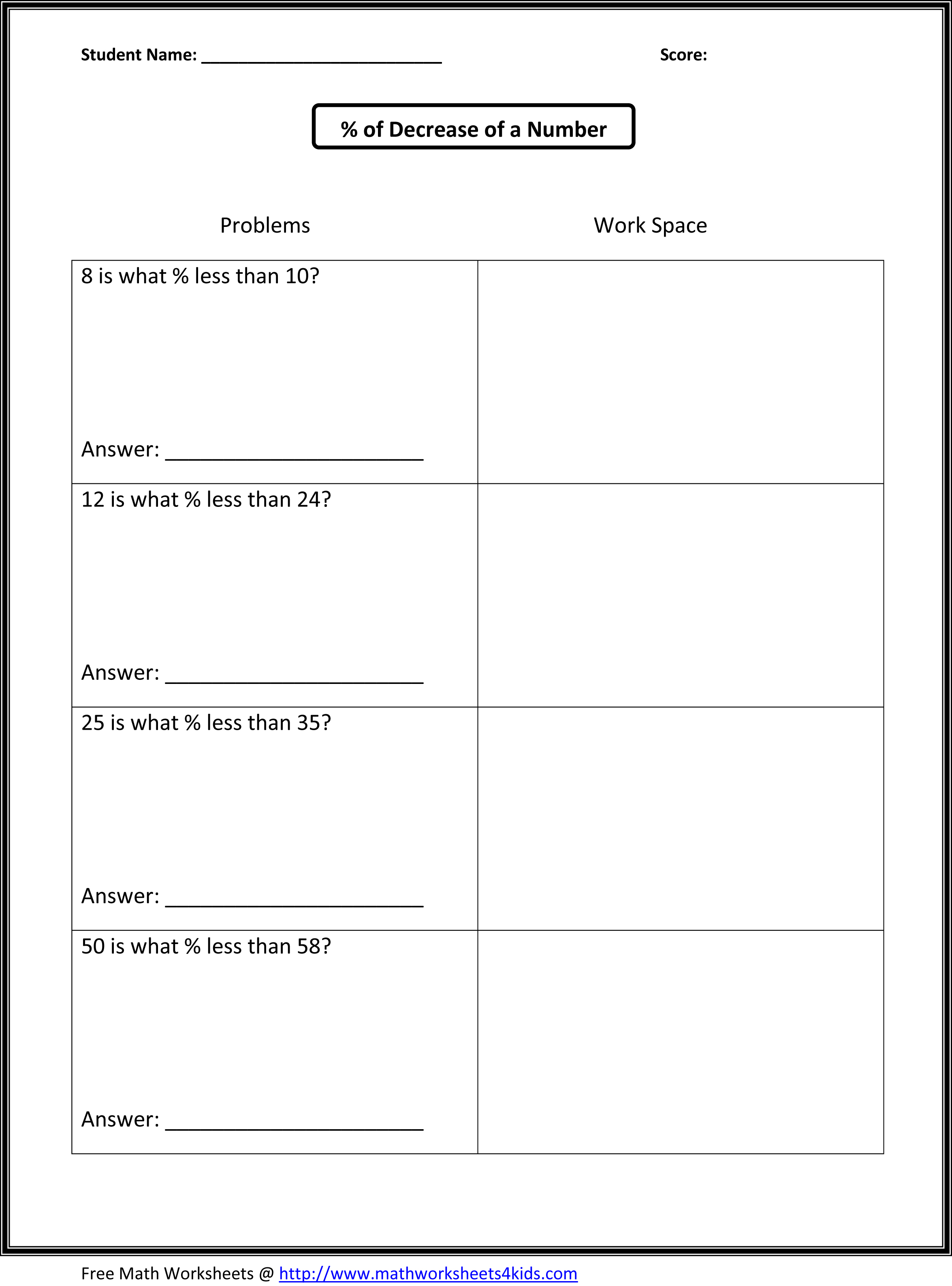
  Unit Rate Word Problems Worksheets 6th Grade

---

  Unit Rate Problems 7th Grade

---

  7th Grade Ratios Worksheet

---

  Metric Conversion Worksheets 6th Grade

---

  Unit Rates Worksheet 7th Grade

---

  Unit Rates Ratios and Proportion Worksheet

---

  Unit Rates Worksheet 7th Grade

---

  6th Grade Math Worksheets Algebra

---

  Ratio Double Number Line Worksheet

---

  Unit Rate Examples Math

---

  7th Grade Ratio Word Problems Worksheets

---

  Ratio and Proportion Worksheets

---

  7th Grade Math Worksheets

---

  Unit Rate Worksheet

---

What is a unit rate?

A unit rate is a comparison of two different quantities measured in different units. It is expressed as a rate of one unit of one quantity to another unit of a different quantity, making it easier to compare prices, speeds, or any other measurement that involves two different units.

How is a unit rate different from a rate?

A unit rate is a type of rate that compares a quantity to one unit of another quantity, such as miles per hour or cost per item. It expresses the relationship between two different units of measurement. On the other hand, a rate is a ratio that compares two quantities with different units, such as kilometers per hour or dollars per hour. While both unit rates and rates involve comparing quantities, a unit rate specifically compares the quantities to one unit of another quantity, making it more standardized and easier to compare across different scenarios.

Give an example of finding a unit rate.

Sure! Let's say you are buying 4 pounds of apples for $6. To find the unit rate (price per pound), you would divide the total cost ($6) by the number of pounds (4). In this case, the unit rate would be $6 divided by 4 pounds, which equals $1.50 per pound.

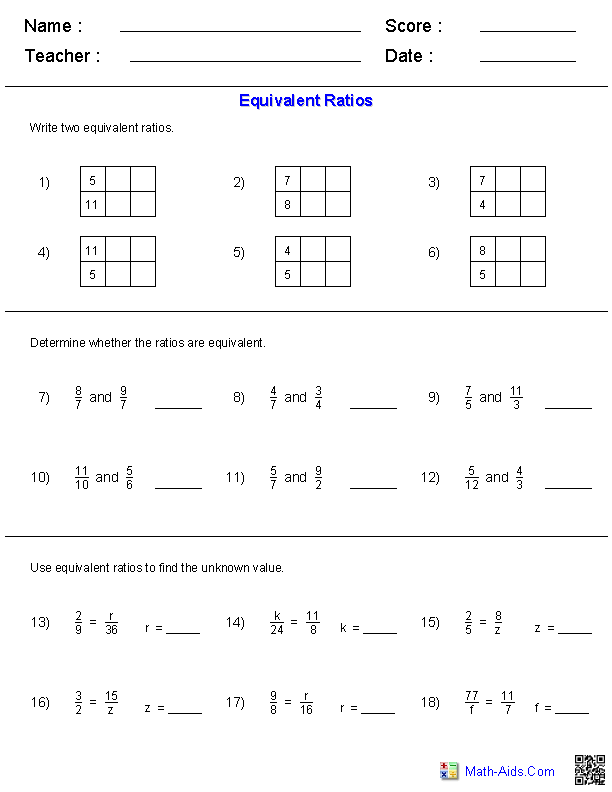
How can ratios be used to calculate a unit rate?

To calculate a unit rate using ratios, you simply divide one quantity by another in order to determine how much of one quantity corresponds to one unit of another. This can be particularly helpful when comparing different quantities or prices. By setting up a ratio and dividing the quantities, you can find the rate at which one item corresponds to another or determine the cost per unit of a product.

Explain how to convert a rate into a unit rate.

To convert a rate into a unit rate, you simply need to divide the given rate by the denominator in the rate. This will give you the amount of the quantity being measured for each unit of the denominator. For example, if the rate is 120 miles in 3 hours, you would divide 120 by 3 to obtain the unit rate of 40 miles per hour. This allows you to easily compare the rate across different denominators and calculate the quantity for a single unit of the denominator.

What is the purpose of using unit rates in real-life situations?

The purpose of using unit rates in real-life situations is to easily compare prices, quantities, or rates of change between different items or situations. Unit rates allow for a standardized measurement that can help in making informed decisions when comparing products, services, or other variables. By using unit rates, individuals can determine the best value for their money, make efficient use of resources, and understand the relationships between different quantities or rates within a given context.

How can unit rates be used to compare prices?

Unit rates can be used to compare prices by calculating the cost of a single unit of a product or service. By determining the cost per unit, such as cost per ounce or cost per kilogram, it becomes easier to compare prices of similar items regardless of differences in packaging or quantity. This allows consumers to make more accurate comparisons and choose the most cost-effective option when making purchasing decisions.

Give an example of using unit rates to solve a problem involving speed.

Sure, let's say a car is traveling at a constant speed of 60 miles per hour. To calculate how far the car will travel in 3 hours, we can use a unit rate by dividing the speed by the time traveled: 60 miles per hour ÷ 1 hour = 60 miles. Therefore, in 3 hours, the car will travel 60 miles/hour x 3 hours = 180 miles.

How can unit rates be used to determine the best deal when shopping?

Unit rates can be used to determine the best deal when shopping by comparing the cost per unit of different products. By calculating the unit rate (e.g. cost per ounce, cost per pound), you can accurately compare prices and identify which product offers the best value for money. This allows you to make informed decisions and choose the option that provides the most quantity or quality for the price, ultimately helping you save money and get the best deal while shopping.

Explain the steps to find a unit rate when given a word problem.

To find a unit rate in a word problem, first identify the two quantities involved, such as distance and time or cost and number of items. Then, divide the total quantity of the first variable by the total quantity of the second variable to get the rate. Make sure both quantities have the same unit of measure for accurate comparison. Finally, simplify the ratio to its most basic form to express the unit rate, which represents the amount of the first quantity per one unit of the second quantity.