Triangular Pyramid Volume Worksheet
Are you a math teacher looking for a resource to improve your students' understanding of volume calculations for triangular pyramids? Look no further - in this blog post, we will provide you with a comprehensive triangular pyramid volume worksheet that covers all the essential concepts and exercises needed for your students to excel in this topic. With carefully selected problems and clear instructions, this worksheet is designed to engage and challenge your students while reinforcing their knowledge of calculating the volume of triangular pyramids.
Table of Images 👆
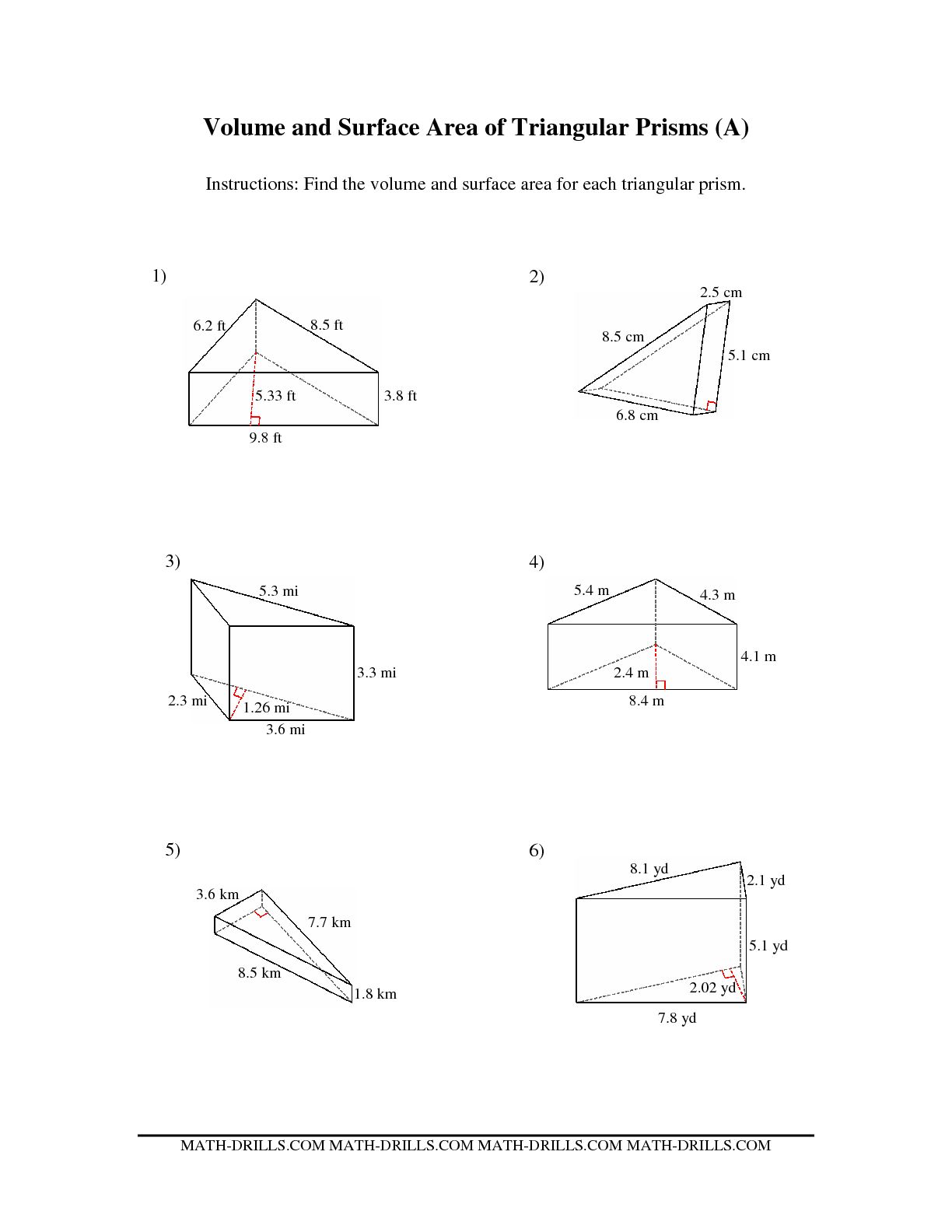
Triangular Prism Surface Area Worksheet
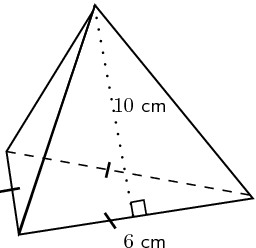
Triangular Pyramid Surface Area
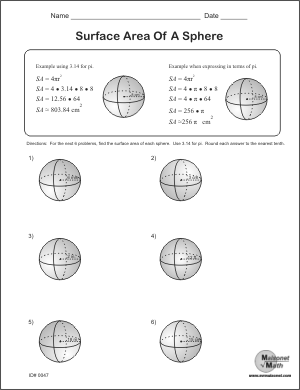
Surface Area Cone Sphere Worksheet
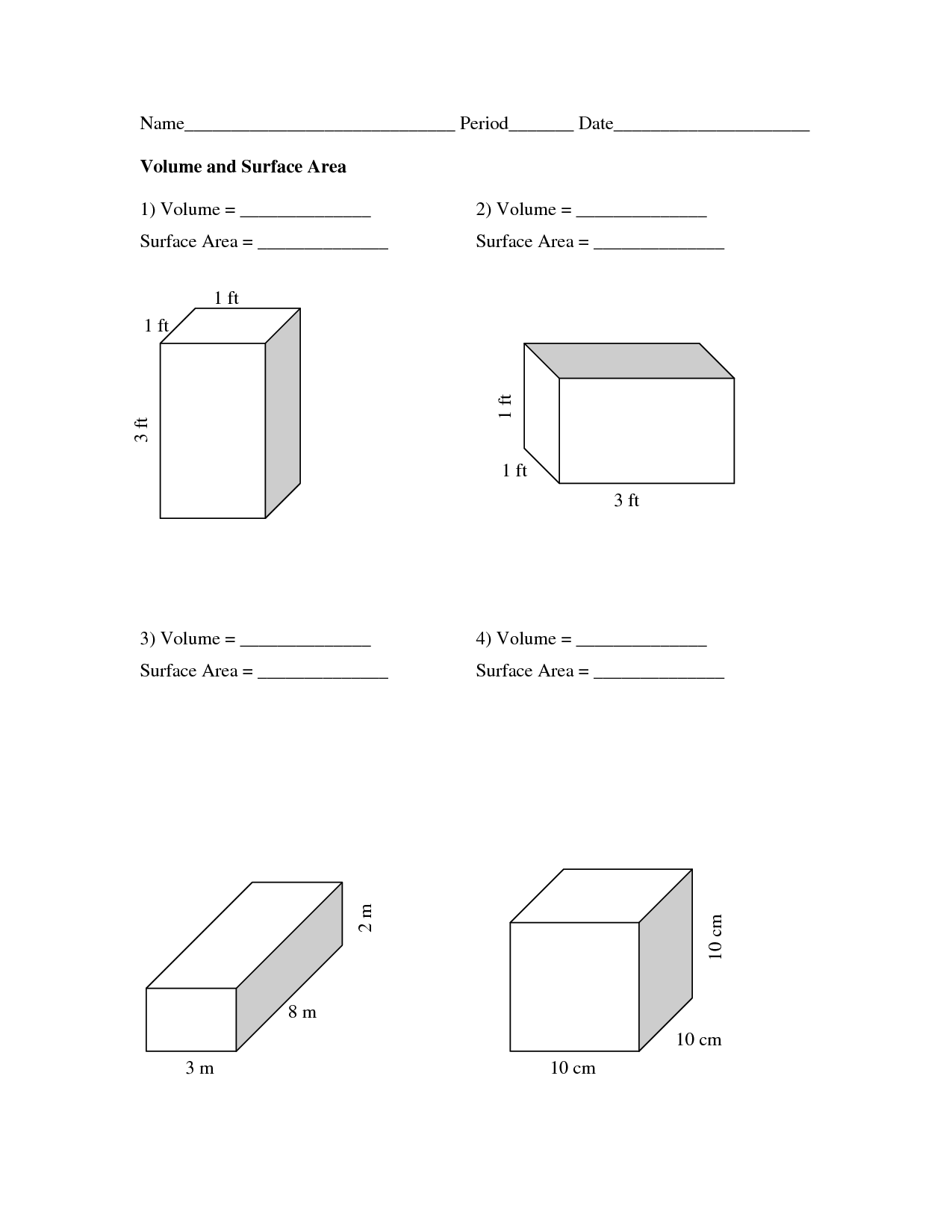
Surface Area and Volume Worksheets
Surface Area and Volume of Cones Worksheets
Volume Prisms and Pyramids Worksheet
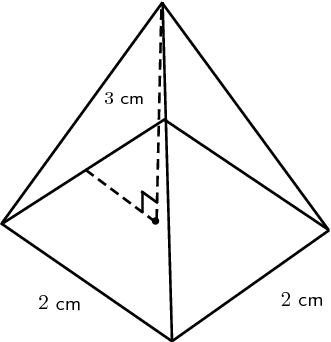
Volume Square Pyramid
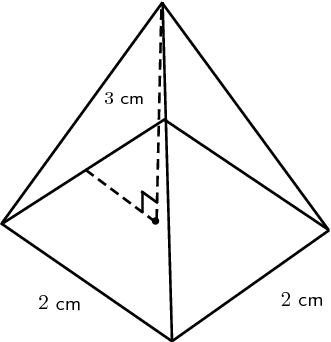
Volume Square Pyramid
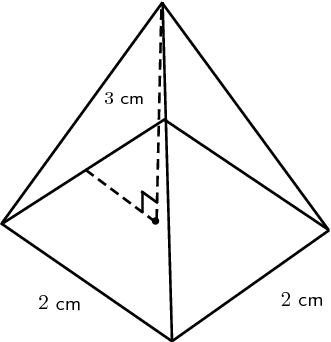
Volume Square Pyramid
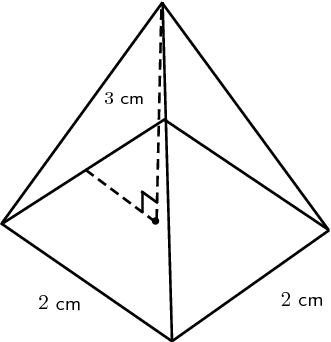
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
Volume Square Pyramid
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
All Amendment Worksheet
Symmetry Art Worksheets
Daily Meal Planning Worksheet
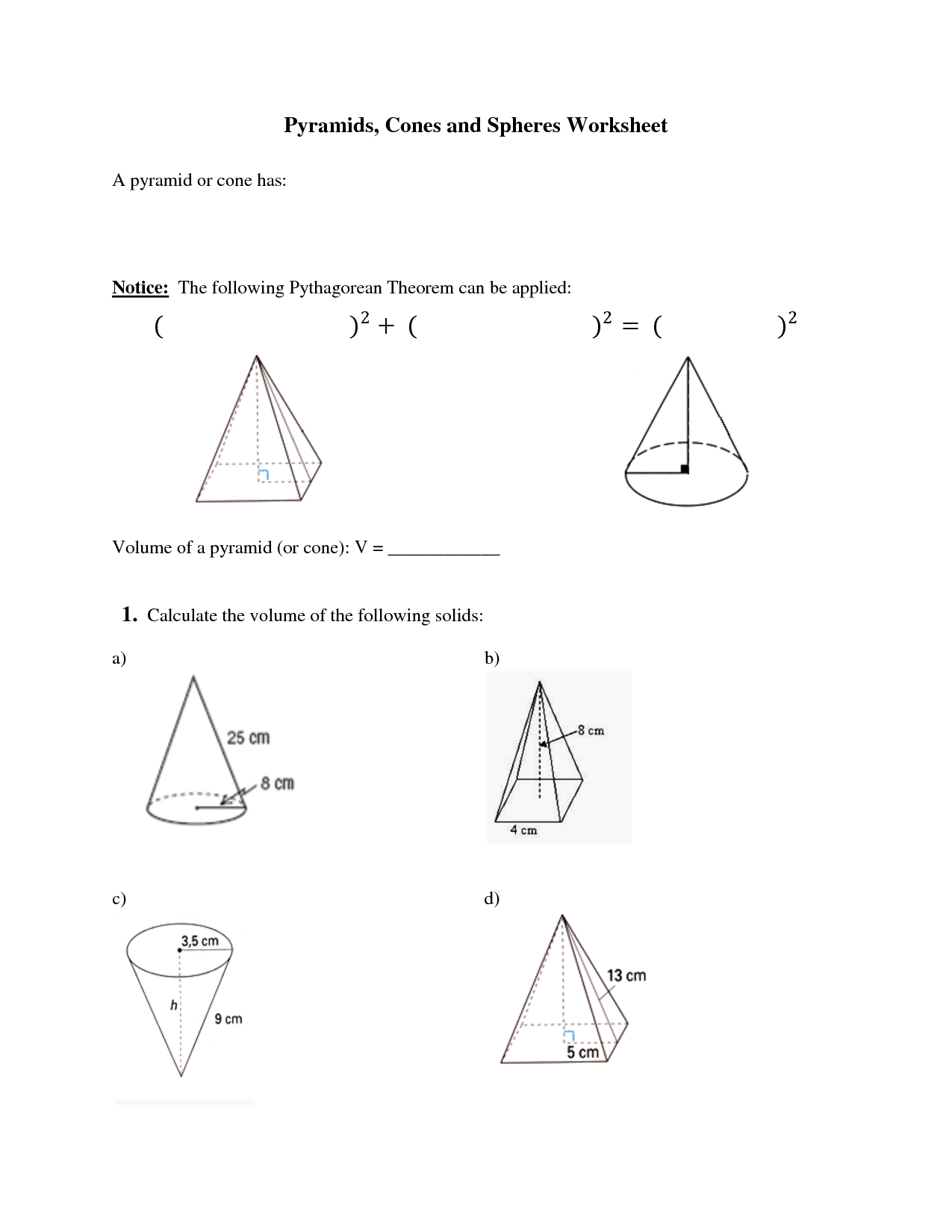
What is the formula for calculating the volume of a triangular pyramid?
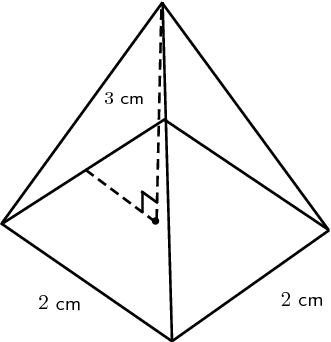
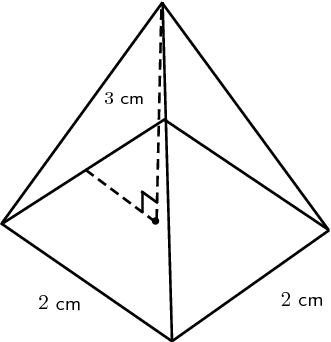
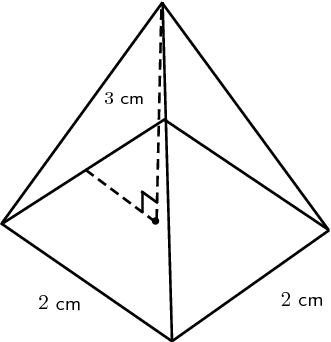
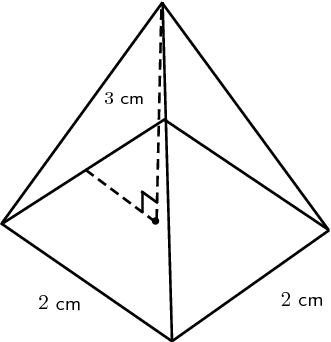
The formula for calculating the volume of a triangular pyramid is V = (1/3) x Base Area x Height, where the Base Area is the area of the triangular base and the Height is the perpendicular distance between the base and the apex of the pyramid.
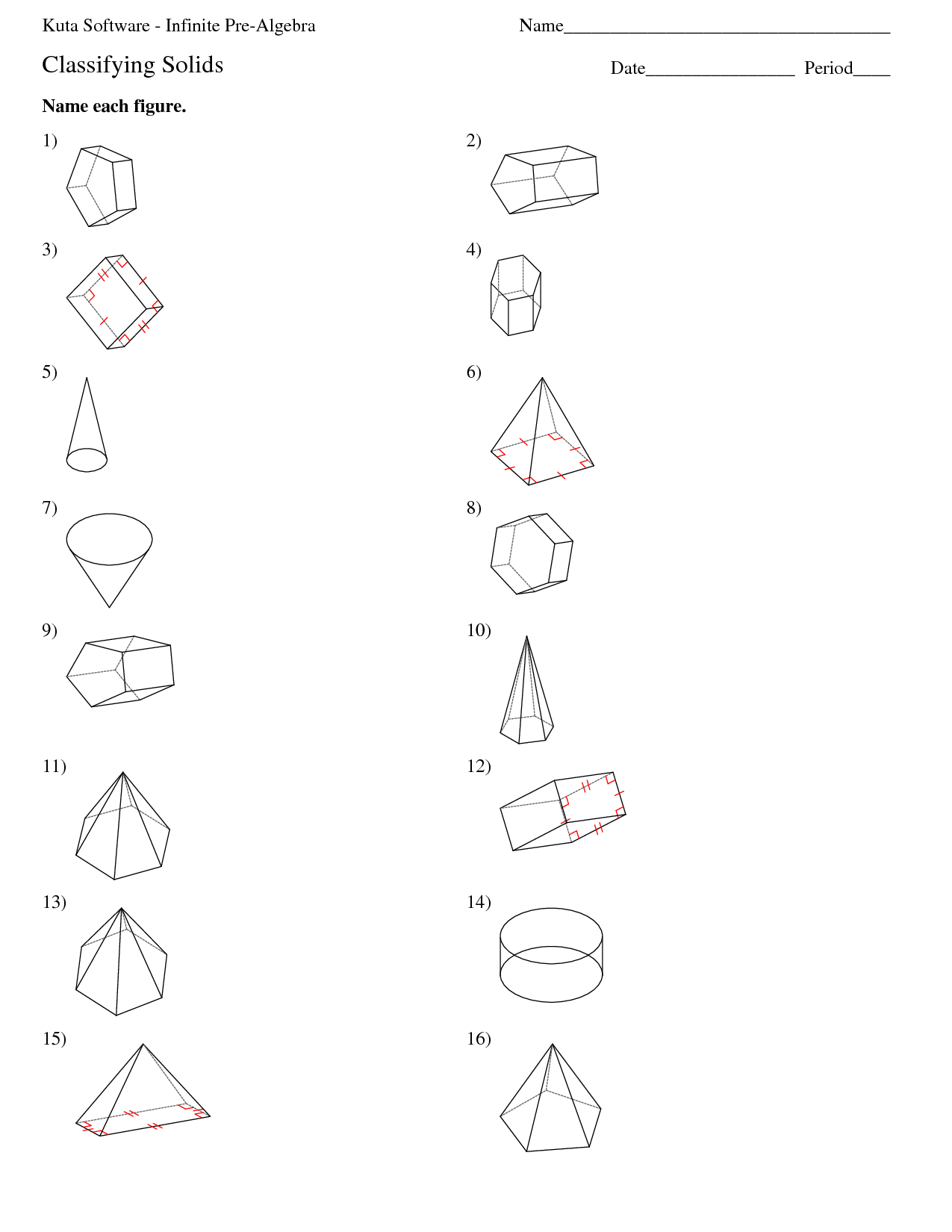
How many faces does a triangular pyramid have?
A triangular pyramid has four faces: one base that is a triangle and three triangular faces that meet at the apex of the pyramid.
How many vertices does a triangular pyramid have?
A triangular pyramid has 4 vertices.
How many edges does a triangular pyramid have?
A triangular pyramid has a total of 6 edges.
What type of shape does the base of a triangular pyramid have?
The base of a triangular pyramid has a triangular shape.
How is the height of a triangular pyramid measured?
The height of a triangular pyramid is measured as the perpendicular distance from the base of the pyramid to the apex (top) of the pyramid. This measurement helps determine the overall vertical height of the pyramid, which is essential for various geometric calculations and applications.
Can a triangular pyramid have different side lengths for each face?
No, a triangular pyramid must have congruent base triangles with equal side lengths in order to form a uniform structure. This ensures that the faces of the pyramid have equal side lengths, making it a regular triangular pyramid. If the base triangles had different side lengths, the pyramid would not be geometrically consistent and would not form a proper triangular pyramid.
Can a triangular pyramid have different angles for each face?
No, a triangular pyramid has three triangular faces, and by definition, all triangles have interior angles that add up to 180 degrees. Therefore, each face of a triangular pyramid must have the same angles, with the base being an equilateral triangle and the other two faces being isosceles triangles.
How does increasing the base size affect the volume of a triangular pyramid?
Increasing the base size of a triangular pyramid will result in an increase in its volume. This is because the volume of a pyramid is directly proportional to the area of its base. Therefore, a larger base will lead to a greater volume as the height remains constant.
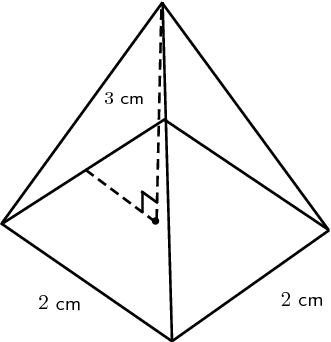
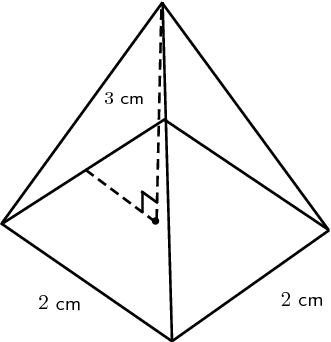
Can you find the volume of a triangular pyramid if you only know the side lengths of the base and the height?
Yes, you can find the volume of a triangular pyramid if you know the side lengths of the base and the height. The volume of a triangular pyramid is given by the formula V = (1/3) * base area * height, where the base area is calculated as (1/4) * base side length * height of the equilateral triangle formed by the base. By substituting the given side lengths of the base and the height into these formulas, you can calculate the volume of the triangular pyramid.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.
Comments