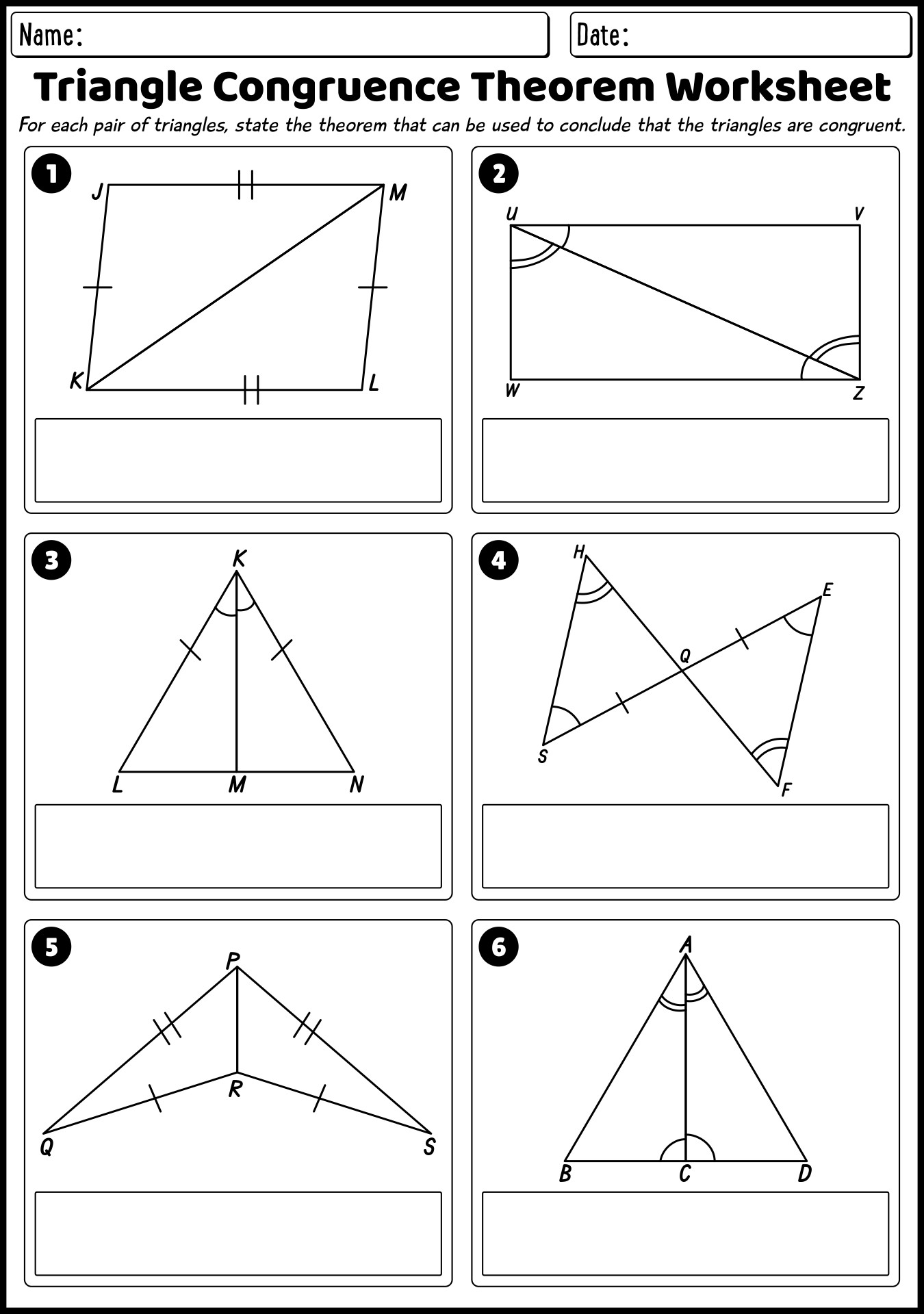
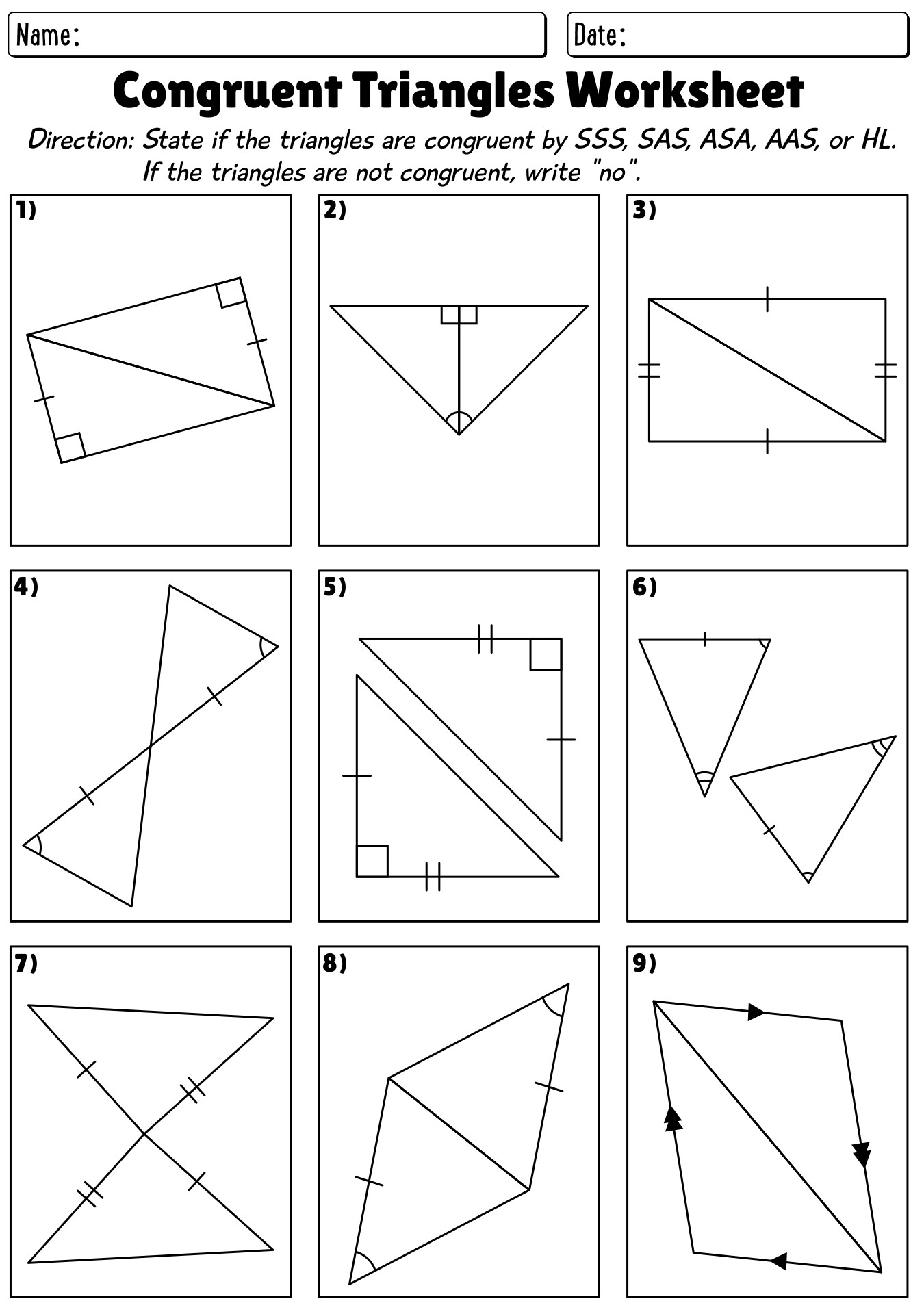
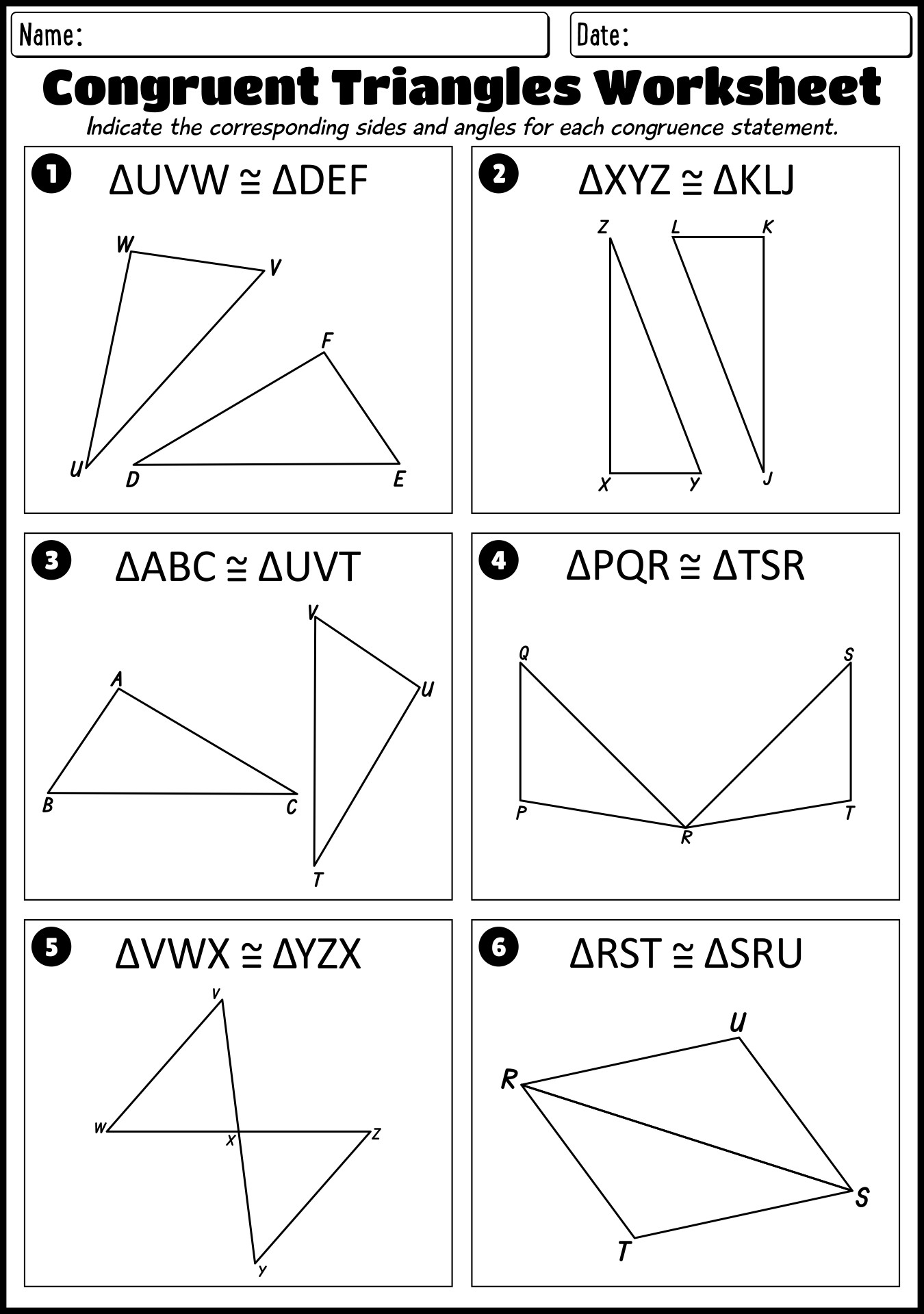
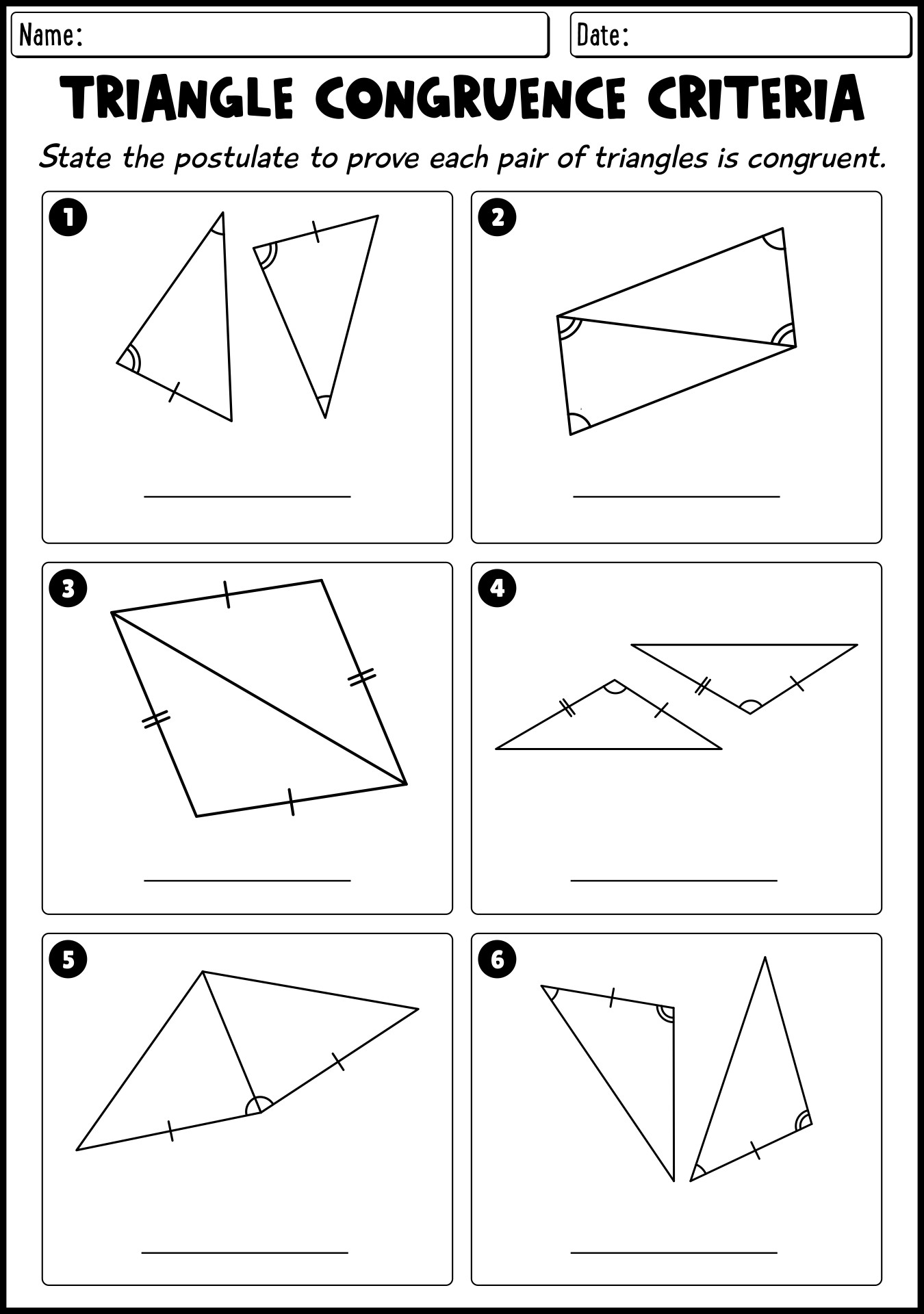
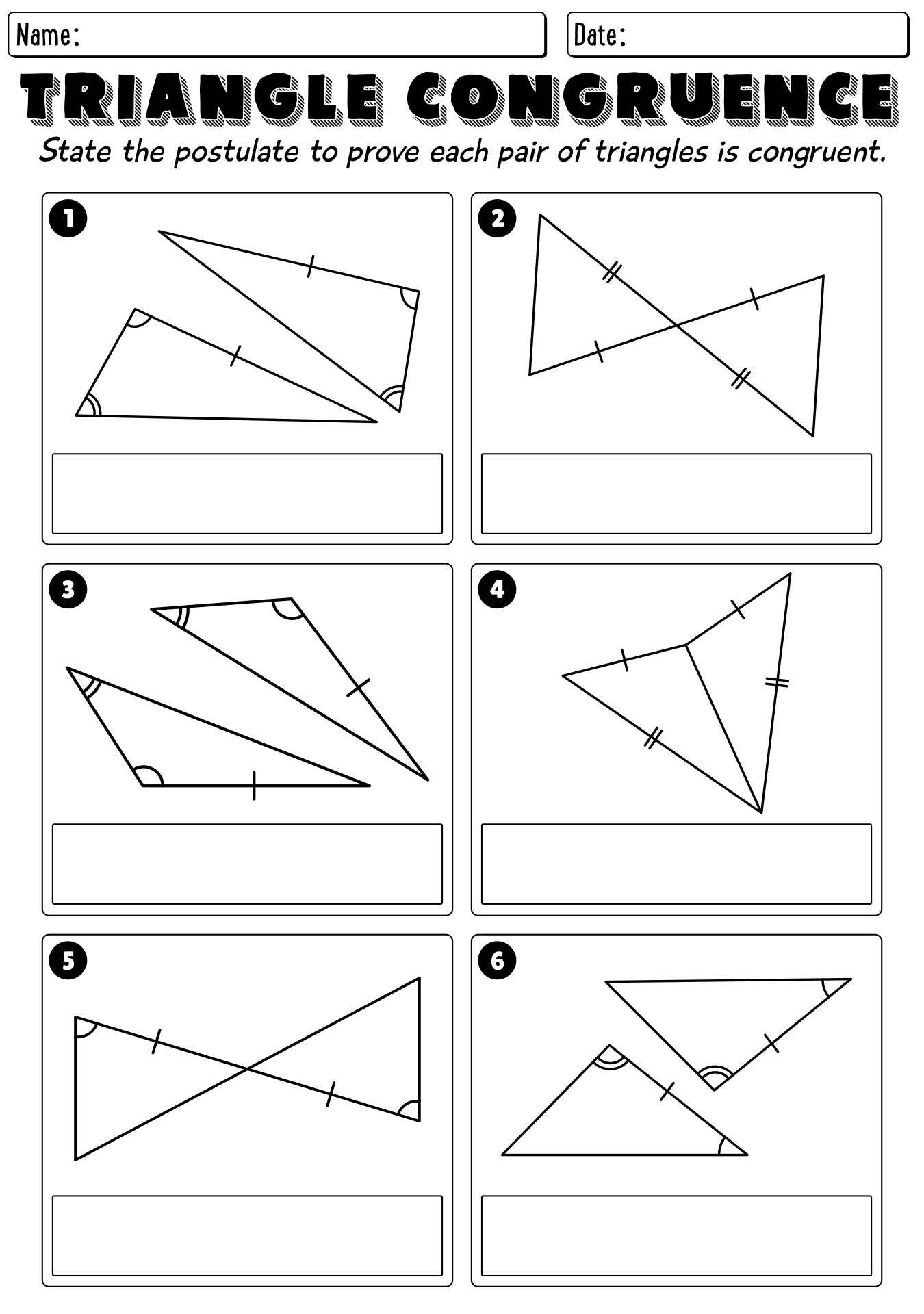
Triangle Congruence Theorems Worksheet

For students learning about triangle congruence theorems, an effective way to reinforce and test their understanding is through the use of worksheets. These worksheets provide students with the opportunity to practice applying the theorems to various types of triangles and engaging with real-world examples.

  15 Images of Triangle Congruence Theorems Worksheet

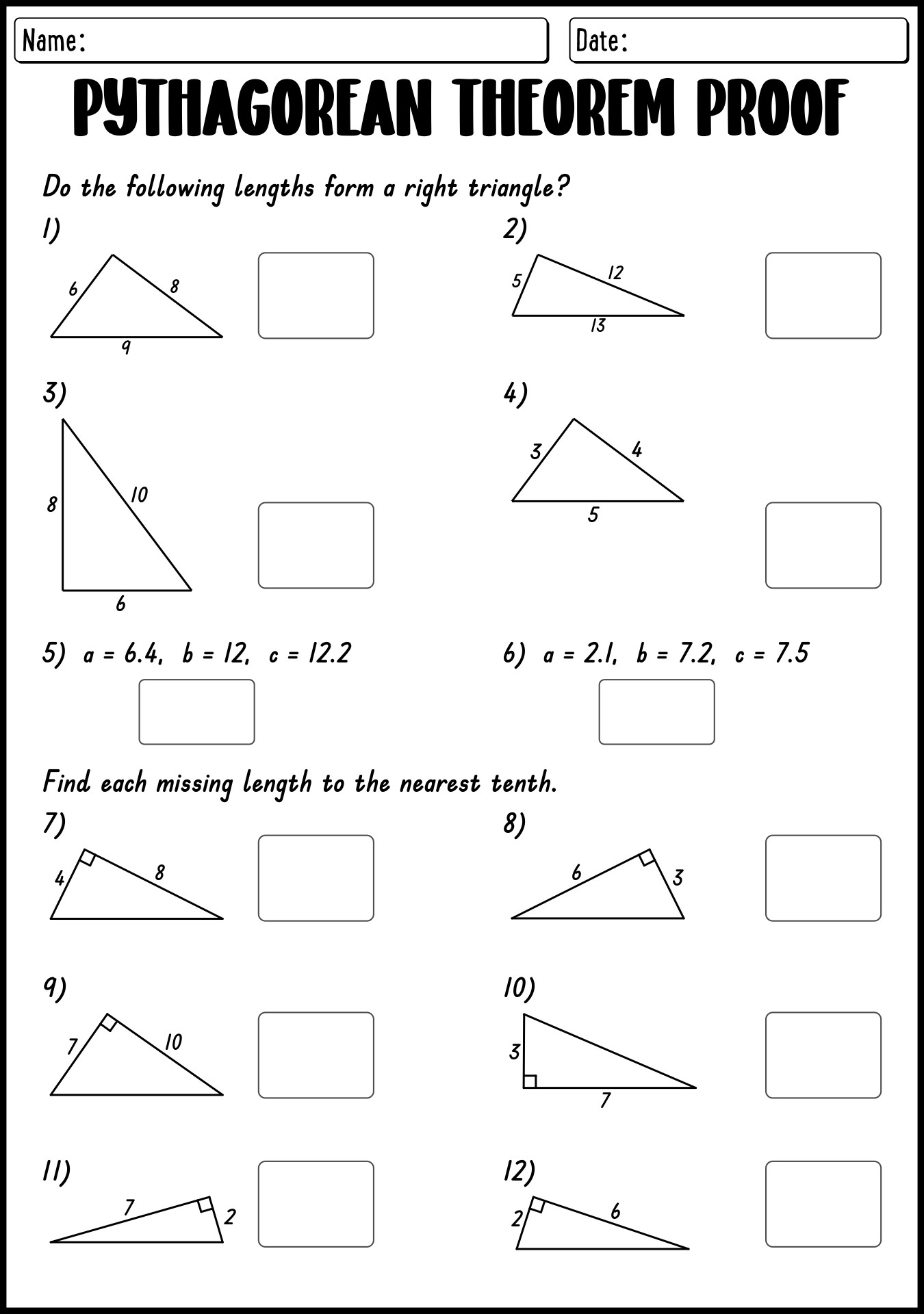
  Pythagorean Theorem Proof

---

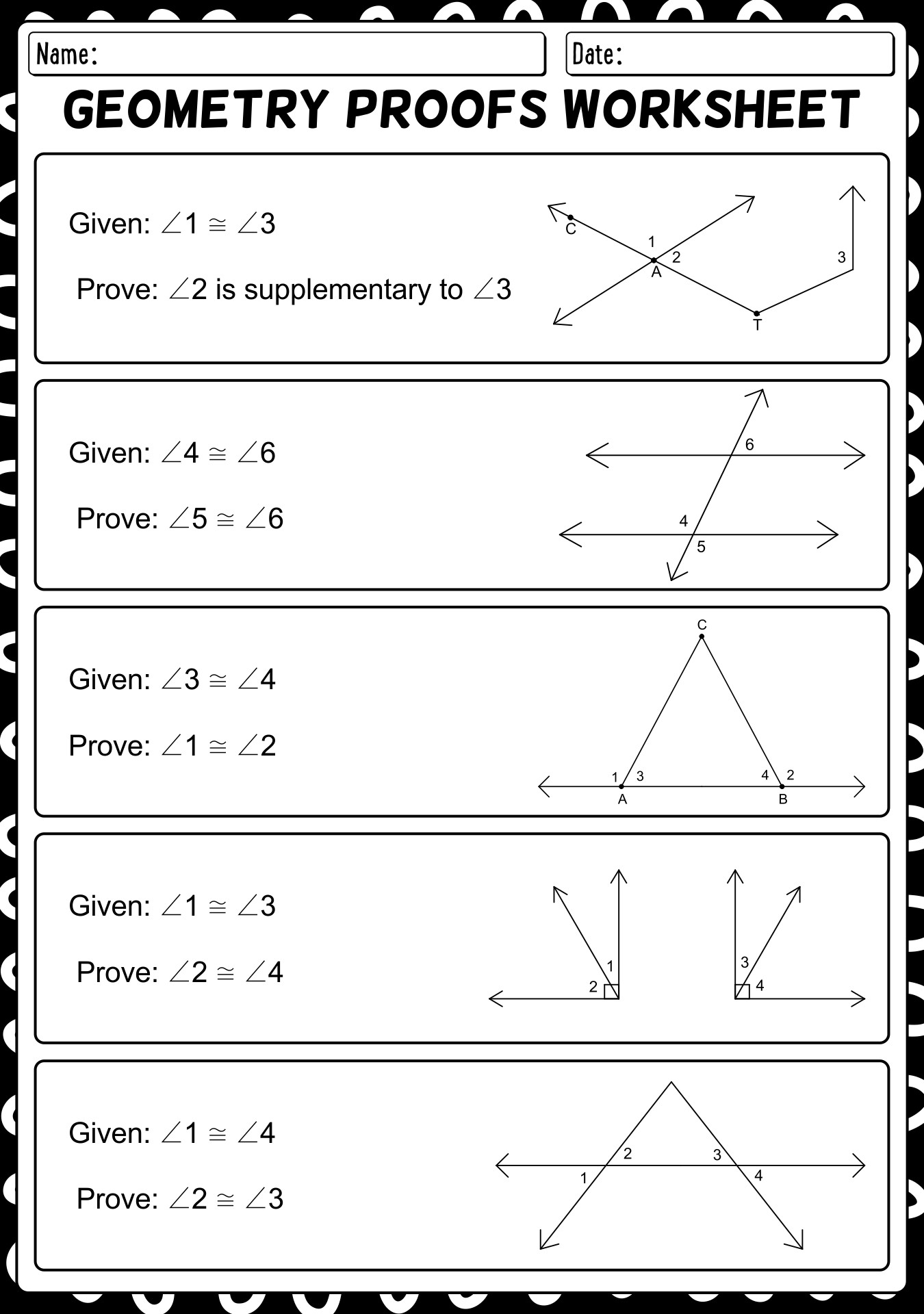
  High School Geometry Proofs Worksheets

---

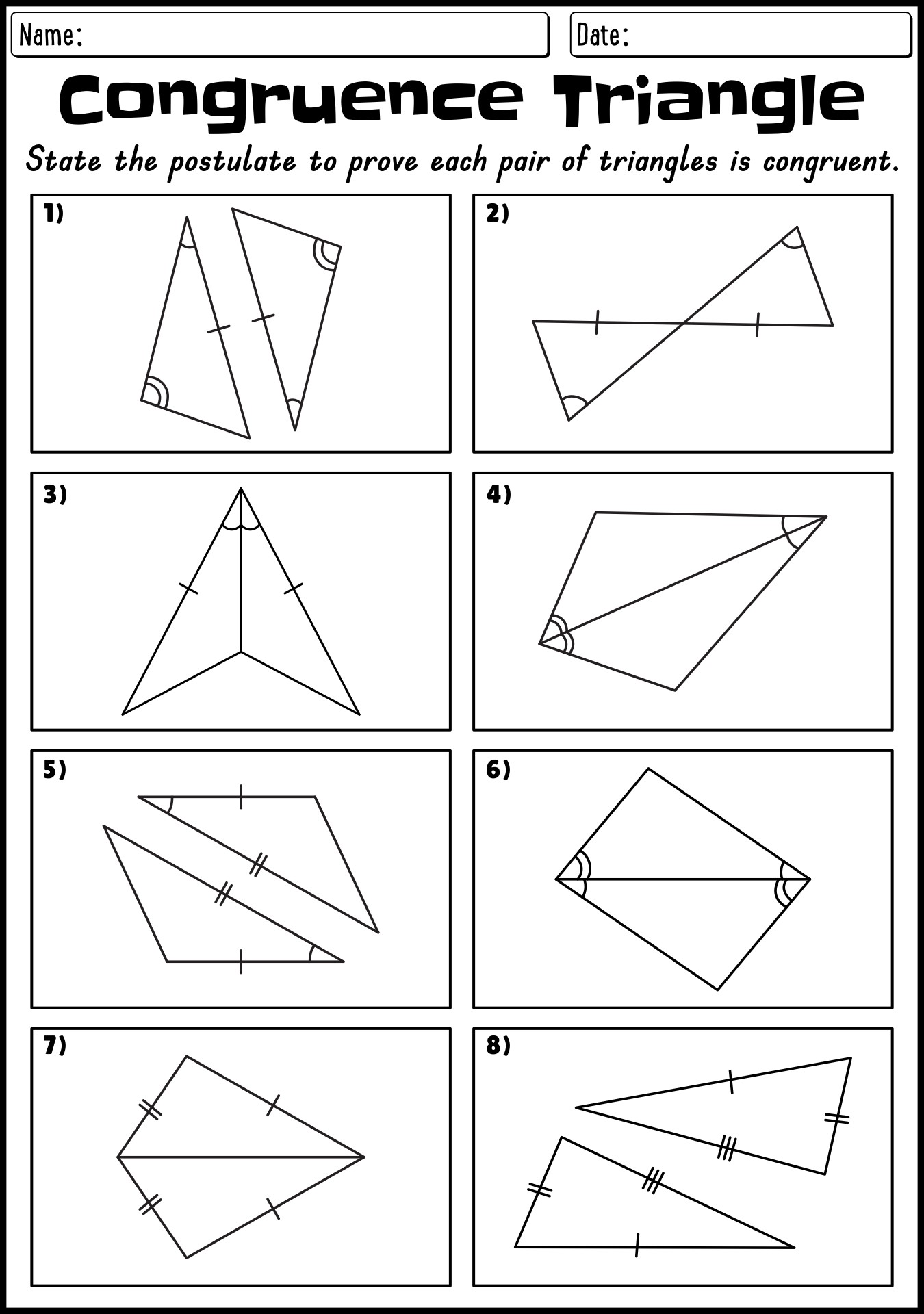
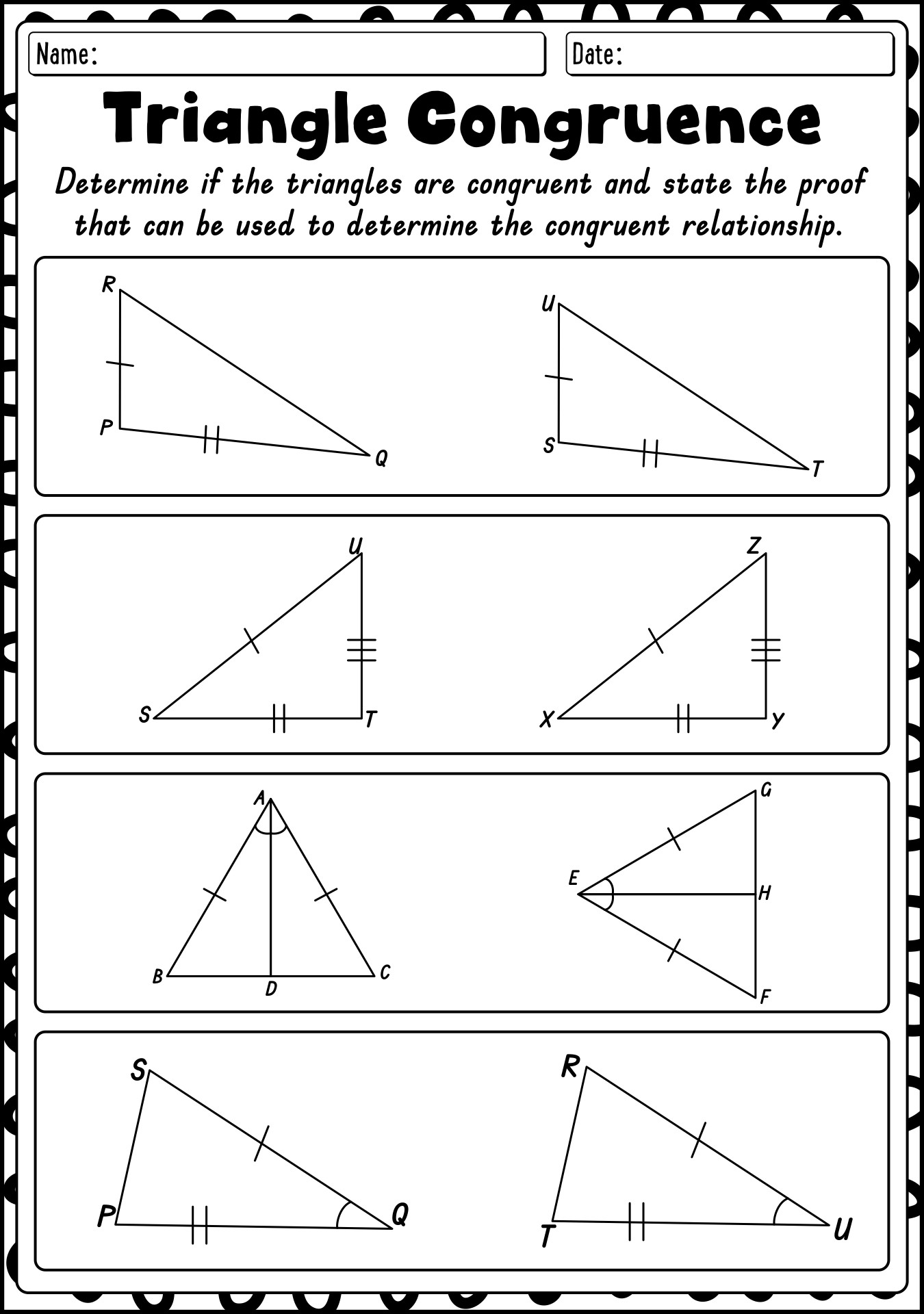
  Geometry Triangle Congruence Worksheet Exercises

---

  Triangle Congruence Theorems Worksheet

---

  Comprehensive Worksheet Triangle Congruence Theorems

---

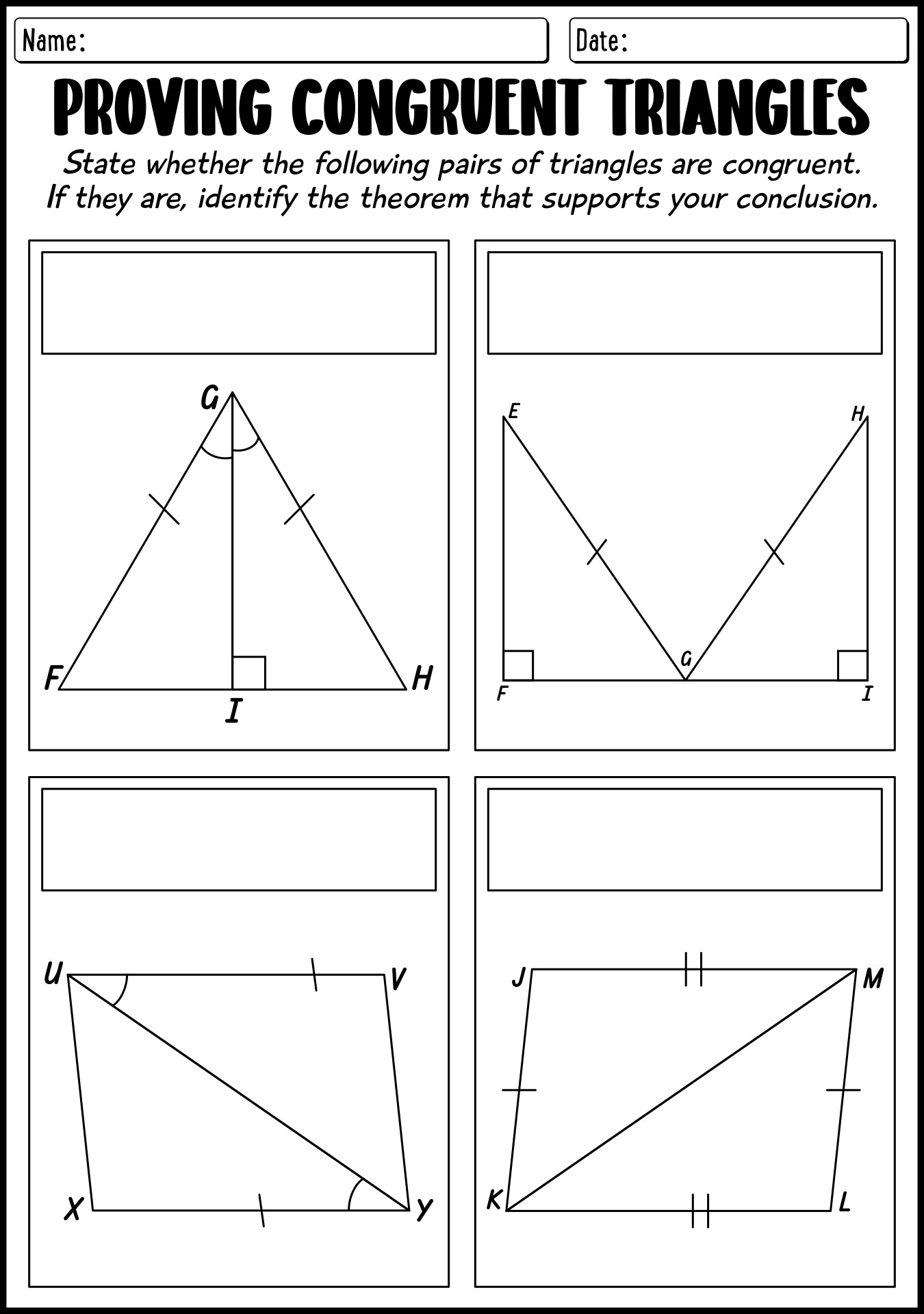
  Worksheet On Proving Triangle Congruence

---

  Triangle Congruence Theorems Practice Worksheet

---

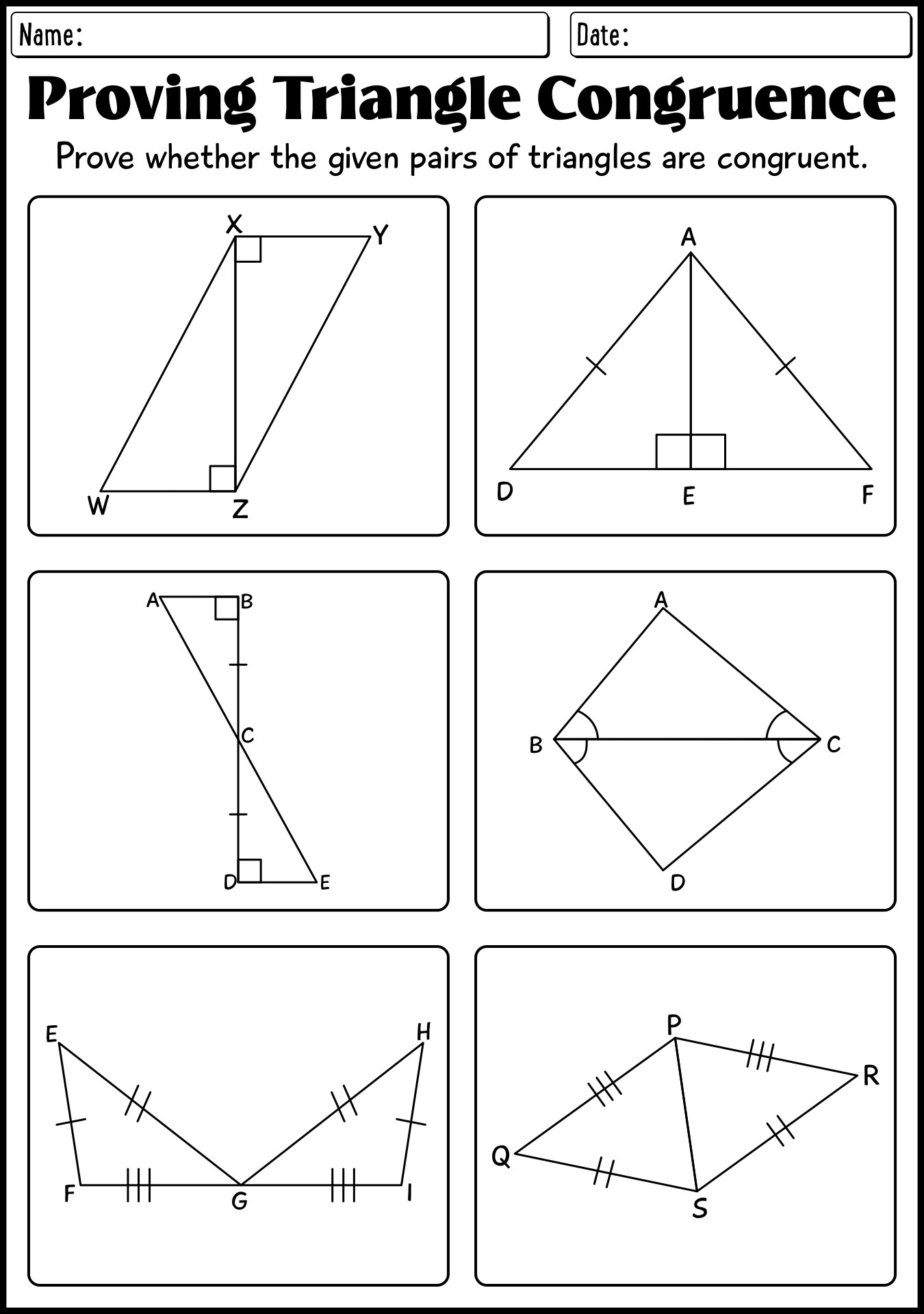
  Triangle Congruence Proofs Worksheet

---

  High School Triangle Congruence Theorems Worksheet

---

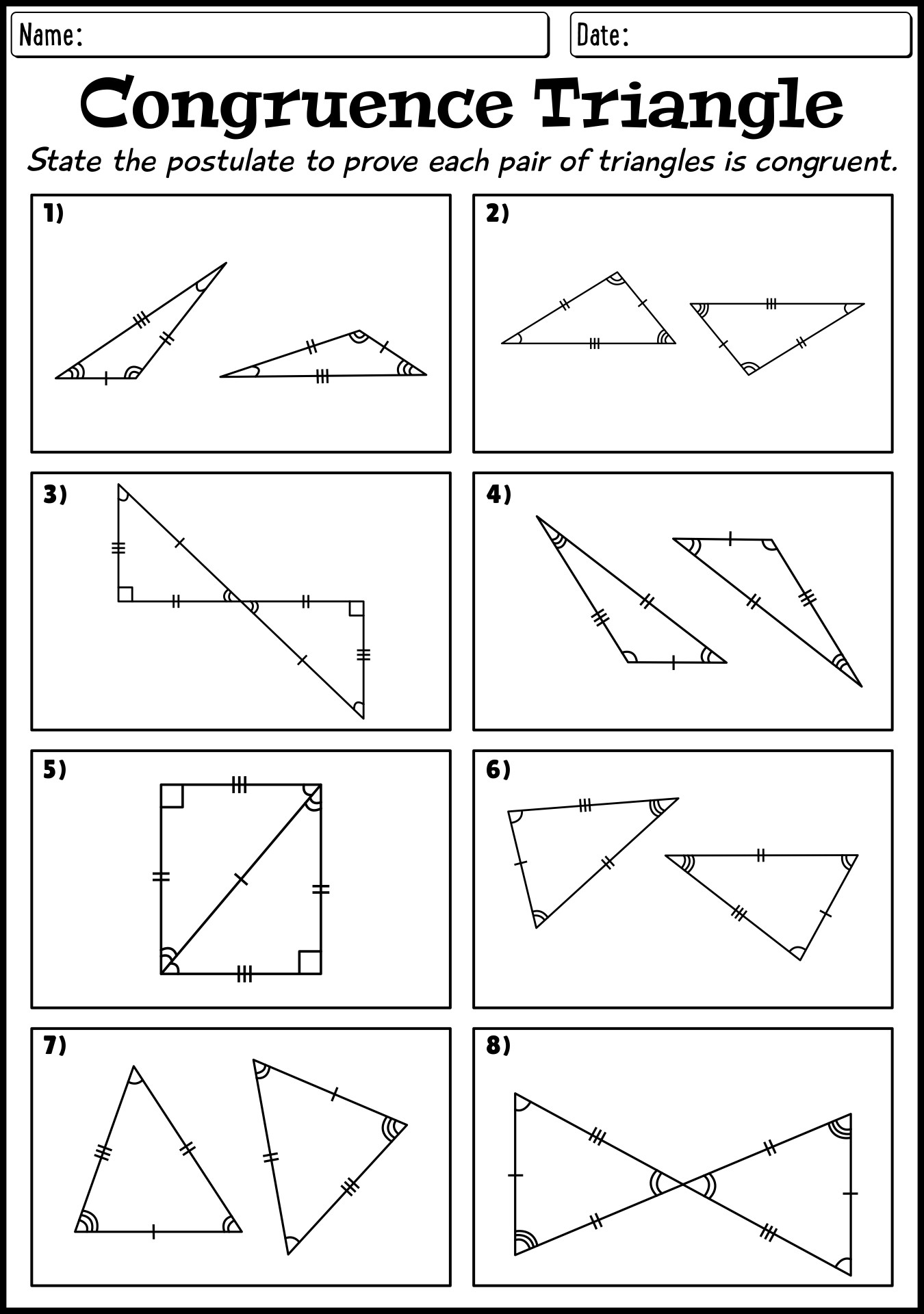
  Triangle Congruence Criteria Worksheet

---

  Worksheet Triangle Congruence Theorems

---

  Triangle Congruence Theorems Worksheet Classwork

---

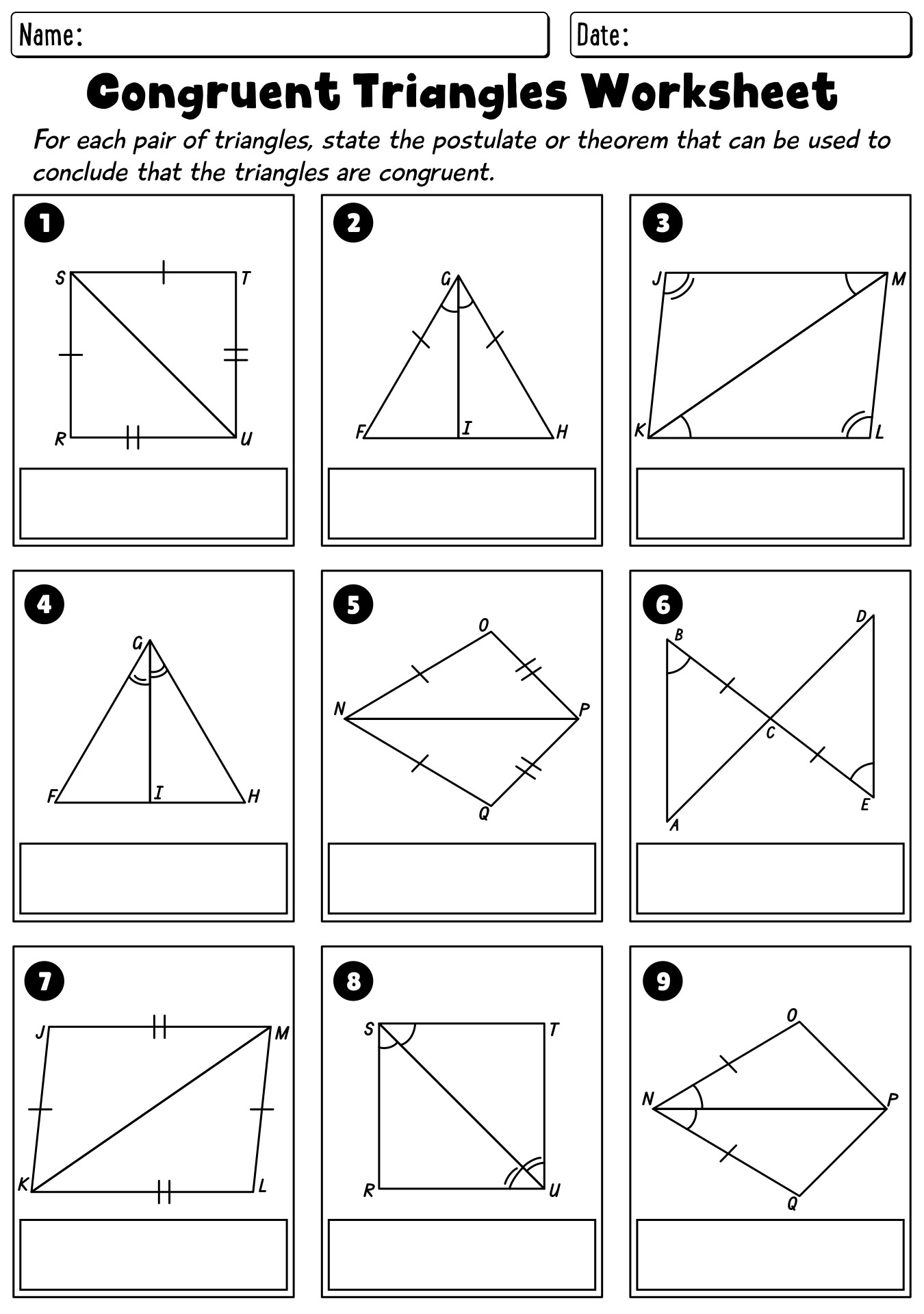
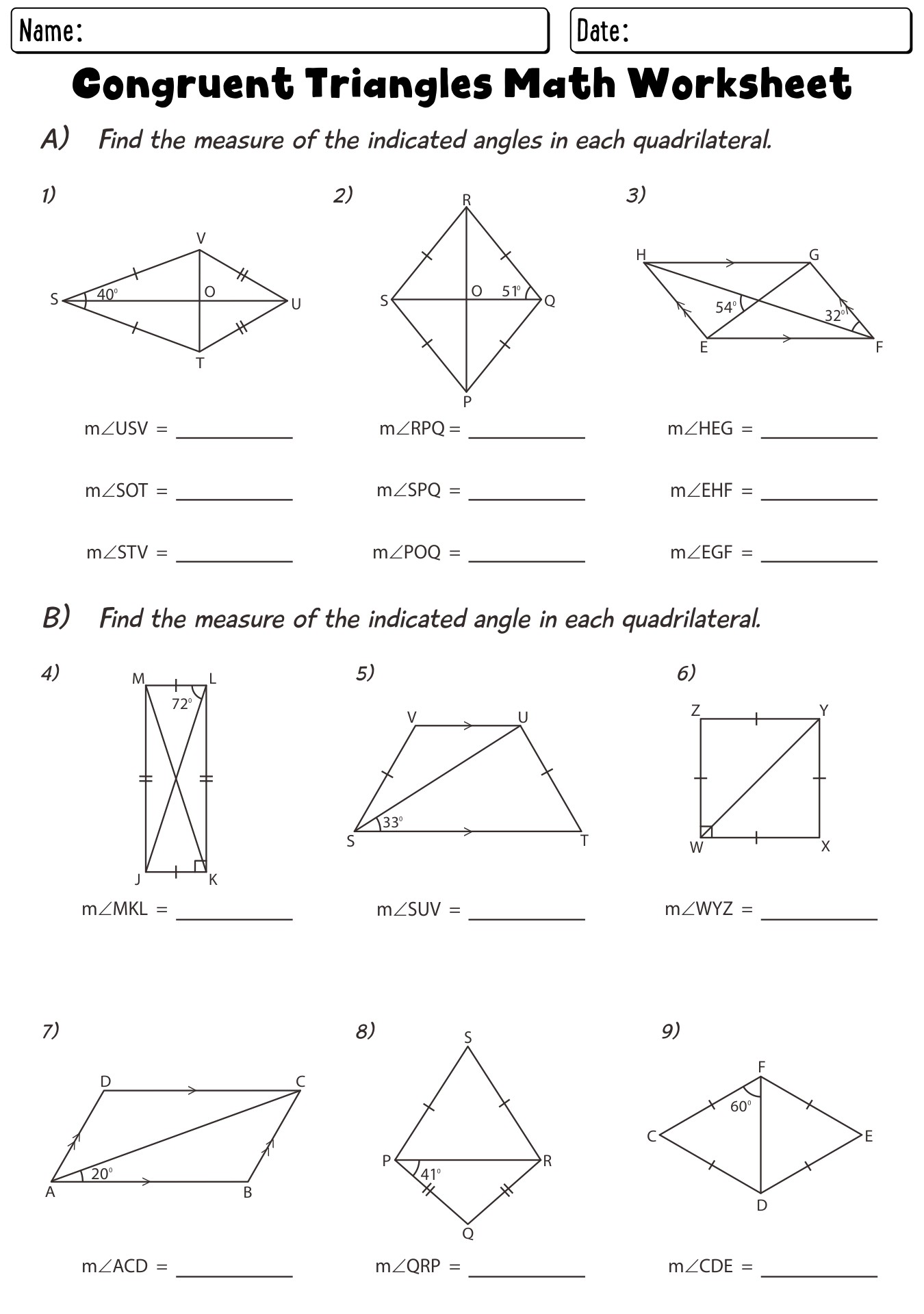
  Congruent Triangles Math Worksheets

---

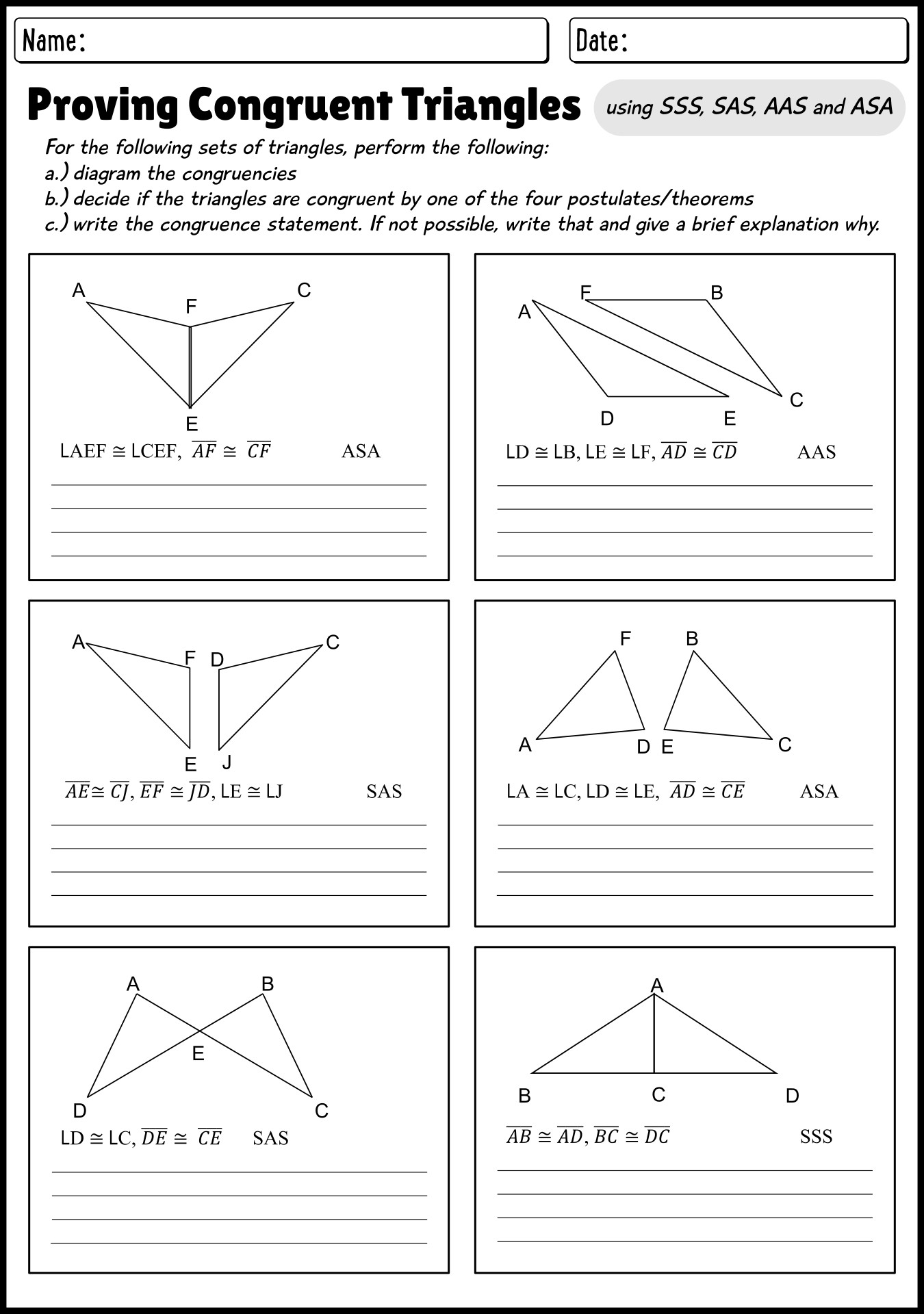
  Proving Triangle Congruence Worksheets

---

  Triangle Congruence Proofs Worksheets

---

Understanding triangle congruence theorems is crucial, and our triangle congruence theorems worksheet provides an excellent resource to support your learning.

What are the triangle congruence theorems?

The triangle congruence theorems are the rules that determine when two triangles are congruent, meaning they have the same size and shape. The four main congruence theorems are Side-Side-Side (SSS), Side-Angle-Side (SAS), Angle-Side-Angle (ASA), and Angle-Angle-Side (AAS), which state the conditions under which triangles are guaranteed to be congruent. These theorems are essential in geometry to prove that two triangles are identical in size and shape.

How many triangle congruence theorems are there?

There are five triangle congruence theorems: Side-Side-Side (SSS), Side-Angle-Side (SAS), Angle-Side-Angle (ASA), Angle-Angle-Side (AAS), and Hypotenuse-Leg (HL). Each of these theorems outlines specific criteria that must be met in order to prove that two triangles are congruent.

What conditions must be met for two triangles to be congruent using SAS?

For two triangles to be congruent using the Side-Angle-Side (SAS) criterion, the following conditions must be met: first, the two sides that are included between the given angles in each triangle must be equal in length; second, the included angles in both triangles must be congruent; and third, the remaining side that is not included between the given angles must also be equal in length for both triangles. If all these conditions are satisfied, then the two triangles are considered congruent by the SAS criterion.

What conditions must be met for two triangles to be congruent using SSS?

Two triangles are congruent using the Side-Side-Side (SSS) criterion if the three sides of one triangle are equal to the corresponding three sides of the other triangle. In other words, if the three sides of one triangle are equal in length to the three sides of the other triangle, then the two triangles are congruent by the SSS criterion.

In which situations can two triangles be proven congruent using ASA?

Two triangles can be proven congruent using Angle-Side-Angle (ASA) when they have two corresponding angles that are congruent and the included side between these angles is also congruent. This means that if two triangles share two angles and the side between those angles has the same length in both triangles, then they are congruent by ASA.

How are two triangles proven congruent using AAS?

Two triangles can be proven congruent using the Angle-Angle-Side (AAS) postulate if they have two corresponding angles that are congruent and a corresponding side that is congruent. This means that if two pairs of corresponding angles in the triangles are equal, and a pair of corresponding sides between the angles are equal, then the two triangles are congruent.

What does the HL congruence theorem state?

The HL (Hypotenuse Leg) congruence theorem states that if the hypotenuse and a leg of one right triangle are congruent to the hypotenuse and a leg of another right triangle, then the two triangles are congruent. This means that the two right triangles are identical in size and shape.

Can two triangles be congruent if only two sides are equal?

No, two triangles cannot be congruent if only two sides are equal. In order for two triangles to be congruent, all three sides and all three angles of one triangle must be congruent to the corresponding sides and angles of the other triangle.

Can two triangles be congruent if only one angle and two sides are equal?

No, in order for two triangles to be congruent based on one angle and two sides, the given information must be the included angle and sides between that angle in both triangles. This is known as the Side-Angle-Side (SAS) congruence criterion. So, if only one angle and two sides are equal but not in the correct position, the triangles will not be congruent.

How are congruent triangles important in geometry and mathematics?

Congruent triangles are important in geometry and mathematics because they provide a way to establish the equality or similarity of two triangles based on their corresponding sides and angles. By proving that two triangles are congruent, we can infer information about their properties and relationships, such as the equality of corresponding angles, the equality of corresponding sides, and the similarity of other geometric figures. This helps in solving various geometric problems, constructing accurate diagrams, and making deductions in mathematical proofs. Congruent triangles serve as a fundamental concept in geometry, forming the basis for various geometric theorems and principles.