Simplifying Radicals Math Worksheets

If you're a student or an educator in search of practical and effective tools to deepen your understanding of simplifying radicals in math, you'll find that worksheets are an invaluable resource. These structured learning materials present a variety of problems and exercises that specifically focus on the entity and subject of simplifying radicals. By providing step-by-step instructions and relevant examples, these worksheets offer a thorough and systematic approach to mastering this essential math topic.

  Algebra 1 Simplifying Radical Expressions Worksheet

---

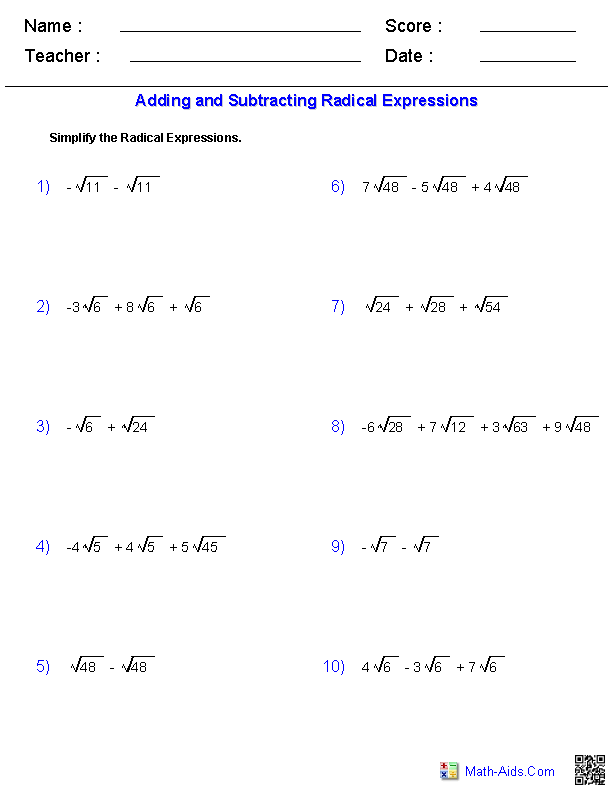
  Adding and Subtracting Radicals Worksheet

---

  Multiplying Radicals Worksheet

---

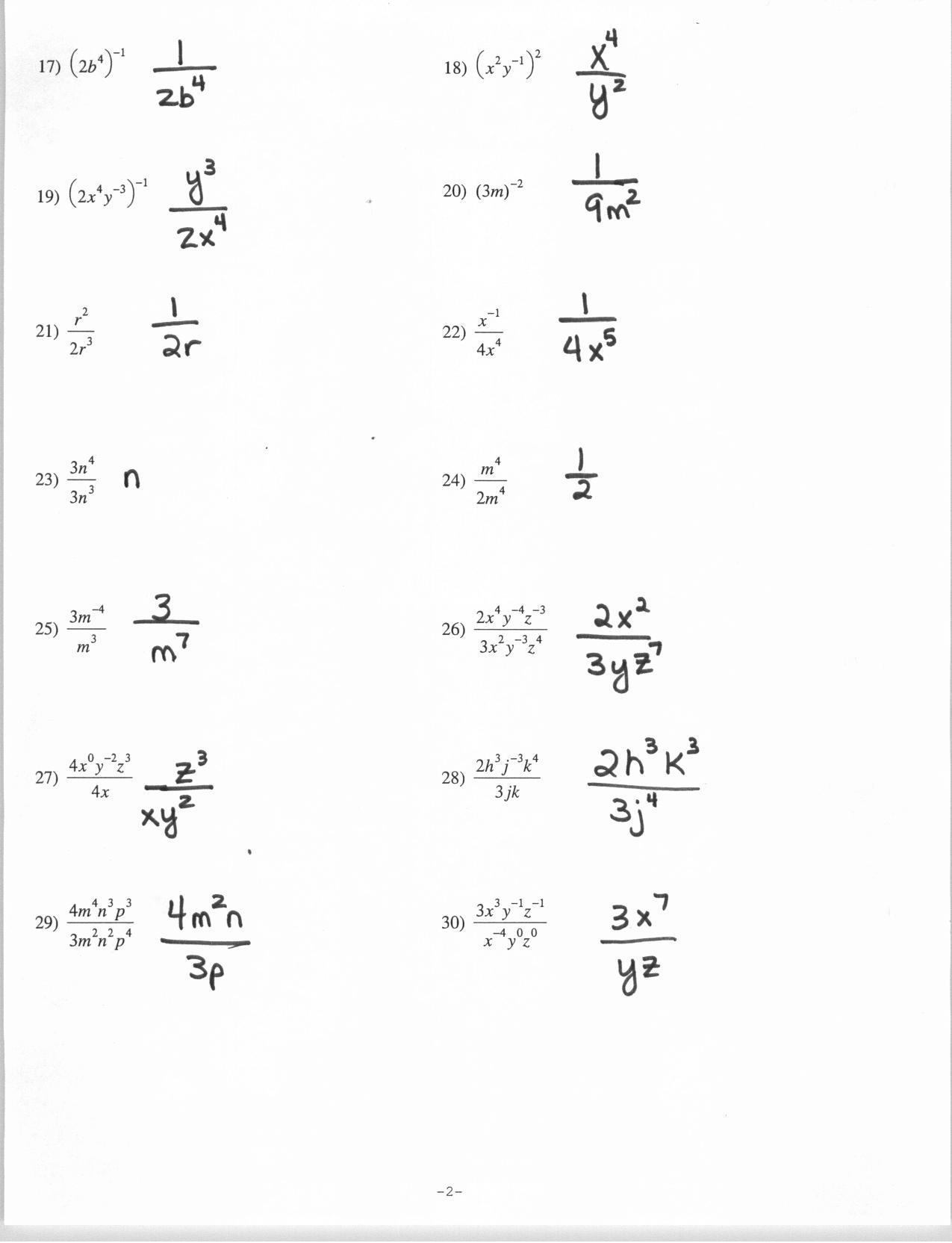
  Simplifying Radicals with Variables and Exponents

---

  Operations with Scientific Notation Worksheet

---

  Algebra 2 Puzzle Worksheets

---

  6th Grade Math Worksheets Exponents

---

  Quotient Rule Worksheet

---

  Solving Algebra Equations Worksheets

---

  Fifth Grade Math Worksheets

---

  One Whole Fraction Circle

---

  Exponents Worksheets with Answers

---

  Practice Homework Lesson 6 Answers

---

  Slope-Intercept Graphic Organizer

---

  Slope-Intercept Graphic Organizer

---

  Slope-Intercept Graphic Organizer

---

  Slope-Intercept Graphic Organizer

---

What is a radical expression?

A radical expression is an algebraic expression that includes a square root, cube root, or other roots. It typically involves some form of root symbol (?) and a radicand (the number or expression under the root symbol). Radical expressions are commonly used in mathematics to represent solutions to equations and to simplify complex expressions.

How do you simplify a radical expression?

To simplify a radical expression, you can factor the radicand into its prime factors and then bring any perfect square factors outside of the radical. This process allows you to simplify the radical and make it easier to work with. Remember to simplify and combine any like terms as needed to make the expression as simple as possible.

What is the difference between a square root and a cube root?

A square root is the value that, when multiplied by itself, gives the number being square rooted, while a cube root is the value that, when multiplied by itself twice (or cubed), gives the number being cubed rooted. In simpler terms, a square root finds the number that can be multiplied by itself to get the original number, while a cube root finds the number that, when multiplied by itself three times, equals the original number.

What is the principal square root of a number?

The principal square root of a number is the non-negative square root. For example, the principal square root of 16 is 4, not -4, because the principal square root is always the positive value.

How do you simplify radicals with variables?

To simplify radicals with variables, first identify any common factors between the variable and the number inside the radical. Then, apply the rules of exponents to rewrite the radical in simpler form. Finally, if necessary, multiply the simplified expressions inside and outside the radical to get the final simplified radical form. Remember to simplify any square roots of perfect squares that can be simplified further.

Can you simplify a radical if the number under the root sign is not a perfect square?

No, you cannot simplify a radical if the number under the root sign is not a perfect square. A radical can only be simplified when the number under the root sign can be expressed as the product of a perfect square and another number. Otherwise, the radical is considered in simplest form.

How do you simplify radicals that involve fractions?

To simplify radicals that involve fractions, you can first simplify the fraction part separately and then combine it with the radical. For example, if you have ?(9/4), you can simplify the fraction to 3/2 and then take the square root of 3/2 to get ?(3/2). This way, you can simplify radicals involving fractions by simplifying the fraction part first and then taking the square root of the simplified fraction.

What is the difference between a rational exponent and a radical?

A rational exponent is a fraction exponent where the numerator is the power to which the base is raised, and the denominator is the root of the exponent. For example, x^(2/3) represents the cube root of x squared. On the other hand, a radical is a symbol ? that indicates a specific root of a number or expression. For instance, ?x is the square root of x. Ultimately, both rational exponents and radicals involve raising a base to a certain power or finding a specific root, but they are represented and used in slightly different ways.

Can you simplify a radical if the number under the root sign is negative?

No, you cannot simplify a radical if the number under the root sign is negative. This is because the square root of a negative number is not a real number; it is an imaginary number. In algebra, we define the square root of a negative number as the imaginary unit "i" multiplied by the square root of the positive value of the number.

What are some real-life applications of simplifying radicals?

Real-life applications of simplifying radicals include designing efficient irrigation systems for farming to calculate water flow rates, constructing buildings to determine accurate measurements for materials needed, and in cryptography to secure sensitive information by using complex mathematical calculations involving square roots. Simplifying radicals helps in simplifying complex calculations and making them more manageable in various fields such as engineering, architecture, and cybersecurity.