Simplifying Expressions Worksheets 7th Grade
Are you a 7th-grade math student who is struggling with simplifying expressions? If so, you've come to the right place. Simplifying expressions can be challenging, but with the help of carefully designed worksheets, you can gain a better understanding of this important concept. These worksheets are designed to engage and support students in mastering the skills needed to simplify expressions effectively.
Table of Images 👆
- Simplifying Algebraic Expressions Worksheet
- 5th Grade Algebra Variables Worksheets
- Simplify Expressions Worksheet
- 7th Grade Combining Like Terms Worksheet
- Variable Expressions 7th Grade
- Algebraic Expressions Worksheets
- Math Expressions Worksheets 7th Grade
- Solving Equations Worksheets 7th Grade Math
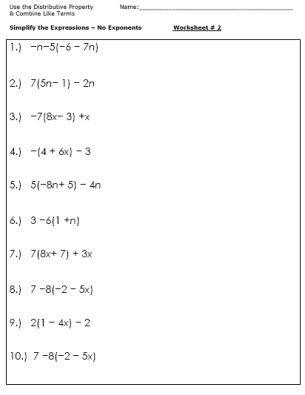
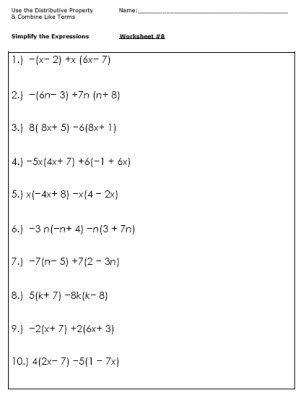
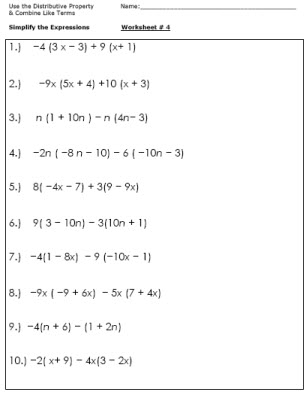
- Distributive Property and Combining Like Terms Worksheet
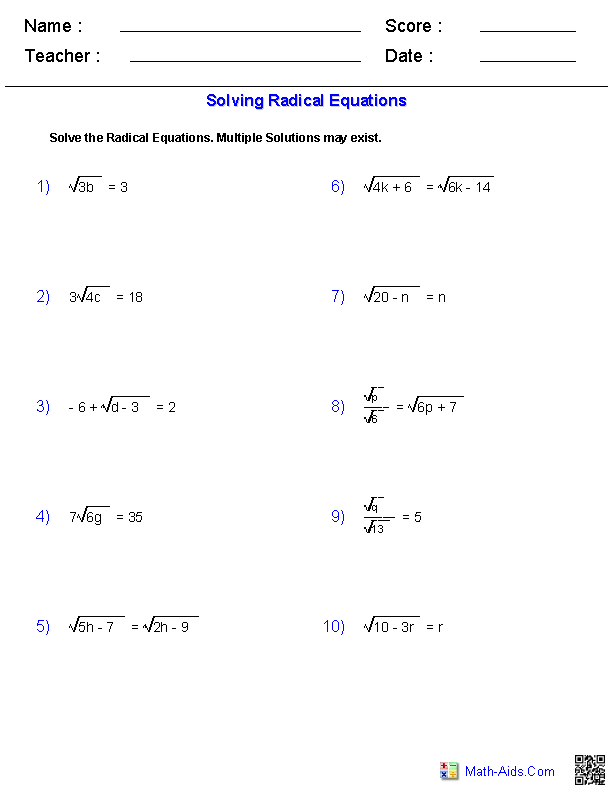
- Simplifying Radical Expressions Worksheet
- Evaluating Expressions Worksheets 6th Grade Printable
- Algebra 1 Worksheets
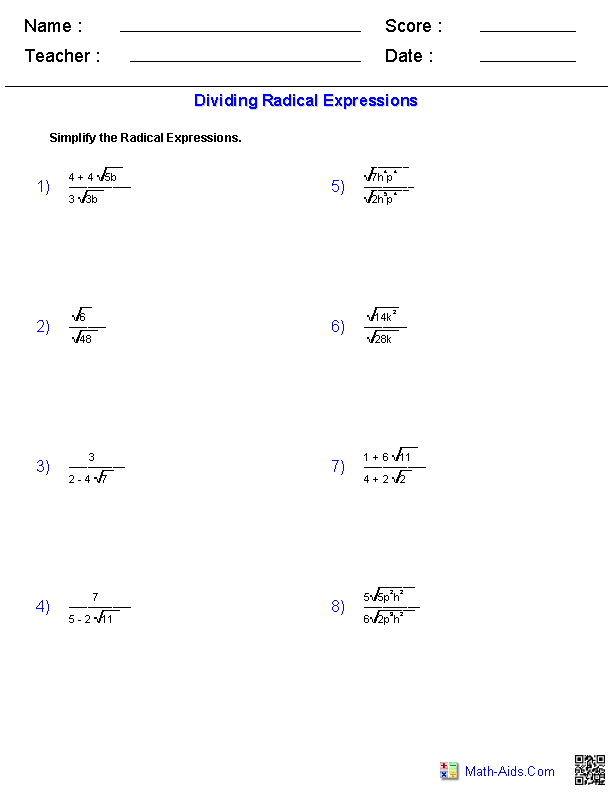
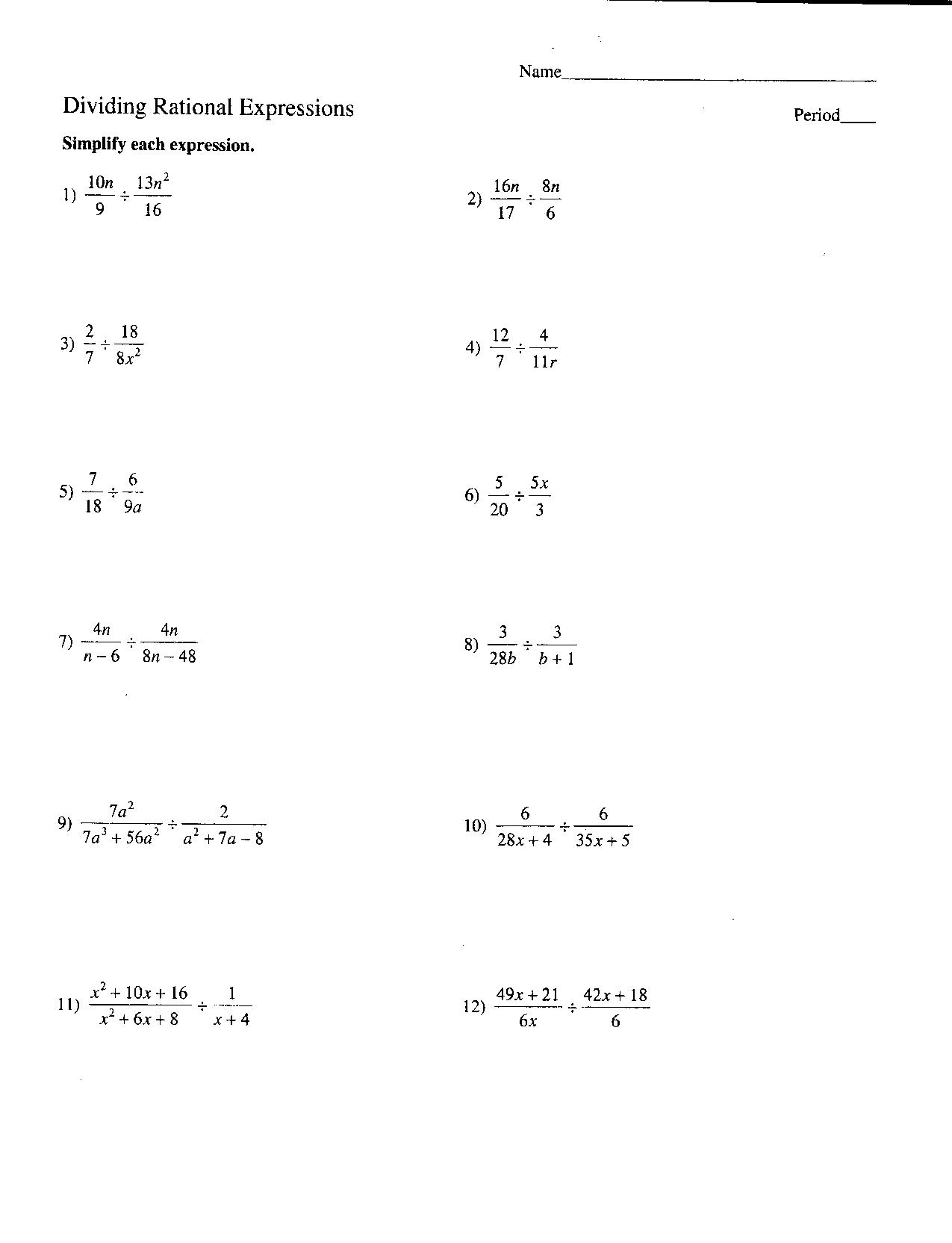
- Dividing Radical Expressions Worksheets
- 7th Grade Pre-Algebra Worksheets
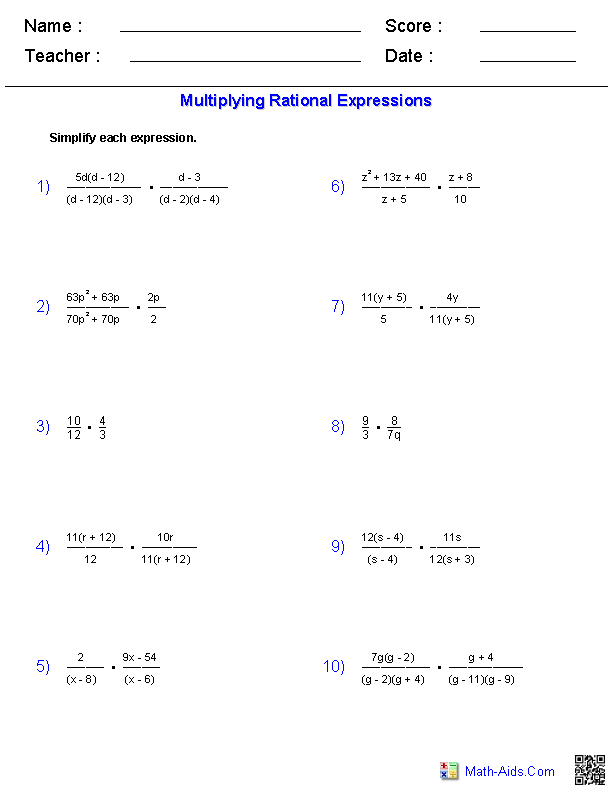
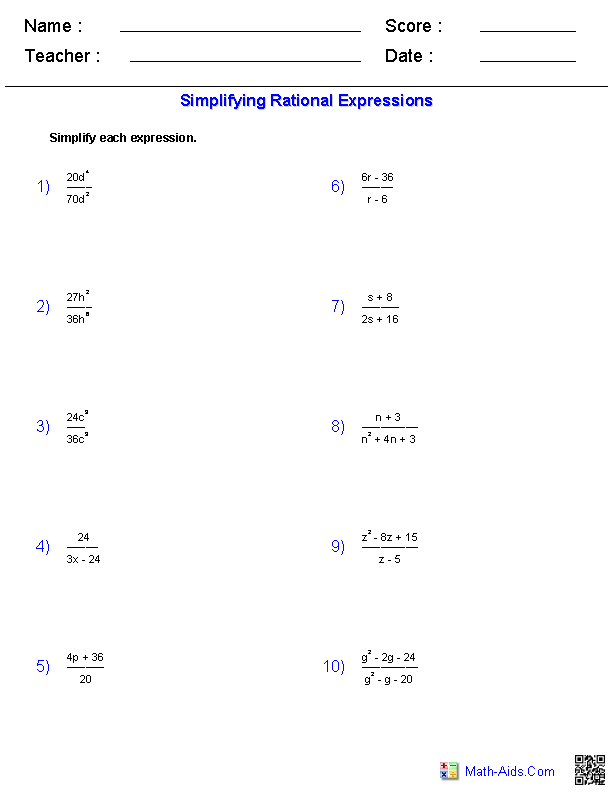
- Simplifying Rational Expressions Worksheets
More 7th Grade Worksheets
7th Grade Math Worksheets with Answer Key7th Grade Math Coloring Worksheets
7th Grade Vocabulary Worksheets
Pre-Algebra 7th Grade Math Worksheets
Algebraic Expressions Worksheets 7th Grade
Reading Comprehension Worksheets 7th Grade
7th Grade Math Worksheets Proportions
Complex Sentence Worksheets 7th Grade
Geometry Angles Worksheet 7th Grade Math
What is the purpose of simplifying expressions in 7th grade?
Simplifying expressions in 7th grade helps students develop a deeper understanding of mathematical concepts by practicing essential skills like combining like terms, following order of operations, and identifying equivalent forms of expressions. This process encourages critical thinking, problem-solving, and lays the foundation for more complex algebraic concepts in higher grade levels.
What are the basic steps involved in simplifying an expression?
To simplify an expression, first simplify within parentheses, then combine like terms, perform any operations, and finally, simplify any remaining terms or numbers. Remember to follow the order of operations (PEMDAS) and look for common factors to factor out if necessary. Always simplify as much as possible to make the expression easier to work with.
How do you simplify expressions with combining like terms?
To simplify expressions with combining like terms, you need to first identify the terms that are similar or have the same variables raised to the same power. Then, you can combine these like terms by adding or subtracting their coefficients. Finally, you can rearrange the simplified expression to make it more concise and easier to read. Make sure to pay attention to the signs of the coefficients when combining like terms.
How do you simplify expressions with parentheses?
To simplify expressions with parentheses, start by following the order of operations—PEMDAS (parentheses, exponents, multiplication and division from left to right, addition and subtraction from left to right). Begin by simplifying expressions within the parentheses first, then proceed to simplify the rest of the expression. Combine like terms and perform any remaining operations to arrive at the final simplified form of the expression.
What is the difference between simplifying expressions with addition and subtraction versus multiplication and division?
When simplifying expressions with addition and subtraction, you combine like terms by adding or subtracting them. In contrast, when simplifying expressions with multiplication and division, you multiply or divide the terms accordingly. With addition and subtraction, terms must have the same variables and exponents to be combined, while with multiplication and division, you can use the rules of exponents and distribute operations as needed.
How do you simplify expressions involving exponents?
To simplify expressions involving exponents, you can use the properties of exponents such as the Product Rule (a^m * a^n = a^(m+n)), Quotient Rule (a^m / a^n = a^(m-n)), and Power Rule ((a^m)^n = a^(m*n)). Additionally, consider simplifying by combining like terms and reducing any coefficients or constants. It's also helpful to remember that any number raised to the power of 0 is equal to 1. By applying these rules and techniques, you can simplify expressions with exponents efficiently.
How do you simplify expressions involving fractions?
To simplify expressions involving fractions, you can start by finding the greatest common factor (GCF) of the numerator and the denominator. Then, divide both the numerator and denominator by the GCF to reduce the fraction to its simplest form. Additionally, you can combine like terms in the fraction by adding or subtracting them as needed. Finally, make sure to follow the order of operations when simplifying the expression to ensure accuracy.
What are some common mistakes to avoid when simplifying expressions?
Some common mistakes to avoid when simplifying expressions include not distributing correctly, forgetting negative signs when combining like terms, mixing up addition and subtraction rules, incorrectly applying the order of operations, and neglecting to simplify fractions or exponents fully. It is also important to double-check the final answer to ensure it is in simplest form and to always show each step of simplification to avoid errors.
How does simplifying expressions help with problem-solving in math?
Simplifying expressions in math helps to make complex problems more manageable and easier to work with. By reducing expressions to their simplest form, it allows for clearer analysis of the problem at hand, enabling students to focus on the underlying principles and relationships involved. This streamlined approach ultimately facilitates problem-solving by providing a clearer understanding of the problem, making it easier to identify patterns, apply relevant formulas, and come up with strategic solutions.
How can practicing simplifying expressions worksheets improve your overall math skills?
Practicing simplifying expressions worksheets can improve your overall math skills by honing your ability to identify patterns, manipulate algebraic terms, and apply various rules of arithmetic and algebra. This practice helps to deepen your understanding of fundamental math concepts, enhances problem-solving skills, and builds confidence in tackling more complex mathematical problems. Additionally, consistently working on simplifying expressions can improve your mental math abilities and critical thinking skills, which are essential for success in higher-level mathematics and various real-world applications.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.





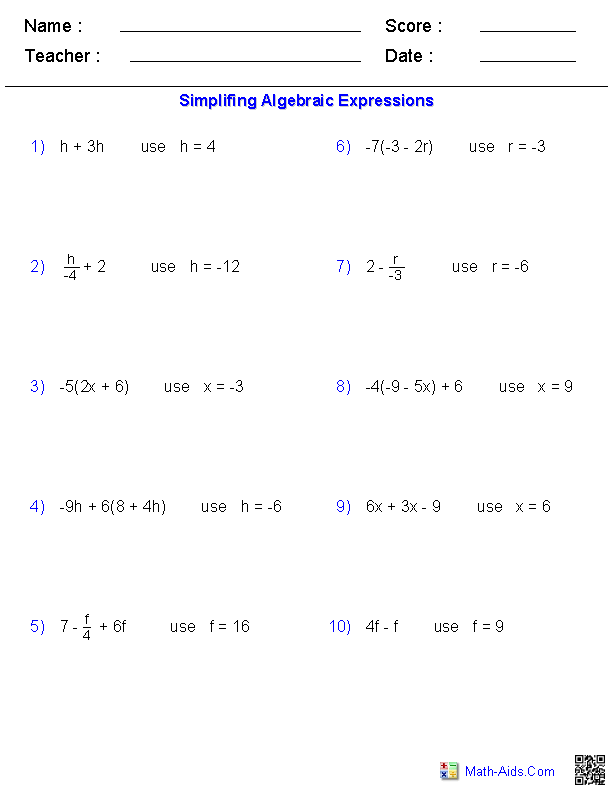



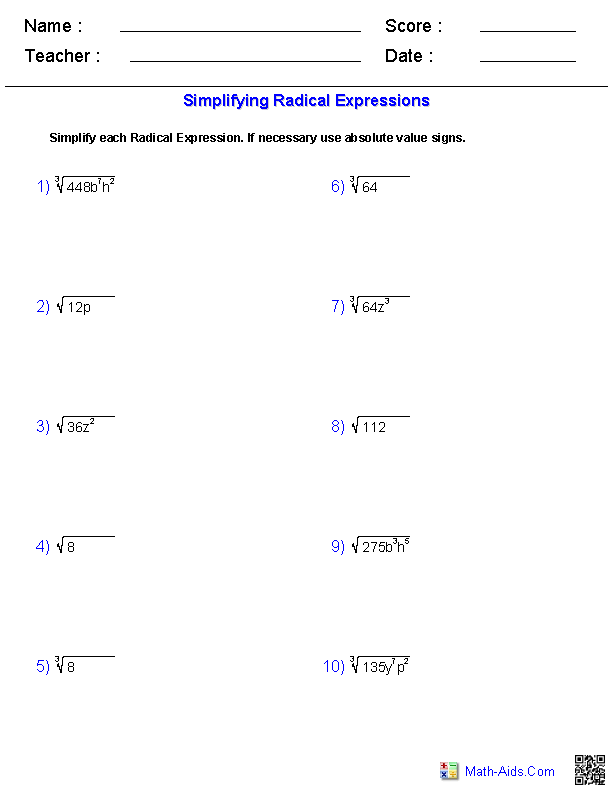















Comments