Simple Linear Equations Worksheets
If you're a teacher or a parent looking for a straightforward and effective way to help students practice solving linear equations, you've come to the right place. Worksheets focused on simple linear equations provide a structured platform for students to understand and apply mathematical concepts surrounding variables, coefficients, and graphing.
Table of Images 👆
- Solving Algebra Equations Worksheets
- Algebra Equations Worksheets
- Slope and Linear Equations Worksheets
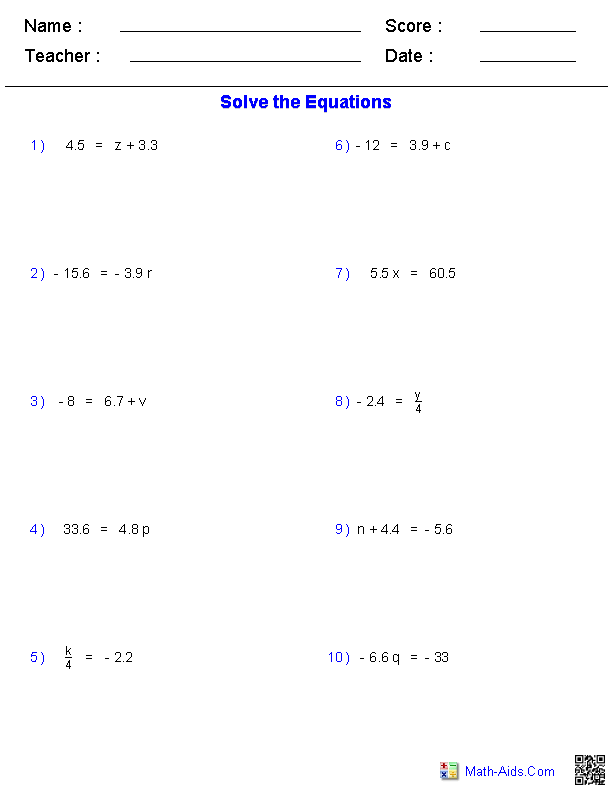
- Linear Equations Worksheets
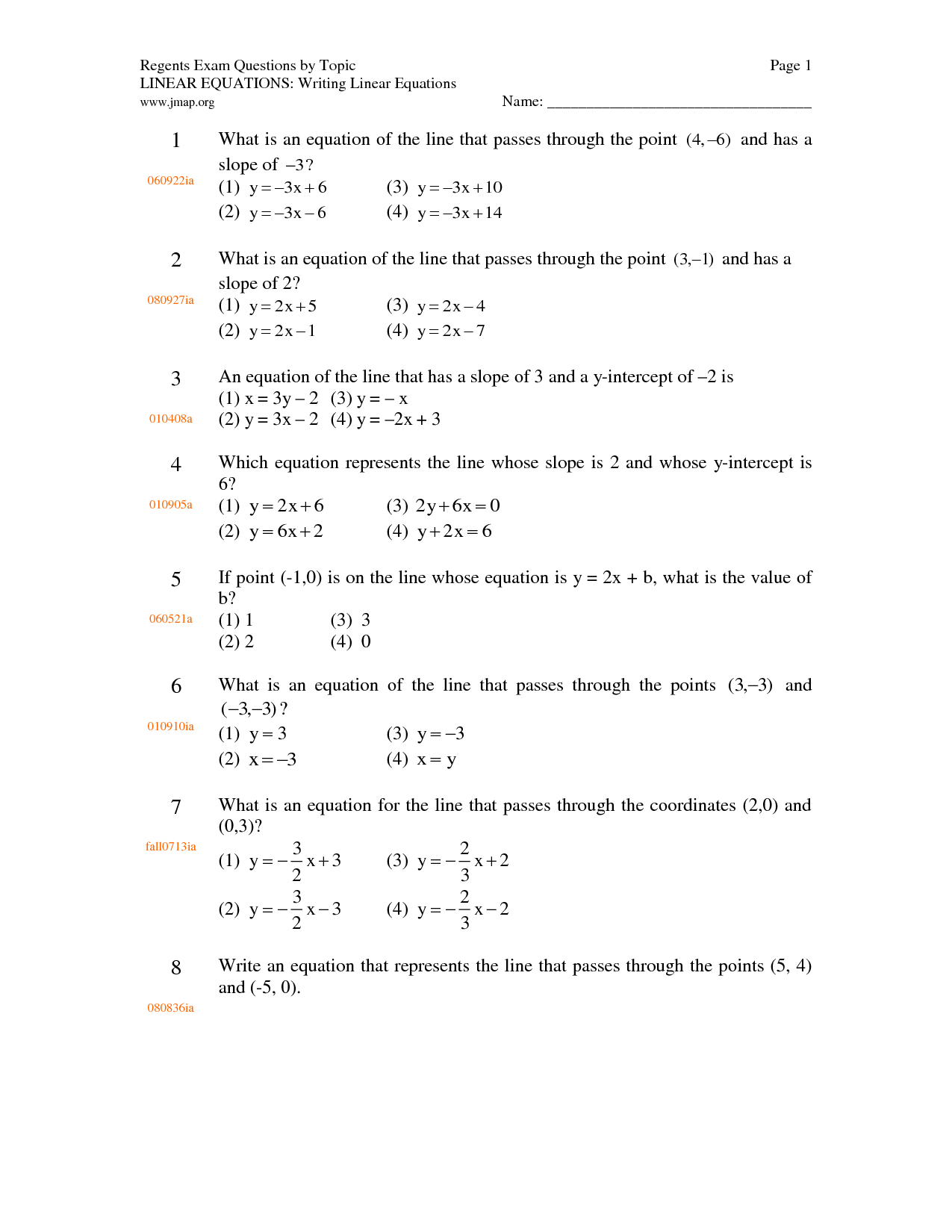
- Writing Linear Equations Worksheets
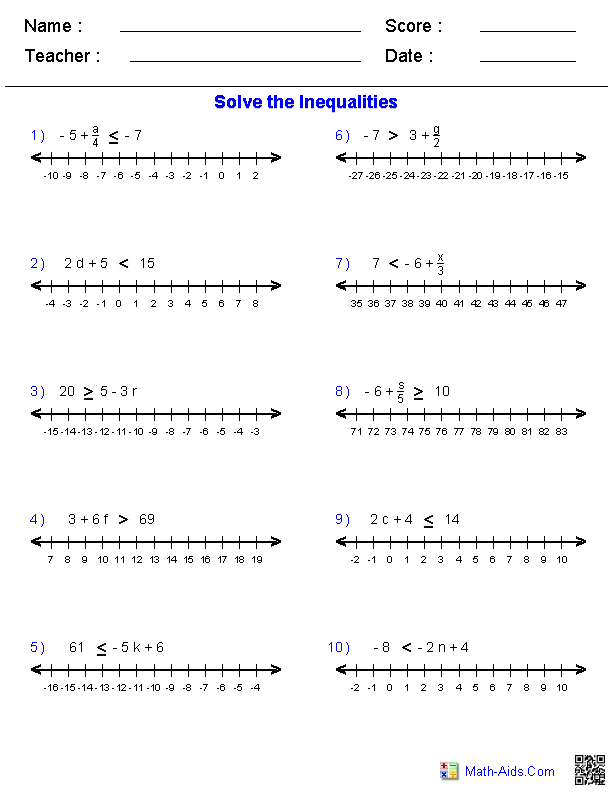
- Graphing Linear Inequalities Worksheet
- One Step Inequalities Worksheet
- 4th Grade Math Worksheets PDF
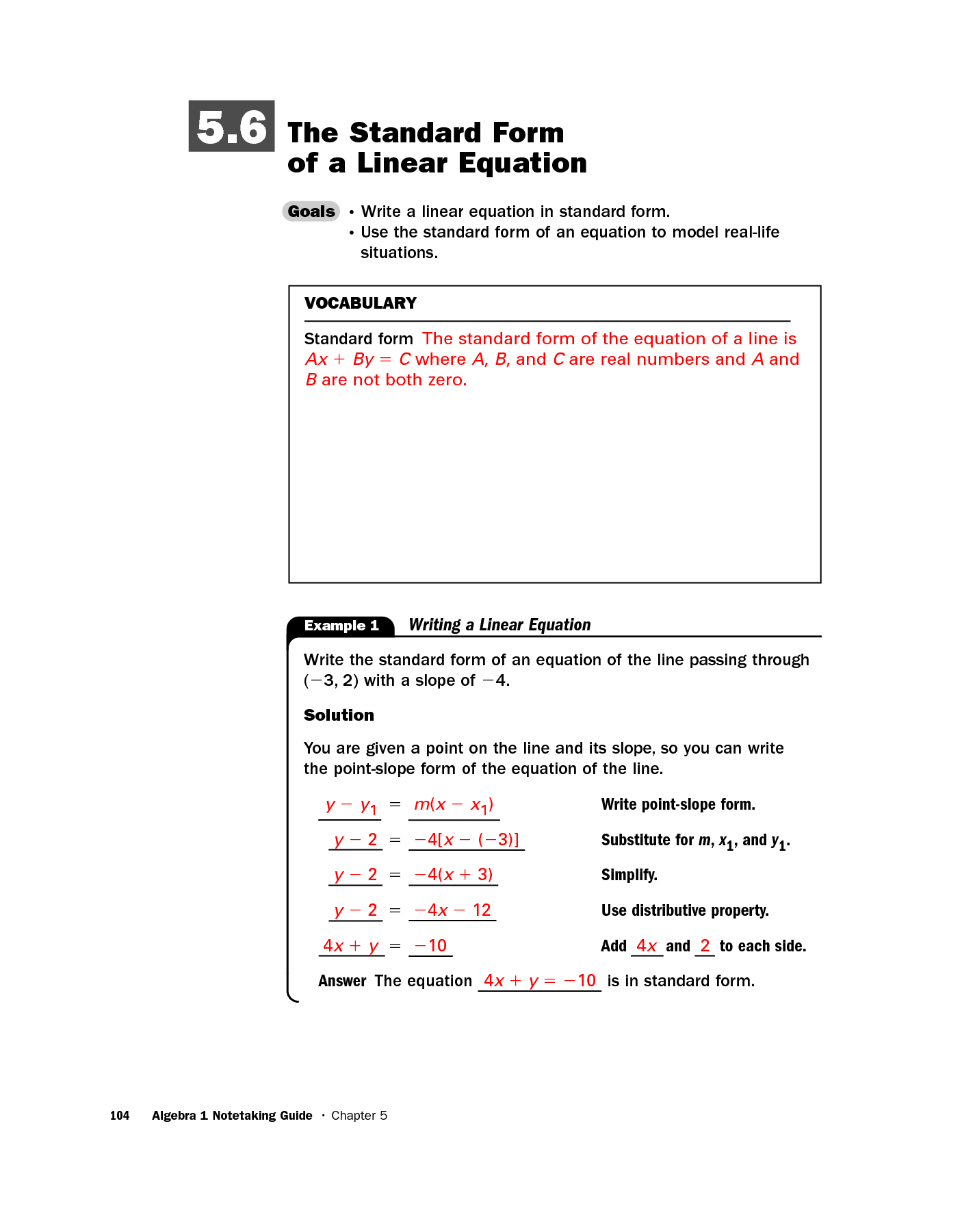
- Standard Form Linear Equations Worksheets
- Quadratic Equations Math Questions
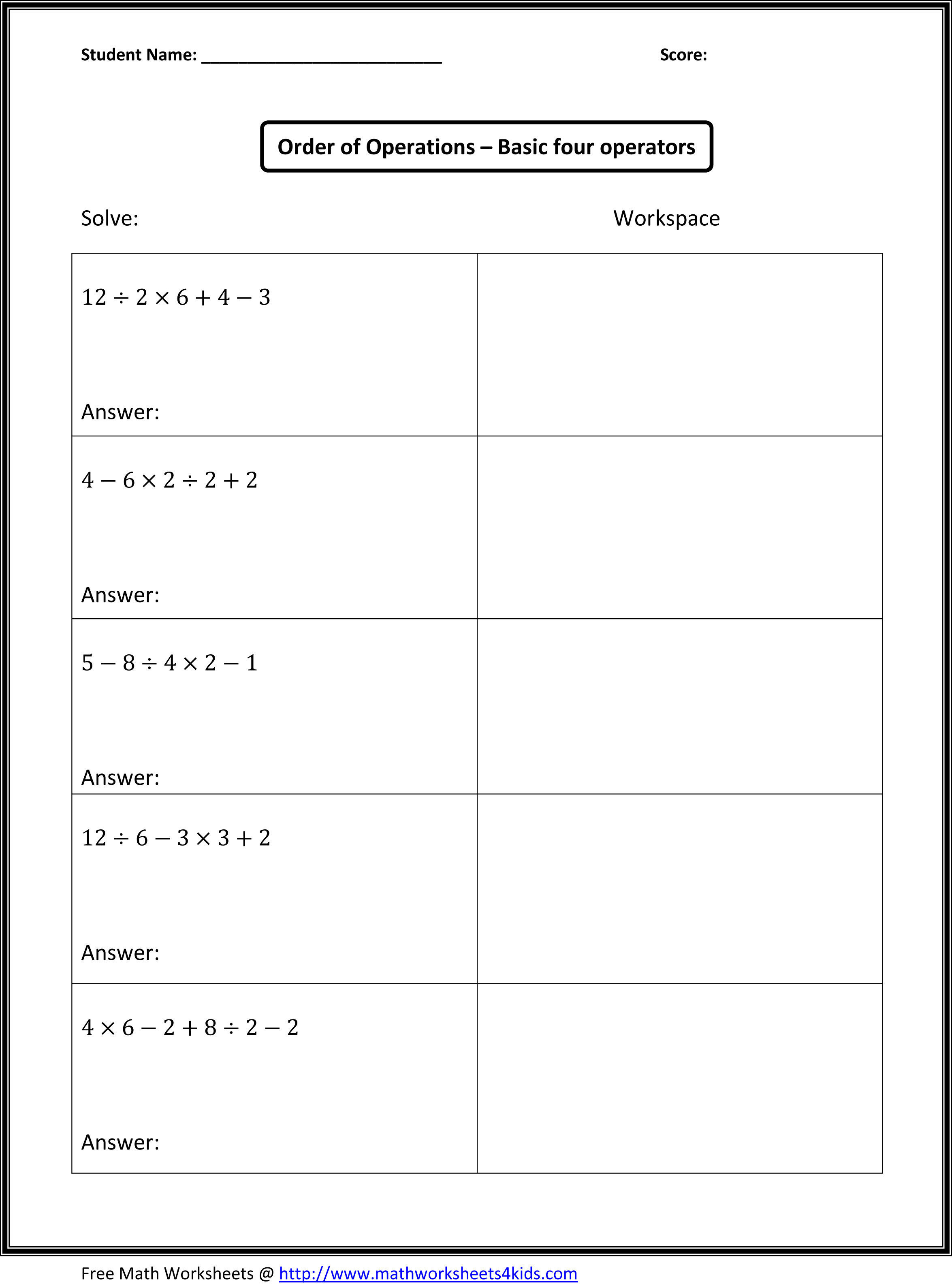
- 5th Grade PEMDAS Worksheets Order Operations
- Solving Linear Equations with Brackets
- Converting Metric Units Worksheet 5th Grade
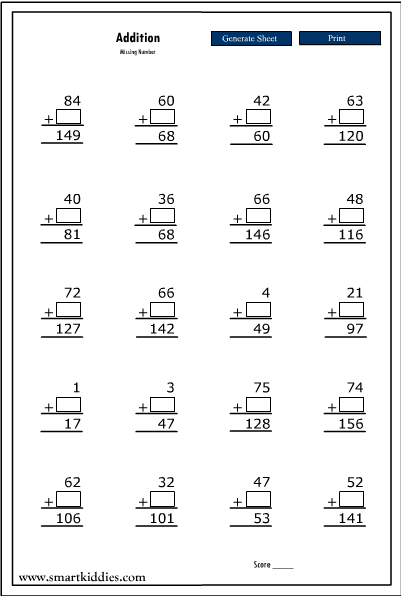
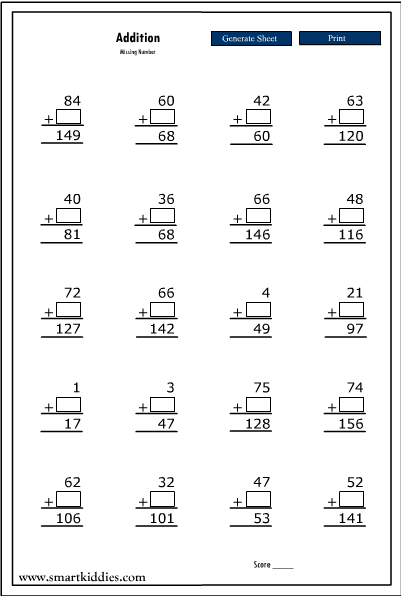
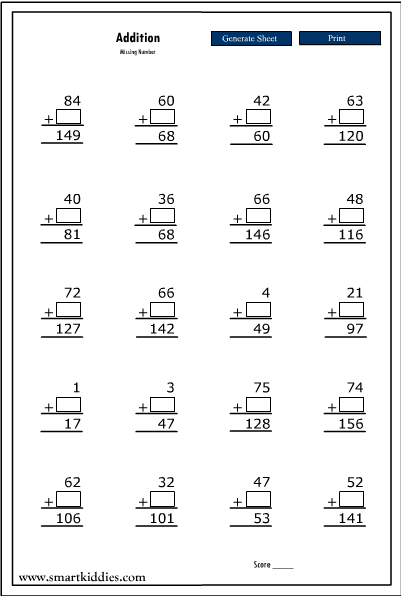
- Missing Number Addition Worksheets
- Missing Number Addition Worksheets
- Missing Number Addition Worksheets
More Line Worksheets
Lines of Symmetry WorksheetsLine Drawing Art Worksheets
Drawing Contour Lines Worksheet
Blank Printable Timeline Worksheets
2 Lines of Symmetry Worksheets
Linear Equations Worksheet 7th Grade
Rounding Decimals Number Line Worksheet
Graphing Inequalities On a Number Line Worksheets
College Essay Outline Worksheet
Texture Line Drawing Techniques Worksheet
What is a simple linear equation?
A simple linear equation is an equation that represents a straight line on a graph and has only one variable raised to the power of 1. It can be written in the form of y = mx + b, where y is the dependent variable, x is the independent variable, m is the slope of the line, and b is the y-intercept. This type of equation is commonly used to describe relationships between two variables in mathematics and can be solved to find the value of the variable.
How can you determine the slope of a simple linear equation?
To determine the slope of a simple linear equation in the form y = mx + b, you can identify the coefficient of the variable x, which is represented by 'm'. The slope of the line is equal to 'm', and it indicates the rate at which the line is rising or falling as x increases.
How can you determine the y-intercept of a simple linear equation?
To determine the y-intercept of a simple linear equation, you can set the x-value to zero and solve for the y-value. The y-intercept is the point where the line intersects the y-axis, and it represents the value of y when x is zero. By substituting x=0 into the equation, you can find the y-intercept and plot it on a graph to locate where the line crosses the y-axis.
What is the purpose of graphing simple linear equations?
The purpose of graphing simple linear equations is to visually represent the relationship between two variables and to see how they interact with each other on a coordinate plane. By plotting points and drawing a line that connects them, we can easily determine the slope, y-intercept, and the overall trend of the relationship between the variables. This visual representation helps in understanding and analyzing the data, making predictions, and solving real-world problems efficiently.
What does it mean for two simple linear equations to be parallel?
Two simple linear equations are parallel if they have the same slope but different y-intercepts. This means that the lines represented by the equations will never intersect, as they run in the same direction along the plane. In other words, parallel lines have the same steepness but are offset vertically from each other.
How can you determine if two simple linear equations are parallel?
Two simple linear equations are parallel if their slopes are equal. In other words, if the coefficients of the variables (usually represented as m or a) in both equations are the same, then the two lines defined by the equations are parallel. This means that the lines will never intersect and will maintain a constant distance between them as they extend infinitely in both directions.
What does it mean for two simple linear equations to be perpendicular?
Two simple linear equations are perpendicular if the slopes of their lines are negative reciprocals of each other. In other words, when two lines intersect at a right angle, the product of their slopes is -1. This relationship between the slopes of perpendicular lines is a key concept in understanding the geometry of linear equations.
How can you determine if two simple linear equations are perpendicular?
Two simple linear equations are perpendicular if the product of their slopes is equal to -1. To determine this, calculate the slope for each equation by rearranging them into the form y = mx + b, where m is the slope. Then, multiply the slopes of the two equations together. If the product is -1, then the two lines are perpendicular.
How can you use a simple linear equation to solve real-life problems?
Simple linear equations can be used to solve real-life problems by modeling relationships between two variables that have a linear pattern, such as distance over time or cost per item. By setting up an equation in the form of y = mx + b, where y represents the dependent variable, x represents the independent variable, m is the slope, and b is the y-intercept, you can calculate and predict values based on known data points. This can be especially useful in budgeting, determining rates of change, or making forecasts in various scenarios by analyzing the relationship between variables in a straight-line context.
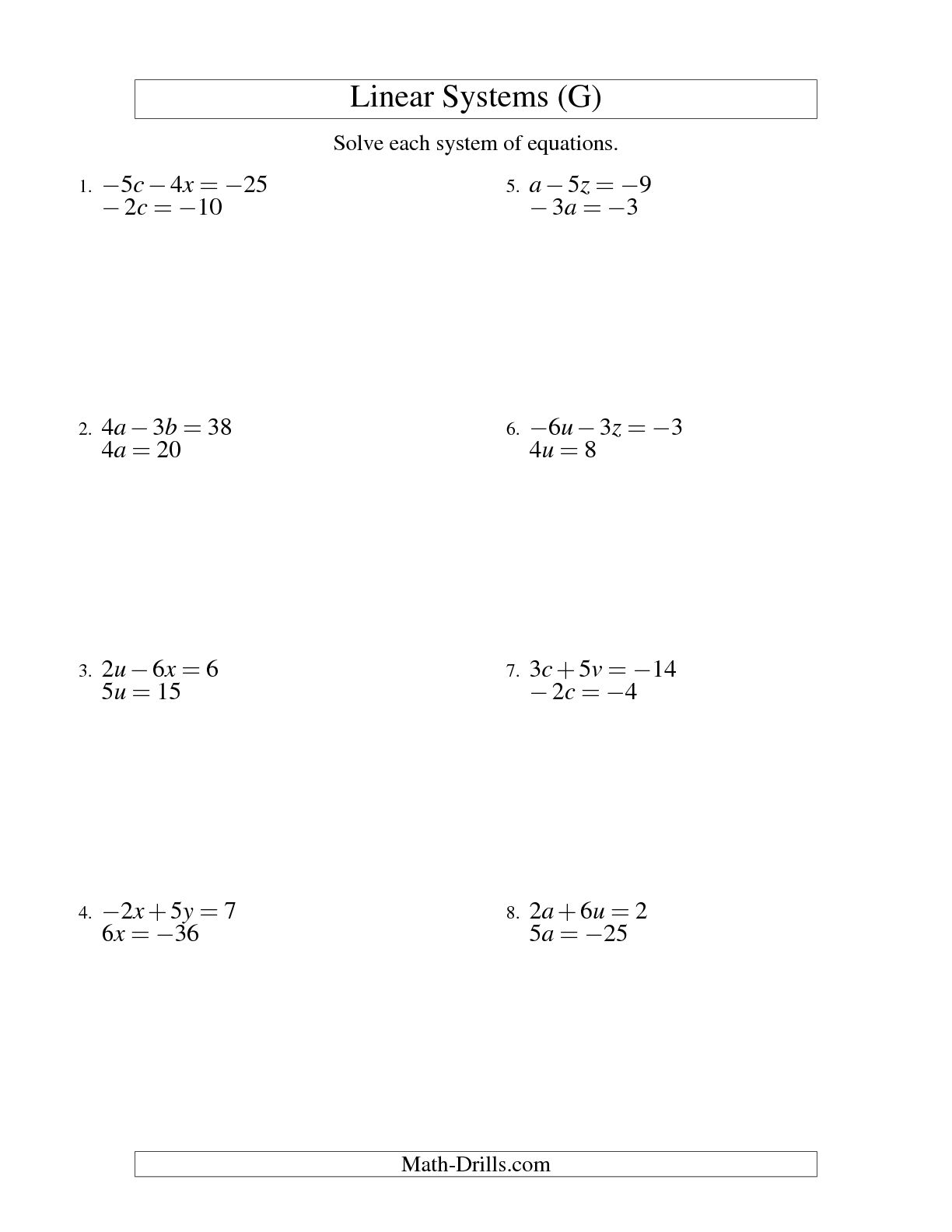
How can you solve a system of two simple linear equations algebraically?
To solve a system of two simple linear equations algebraically, you can use substitution or elimination methods. First, rearrange both equations so that one variable is isolated. Then, substitute or eliminate one variable from one equation into the other to find the value of the remaining variable. Finally, substitute the value back into one of the original equations to solve for the second variable. The point of intersection of the two equations represents the solution to the system.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.





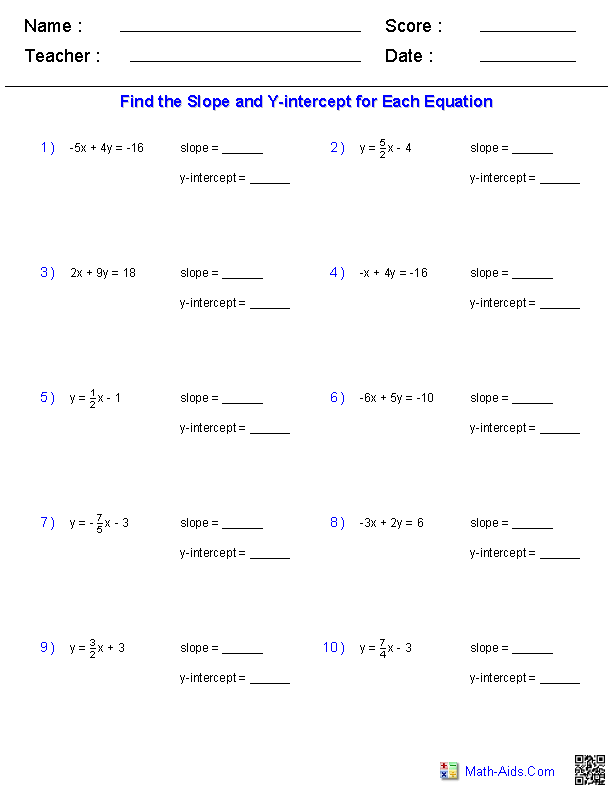

















Comments