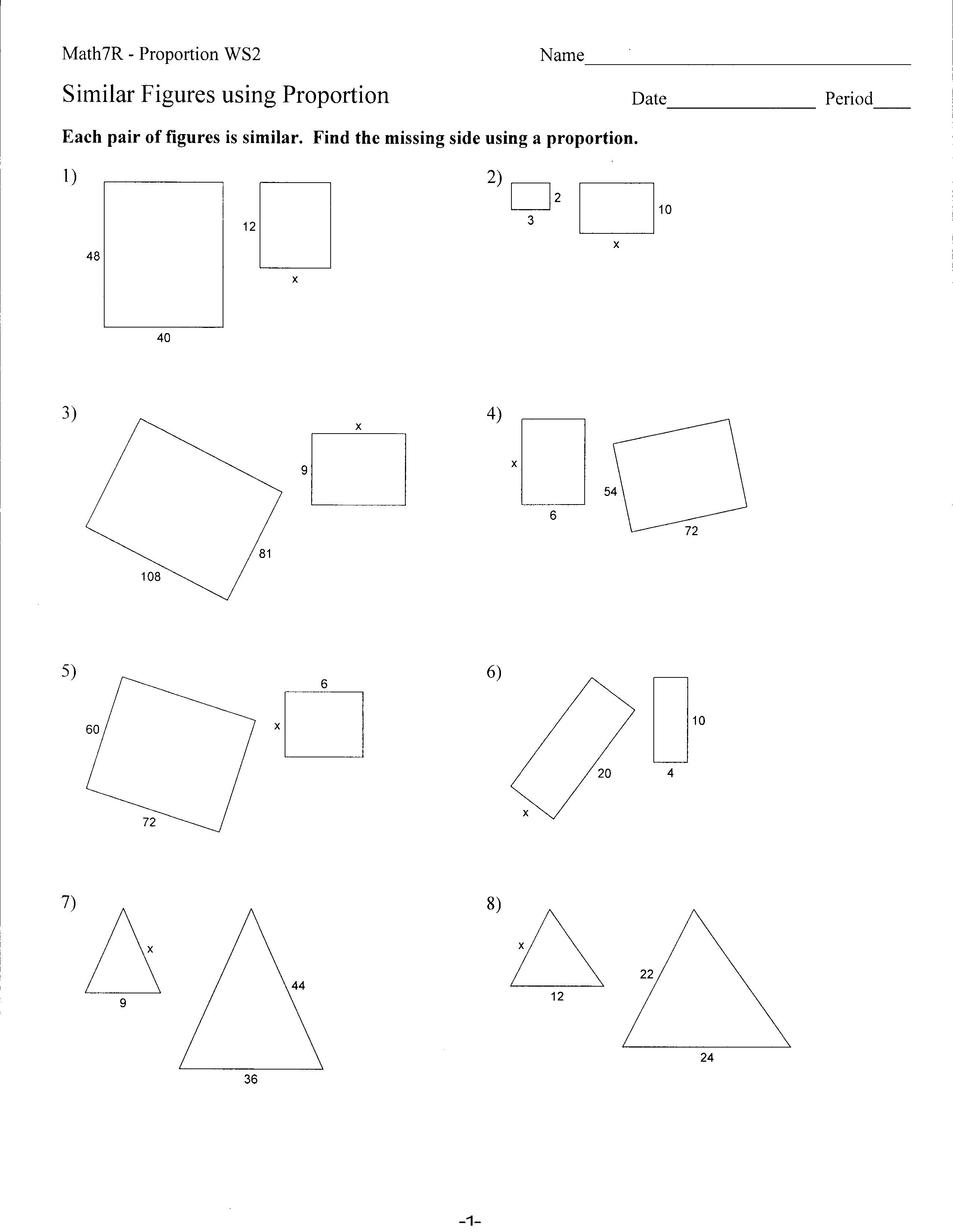
Similar Figures Worksheets 7th Grade
In 7th grade math, one of the important concepts that students learn is similar figures. Understanding and identifying similar figures is crucial for solving problems involving proportions and ratios. That's why having access to well-designed worksheets is essential for reinforcing this topic and providing students with ample practice. In this blog post, we will explore some useful worksheets on similar figures that are tailored specifically for 7th-grade students.
Table of Images 👆
- Similar Figures 7th Grade Worksheets
- Similar Figures and Proportions Worksheets
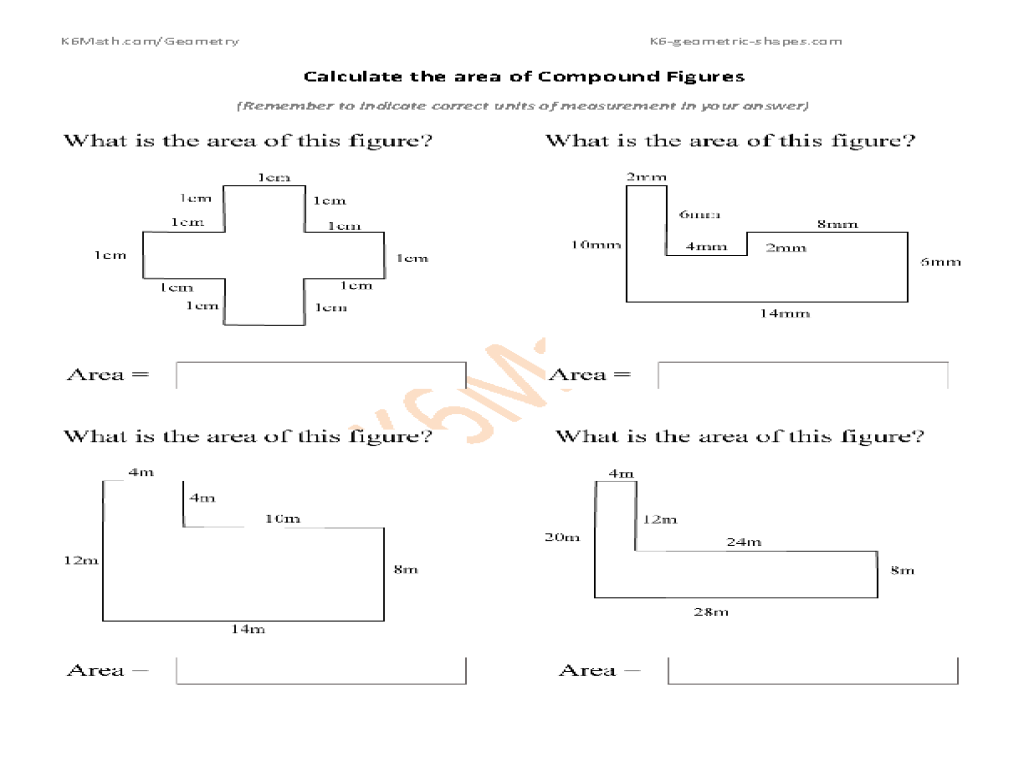
- Area of Composite Figures Worksheet 6th Grade
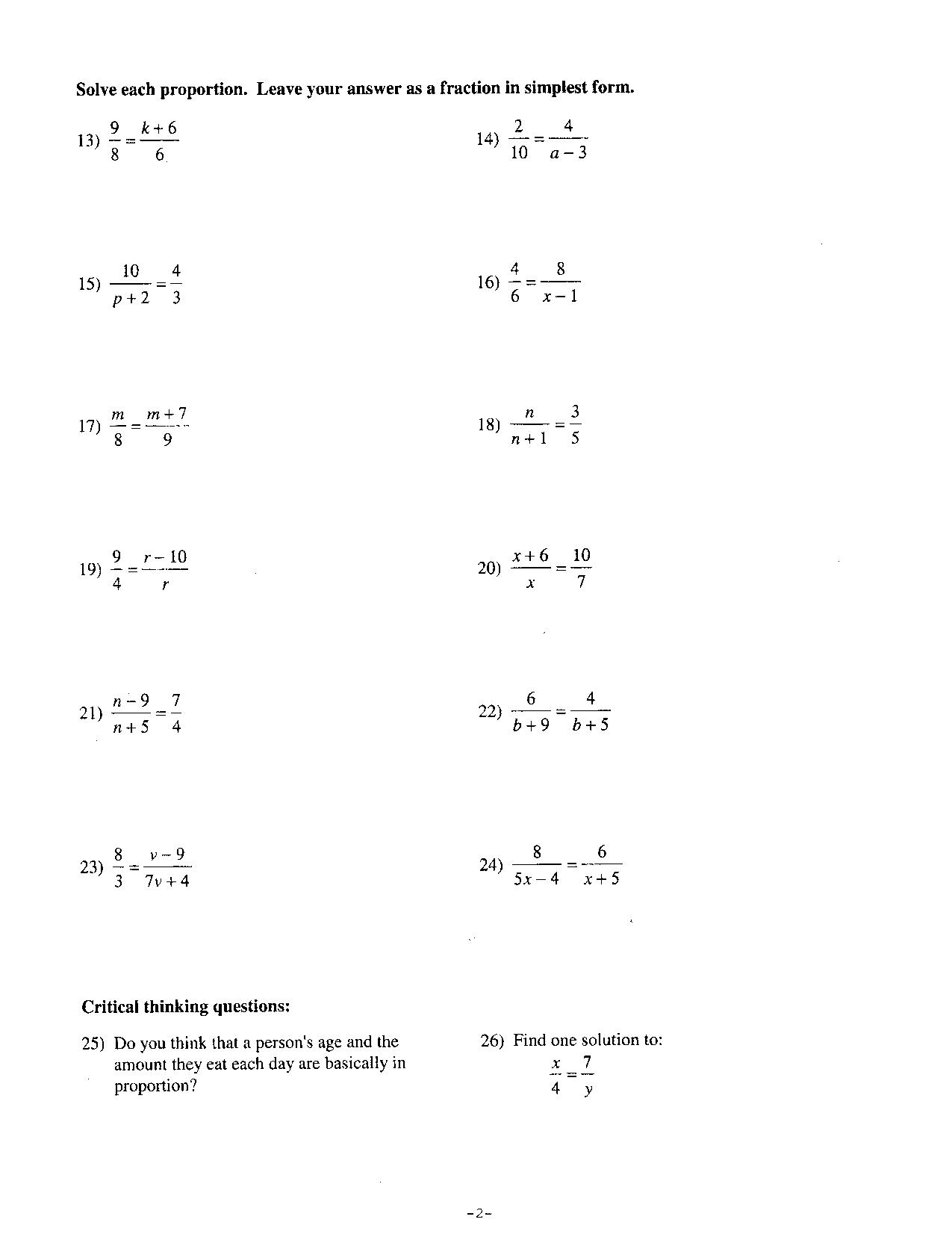
- 7th Grade Proportions Worksheet Answers
- Similar Figures 7th Grade Worksheets
- Similar Triangles and Polygons Worksheet
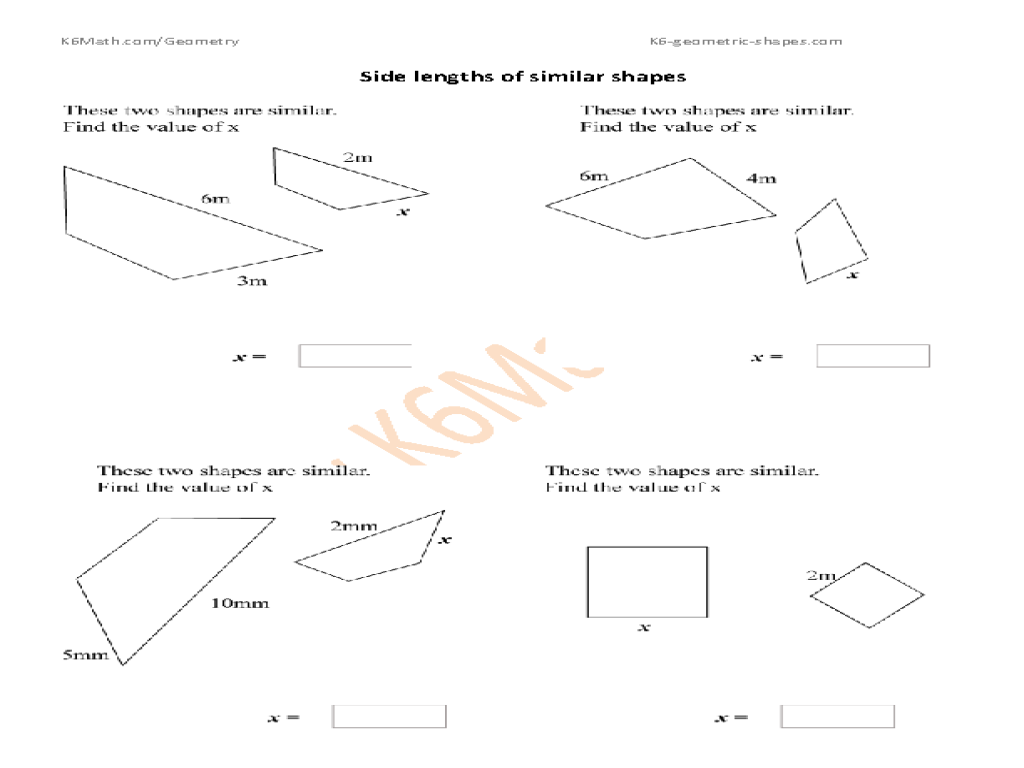
- Similar Polygons Worksheet
- 7th Grade Area Math Worksheets
- Similar Figures Proportions Worksheet
- Similar Figures 7th Grade Worksheets
- Similar Figures 7th Grade Worksheets
- 7th Grade Math Worksheets Proportions
- Congruent and Similar Shapes Worksheet
Similar Figures 7th Grade Worksheets
Similar Figures and Proportions Worksheets
Area of Composite Figures Worksheet 6th Grade
7th Grade Proportions Worksheet Answers
Similar Figures 7th Grade Worksheets
Similar Triangles and Polygons Worksheet
Similar Polygons Worksheet
7th Grade Area Math Worksheets
Similar Figures Proportions Worksheet
Similar Figures 7th Grade Worksheets
Similar Figures 7th Grade Worksheets
7th Grade Math Worksheets Proportions
Congruent and Similar Shapes Worksheet
More 7th Grade Worksheets
7th Grade Math Worksheets with Answer Key7th Grade Vocabulary Worksheets
Pre-Algebra 7th Grade Math Worksheets
7th Grade Math Worksheets Proportions
Complex Sentence Worksheets 7th Grade
Geometry Angles Worksheet 7th Grade Math
What are similar figures?
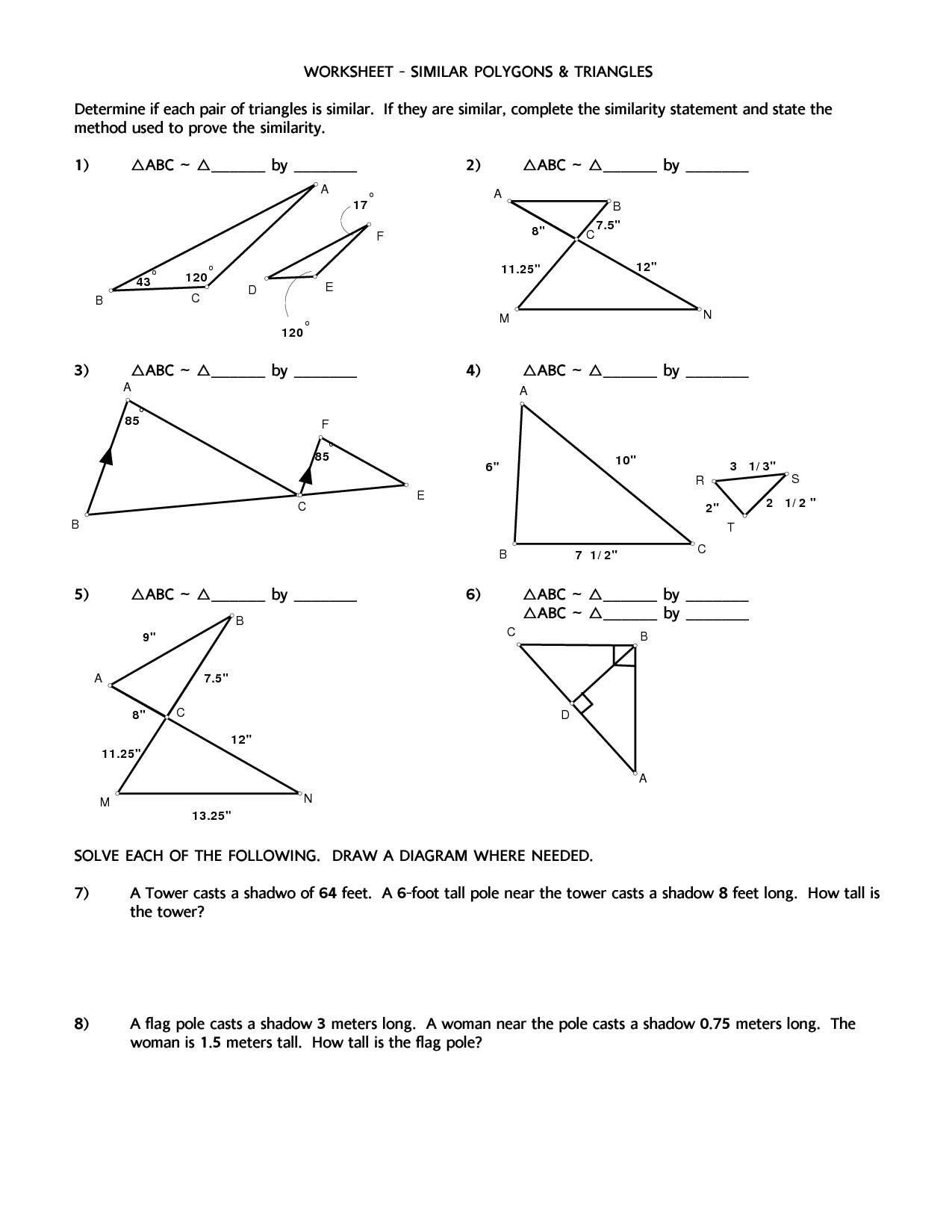
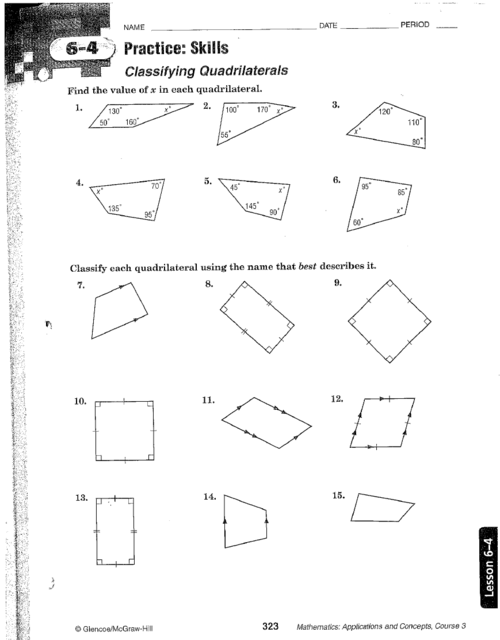
Similar figures are shapes that have the same shape but not necessarily the same size. This means that their corresponding sides are in proportion and their corresponding angles are equal. This allows for the figures to be scaled up or down while maintaining their overall shape.
How can you determine if two figures are similar?
Two figures are considered similar if they have the same shape but not necessarily the same size. To determine if two figures are similar, you can compare their corresponding angles to see if they are congruent and compare the lengths of their sides to see if they are proportional. If the angles are congruent and the sides are in proportion, then the figures are similar. Additionally, you can use scale factors to compare the measurements of the corresponding sides and determine if the figures are similar.
What is the significance of corresponding angles in similar figures?
Corresponding angles in similar figures are important because they are equal in measure. This means that when two figures are similar, their corresponding angles will have the same degree of rotation or inclination, providing a crucial relationship between the angles of the figures. This property helps in identifying and proving similarity between geometric shapes, and it is a fundamental concept in geometry that allows for the comparison and analysis of different shapes and their angles.
How are corresponding sides related in similar figures?
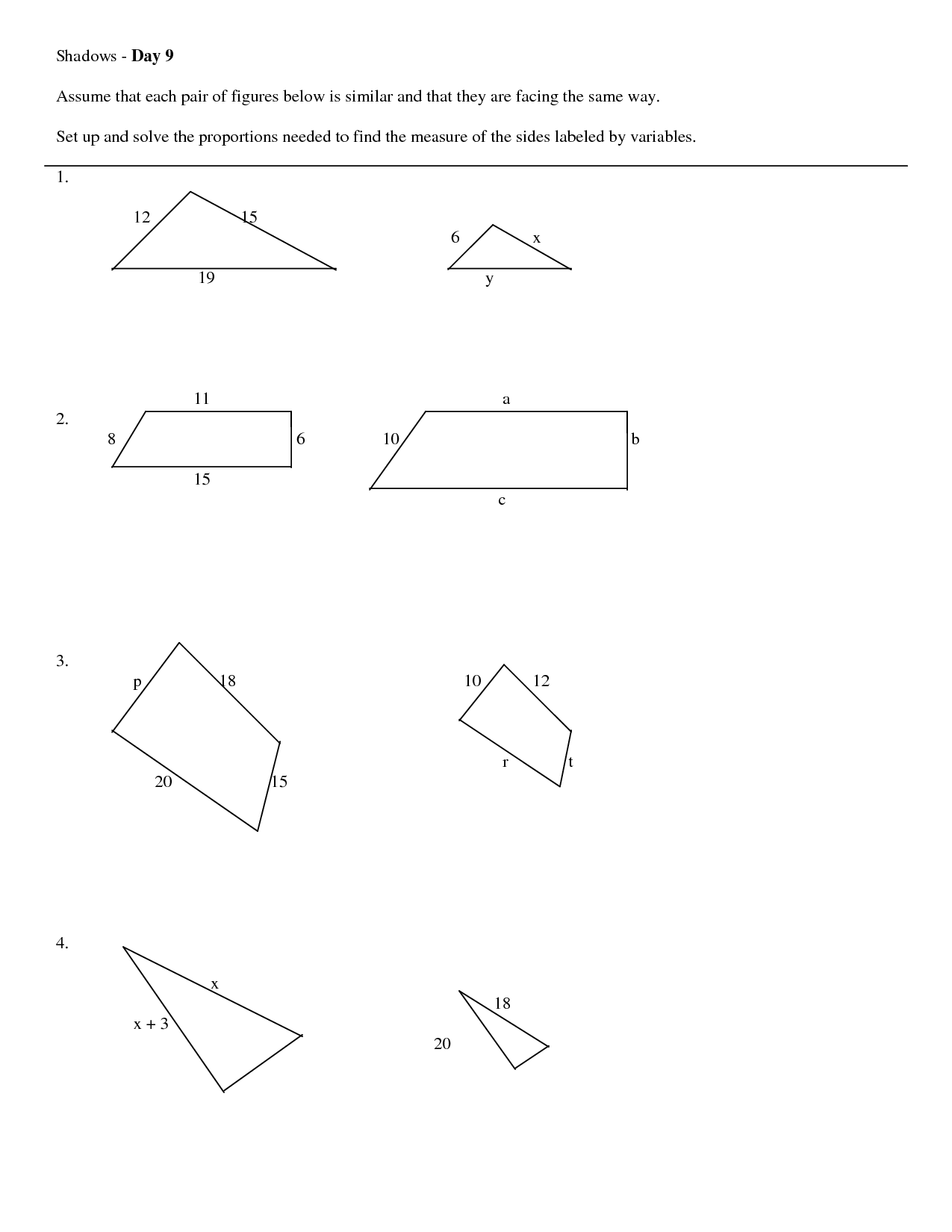
In similar figures, corresponding sides are proportional. This means that if two figures are similar, then the ratio of the lengths of corresponding sides in the two figures will be the same. For example, if two triangles are similar, then their corresponding sides will be in the same ratio. This property allows us to determine missing side lengths in similar figures using proportions.
How can you use scale factor to find missing lengths in similar figures?
You can use the scale factor to find missing lengths in similar figures by setting up a proportion. To do this, compare the corresponding side lengths of the two similar figures and set up a ratio using the scale factor. Then, cross multiply to find the missing length. By using the scale factor to compare the sizes of corresponding sides in similar figures, you can easily determine the missing lengths.
What is the relationship between the perimeters of similar figures?
The relationship between the perimeters of similar figures is that they are proportional to the ratio of their corresponding sides. This means that if two figures are similar, their perimeters will have the same scale factor as their corresponding sides. In other words, if the linear dimensions of a figure are all multiplied by a certain factor to obtain a similar figure, then the perimeter of the similar figure will also be multiplied by the same factor.
How do you find the area of a figure that is similar to another figure?
To find the area of a figure that is similar to another figure, you can use the scale factor between the two figures. Square the scale factor to find the area scale factor, then multiply the area of the original figure by the area scale factor to get the area of the similar figure.
How does the volume of a three-dimensional figure change in similar figures?
The volume of a three-dimensional figure increases or decreases according to the cube of the scale factor between similar figures. This means that if the scale factor is k, then the volume will change by a factor of k³. So, if one figure is an enlargement of another by a scale factor of 2, then the volume of the larger figure will be 2³ = 8 times the volume of the smaller figure. Similarly, if one figure is a reduction of another by a scale factor of 1/2, then the volume of the smaller figure will be (1/2)³ = 1/8 times the volume of the larger figure.
Is it possible for two figures with the same shape to not be similar? Why or why not?
Yes, it is possible for two figures with the same shape to not be similar. This could occur if the figures are scaled or distorted differently, such that their corresponding sides are not proportional. In order for two figures with the same shape to be similar, they must not only have the same shape but also corresponding angles that are equal and corresponding sides that are in proportion to each other.
How can similar figures be used to solve real-world problems?
Similar figures can be used to solve real-world problems by allowing us to analyze and compare information, such as dimensions or proportions, in different contexts. By recognizing and understanding the relationships between similar figures, we can make calculations and predictions about real-world situations, such as scaling up or down measurements, estimating distances or sizes, and designing structures or objects with similar shapes but different scales. This approach helps in solving problems involving maps, blueprints, 3D modeling, and much more, providing a valuable tool for practical applications in various fields such as architecture, engineering, and design.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.
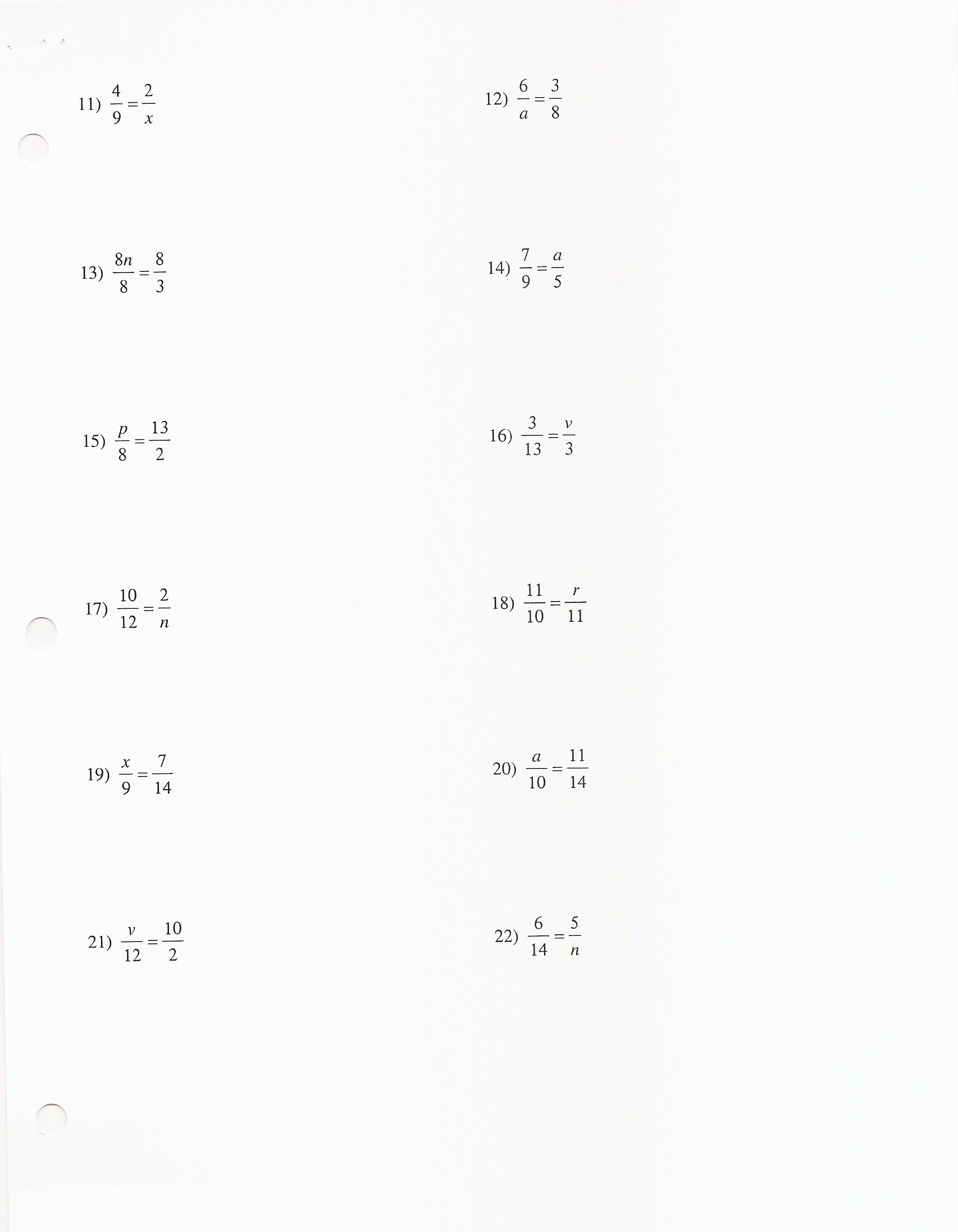
Comments