Multiply Add Subtract Polynomials Worksheet
Polynomials can be quite challenging to work with, especially when it involves multiplying, adding, and subtracting them. If you're a math enthusiast or a student looking for practice materials to enhance your skills in this particular area, you've come to the right place. In this blog post, we will introduce you to a variety of worksheets that focus specifically on multiplying, adding, and subtracting polynomials. These worksheets are designed to help you improve your understanding and mastery of these fundamental mathematical operations.
Table of Images 👆
- Adding Polynomials Worksheet
- Multiplying Polynomials Worksheet
- Adding Polynomials Worksheet
- How to Add Subtract Multiply and Divide Fractions
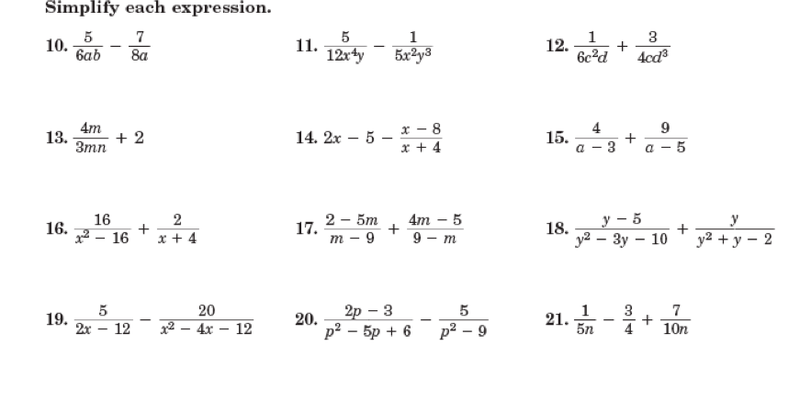
- Polynomials Adding and Subtracting Fractions
- Two-Step Equation Word Problems Worksheets
- Adding and Subtracting Fractions with Variables
- Graphing Quadratic Equations Worksheet PDF
- Algebra 2 Worksheets with Answers 1-6
- Rational Equations Worksheet with Answers
- Complex Numbers Dividing by Polynomials
- Adding and Subtracting Integers Worksheet
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
What is a polynomial?
A polynomial is an algebraic expression consisting of variables, coefficients, and exponents combined through addition, subtraction, and multiplication operations. It is a mathematical expression that can have one or more terms, with each term having a variable raised to a non-negative integer power. Polynomials are fundamental in various mathematical fields and can represent a wide range of functions and relationships.
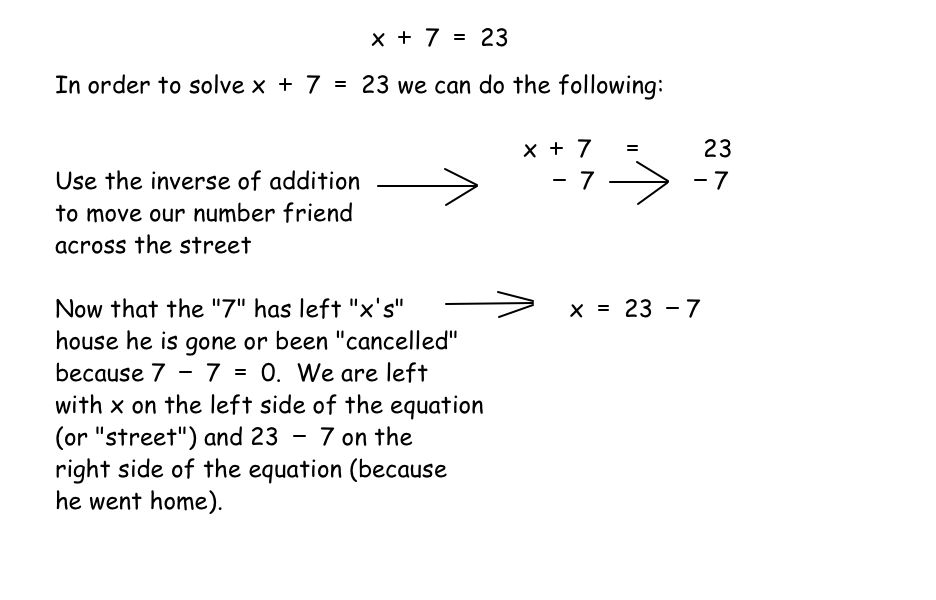
How do you add polynomials?
To add polynomials, you simply combine like terms by adding or subtracting the coefficients of terms with the same variables and exponents. Arrange the terms in descending order by the variable's exponent, and then add or subtract the coefficients to get the final sum. Keep in mind that you cannot combine terms that have different variables or exponents.
How do you subtract polynomials?
To subtract polynomials, you simply distribute the negative sign across each term of the polynomial you are subtracting. Then, you combine like terms by adding or subtracting the coefficients of terms with the same variable and exponent. Make sure to pay attention to the signs of the terms as you combine them.
How do you multiply polynomials?
To multiply polynomials, you use the distributive property to multiply each term in the first polynomial by each term in the second polynomial, and then combine like terms by adding or subtracting them. This process involves multiplying each term in one polynomial by each term in the other polynomial, and then simplifying the resulting expression by combining like terms.
What is the degree of a polynomial?
The degree of a polynomial is the highest power of the variable within the polynomial expression. It helps determine the behavior and complexity of the polynomial function, such as the number of roots or turning points it may have.
Can a polynomial have negative coefficients?
Yes, a polynomial can have negative coefficients. Coefficients in a polynomial can be positive, negative, or zero, and they represent the constant factors in the terms of the polynomial. Negative coefficients would simply imply that the corresponding terms in the polynomial have a negative impact on the overall value of the polynomial when evaluated.
What are like terms in a polynomial?
Like terms in a polynomial are terms that have the same variables raised to the same powers. These terms can be combined by adding or subtracting their coefficients while keeping the variables unchanged. Like terms are essential for simplifying and manipulating polynomials during algebraic operations.
Can you simplify a polynomial expression?
Yes, I can help simplify a polynomial expression by combining like terms, performing any necessary operations, and ultimately reducing the expression to its simplest form. Just provide me with the polynomial expression you would like me to simplify.
What is the distributive property used for in polynomial multiplication?
The distributive property is used in polynomial multiplication to simplify the process by distributing each term in one polynomial to all terms in the other polynomial. This means that each term in one polynomial is multiplied by each term in the other polynomial, ensuring that every combination is accounted for in the final product. This property allows for a systematic and organized method of multiplying polynomials, leading to a more efficient and accurate solution.
How can polynomial operations be applied in real-life situations?
Polynomial operations can be applied in real-life situations such as in engineering for modeling electrical circuits, in finance for calculating interest rates, in physics for describing motion, and in computer science for signal processing. By using polynomial operations, we can analyze and solve complex problems efficiently by representing various phenomena with mathematical expressions involving polynomials.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.












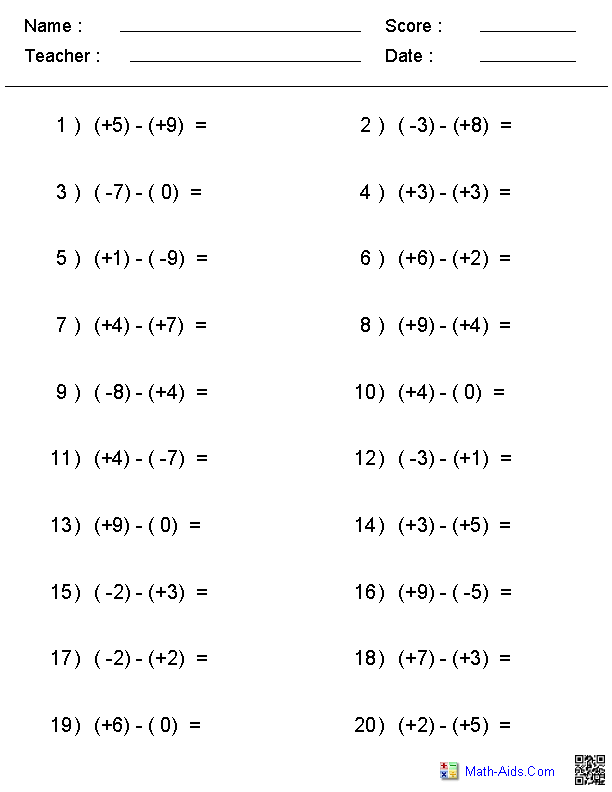














Comments