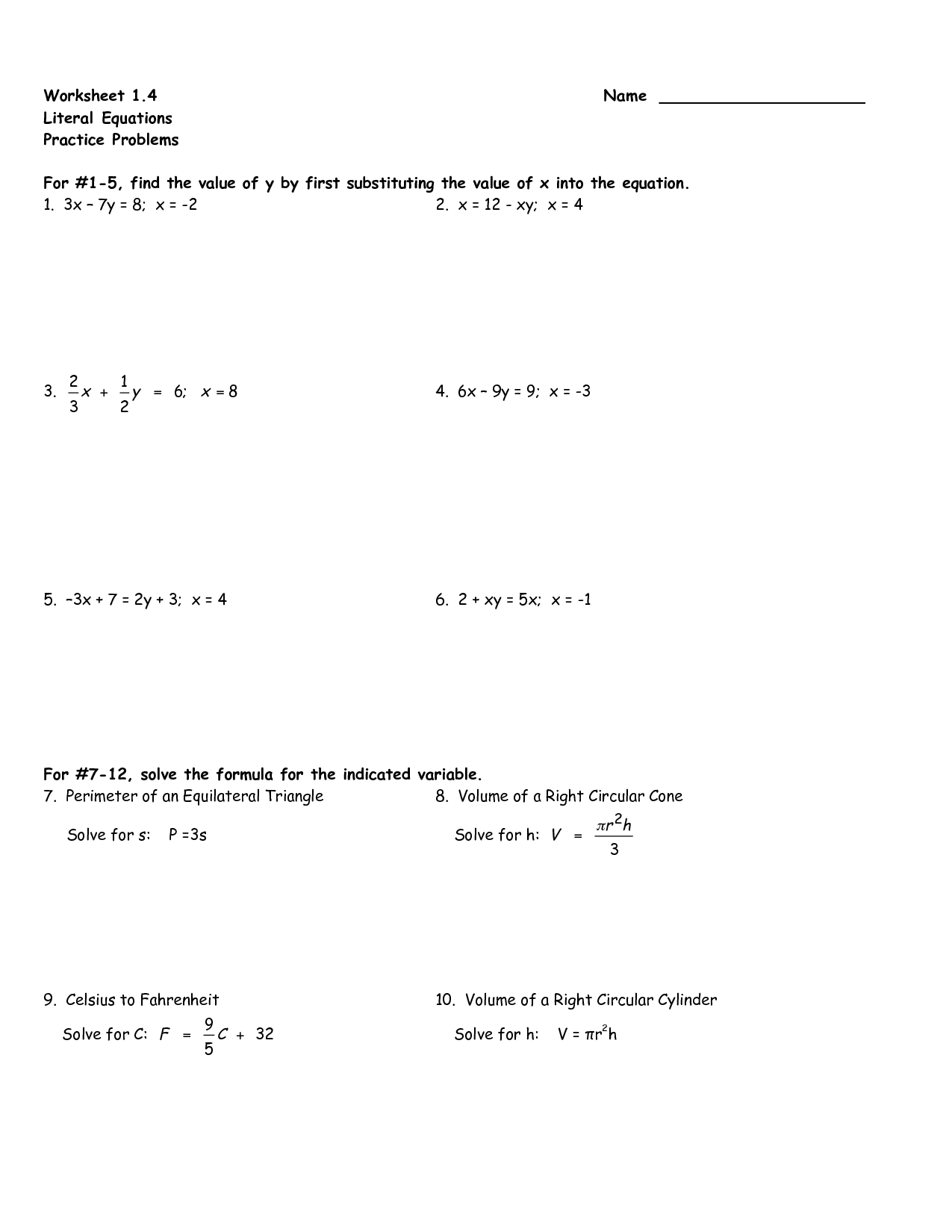
Literal Equations Worksheets 8th Grade

Literal equations can be a challenging topic for 8th grade students. These worksheets provide an excellent resource for practicing and mastering the concept of solving equations with multiple variables. With a focus on entity and subject, these worksheets offer a structured approach to help students develop their problem-solving skills and gain confidence in solving literal equations.

  9th Grade Algebra Printable Worksheets

---

  Literal Equations Worksheet Answer Key

---

  Literal Equations Practice Worksheet

---

  Proportions Worksheets 7th Grade

---

  Free Printable Worksheets On Health

---

  Solving Two-Step Equations Worksheet Answers

---

  Solving Two-Step Equations Worksheet

---

  Algebra Equations Worksheets

---

  Newtons Laws of Motion Worksheets

---

  Solving Linear Equations and Inequalities

---

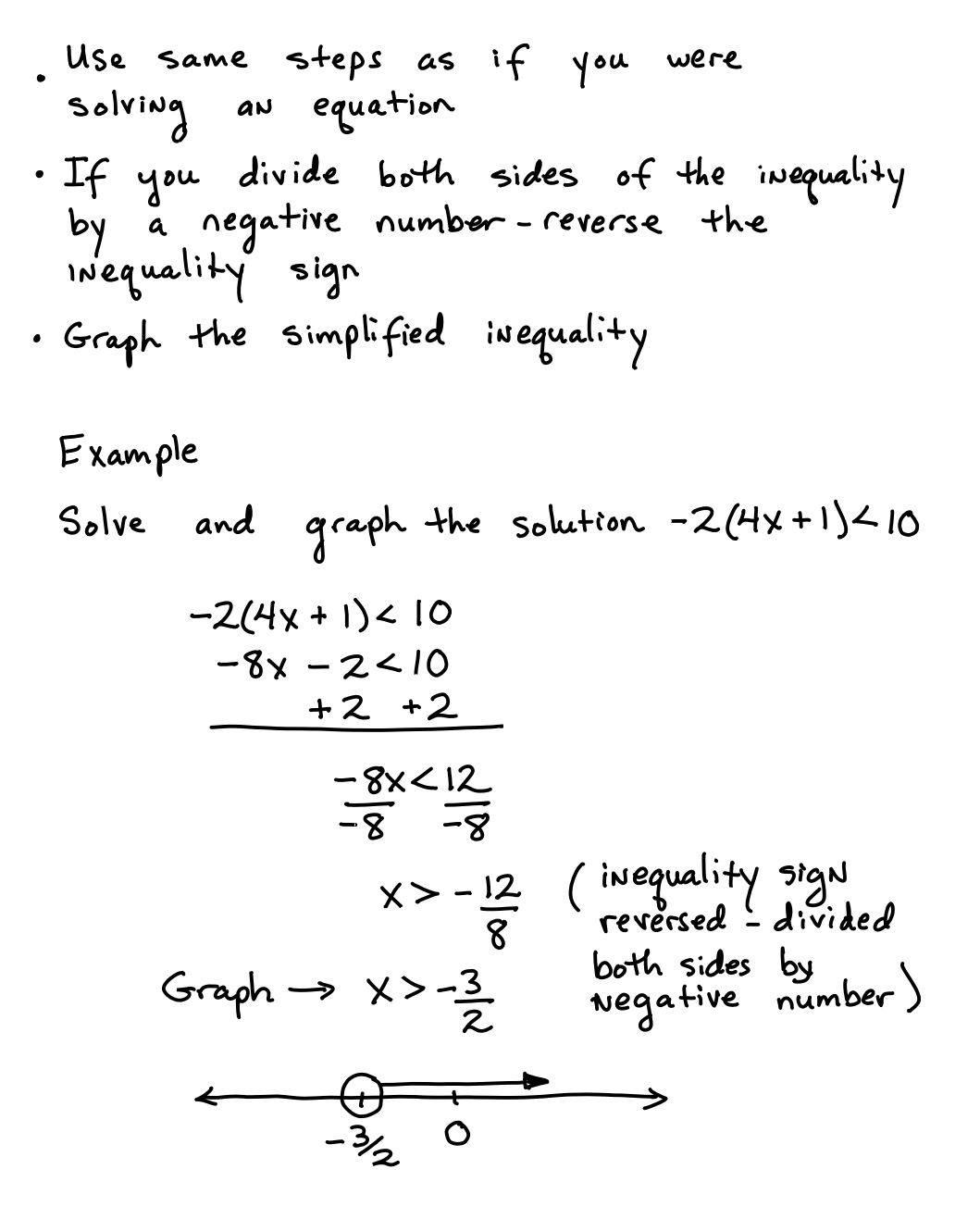
What is a literal equation?

A literal equation is an equation that contains multiple variables and constants. In a literal equation, the goal is usually to solve for one variable in terms of the others, rather than finding a numerical value. Literal equations are commonly used in mathematics and science to represent relationships between different quantities.

How do you solve a literal equation for a specific variable?

To solve a literal equation for a specific variable, you need to isolate the variable on one side of the equation. Follow the same steps as you would for solving a regular algebraic equation, but this time make sure the variable you are solving for is the only one left on the side you want. Perform inverse operations to move terms around until the variable is by itself. Remember to perform the same operations on both sides of the equation to maintain equality.

Can literal equations be used in real-world situations? If so, provide an example.

Yes, literal equations can be used in real-world situations. An example of this is in the field of engineering, where formulas are commonly used to determine properties such as voltage, current, resistance, and power in electrical circuits. By manipulating literal equations such as Ohm's Law (V = IR) or the power formula (P = IV), engineers can analyze and design electrical systems for various applications, such as lighting, telecommunications, and electronics.

How do you use the distributive property to solve a literal equation?

To solve a literal equation using the distributive property, you can distribute a number to each term on one side of the equation to simplify the expression. This helps isolate the variable on one side of the equation, making it easier to solve for its value. Just remember to apply the same operation to both sides of the equation to maintain its equality.

What is the process for isolating a variable in a literal equation?

To isolate a variable in a literal equation, you need to perform a series of reverse operations to move all other terms to the other side of the equation, leaving the variable alone on one side. Start by identifying the variable you want to isolate and then use inverse operations to move terms away from the variable. This may involve addition, subtraction, multiplication, or division. Keep transitioning terms until the variable is by itself on one side of the equation, and then you have successfully isolated the variable.

Can variables be on both sides of a literal equation? Explain.

No, variables cannot be on both sides of a literal equation. In a literal equation, variables are equivalent to numbers and must adhere to the rules of solving an equation, which include maintaining equality by isolating the variable on one side of the equation. Having variables on both sides would disrupt the balance of the equation and render it unsolvable.

How does substitution play a role in solving a literal equation?

Substitution plays a significant role in solving a literal equation by allowing you to replace one variable with an equivalent expression in terms of another variable. This method helps simplify the equation and solve for the desired variable by breaking down complex relationships into more manageable parts. By substituting known values or expressions into the equation, you can isolate the variable of interest and find the solution more effectively.

What is the difference between simplifying and solving a literal equation?

Simplifying a literal equation involves reducing the equation to its simplest form by combining like terms and getting rid of unnecessary elements, such as parentheses or fractions. On the other hand, solving a literal equation involves isolating a specific variable to determine its value. While simplifying focuses on streamlining the equation, solving aims to find the value of a particular variable within the equation.

How are literal equations related to algebraic expressions and formulas?

Literal equations are a type of algebraic equation where the variables are used to represent general numbers, rather than specific values. This makes them closely related to algebraic expressions, as they both involve manipulating symbols to express relationships between quantities. Formulas, on the other hand, are specific types of literal equations that represent mathematical relationships or rules. All three concepts—literal equations, algebraic expressions, and formulas—are fundamental tools in algebra for solving problems and representing mathematical concepts symbolically.

What are some common mistakes to avoid when solving literal equations?

Some common mistakes to avoid when solving literal equations include not isolating the variable correctly, forgetting to distribute or simplify terms, making errors in basic arithmetic operations, not applying the correct rules of solving equations, and missing any negative signs or terms when rearranging the equation. It is important to be systematic and careful with each step to ensure an accurate solution to the literal equation.