Inverse Functions Algebra 2 Worksheets
Inverse functions are a fundamental concept in algebra 2, and worksheets provide a valuable tool for practicing and reinforcing this concept. Designed for students who are familiar with functions and their properties, these worksheets provide ample opportunities to explore the relationship between an original function and its inverse. With carefully crafted questions and exercises, these worksheets guide students to develop a deeper understanding of inverse functions and how they can be utilized to solve equations and analyze real-world situations.
Table of Images 👆
- Inverse Trig Functions Worksheet
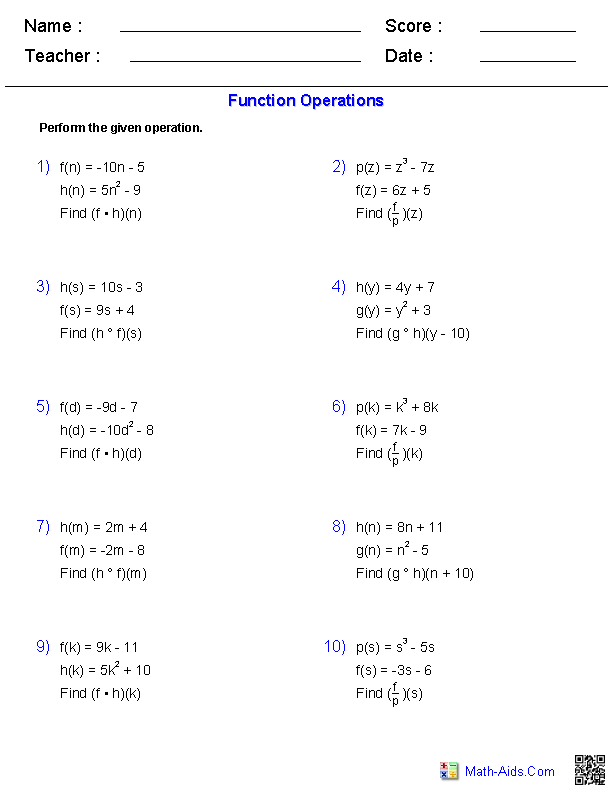
- Algebra 2 Function Operations Worksheet
- Inverse Functions Algebra 1 Worksheet
- Math Worksheets Inverse Functions
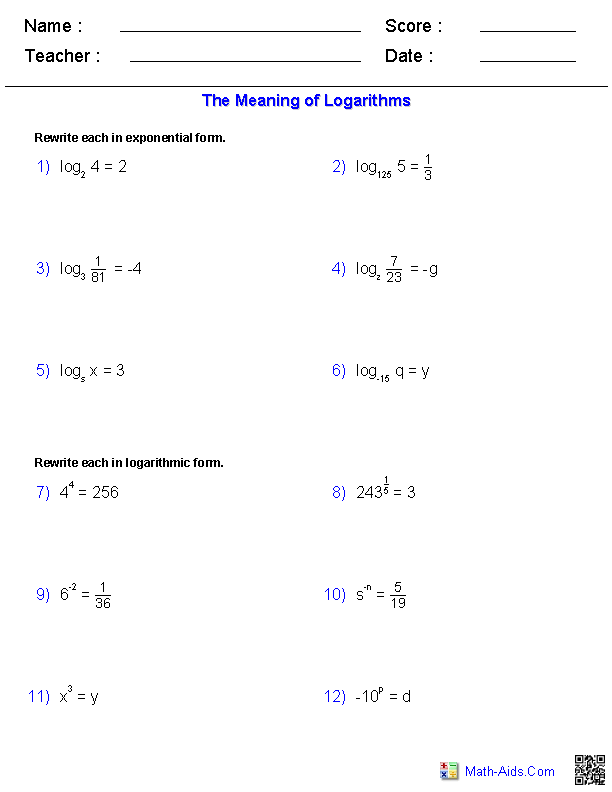
- Properties of Logarithms Algebra 2 Worksheet Answers
- Graph Inverse Functions Worksheet
- Direct and Inverse Variation Worksheet Answers
- Graph Inverse Functions Worksheet
- Properties of Logarithms Algebra 2 Worksheet Answers
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
What are inverse functions?
Inverse functions are functions that "undo" each other. In other words, if a function f(x) maps input values from set A to output values in set B, its inverse function, denoted as f^-1, maps the output values from set B back to the input values in set A. This means that applying f and then its inverse function f^-1 (or vice versa) to a value x results in x itself.
How do you find the inverse of a function algebraically?
To find the inverse of a function algebraically, you typically swap the variables x and y in the function and then solve for y. This means that you interchange the roles of x and y in the original function, and then rearrange the equation to isolate y. The resulting equation will represent the inverse function. It's important to verify that the original function is one-to-one, meaning that each input corresponds to exactly one output, to ensure that the inverse function exists.
Can every function have an inverse? Why or why not?
Not every function has an inverse. A function must be both one-to-one and onto (bijective) in order to have an inverse. If a function is not one-to-one (multiple inputs map to the same output), it does not have a unique inverse. Similarly, if a function is not onto (some outputs are not mapped to), it also does not have an inverse. So, the existence of an inverse for a function depends on its properties and whether it satisfies the conditions for being bijective.
What is the relationship between a function and its inverse?
The relationship between a function and its inverse is such that if a function f assigns each input x to a unique output y, then the inverse function f^-1 assigns each output y back to its unique input x. In other words, the inverse function undoes the action of the original function, such that applying them in succession results in the identity function, where f^-1(f(x)) = x for all x in the domain of f.
How can you determine if two functions are inverses of each other?
To determine if two functions are inverses of each other, you can follow these steps: 1) Compose the two functions by feeding the result of one function into the other function and simplify the expression. If you get the input value back, they are inverses. 2) Find the composite function f(g(x)) and g(f(x)) and check if they simplify to x. 3) Plot the functions and see if the resulting graph is a reflection of the line y = x. If both tests confirm these conditions, then the two functions are inverses of each other.
What is the notation used to represent inverse functions?
The notation used to represent inverse functions is f^(-1)(x), where f(x) is the original function.
What is the domain and range of a function and its inverse?
The domain and range of a function refer to the set of input values and output values, respectively. For the function f(x), the domain is the set of all possible input values of x for which f(x) is defined, while the range is the set of all possible output values produced by the function. For the inverse of a function f^(-1)(x), the domain and range are essentially switched - the domain of the inverse function is the range of the original function, and the range of the inverse function is the domain of the original function.
How does the graph of a function relate to the graph of its inverse?
The graph of a function and its inverse are reflections of each other across the line y = x. This means that if you plot the function f(x) and its inverse f^(-1)(x) on the same coordinate plane, they will be symmetrical with respect to the line y = x. This relationship is due to the fact that the inverse of a function "undoes" the original function, resulting in their graphs being mirror images of each other.
What are some real-world applications of inverse functions?
Inverse functions have various real-world applications, including in cryptography for encryption and decryption processes, in optimization problems to find the original input values based on the output, in engineering for modeling and solving equations, in finance for calculating interest rates or investment returns, in medicine for analyzing drug dosages based on its effectiveness, and in computer graphics for transformations and animations. Overall, inverse functions help in solving problems where the reversal of a function's process is needed to determine the original input values from the output.
Can a function and its inverse be the same? Explain why or why not.
No, a function and its inverse cannot be the same because for a function to have an inverse, it must be a one-to-one function, meaning that each input corresponds to exactly one output. If a function and its inverse were the same, then there would be multiple outputs corresponding to the same input, violating the definition of a one-to-one function. Additionally, the composition of a function and its inverse should result in the identity function, which would not be possible if they were the same.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.









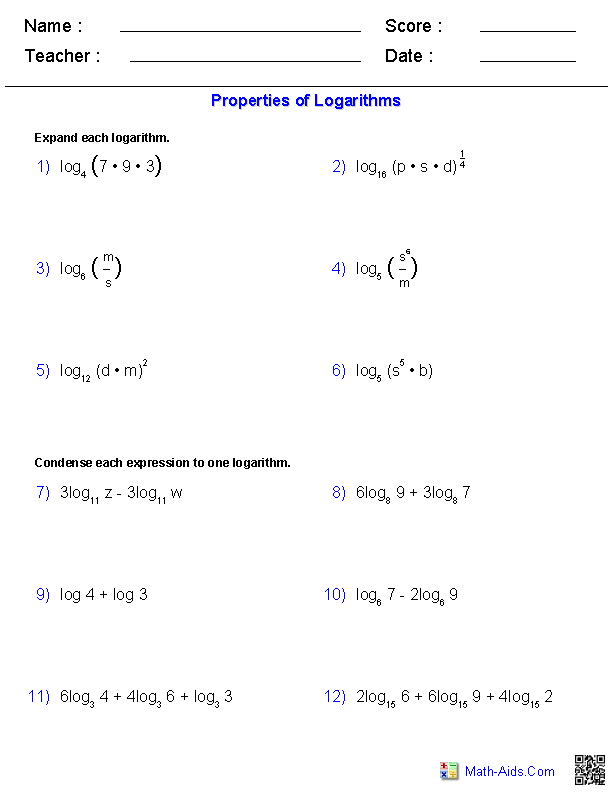














Comments