Inequalities Worksheet Kuta Software

If you're a math teacher in search of a comprehensive set of inequalities worksheets, Kuta Software has you covered. With a range of exercises covering various forms of inequalities, this resource is perfect for middle and high school students looking to solidify their understanding of this important topic.

  Solving Inequalities Worksheets with Answers

---

  Algebra 1 Kuta Software Compound Inequalities Worksheet

---

  Graphing Lines Kuta Software Infinite Algebra 1 Answers Key

---

  Kuta Graphing Linear Equations Worksheets

---

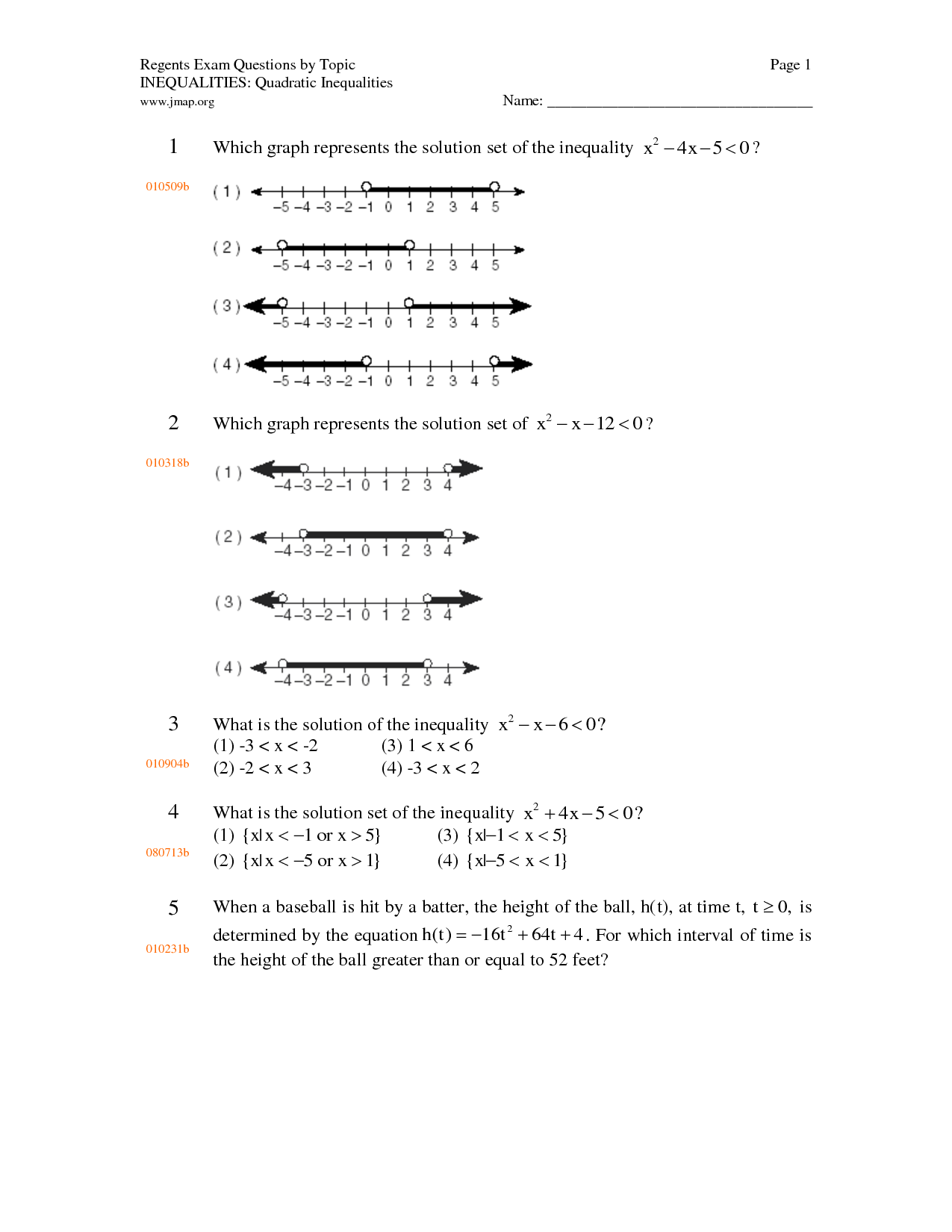
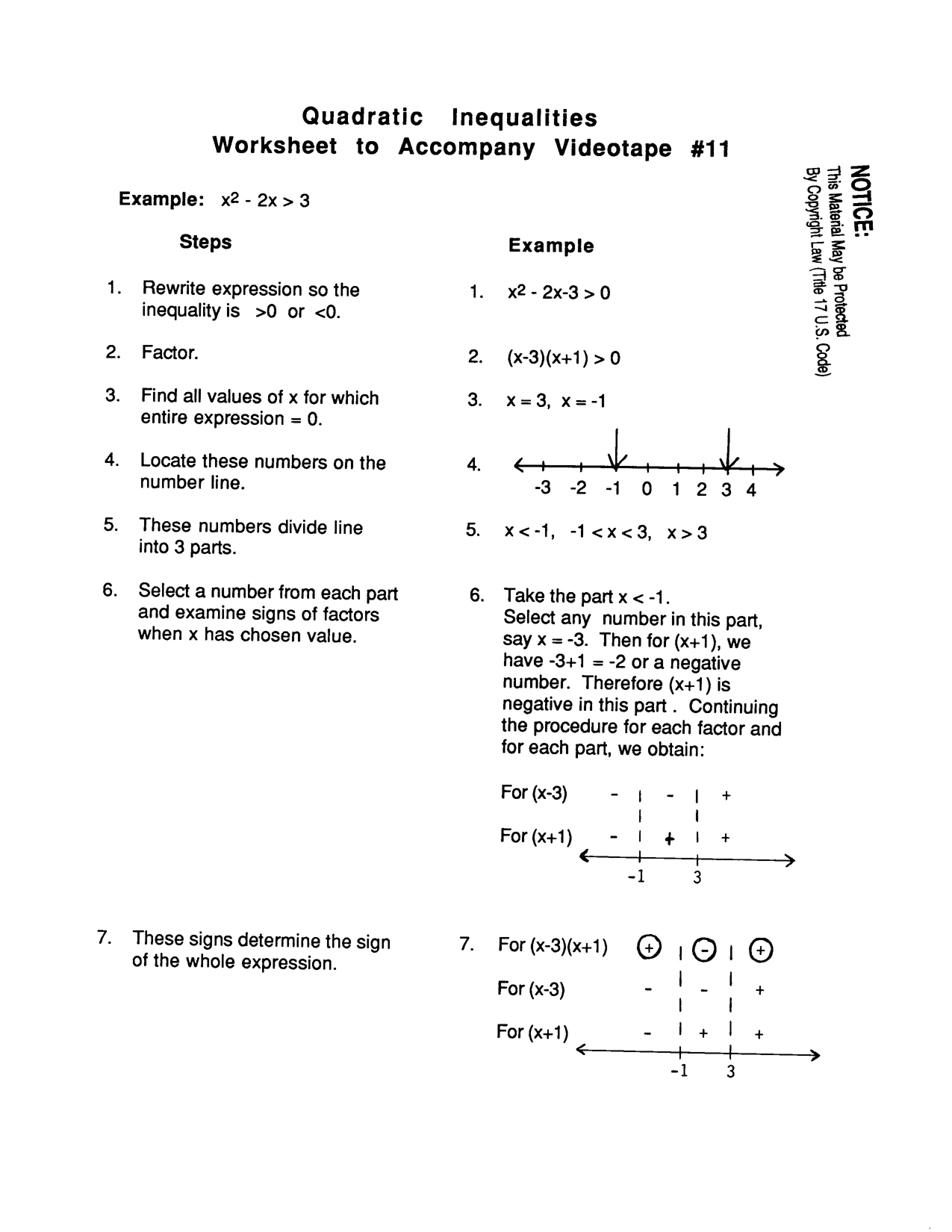
  Graphing Quadratic Inequalities Worksheets

---

  Infinite Algebra 1 Solving Systems of Inequalities

---

  Kuta Software Graphing Linear Inequalities

---

  Proportion Word Problems Worksheet

---

  Quadratic Inequality Worksheet

---

  Kuta Software Infinite Algebra 1 Graphing Lines

---

  Scientific Notation

---

  Kuta Software Infinite Geometry Angles in a Triangle

---

What is the purpose of solving inequalities in mathematics?

The purpose of solving inequalities in mathematics is to determine the range of values that satisfy a given mathematical statement. By solving inequalities, we can find the possible values of a variable that make a mathematical expression or equation true, allowing us to make decisions and draw conclusions based on these relationships.

How does the solution set of an inequality differ from the solution set of an equation?

The solution set of an inequality represents all the values that satisfy the inequality statement, which can include a range of values rather than just specific points. In contrast, the solution set of an equation represents only the specific values that make the equation true. Additionally, inequalities may have multiple solutions that satisfy the inequality statement, whereas equations typically have a unique solution.

What are the different types of inequalities (e.g., less than, greater than)?

In mathematics, the different types of inequalities include less than (<), greater than (>), less than or equal to (?), greater than or equal to (?), not equal to (?), and strict inequality (< or >, without the equal sign). These symbols are used to compare two values or expressions and represent the relationship between them in terms of size or magnitude.

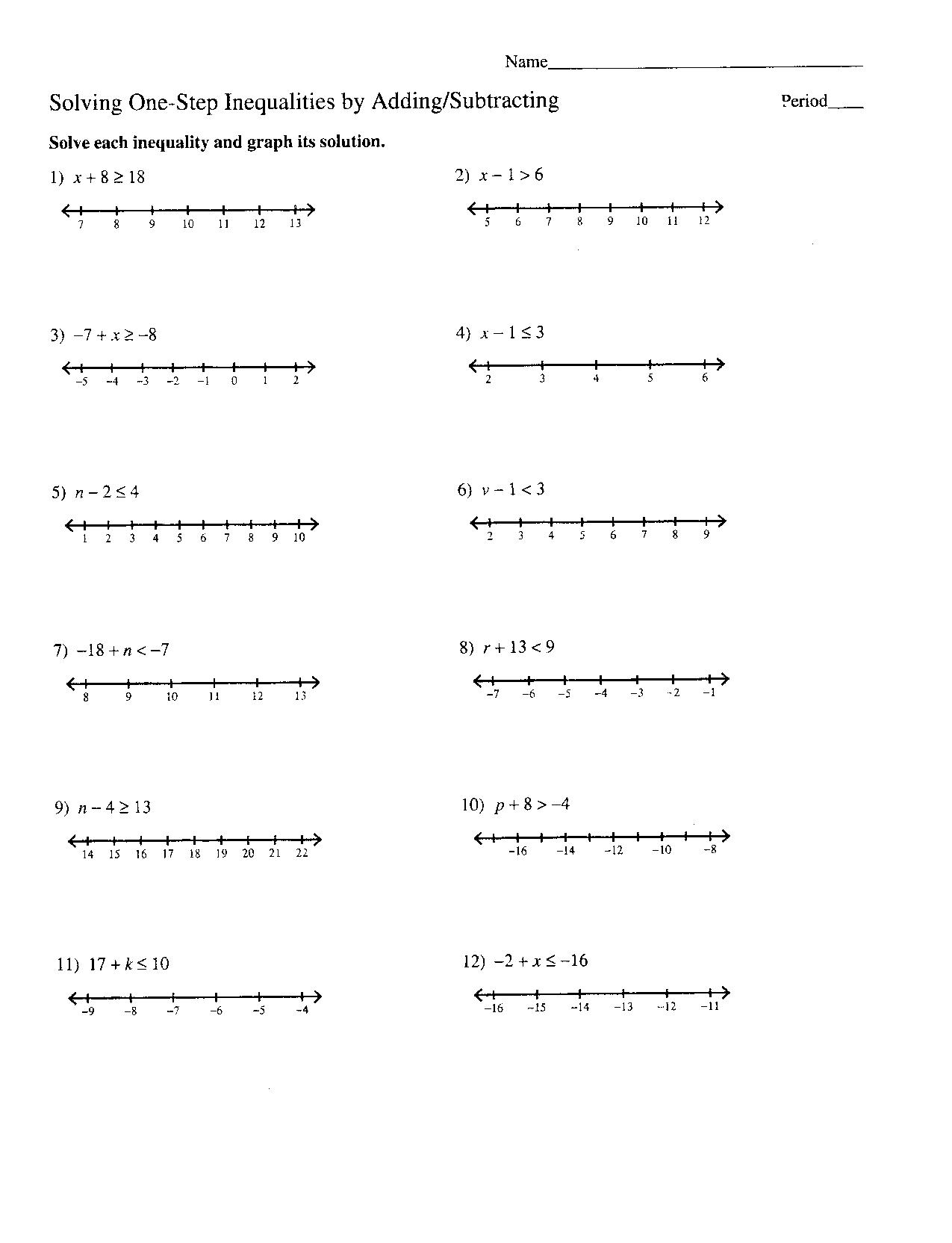
How can inequalities be represented on a number line?

Inequalities can be represented on a number line by using open or closed circles to indicate the boundaries of the inequality and shading the region that satisfies the inequality. For example, for the inequality x > 3, you would put an open circle at 3 on the number line and shade everything to the right of 3 to represent all values greater than 3. If the inequality was x ? 3, you would use a closed circle at 3 to include it as part of the solution set.

What are the rules for solving and simplifying inequalities?

When solving and simplifying inequalities, the same rules for equations generally apply: you can add and subtract the same value on both sides, multiply or divide by a positive number without changing the inequality, and reverse the inequality sign when multiplying or dividing by a negative number. Remember to always keep track of sign changes when performing operations on inequalities and simplify them to their simplest form to get the final solution.

How can you determine if a value is in the solution set of an inequality?

To determine if a value is in the solution set of an inequality, you simply need to substitute that value into the inequality and see if the resulting statement is true. If the inequality is true when the value is substituted in, then it is in the solution set; if the inequality is false, then the value is not in the solution set.

What is the significance of the direction of the inequality symbol?

The direction of the inequality symbol (< or >) signifies the relationship between the two quantities being compared. If the symbol points to the left (<), it indicates that the quantity on the left is smaller than the quantity on the right. On the other hand, if the symbol points to the right (>), it indicates that the quantity on the left is larger than the quantity on the right. This directionality is crucial for understanding the relative size or magnitude of different values in mathematical and real-world scenarios.

How can you graph the solution set of a compound inequality?

To graph the solution set of a compound inequality on a number line, you plot the solutions of each individual inequality and then combine them. For "and" inequalities, you shade the overlapping region where both inequalities are true. For "or" inequalities, you shade the sections where either inequality is true. This allows you to visually represent the solution set and easily understand the range of values that satisfy the compound inequality.

What are the common mistakes to avoid when solving inequalities?

Common mistakes to avoid when solving inequalities include switching the inequality symbol incorrectly when multiplying or dividing by a negative number, forgetting to flip the symbol when squaring or taking the square root of both sides, not distributing negative signs properly when simplifying expressions, and overlooking the restrictions on variables such as dividing by a variable that could potentially be zero. It is essential to pay close attention to these details to accurately solve inequalities.

How are inequalities used in real-world applications, such as problem-solving or decision-making?

Inequalities are used in real-world applications for problem-solving and decision-making by representing constraints or limitations that need to be considered. For example, in budgeting decisions, inequalities can be used to determine maximum spending limits or to compare costs of different options. In fields like economics or engineering, inequalities are used to optimize resources, such as determining the maximum profit that can be achieved given a set of constraints. In healthcare, inequalities can be used to assess risk factors for certain diseases or conditions based on specific criteria. Overall, inequalities play a crucial role in practical situations by providing a framework for making informed decisions and solving complex problems efficiently.