Geometric Math Patterns Worksheets Middle School

Are you searching for engaging and educational resources to reinforce geometric math patterns for middle school students? Look no further! Our comprehensive collection of worksheets is specifically designed to help students solidify their understanding of geometric concepts and patterns. Whether you are a teacher looking for supplemental materials or a parent seeking additional practice for your child, our worksheets provide a valuable learning opportunity for students at this level.

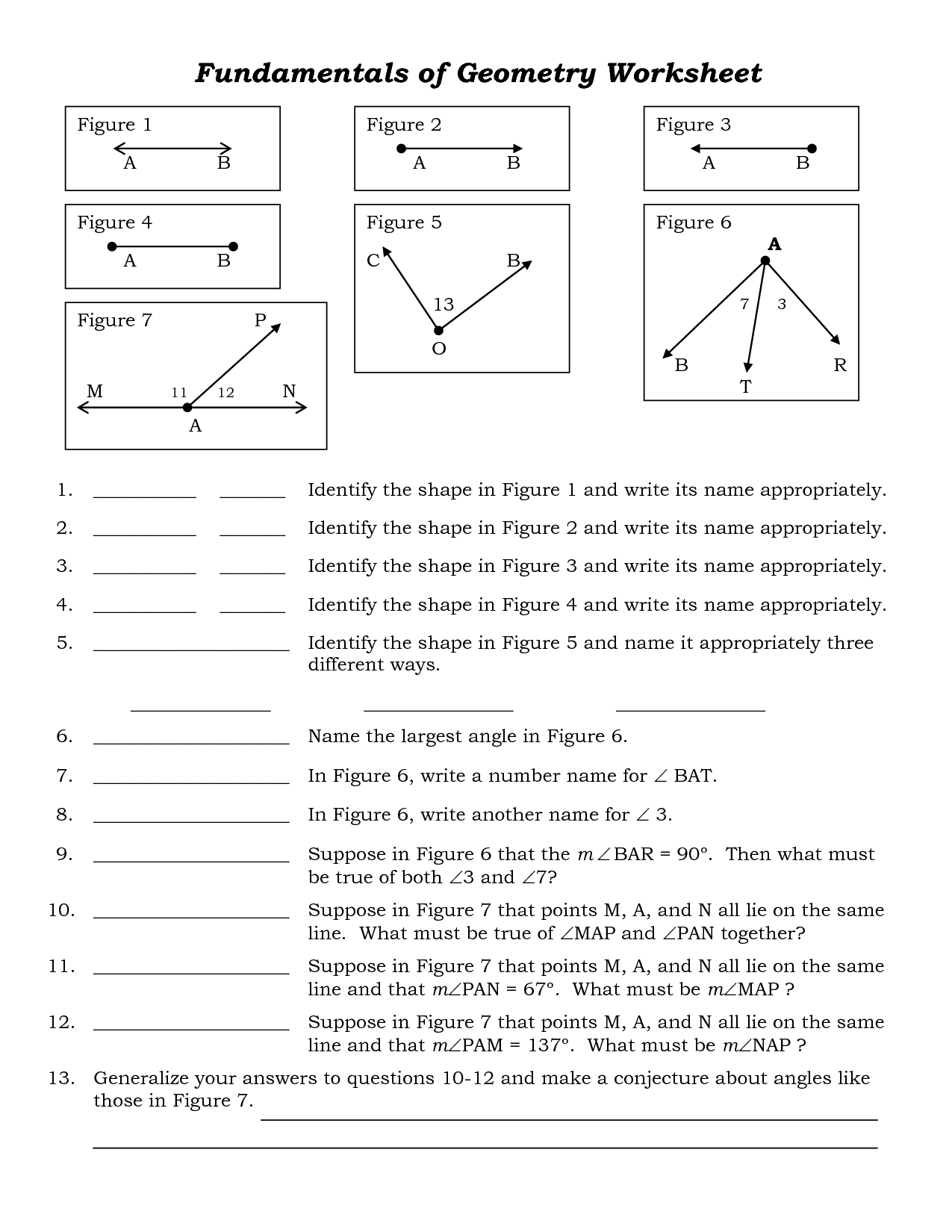
  High School Geometry Math Worksheets

---

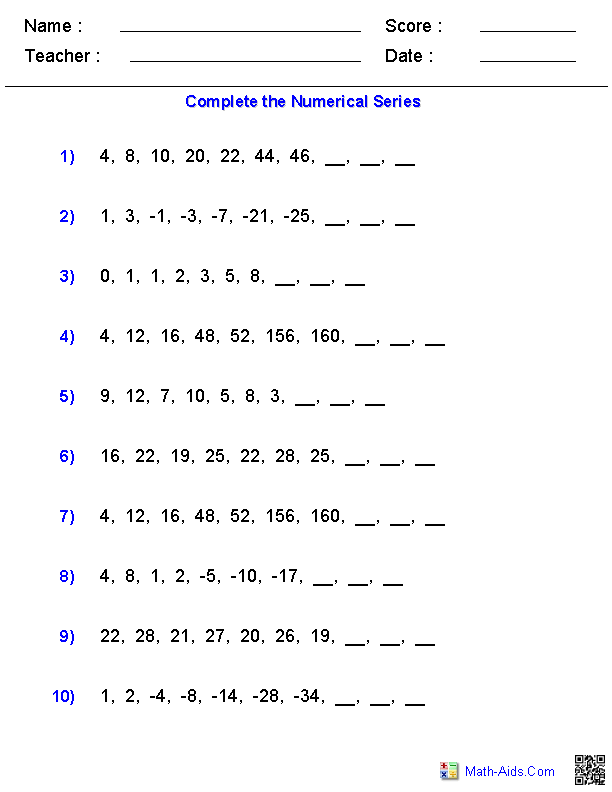
  Geometric Sequences Worksheet Answers

---

  High School Math Worksheets Printable

---

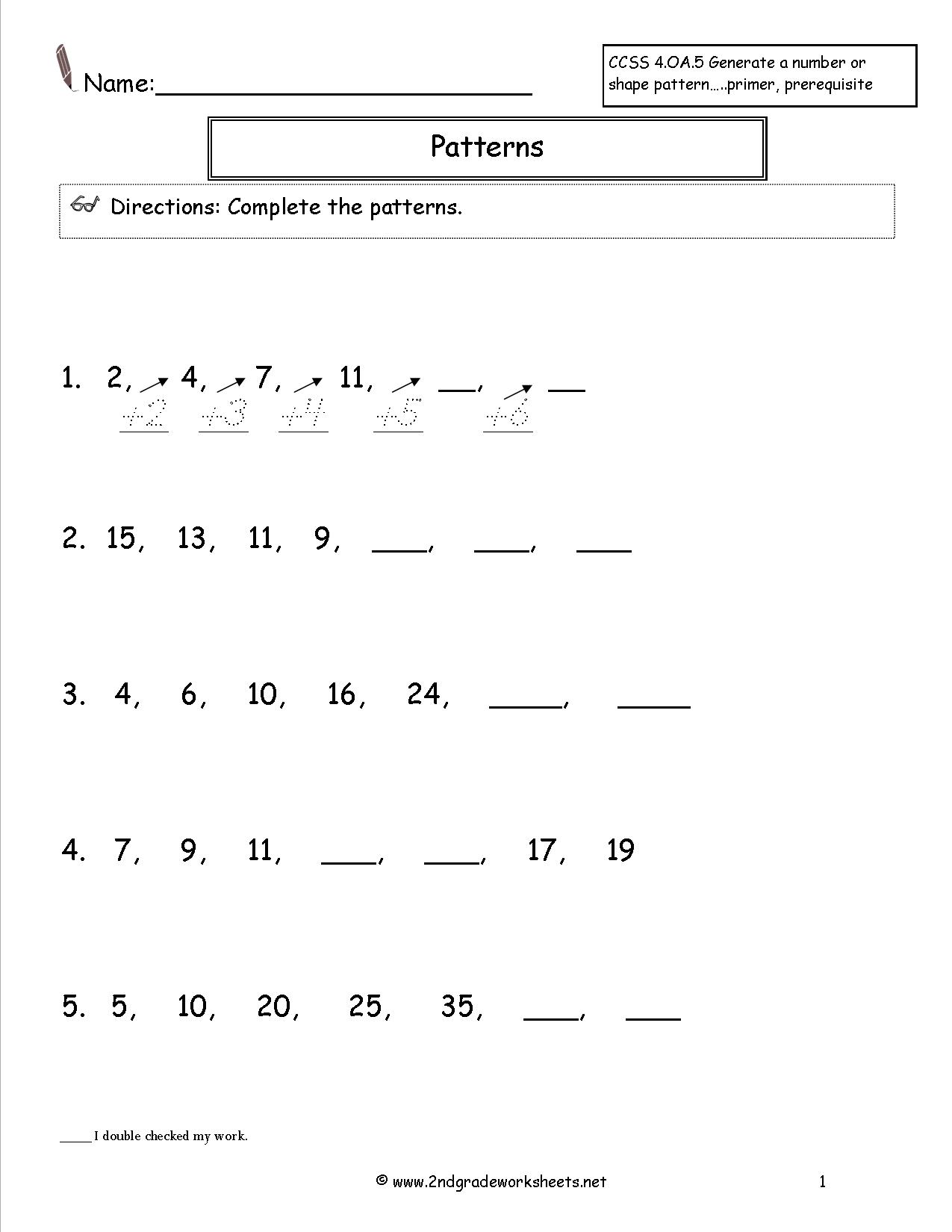
  Number Patterns Worksheets 3rd Grade Math

---

  High School Math Worksheets Printable

---

  Arithmetic Geometric Sequences Worksheet

---

  Holt Middle School Math Course 2 Answer Key

---

  Middle School Math Worksheets Printable

---

  High School Geometry Worksheets

---

  Number Patterns Worksheets

---

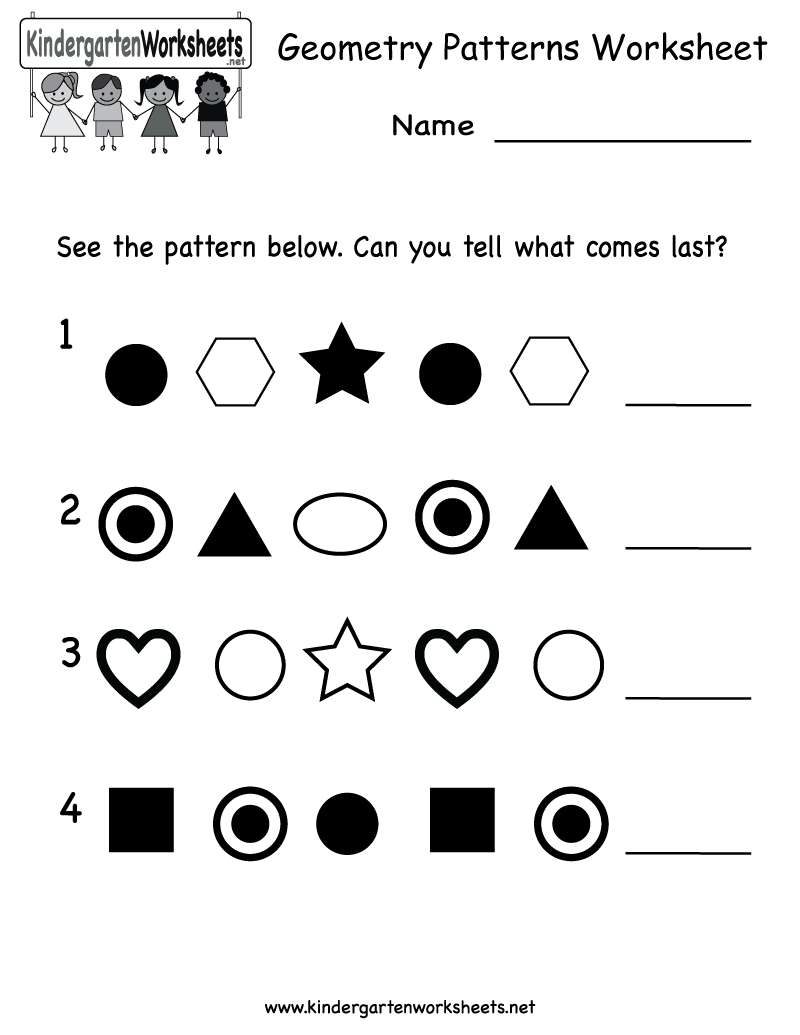
  Kindergarten Math Patterns Worksheets

---

  High School Math Worksheets Printable Fractions

---

What is a geometric pattern?

A geometric pattern is a visually appealing design that is created using geometric shapes such as lines, circles, triangles, squares, and polygons. These patterns often repeat in a regular arrangement to form intricate and symmetrical designs that can be found in architecture, art, textiles, and various other forms of visual expression.

What are some examples of geometric patterns in everyday life?

Geometric patterns can be found in many aspects of everyday life, such as in architecture with the use of grids or symmetrical designs in buildings, in textiles with repetitive shapes or tessellations in fabrics and carpets, in nature with the hexagonal shape of honeycombs or the spiral pattern of a sunflower, and even in technology with the circuitry layout on a computer chip or the symmetrical design of a microchip. These patterns highlight the harmony and order found in our surroundings, showcasing how mathematics and geometry play a role in shaping the world around us.

How can you create a geometric pattern using shapes?

To create a geometric pattern using shapes, start by selecting a geometric shape such as a square, triangle, or circle as your base. Then, repeat this shape in a systematic and organized manner, either by rotating, mirroring, or scaling it. Experiment with varying sizes, colors, and orientations of the shape to create a visually interesting pattern. You can also combine different geometric shapes together to form more complex patterns. Use design principles like symmetry, repetition, and balance to enhance the overall aesthetic of your geometric pattern.

What are the characteristics of a tessellation pattern?

A tessellation pattern is characterized by shapes or figures that fit together seamlessly to cover a surface without overlapping or leaving gaps. These shapes are repeated in a regular, symmetrical manner to create a visually appealing and mathematically precise design. Tessellations can feature simple geometric shapes like triangles, squares, and hexagons, or more complex patterns with irregular shapes, depending on the artist's preferences. The patterns can be two-dimensional or three-dimensional, and are often used in art, architecture, and mathematics for their aesthetic and structural properties.

What is the Fibonacci sequence and how does it relate to geometric patterns?

The Fibonacci sequence is a series of numbers where each number is the sum of the two preceding ones, starting with 0 and 1 (0, 1, 1, 2, 3, 5, 8, 13, and so on). This sequence appears in many natural phenomena and is closely related to geometric patterns through the Fibonacci spiral and Fibonacci sequence in nature, where the ratios of successive Fibonacci numbers converge to the golden ratio. This ratio is prevalent in geometric patterns such as spirals in sunflowers, pinecones, and seashells, showcasing the interconnectedness of mathematics and nature.

How can you determine if a pattern is a fractal?

A pattern can be determined to be a fractal if it displays self-similarity on different scales, meaning that when you zoom in or out, the pattern retains similar or identical shapes or structures. Additionally, fractals often exhibit complexity and detail at all levels of magnification, with intricate and recurring geometric patterns. Fractals can also be characterized by having a fractional or non-integer dimension, such as a dimensionality between 1 and 2 in the case of a fractal line or curve.

What are transformational geometric patterns and how are they created?

Transformational geometric patterns are intricate designs created by repeating and transforming shapes or motifs in a systematic way. These patterns are often found in art, architecture, and decorative objects, and are characterized by their symmetry and complexity. They are typically created using basic geometric shapes such as circles, squares, triangles, and polygons, which are arranged and manipulated through rotation, reflection, translation, and scaling to generate visually captivating patterns. The process of creating transformational geometric patterns involves careful planning and attention to detail to ensure that the elements fit together harmoniously and maintain balance and coherence throughout the design.

How do symmetry and reflection play a role in geometric patterns?

Symmetry and reflection are fundamental concepts in creating, identifying, and understanding geometric patterns. Symmetry occurs when one shape or design can be transformed into an identical shape through reflection, rotation, or translation. Reflection, in particular, plays a significant role in creating symmetry in geometric patterns by mirroring a shape across a line to produce a visually balanced and harmonious design. Reflection is a powerful tool in expanding and manipulating geometric patterns, enhancing their aesthetic appeal and mathematical properties.

What are the different types of geometric patterns found in nature?

Geometric patterns found in nature include fractals, spirals, tessellations, branches, and waves. Fractals such as the branching patterns in trees or the intricate shapes of snowflakes exhibit self-similarity at different scales. Spirals, like the shells of snails or the arrangement of seeds in sunflowers, follow a Fibonacci sequence or golden ratio. Tessellations are repetitive patterns found in things like honeycombs or the scales of a snake. Branching patterns in rivers or blood vessels demonstrate a dendritic structure, while waves in water or sound propagate in geometric patterns such as sine waves.

How can studying geometric patterns improve problem-solving skills in math?

Studying geometric patterns can improve problem-solving skills in math by developing critical thinking, spatial reasoning, and logical deduction. By analyzing and manipulating geometric shapes and patterns, students can enhance their ability to identify relationships, make conjectures, and prove theorems. This process helps build problem-solving strategies that can be applied to a wide range of mathematical problems, fostering a deeper understanding of mathematical concepts and increasing proficiency in solving complex problems.