Factoring Trinomials Practice Worksheet
Are you struggling with factoring trinomials? If you need more practice and are searching for a resource that can help you better understand this topic, you've come to the right place. This blog post introduces a factoring trinomials practice worksheet that can be a valuable tool for students who want to strengthen their skills in factoring trinomials.
Table of Images 👆
- Factoring Polynomials Worksheet
- Factoring Quadratic Equations Worksheet Answers
- Polynomials and Factoring Practice Worksheet Answers
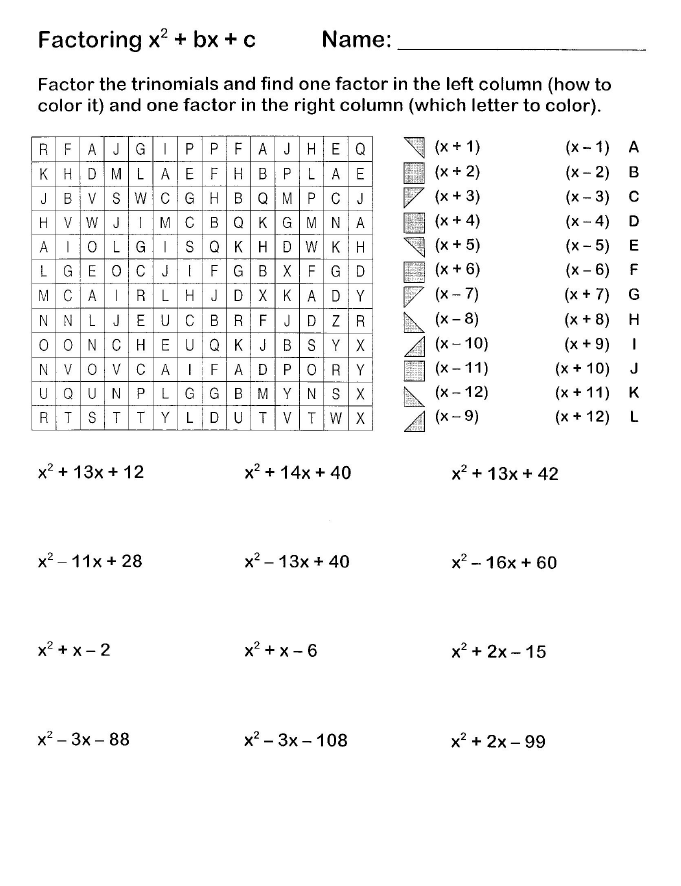
- Factoring Trinomials Worksheet Coloring
- Factoring Binomials Worksheet
- Factoring Greatest Common Factor Worksheet
- Algebra 2 Factoring Review Worksheet Answers
- Algebra Factoring Polynomials Worksheet
- Algebra 1 Factoring Problems and Answers
- Multiplying and Factoring Polynomials Worksheet
- Factoring Polynomials Worksheet with Answers
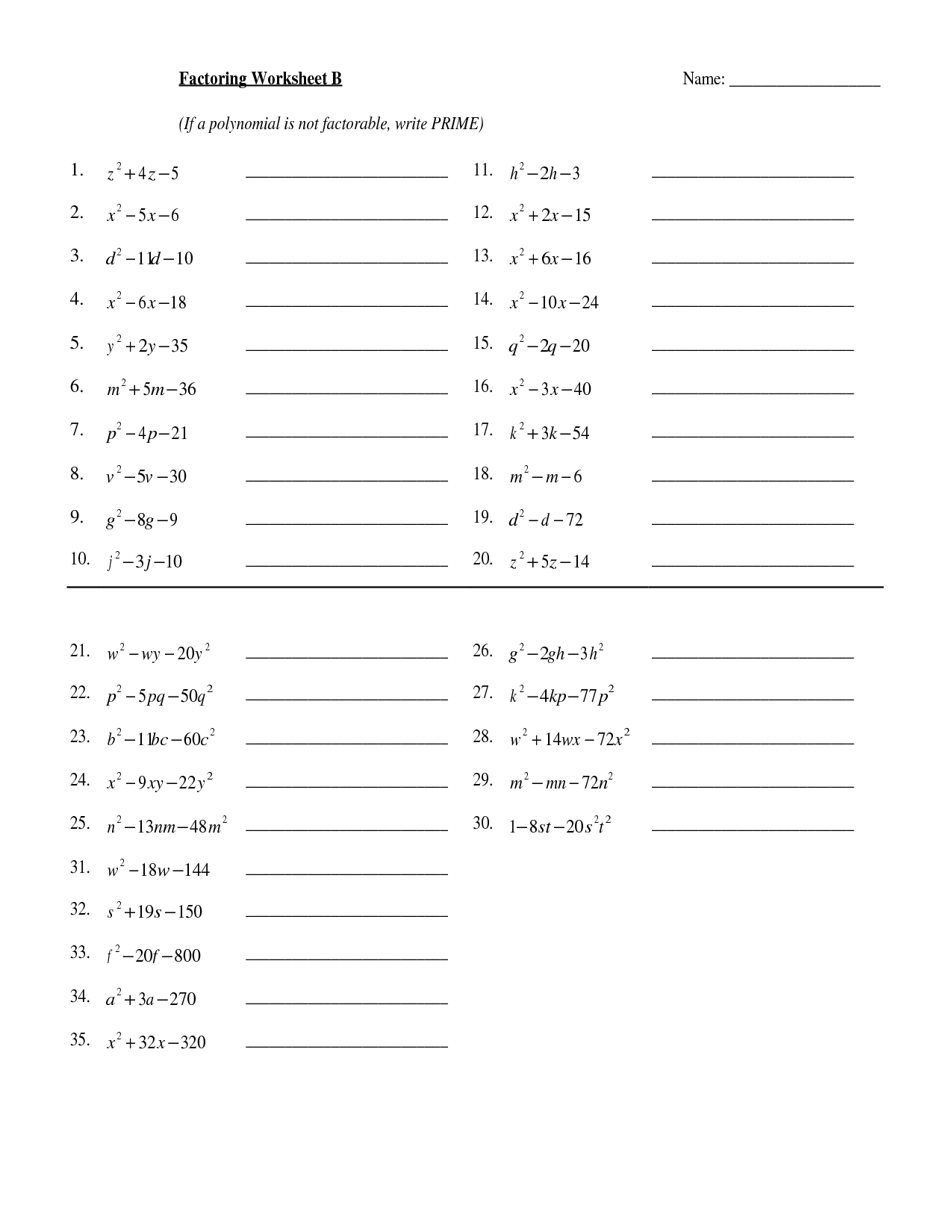
- Factoring Trinomials Worksheet
- Factoring Polynomials Worksheet
- Algebra 2 Factoring Polynomials Worksheet 1
- Factoring Quadratic Equations Worksheet
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is factoring?
Factoring is the process of breaking down a mathematical expression into smaller factors that, when multiplied together, equal the original expression. In simpler terms, it involves finding the numbers or algebraic expressions that can be multiplied to get the given expression. This technique is commonly used in mathematics to simplify equations and solve problems.
What is a trinomial?
A trinomial is a polynomial equation that consists of three terms. It can be expressed in the form of ax^2 + bx + c, where a, b, and c are coefficients and x is the variable raised to different powers.
How do you determine the greatest common factor (GCF) of a trinomial?
To determine the greatest common factor (GCF) of a trinomial, you would first factor each term of the trinomial completely. Then, you identify the common factors among the terms and determine the greatest common factor by finding the highest power of each common factor that appears in all terms. This process allows you to identify the largest factor that can be divided evenly into each term of the trinomial.
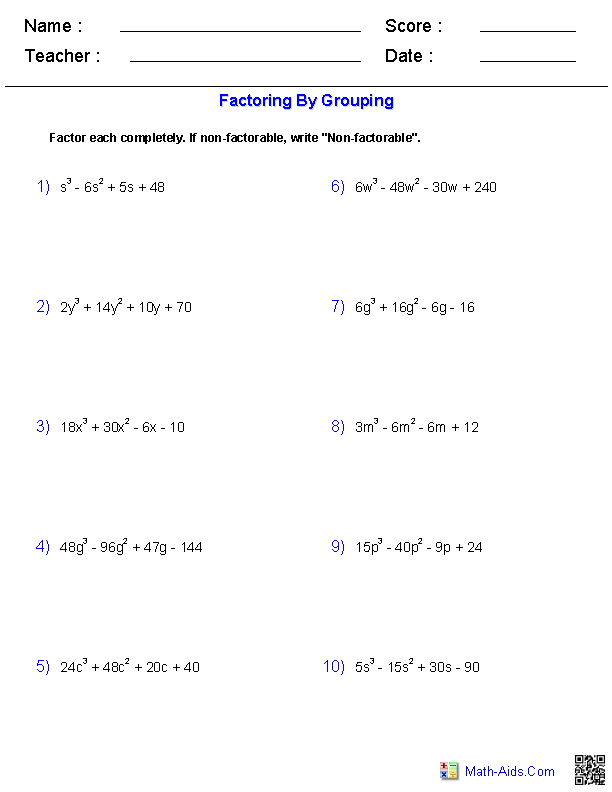
What is the difference between a common monomial factor and a common binomial factor?
A common monomial factor refers to a factor that is a monomial (an expression with only one term), shared by multiple terms in an algebraic expression, while a common binomial factor refers to a factor that is a binomial (an expression with two terms) shared by multiple terms in an algebraic expression. In simpler terms, a common monomial factor involves a single term that is common among different terms, whereas a common binomial factor involves a pair of terms that are common among different terms.
How do you factor a trinomial with a GCF using the distributive property?
To factor a trinomial with a greatest common factor (GCF) using the distributive property, you start by factoring out the GCF from each term of the trinomial. Then, you are left with a common binomial factor inside the parentheses. Finally, you use the distributive property to factor out this common binomial factor to complete the factoring of the trinomial with the GCF.
How do you factor a trinomial with a leading coefficient of 1?
To factor a trinomial with a leading coefficient of 1, you need to find two numbers that multiply to the constant term of the trinomial and add up to the coefficient of the middle term. Then, you use these two numbers to rewrite the middle term, leading to a factorable form. Finally, you can factor the trinomial by writing it as the product of two binomials with the first terms being the factors of the quadratic term and the second terms being the factors of the constant term.
How do you factor a trinomial with a leading coefficient greater than 1?
To factor a trinomial with a leading coefficient greater than 1, you first need to check if it is possible to factor out a common factor. If not, you can use the AC method or trial and error to find two binomials that multiply to the trinomial. This involves finding two numbers that multiply to the product of the leading coefficient and the constant term, and also add up to the middle coefficient. Once you have determined these numbers, you can write the trinomial as the product of the two binomials.
What is the purpose of factoring trinomials?
The purpose of factoring trinomials is to simplify and break down the expression into its factors, which can help in solving equations, graphing functions, and finding solutions to problems involving quadratic equations. Factoring trinomials allows us to identify common factors and patterns within the expression, making it easier to work with and understand the relationship between the different components of the equation.
Can all trinomials be factored?
No, not all trinomials can be factored. Some trinomials may not have easily identifiable factors or may only be factorable using more complex methods like the quadratic formula or completing the square.
What are some common mistakes to watch out for when factoring trinomials?
Some common mistakes to watch out for when factoring trinomials include not properly verifying the signs when splitting the middle term, forgetting to check for a greatest common factor before factoring, and incorrectly applying the distributive property when factoring. Additionally, overlooking the possibility of a difference of squares pattern or misidentifying the correct factors can also lead to errors in factoring trinomials. It is crucial to remain attentive and methodical while factoring trinomials to avoid these common mistakes.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.









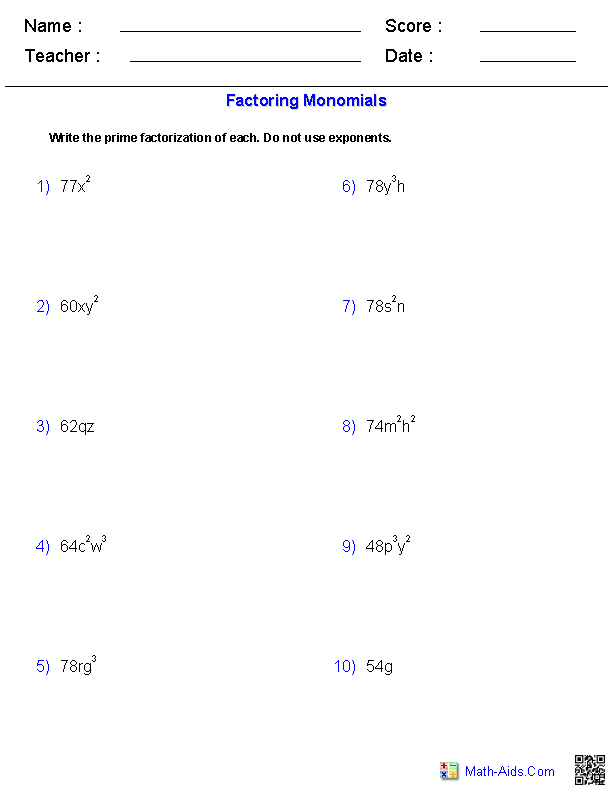


















Comments