Factoring Integers Worksheets
Factoring integers is an essential concept for students studying mathematics in middle school and high school. These worksheets are designed to help students practice and strengthen their skills in factoring numbers into their prime factors. By providing a range of exercises covering various difficulty levels, students can enhance their understanding of this important mathematical concept and improve their problem-solving abilities.
Table of Images 👆
- 8th Grade Math Worksheets Algebra
- 7th Grade Math Worksheets
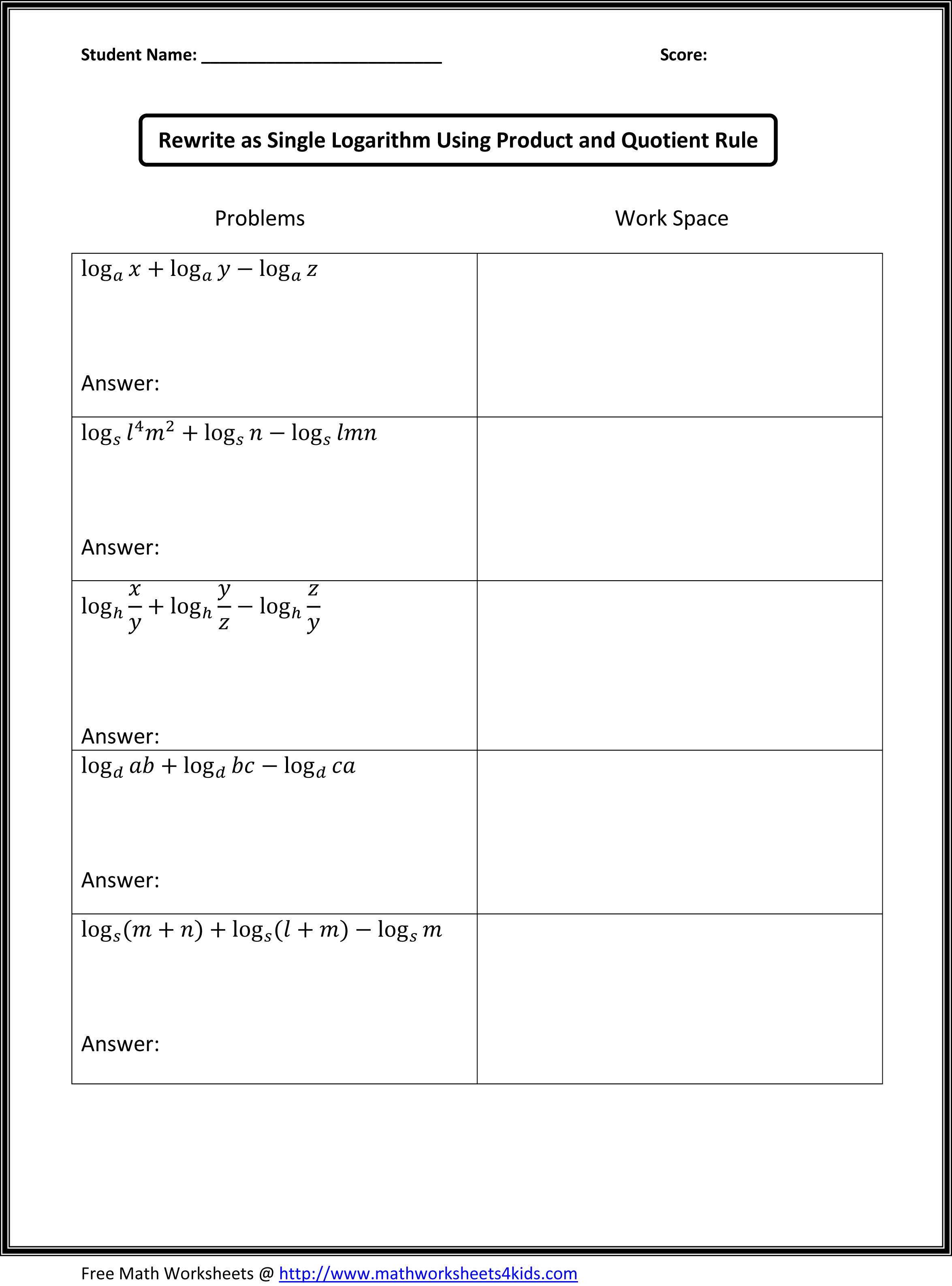
- Factoring GCF Worksheet Kuta
- 7th Grade Math Problems Worksheets
- Solving Linear Equations Worksheets
- 6th Grade Probability Worksheets
- Subtracting Fractions with Whole Numbers Worksheets
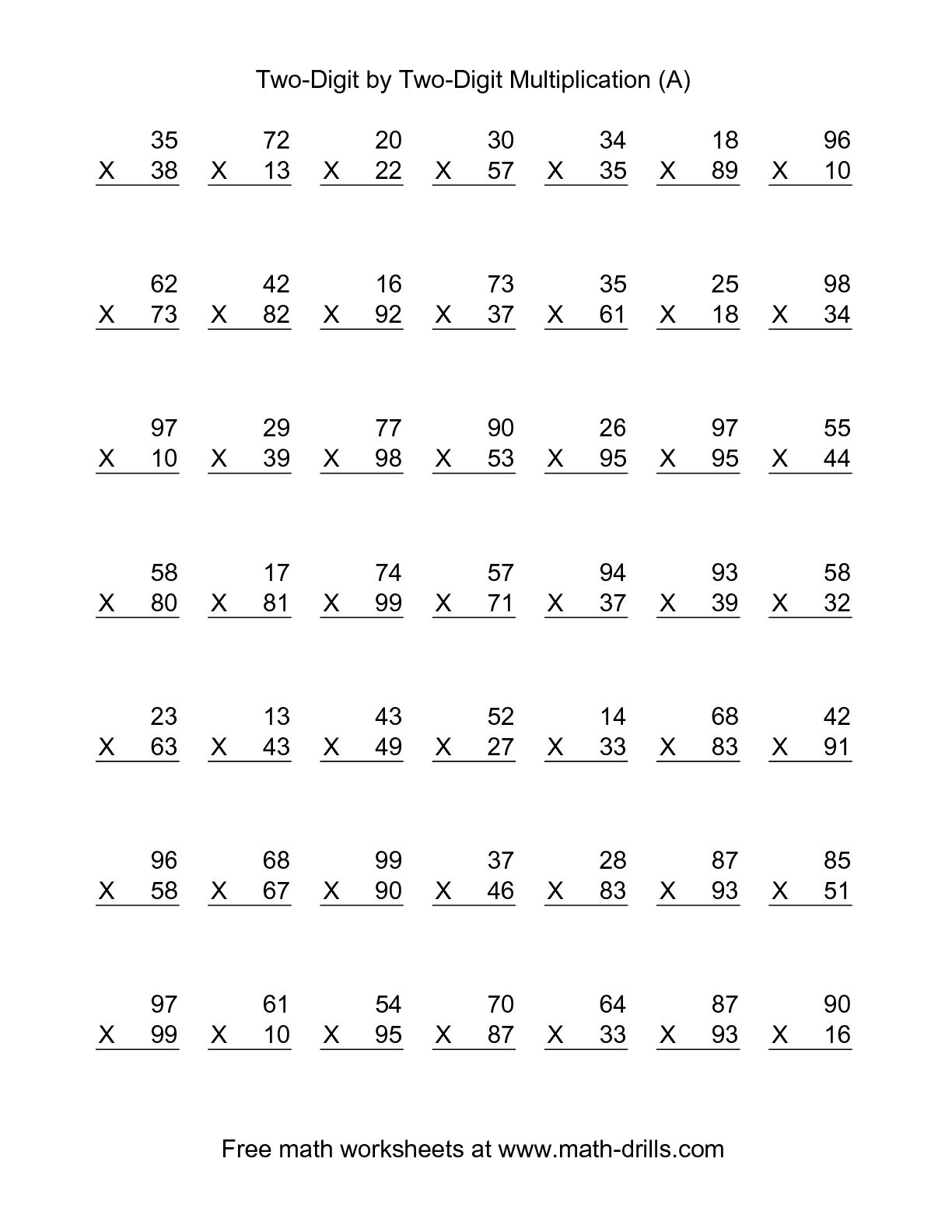
- 2-Digit Multiplication Worksheets
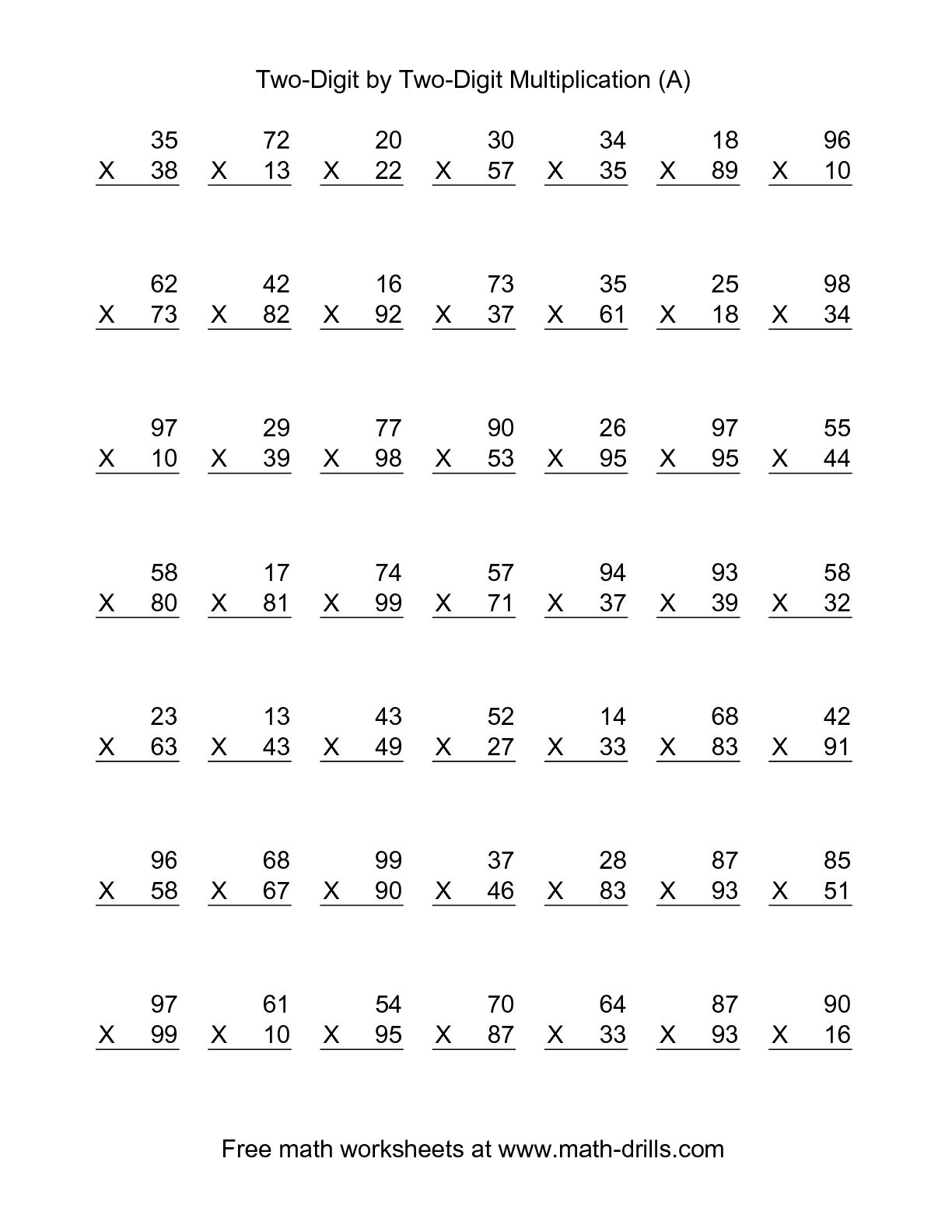
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
- 2-Digit Multiplication Worksheets
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is factoring?
Factoring is the process of breaking down a number or algebraic expression into its smaller, simpler components. In mathematics, factoring involves finding the prime factors of a number or identifying the common factors of an algebraic expression to simplify or solve problems. This technique is commonly used in algebra to simplify equations, solve for variables, and find solutions to polynomial expressions.
What is an integer?
An integer is a whole number that can be positive, negative, or zero, and does not have any decimal or fractional parts. It includes numbers such as -3, 0, 5, and 100.
How can you determine the factors of an integer?
To determine the factors of an integer, you would need to identify all the whole numbers that can evenly divide the integer without leaving a remainder. Start by finding the smallest factor, which is always 1, then continue finding pairs of factors until reaching the integer itself. A factor is always a divisor of the integer and is typically listed in ascending order. Factors play a significant role in simplifying fractions, determining the GCF (Greatest Common Factor), and solving mathematical problems involving multiples and divisors.
What is the difference between a prime factor and a composite factor?
A prime factor is a factor of a number that is a prime number, meaning it can only be divided by 1 and itself, such as 2, 3, 5, etc. On the other hand, a composite factor is a factor of a number that is not a prime number, as it can be divided by multiple numbers other than 1 and itself, such as 4, 6, 9, etc.
How can you use prime factorization to factor an integer?
To factor an integer using prime factorization, you first find the prime factors of the number by dividing it by the smallest prime numbers (2, 3, 5, 7, 11, etc.) until the quotient is a prime number. Write out the prime factors as a product. For example, for the number 72, the prime factorization would be 2 * 2 * 2 * 3 * 3, which simplifies to 2^3 * 3^2. This method helps break down the integer into its prime components, making it easier to find its factors or simplify expressions involving the number.
Explain the concept of greatest common factor (GCF) in factoring integers.
The greatest common factor (GCF) is the largest integer that divides evenly into two or more numbers. When factoring integers, the GCF is the largest factor that is common to all the numbers being factored. It is used to simplify fractions, factor polynomials, and find common factors in multiples. Calculating the GCF can help in finding the simplest form of a number or expression by dividing out common factors.
What is the relationship between prime factors and GCF?
The greatest common factor (GCF) of two numbers is made up of the prime factors that those numbers have in common. To find the GCF, you identify the prime factors of each number and then take the product of the common prime factors, raising each to the power of the lowest exponent shared between the numbers. This means that prime factors play a crucial role in determining the GCF of two numbers.
How can you apply factoring to simplify fractions?
To simplify fractions using factoring, you can factor both the numerator and the denominator of the fraction. Then, identify common factors that can be cancelled out from the numerator and denominator. This process helps reduce the fraction to its simplest form. By factoring and cancelling common factors, you can simplify fractions efficiently and accurately.
Describe the process of factoring out a common factor (also known as factoring by grouping).
Factoring out a common factor, also known as factoring by grouping, involves identifying and pulling out a common factor that is shared by all terms in an expression. First, look for the greatest common factor among all terms. Then, divide each term in the expression by this common factor, which results in a simplified expression with the common factor factored out. Next, group terms that share common factors together, and factor out these common factors from each group. Finally, simplify the expression by factoring out the common factor from the grouped terms, which can help to simplify and solve the original expression.
What are the real-world applications of factoring integers?
Factoring integers has several real-world applications, including cryptography, computer security, and number theory research. In cryptography, factoring large integers is used in algorithms to secure communication and data. It is also used in computer security to create secure encryption systems. Additionally, factoring integers is important in number theory research to understand the properties of numbers and their relationships.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.









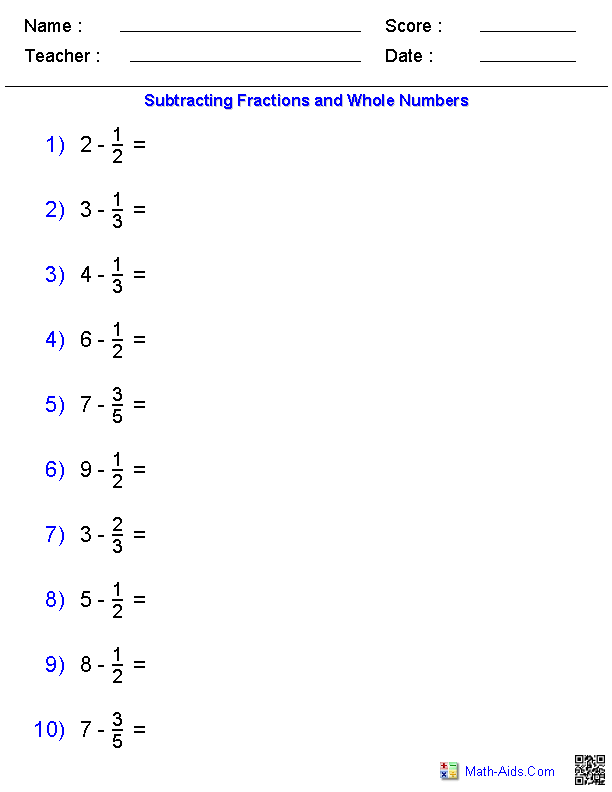




















Comments