Distributive Property Worksheets for Elementary

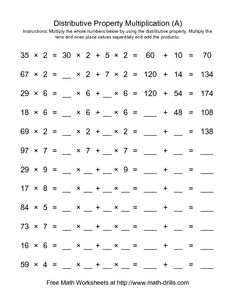
The distributive property is a fundamental concept in mathematics that is introduced to elementary students. It allows them to break down and simplify complex multiplication problems. If you are searching for worksheets to reinforce and enhance your child's understanding of the distributive property, we have just what you need. Our selection of distributive property worksheets provides engaging and interactive exercises suitable for elementary students.

  Distributive Property Multiplication Worksheets

---

  Distributive Property Worksheets

---

  Distributive Property 4th Grade

---

  Distributive Properties Examples

---

  Distributive Property Worksheets

---

  Distributive Property Multiplication Worksheets

---

  Simplifying Expressions Worksheets 7th Grade

---

  Math Properties Worksheets 6th Grade

---

  Distributive Property Worksheets 3rd Grade

---

  Distributive Property Worksheets

---

What is the distributive property?

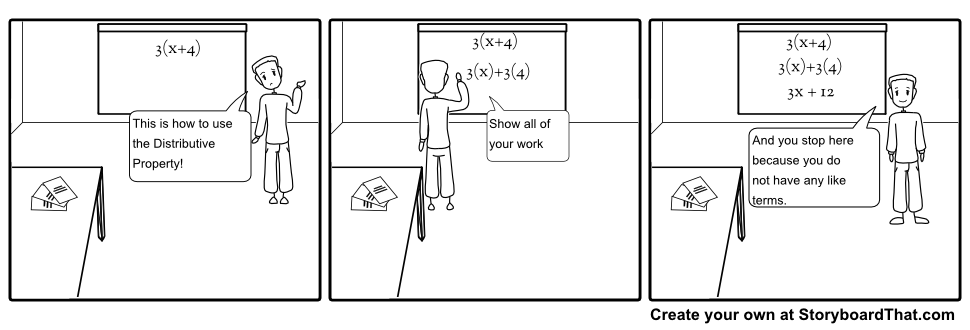
The distributive property states that for any numbers a, b, and c, the product of a and the sum of b and c is equal to the sum of the products of a and b and a and c. In other words, a(b+c) = ab + ac. This property is fundamental in algebra and is used to simplify and evaluate expressions involving multiplication and addition.

How is the distributive property used in multiplication?

In multiplication, the distributive property states that you can distribute the multiplication operation over addition. This means that for any three numbers a, b, and c, a(b + c) is equal to ab + ac. By applying the distributive property, you can simplify multiplication calculations by breaking down complex expressions into simpler terms. This property is fundamental in algebraic manipulations and helps in solving various math problems efficiently.

How is the distributive property used in addition?

The distributive property states that when you multiply a number by a sum, you can simply distribute the multiplication across the terms inside the parentheses. In the context of addition, this means that when you have a number outside of the parentheses that needs to be added to the result inside the parentheses, you can apply the distributive property to perform the addition. For example, in the expression 3(4 + 2), you can first distribute the 3 to both terms inside the parentheses to get 3*4 + 3*2, and then add these results together to get 12 + 6 = 18.

How can the distributive property be applied to simplify expressions?

The distributive property can be applied to simplify expressions by distributing a factor outside the parentheses to each term inside the parentheses. This involves multiplying the factor with each term individually before combining like terms. By applying the distributive property, you can consolidate terms and make the expression more manageable and easier to solve.

Can the distributive property be used with division?

Yes, the distributive property can be applied to division. When dividing a number by a sum or difference of numbers, the distributive property can be used to distribute the division operation to each term separately. For example, if you have a ÷ (b + c), you can distribute the division to both b and c individually as a ÷ b + a ÷ c.

Can the distributive property be used with subtraction?

Yes, the distributive property can be used with subtraction. It states that a(b - c) = ab - ac, meaning that you can distribute a factor to both terms inside the parentheses, regardless of whether the operation is addition or subtraction.

How can the distributive property be applied to solve equations?

The distributive property can be applied to solve equations by distributing a number or term outside of parentheses to every term inside the parentheses. This helps in simplifying the equation and solving for the variable. By distributing the number or term, you can then combine like terms and isolate the variable to find its value.

How can the distributive property be used to simplify fractions?

The distributive property can be used to simplify fractions by breaking down the numerator and denominator into their factors and then cancelling out common factors. By distributing the factors in the numerator and denominator separately, you can identify and eliminate common factors to reduce the fraction to its simplest form. This process helps in simplifying fractions efficiently and accurately using the distributive property.

How does the distributive property relate to factoring?

The distributive property is the reverse process of factoring. The distributive property states that for any numbers a, b, and c, a(b + c) = ab + ac. Factoring involves breaking down an expression into simpler terms by striking out common factors or distributing a common factor from each term. So, when factoring, you are essentially applying the distributive property in reverse to simplify an expression by finding a common factor among all terms.

Can the distributive property be used with variables and constants?

Yes, the distributive property can be used with variables and constants. This property states that for any numbers a, b, and c, a(b + c) = ab + ac. When variables are involved, the same rule applies, for example, x(y + z) = xy + xz. The distributive property can be used regardless of whether the terms being multiplied are variables, constants, or a combination of both.