Composite Area Worksheet

Are you a math teacher searching for a resource that will engage your students and strengthen their understanding of composite area? Look no further than our comprehensive composite area worksheet. This worksheet is designed specifically for middle school students who are studying geometry and want to practice their skills in finding the area of irregular shapes composed of multiple polygons.

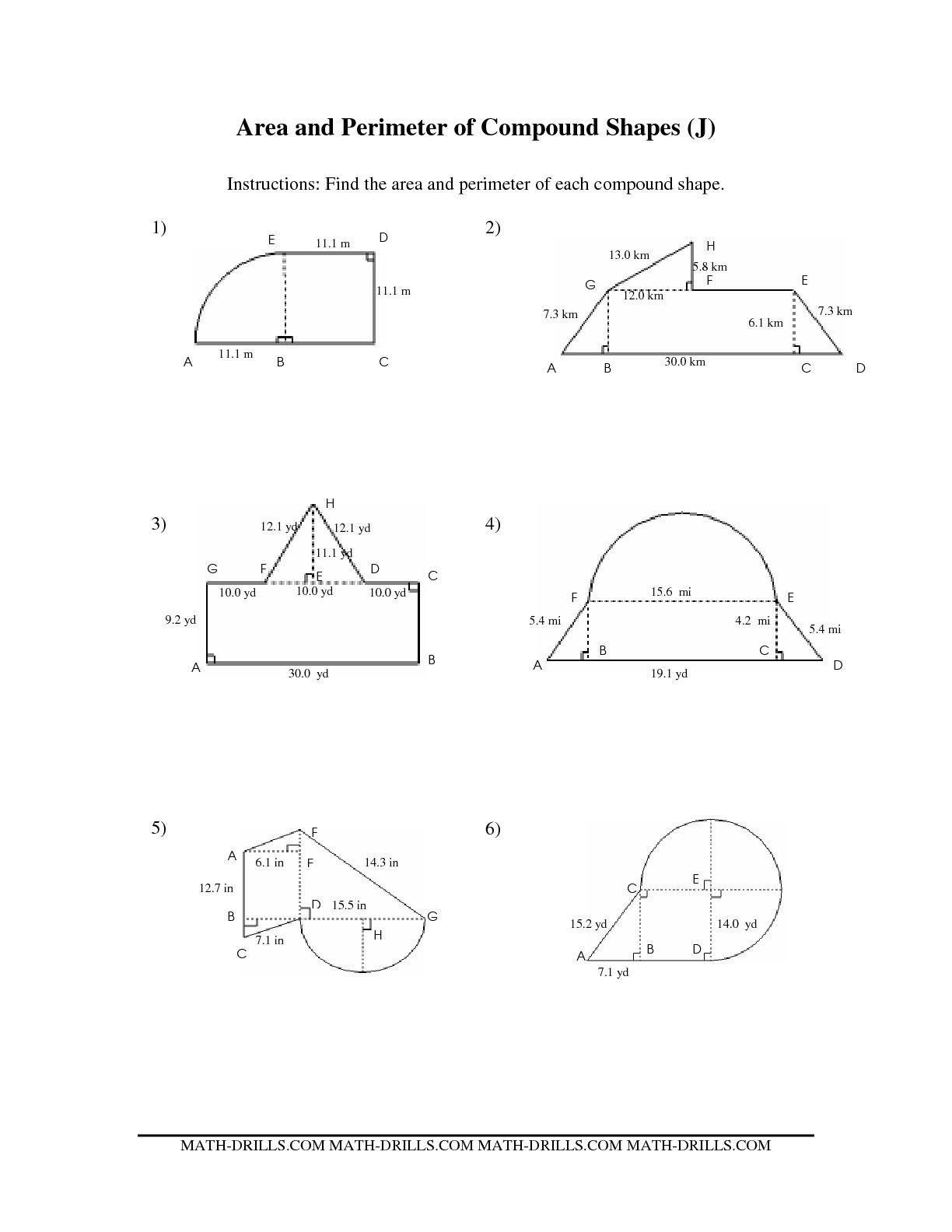
  Compound Shapes Area and Perimeter

---

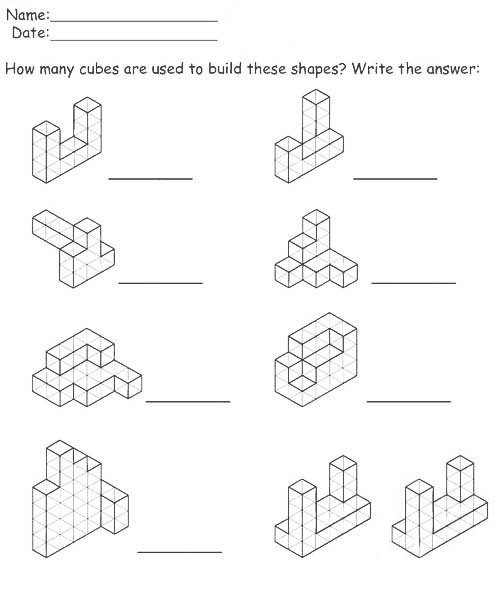
  Composite Figures Volume Worksheet and Answers

---

  Surface Area Worksheets 8th Grade

---

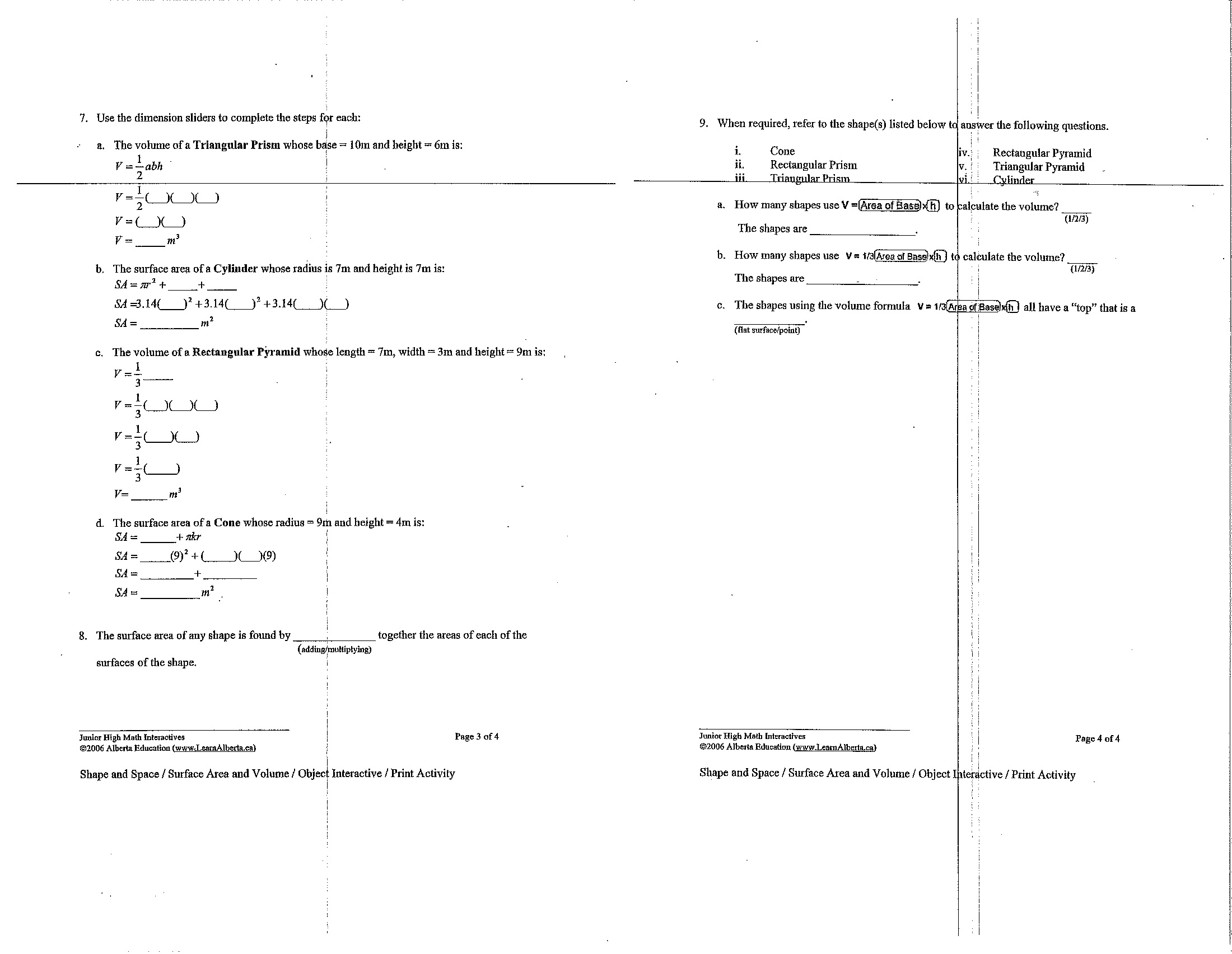
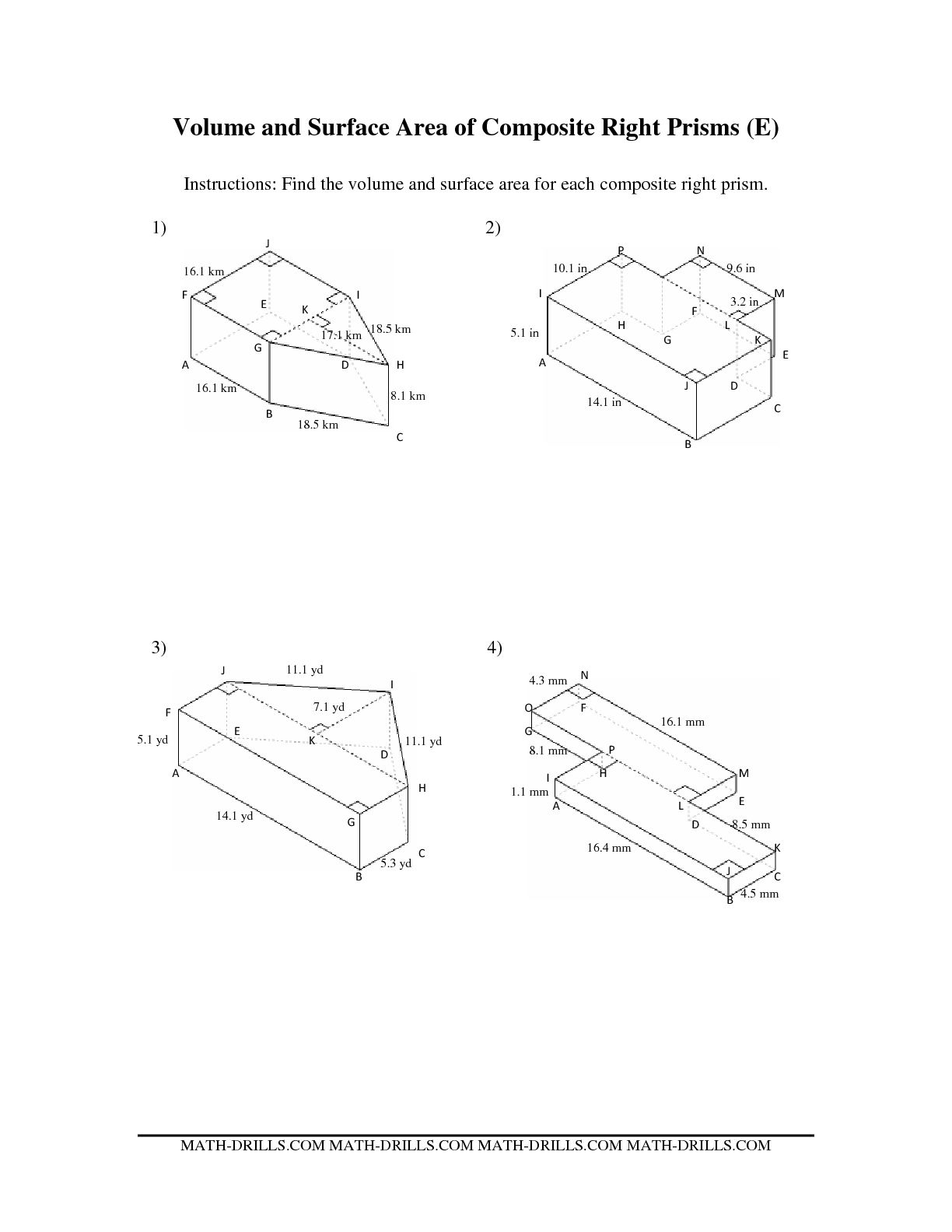
  Surface Area of Composite Prisms Worksheet

---

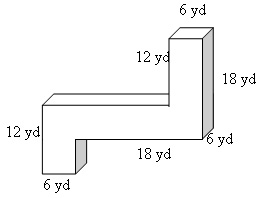
  Composite Figure Volume Rectangular Prisms

---

  5th Grade Reading Comprehension Worksheets

---

  Composite Wing Spar Design

---

  Army Composite Risk Management

---

  7th Grade Math PSSA Formula Sheet

---

What is composite area?

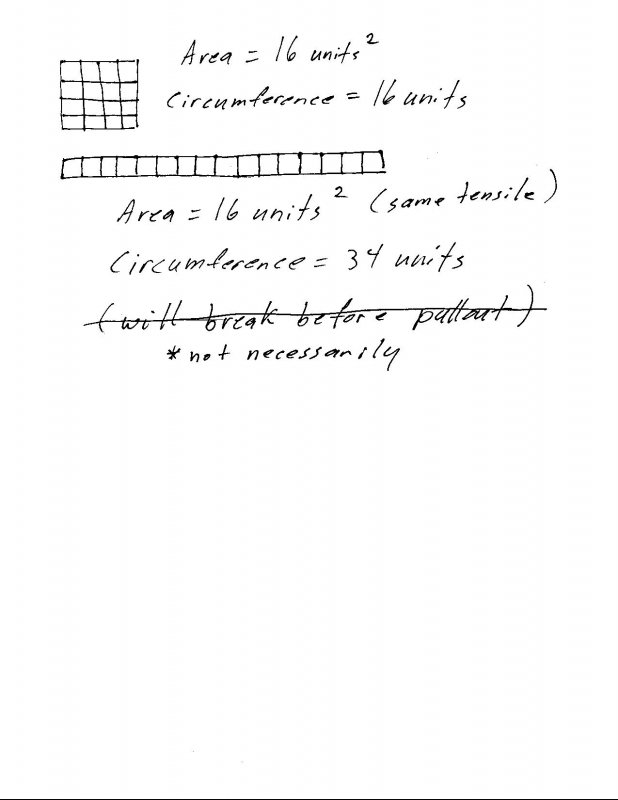
Composite area refers to the total surface area of a complex shape that is made up of multiple simpler shapes or components. To find the composite area, the individual areas of each component must be calculated and then added together to get the total surface area of the composite shape.

How is composite area calculated?

The composite area of a shape is calculated by dividing the shape into simpler, known shapes (such as rectangles, triangles, circles, etc.), calculating the area of each individual shape, and then summing these areas together. For example, the composite area of a shape made of a rectangle and a triangle would be calculated by finding the area of the rectangle and the area of the triangle separately, and then adding them together to get the total composite area of the shape.

What are some common shapes used in composite area calculations?

Some common shapes used in composite area calculations include rectangles, triangles, trapezoids, circles, and semi-circles. These shapes can be combined and manipulated together to form more complex composite figures, allowing for the calculation of their overall area based on the known formulas for each individual shape.

How do you find the area of a rectangle in a composite figure?

To find the area of a rectangle in a composite figure, you would first identify the dimensions (length and width) of the rectangle. Then, you would multiply the length by the width to calculate the area of the rectangle. Finally, you can sum up the areas of all the individual shapes in the composite figure to obtain the total area.

How do you find the area of a triangle in a composite figure?

To find the area of a triangle in a composite figure, you would first divide the composite figure into separate shapes, such as triangles, rectangles, or trapezoids. Next, calculate the area of each individual shape using their respective formulas (area of a triangle = 1/2 * base * height, area of a rectangle = length * width, area of a trapezoid = 1/2 * (sum of parallel sides) * height). Finally, add the areas of all the shapes together to find the total area of the composite figure.

How do you find the area of a circle in a composite figure?

To find the area of a circle within a composite figure, you need to calculate the area of the circle itself using the formula A = ?r^2, where r is the radius of the circle. Then, you can subtract the area of any other shapes within the composite figure from the total area of the circle to find the final area of the circle within the composite figure.

How do you find the area of a trapezoid in a composite figure?

To find the area of a trapezoid in a composite figure, you need to separate the trapezoid from the rest of the shape. Then, calculate the area of the trapezoid using the formula A = 0.5 * (b1 + b2) * h, where b1 and b2 are the lengths of the parallel bases of the trapezoid, and h is the height of the trapezoid. Finally, add the area of the trapezoid to the areas of the other shapes in the composite figure to get the total area.

What is the formula for finding the perimeter of a composite figure?

To find the perimeter of a composite figure, you would add up the lengths of all the sides of the individual shapes that make up the composite figure. This means identifying each side, measuring its length, and then adding all the lengths together to calculate the total perimeter of the composite figure.

How do you find the area of irregular shapes in a composite figure?

To find the area of irregular shapes in a composite figure, break down the figure into smaller, regular shapes like triangles, squares, rectangles, and circles. Find the individual area of each regular shape using its specific formula (e.g. area of a triangle = 0.5 * base * height). Then, sum up the areas of all the individual shapes to get the total area of the irregular shape in the composite figure.

Why is understanding composite area important in real-life situations?

Understanding composite area is important in real-life situations because many objects and structures have complex shapes that require a combination of different basic shapes to calculate their total area. For example, in construction, architects and engineers need to calculate the total area of irregularly shaped buildings to determine the amount of materials needed. Similarly, in manufacturing, designers need to know the composite area of components to optimize production processes. Overall, having a grasp of composite area allows individuals to make informed decisions and solve practical problems efficiently in various fields.