Commutative Multiplication Worksheets

Commutative multiplication worksheets provide a valuable learning tool for students who are studying the concept of commutative property in mathematics. These worksheets focus on the entity of multiplication and explore the subject of commutativity, teaching students that the order of factors does not affect the product. By practicing with commutative multiplication worksheets, students can solidify their understanding of this important mathematical principle.

  Commutative Property Worksheets 1st Grade

---

  Flip Flop Commutative Property of Addition

---

  3 Grade Math Worksheets

---

  Distributive Property Multiplication Worksheets

---

  Associative Property of Multiplication Worksheets

---

  3rd Grade Math Worksheets Multiplication Table

---

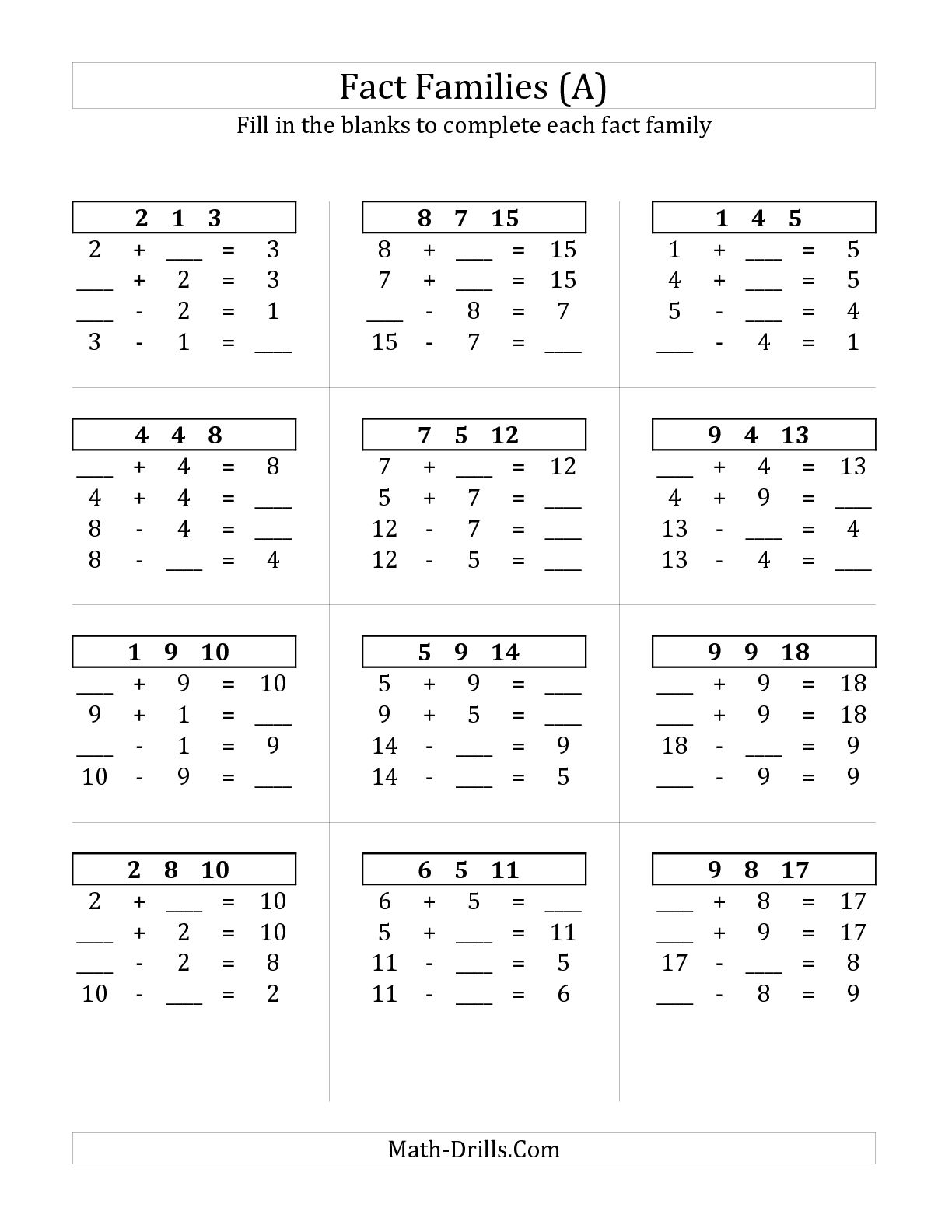
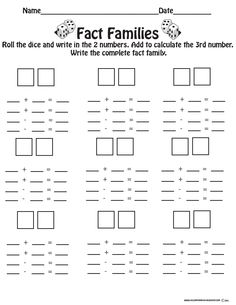
  Math Fact Families Worksheets First Grade

---

  Math Properties Worksheets 7th Grade

---

  Addition and Subtraction Fact Family Worksheets

---

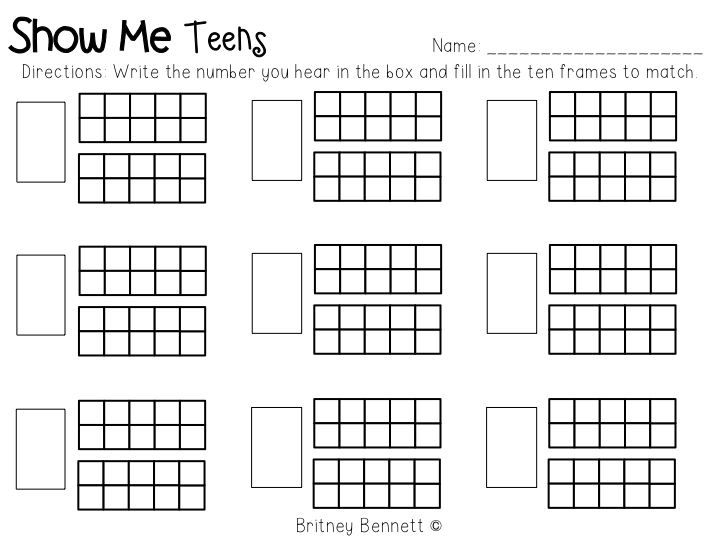
  Kindergarten Ten Frame Teen Number Worksheets

---

  Multiplication and Division Fact Families

---

  Associative Property Addition Worksheets

---

  Domino Addition Worksheets for First Grade

---

  Multiplication Cross Number Puzzle

---

  5 Themes of Geography Worksheets

---

  5 Themes of Geography Worksheets

---

What is commutative multiplication?

Commutative multiplication is a property of multiplication in mathematics that states that changing the order of the numbers being multiplied does not change the result. In other words, if you multiply two numbers a and b, then the result will be the same if you multiply b and a. This property holds true for all real numbers and is a fundamental property of multiplication.

How is commutative multiplication different from regular multiplication?

Commutative multiplication and regular multiplication refer to the same arithmetic operation which is multiplication. The term "commutative" in commutative multiplication signifies that the order of the numbers being multiplied does not affect the result, meaning that a x b will give the same answer as b x a. So, commutative multiplication is not different from regular multiplication, it simply refers to this specific property where the order of multiplicands can be interchanged without affecting the answer.

Can you give an example of a commutative multiplication problem?

Sure! An example of a commutative multiplication problem is 4 × 7 = 7 × 4. This demonstrates the commutative property of multiplication, which states that the order of the numbers being multiplied does not affect the product.

Why is commutative multiplication a useful concept in mathematics?

Commutative multiplication is a useful concept in mathematics because it allows for the order of multiplication to be changed without altering the result. This property simplifies calculations and makes it easier to work with mathematical expressions, providing a more intuitive and straightforward approach to solving problems in various mathematical disciplines. It also plays a crucial role in algebraic manipulations, helping to streamline equations and proofs. This property of commutative multiplication is fundamental in arithmetic and algebra, making it an essential concept in mathematical operations.

What are the properties of commutative multiplication?

Commutative multiplication is a property that states that changing the order of the numbers being multiplied does not affect the result. In other words, for any two numbers a and b, a x b is equal to b x a. This property holds true for all real numbers, making multiplication a commutative operation.

How does commutative multiplication relate to other mathematical operations?

Commutative multiplication is a property of multiplication where the order of the numbers does not change the result (e.g., 2 x 3 = 3 x 2). This property is closely related to the commutative property of addition, but also has connections to other mathematical operations such as exponentiation, scalar multiplication, and matrix multiplication. In general, commutativity simplifies operations and allows for more flexible manipulation of numbers and variables in mathematical equations and expressions.

Are there any real-world applications of commutative multiplication?

Yes, commutative multiplication is widely used in various real-world applications such as computing areas of rectangles and squares, calculating total costs and prices in business transactions, determining total quantities in manufacturing processes, and analyzing data in scientific research and statistics. The property of commutative multiplication allows for efficient and flexible mathematical operations that are essential in many practical scenarios.

Can commutative multiplication be applied to non-numeric situations?

Yes, commutative multiplication can be applied to non-numeric situations, such as in mathematics with variables or in real-world scenarios like combining various quantities or characteristics. For example, if A * B represents the product of two quantities, the commutative property of multiplication states that A * B is equal to B * A, regardless of the specific nature of A and B. This property holds true even without numerical values, emphasizing the order in which quantities are multiplied does not impact the final result.

How can commutative multiplication be utilized to simplify complex calculations?

Commutative multiplication states that the order of numbers being multiplied does not affect the result. By utilizing commutative multiplication, we can rearrange the order of multiplication to simplify complex calculations. This allows us to multiply numbers in an order that makes the calculation easier and more efficient, which can help streamline the process and reduce the chances of making mistakes in complex mathematical problems.

What age group or grade level is typically introduced to commutative multiplication?

Commutative multiplication is usually introduced to students in elementary school, around the ages of 7-9 years old, typically in 2nd or 3rd grade. This concept helps students understand that the order of numbers being multiplied does not affect the result, and lays the foundation for more complex mathematical operations.