Arithmetic Series Worksheet
Are you struggling to find a comprehensive arithmetic series worksheet for your students? Look no further! We have designed a worksheet that focuses specifically on the concept of arithmetic series, making it perfect for math teachers and students who are looking to practice and reinforce their understanding of this fundamental topic.
Table of Images 👆
- Arithmetic Geometric Sequences Worksheet
- Geometric Sequences Worksheets
- Arithmetic and Geometric Sequences
- Oxidation Numbers Worksheet Answers
- Algebra with Pizzazz Answer Key
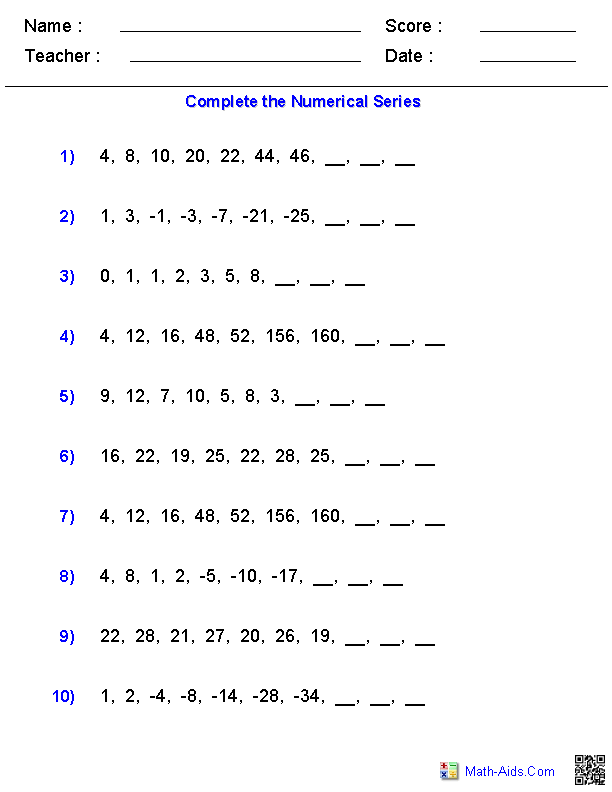
- Number Patterns Worksheets 3rd Grade Math
- Infinite Geometric Series Sum Formula
- Statements and Questions Worksheets 2nd Grade
- 4th Grade Math Problems Worksheets
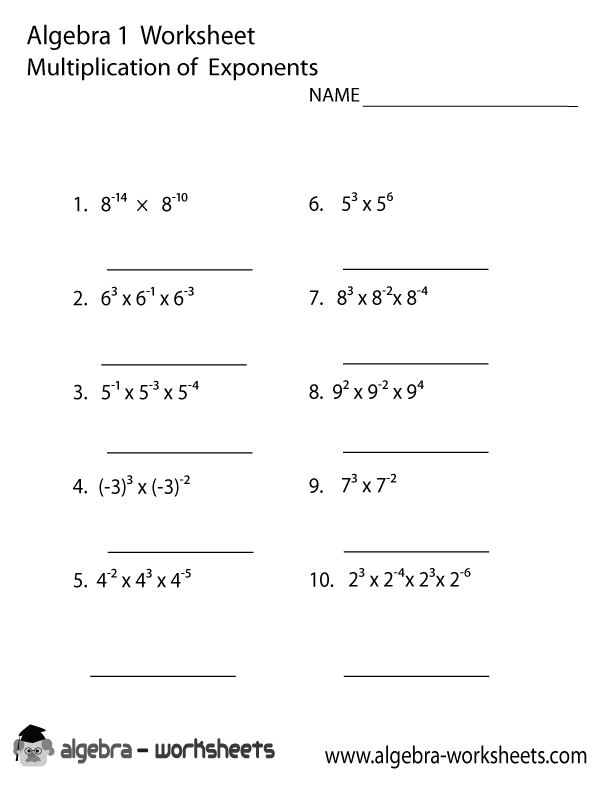
- Exponents Algebra 1 Worksheets
- Pythagorean Identities Worksheet
- Pythagorean Identities Worksheet
- Pythagorean Identities Worksheet
- Pythagorean Identities Worksheet
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is an arithmetic series?
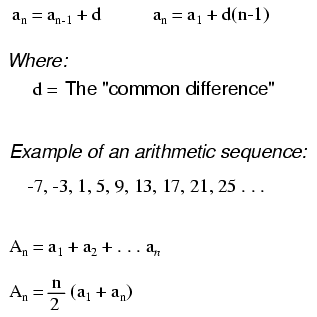
An arithmetic series is a sequence of numbers in which each term is obtained by adding a constant difference to the previous term. In other words, the series follows a pattern where each term is calculated by adding (or subtracting) the same value from the previous term. This constant difference is called the common difference, and the sum of all the terms in an arithmetic series can be calculated using a specific formula.
How is the common difference calculated in an arithmetic series?
The common difference in an arithmetic series is calculated by subtracting any term in the series from the term that follows it. This common difference remains constant throughout the series, allowing for the easy calculation of any term in the sequence by adding or subtracting the common difference as needed.
How is the nth term of an arithmetic series found?
The nth term of an arithmetic series is found using the formula: \( a_n = a_1 + (n-1)d \), where \( a_n \) represents the nth term, \( a_1 \) is the first term of the series, \( n \) is the position of the term being found, and \( d \) is the common difference between consecutive terms.
What is the formula to find the sum of an arithmetic series?
The formula to find the sum of an arithmetic series is Sn = n/2 * (2a + (n-1)d), where Sn is the sum of the series, n is the number of terms in the series, a is the first term, and d is the common difference between the terms.
How are partial sums of an arithmetic series calculated?
The partial sum of an arithmetic series can be calculated using the formula Sn = n/2 * (a1 + an), where Sn is the sum of the first n terms, a1 is the first term, and an is the nth term. This formula calculates the sum of all terms from a1 to an in the arithmetic sequence.
Can the common difference in an arithmetic series be negative?
Yes, the common difference in an arithmetic series can be negative. In an arithmetic sequence, the common difference is the constant value that is added (or subtracted) to each term to get the next term in the sequence. This means that the common difference can be a positive or negative number, depending on the direction of the progression of the sequence.
Are all arithmetic sequences also arithmetic series?
Yes, all arithmetic sequences can also be considered as arithmetic series. An arithmetic sequence is a list of numbers with a common difference between consecutive terms, while an arithmetic series is the sum of all terms in an arithmetic sequence. Each term in the sequence contributes to the sum in the series, making them interconnected concepts within mathematics.
What is the relationship between the sum of an arithmetic series and its terms?
The sum of an arithmetic series is directly related to its terms, as it is the total result of adding all the terms in the series together. The sum is calculated using the formula: Sum = (n/2) * (2a + (n-1)d), where n is the number of terms, a is the first term, and d is the common difference between terms. In other words, the sum of an arithmetic series is dependent on the individual terms and their sequence in the series.
Is it possible for an arithmetic series to have an infinite number of terms?
No, an arithmetic series cannot have an infinite number of terms. An arithmetic series is a finite sequence of numbers where each term is obtained by adding a constant value to the previous term. The series will go on indefinitely if no limits are set to the number of terms, but it cannot have an infinite number of terms within a traditional arithmetic series framework.
What are some real-world applications of arithmetic series?
Real-world applications of arithmetic series include calculating compound interest on a loan or investment, determining the total cost of a series of regular payments like car loan installments or mortgage payments, and analyzing the rate of growth or depreciation of a quantity over time. These applications are commonly used in finance, economics, and various scientific fields to model and predict patterns of change or accumulation.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.








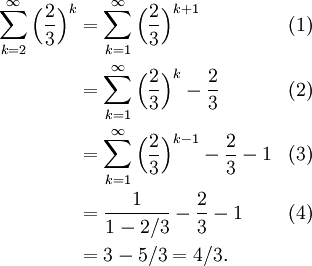


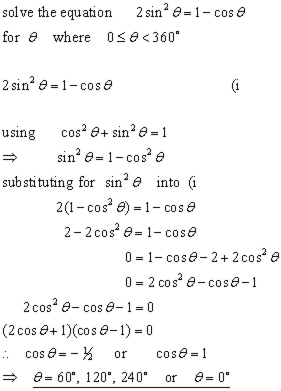
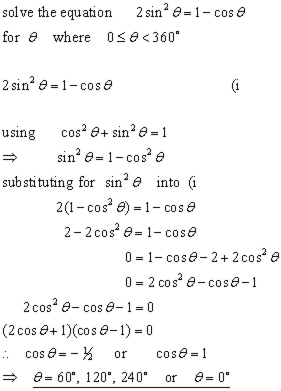
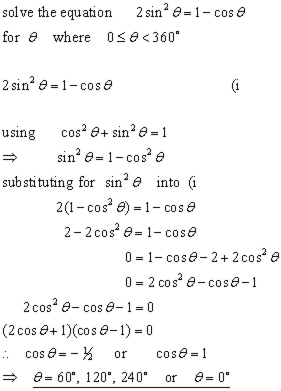
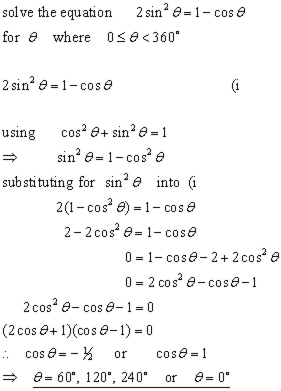














Comments