AP Calculus Worksheets

AP Calculus worksheets provide valuable practice and reinforcement for students studying advanced calculus concepts. These worksheets cover a wide range of topics, including limits, derivatives, integrals, and applications of calculus. They are designed to help students better understand and master the material by offering a variety of problem-solving exercises and real-world applications.

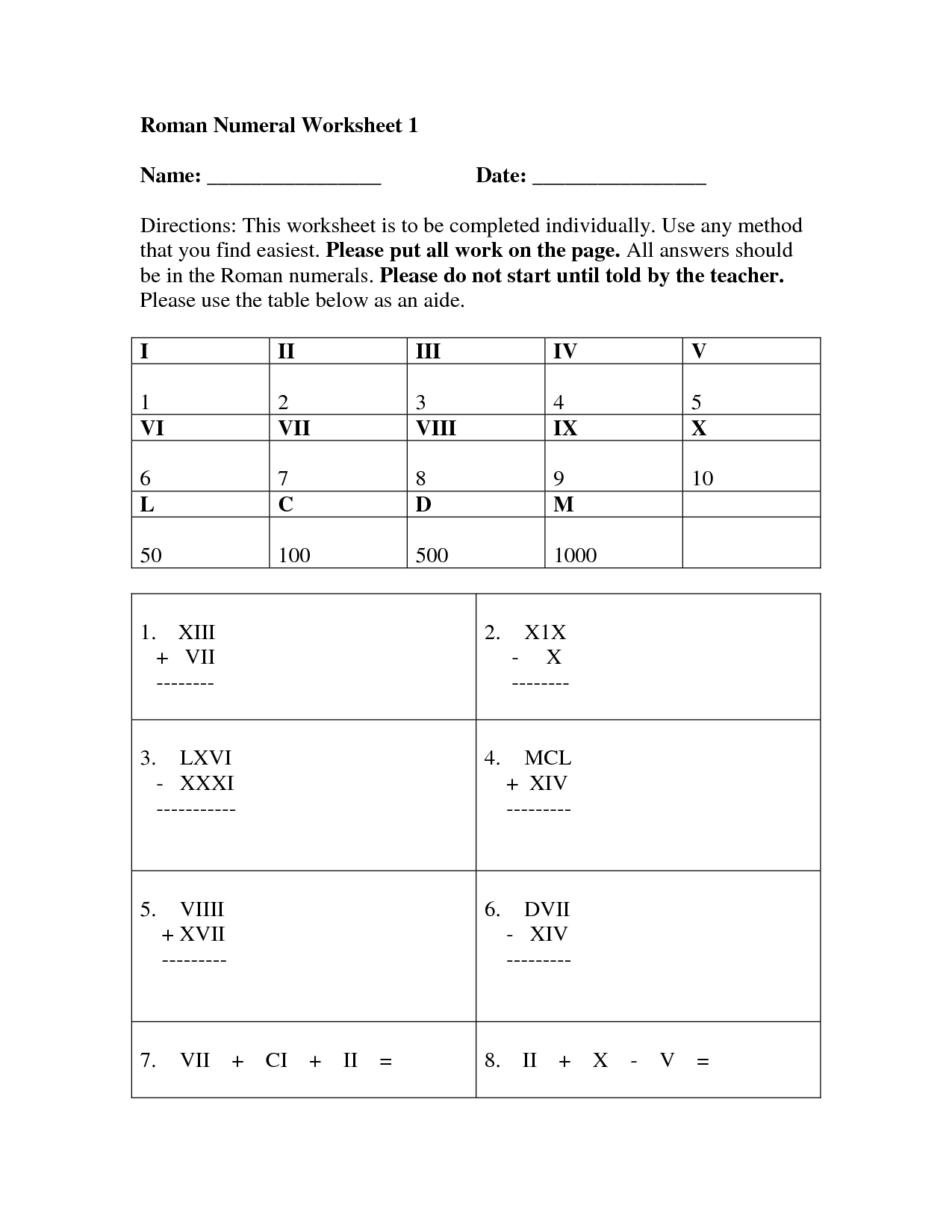
  Roman Numerals Printable Worksheets

---

  Function Notation Worksheet

---

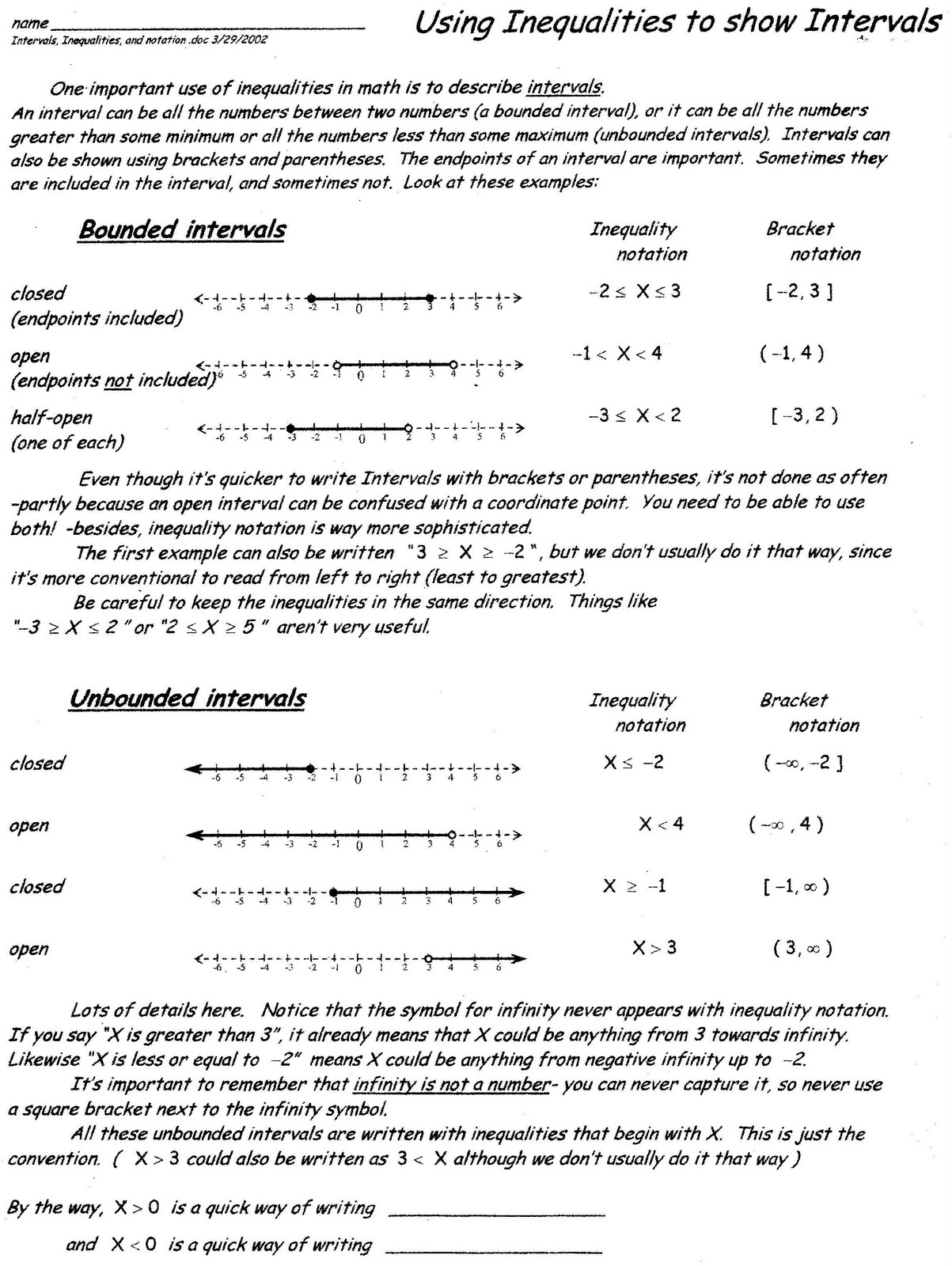
  Slope as Rate of Change Worksheet

---

  Funny Math Cartoons

---

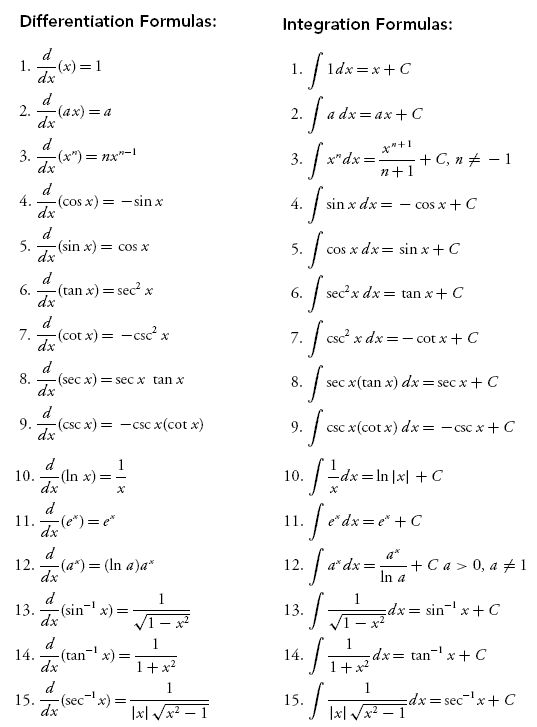
  Calculus Integration Formulas

---

  Derivative Inverse Trig Functions Worksheet

---

  Star Wars Yoda Quotes

---

  College Physics Formula Sheet

---

  Funny Math Jokes for Teachers

---

  Calculus 2 Final Exam Cheat Sheet

---

  Diamond Bar High School

---

Define the concept of limits in calculus.

In calculus, the concept of limits refers to the value that a function approaches as its input (such as a variable) approaches a certain value. The limit of a function at a particular point represents the behavior of the function around that point, providing insight into the function's values and behavior as it gets closer to that point. Limits are fundamental in calculus as they help analyze functions, define derivatives and integrals, and understand the nature of functions at specific points.

Explain the process of finding the derivative of a function.

To find the derivative of a function, you need to apply the rules of differentiation, such as the power rule, product rule, quotient rule, and chain rule. Start by identifying the function you want to differentiate, then apply these rules step by step to find the derivative with respect to the variable. Simplify the expression until you have the derivative of the function in its simplest form. Remember that the derivative represents the rate of change of the function at any given point, providing valuable information about its behavior.

Describe the fundamental theorem of calculus and its significance.

The fundamental theorem of calculus states that if a function is continuous on a closed interval [a, b] and F(x) is the antiderivative of f(x) on [a, b], then the definite integral of f(x) from a to b is equal to F(b) - F(a). This theorem essentially connects two branches of calculus, differential and integral calculus, by providing a method to compute definite integrals using antiderivatives. It is significant because it allows for the computation of areas under curves, volumes of solids, work done by a force, and many other applications in various fields such as physics, engineering, and economics.

Discuss the concept of indefinite integrals and how they relate to antiderivatives.

Indefinite integrals represent the family of antiderivatives of a function, indicating all possible functions that, when differentiated, yield the original function. The indefinite integral of a function involves finding a general expression that represents an antiderivative of the function, denoted as ?f(x)dx, where f(x) is the function to be integrated and dx signifies the variable of integration. The relationship between indefinite integrals and antiderivatives is crucial in calculus as it allows us to find functions whose derivative is a given function, enabling the fundamental theorem of calculus to evaluate areas under curves, determine changes in quantities, and solve various differential equations.

Detail the steps involved in solving related rate problems in calculus.

To solve related rates problems in calculus, one must first identify the rates that are changing in the problem. Next, establish the equation that relates the variables that are changing, typically using geometry or physics principles. Then, differentiate the equation with respect to time. Subsequently, plug in the given values and solve for the unknown rate using implicit differentiation. Finally, present the answer with appropriate units and ensure it aligns with the context of the problem. Familiarity with differentiation rules and understanding how variables are related are crucial in successfully solving related rates problems in calculus.

Explain the concept of concavity and how it is determined using the second derivative.

Concavity refers to the shape of a graph and is determined by the curvature of the graph. A function is said to be concave up if it curves upward like a smile, and concave down if it curves downward like a frown. The concavity of a function is determined by analyzing the sign of its second derivative. If the second derivative is positive, the function is concave up; if it is negative, the function is concave down. Points where the second derivative is zero are inflection points, where the concavity may change.

Discuss Riemann sums and their role in approximating definite integrals.

Riemann sums are a method used in calculus to approximate the value of a definite integral by dividing the area under a curve into smaller rectangles and summing their areas. By increasing the number of rectangles and making them narrower, the Riemann sum becomes a more accurate approximation of the definite integral. This method is essential in numerical integration as it allows us to compute the area under a curve when an exact solution is not available, providing a useful tool in various fields such as physics, engineering, and economics for estimating quantities that can be represented as definite integrals.

Describe the process of solving optimization problems using calculus techniques.

In solving optimization problems using calculus techniques, the first step is to define the objective function to be optimized, often representing the quantity to be minimized or maximized. Then, identify the constraints that restrict the possible solutions of the problem. Next, the critical points of the objective function are found by taking the derivative and setting it equal to zero. These critical points are then tested using the first or second derivative test to determine whether they correspond to a maximum, minimum, or neither. Finally, the optimal solution is determined by evaluating the objective function at the critical points and any boundary points to find the maximum or minimum value that satisfies the constraints.

Explain how to find the area between two curves using definite integrals.

To find the area between two curves using definite integrals, first, find the points of intersection of the two curves by setting them equal to each other and solving for the x-values. Next, determine which curve is above the other between the points of intersection. Then, subtract the lower curve from the upper curve to find the function representing the vertical distance between the two curves. Finally, integrate this vertical distance function with respect to x over the interval of the points of intersection using a definite integral to find the area between the two curves.

Discuss the concept of exponential growth or decay and its representation through calculus functions.

Exponential growth or decay is a fundamental concept in mathematics and can be represented through calculus functions. In the case of exponential growth, a function increases at a rate proportional to its current value, leading to rapid growth over time. Mathematically, exponential growth can be expressed as the function f(x) = a*(1+r)^x, where a is the initial value, r is the growth rate, and x is the time variable. Conversely, exponential decay involves a decrease in value at a rate proportional to its current value. The formula for exponential decay is f(x) = a*(1-r)^x, where a is the initial value, r is the decay rate, and x is the time variable. Through calculus functions, we can analyze and model the behavior of exponential growth or decay in various real-world applications.