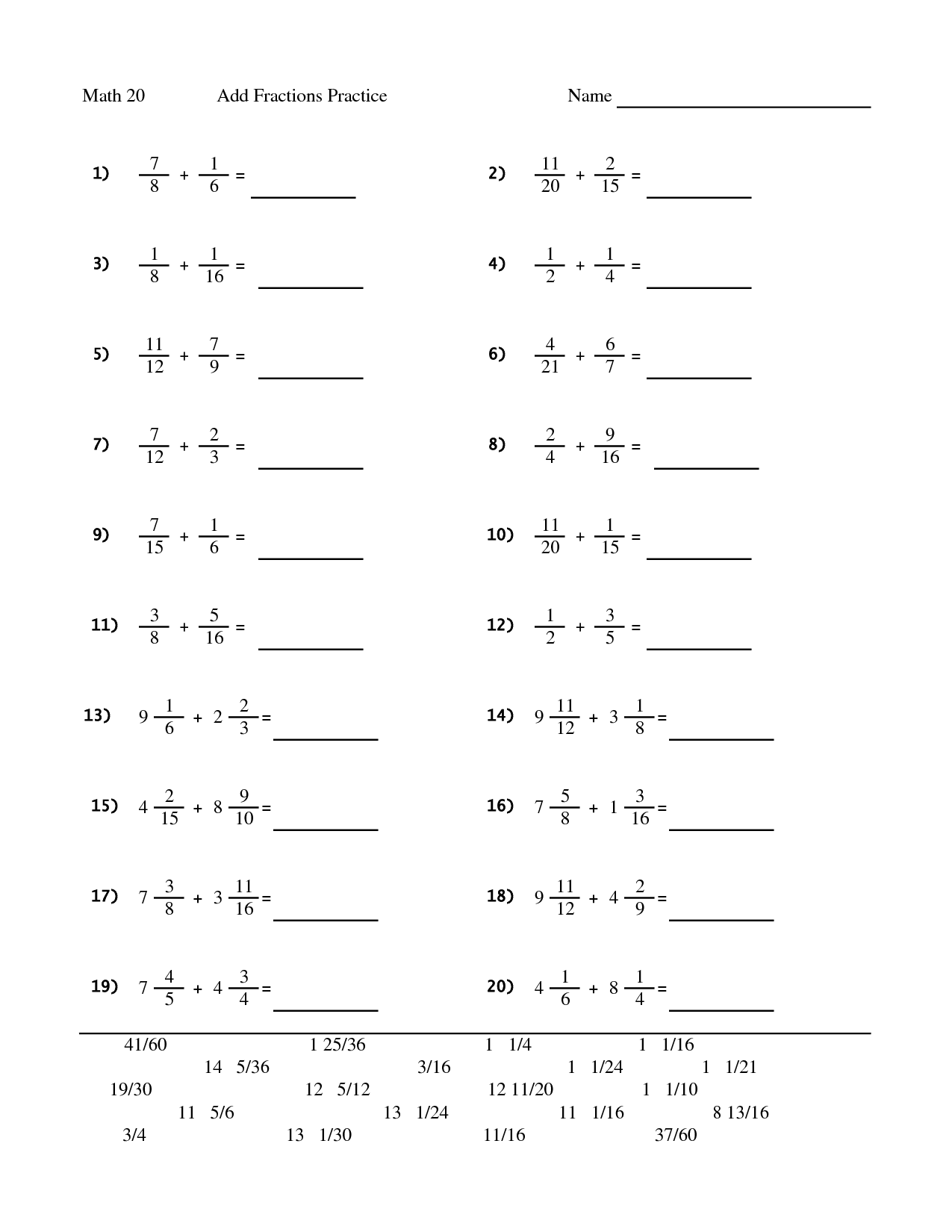
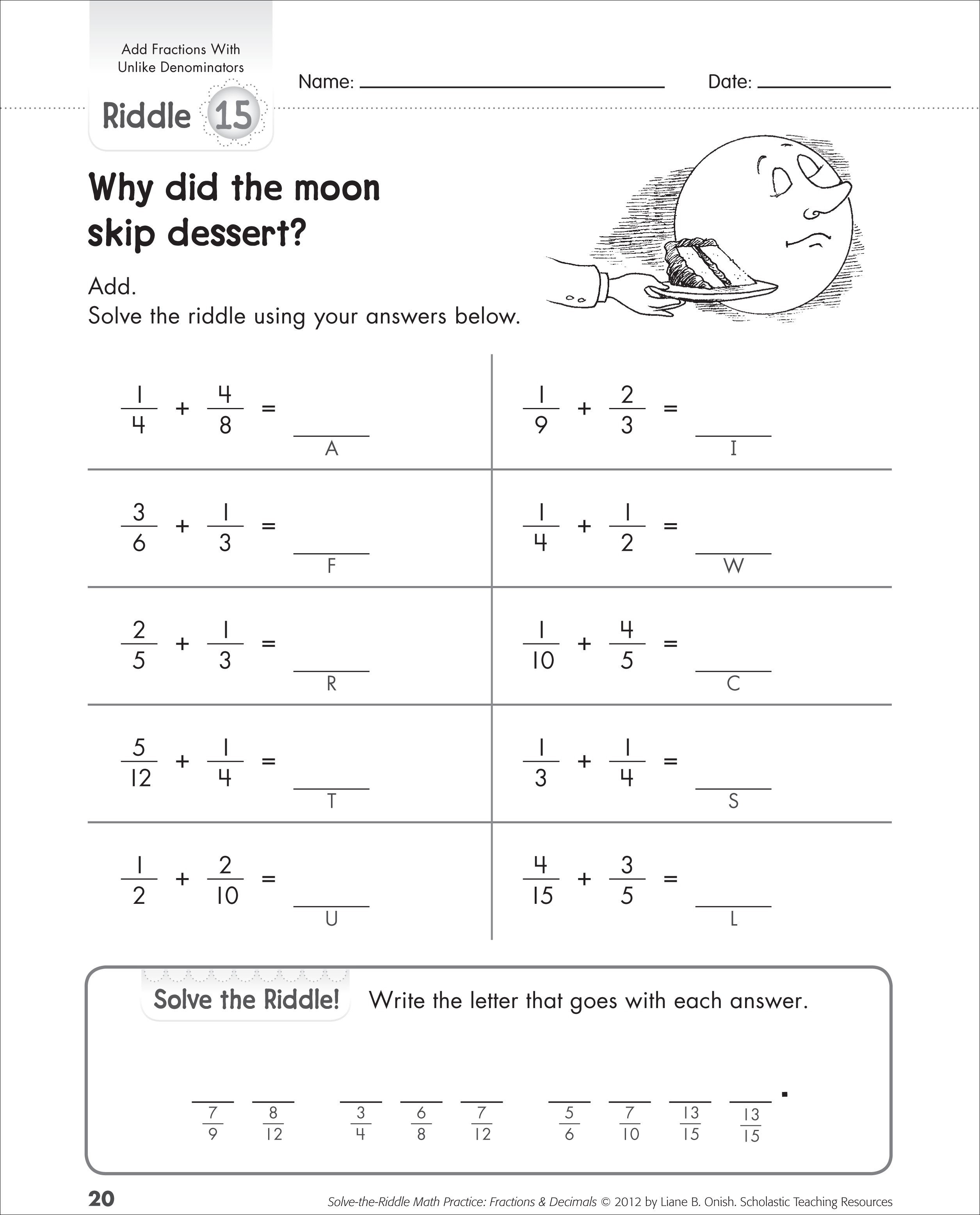
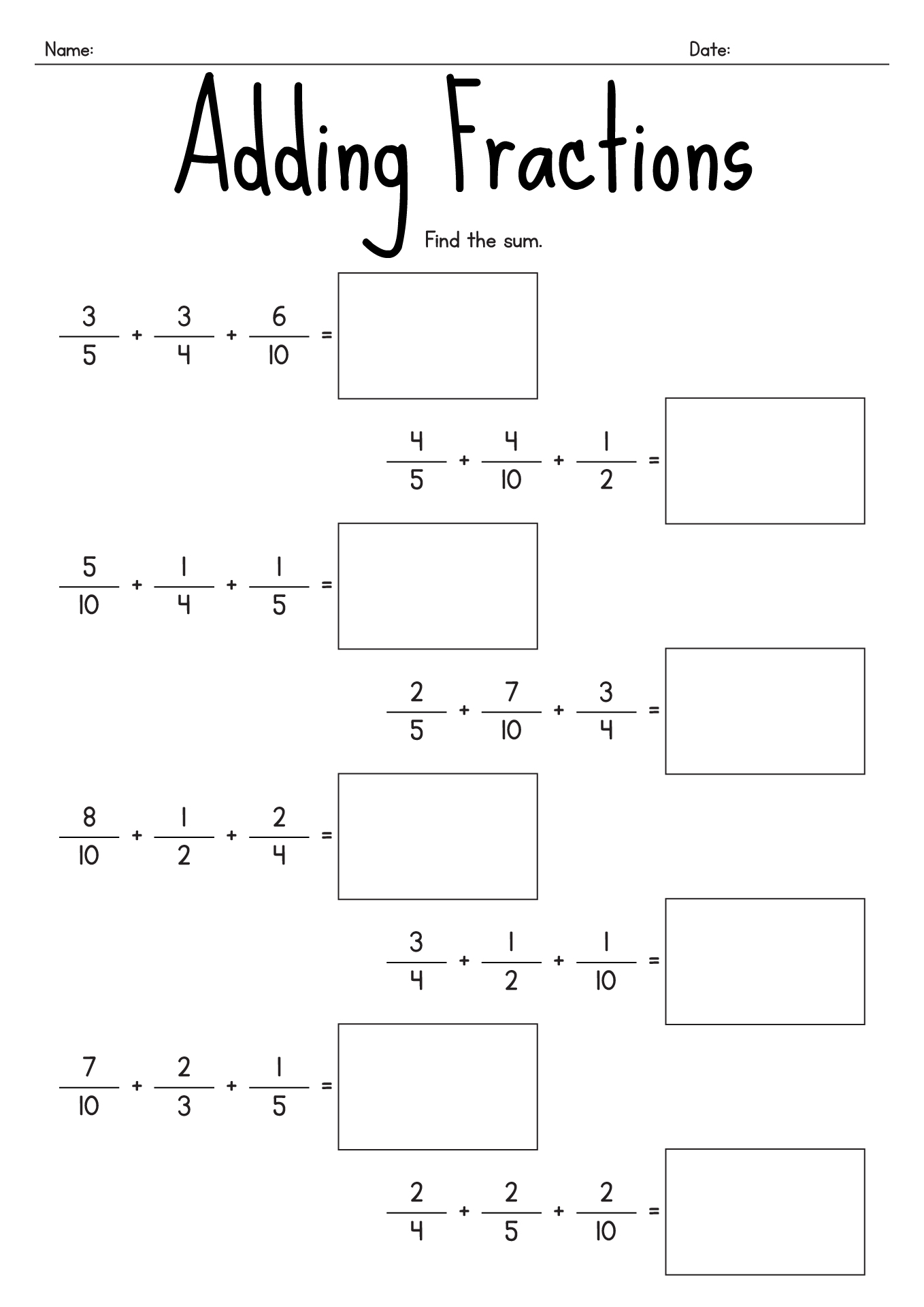
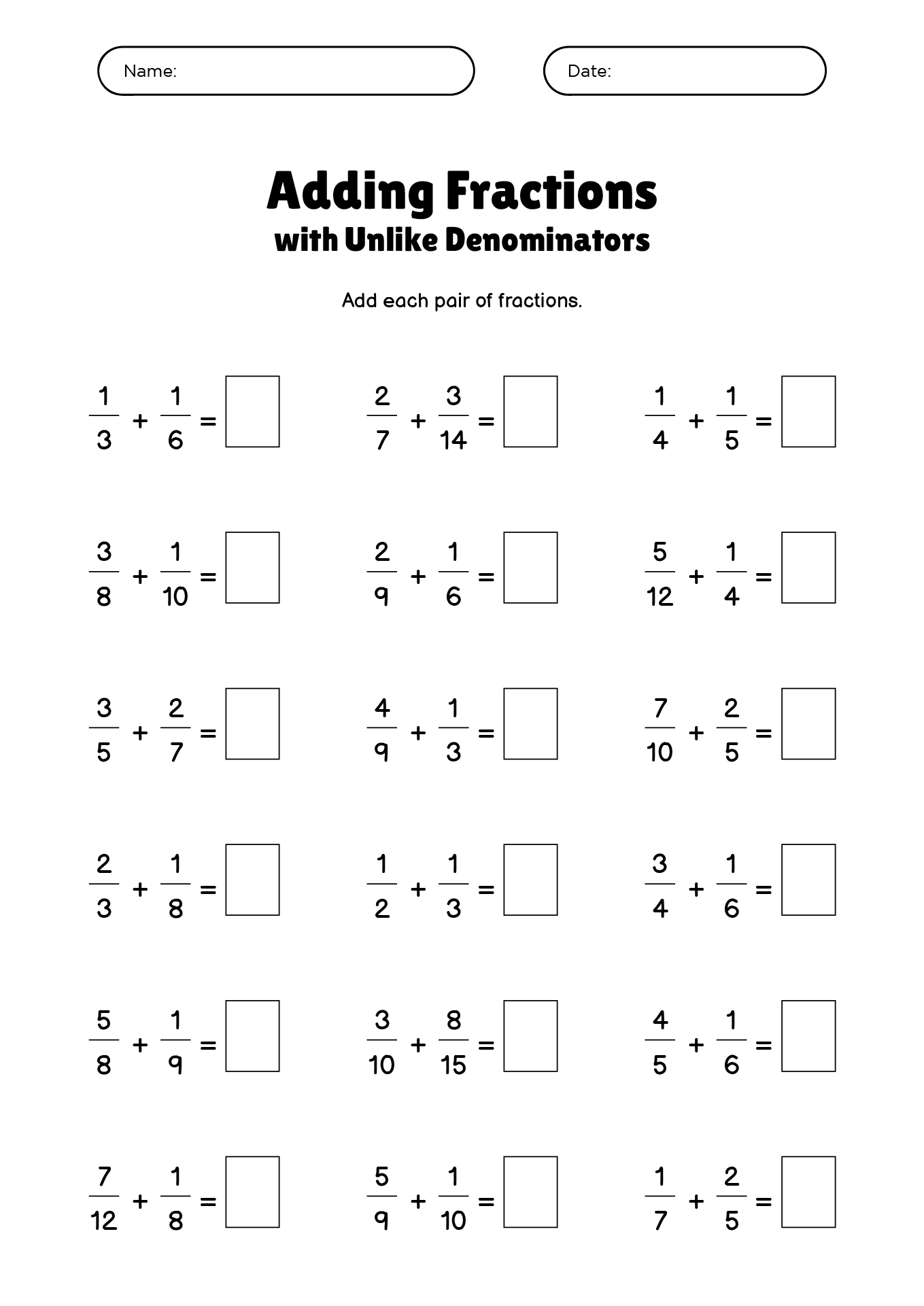
Adding Fractions Worksheets with Answer Key

If you're in need of practice activities to strengthen your skills in adding fractions, you'll find a collection of helpful worksheets right here. These worksheets feature a range of exercises that focus on adding fractions with like denominators, as well as those with different denominators. Each worksheet includes an answer key for easy self-assessment and progress tracking. Whether you're a student aiming to improve your math abilities or a teacher looking for additional resources for your classroom, these worksheets provide a valuable tool for honing your skills in adding fractions.

  11 Images of Adding Fractions Worksheets With Answer Key

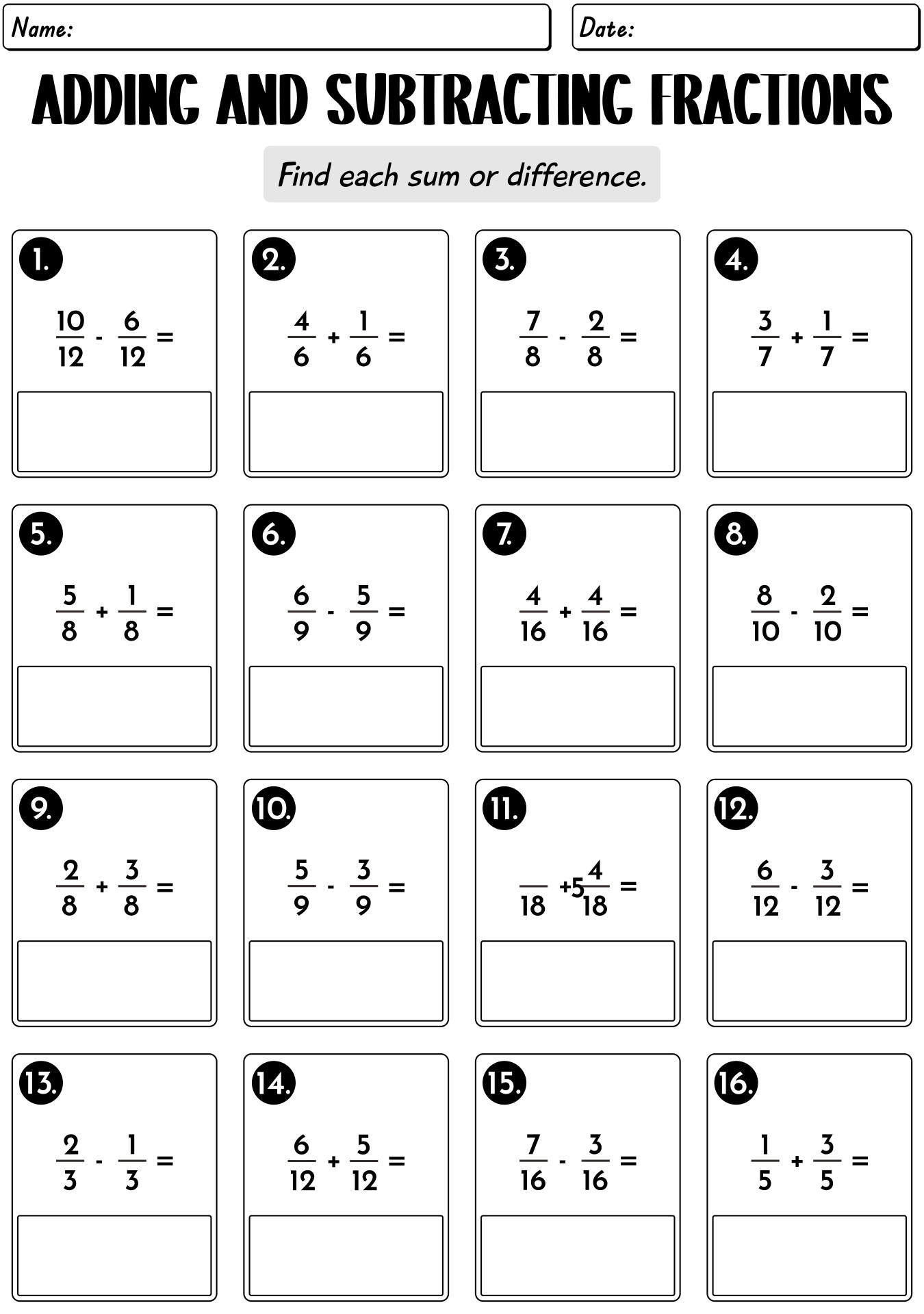
  Common Core Adding and Subtracting Fractions Worksheets

---

  Adding Fractions Worksheets 4th Grade

---

  Adding Fractions Common Denominator Worksheet

---

  Adding Fractions Worksheets Grade 4

---

  Subtracting Fractions with Unlike Denominators Worksheet

---

  Adding Fractions Worksheets 5th Grade

---

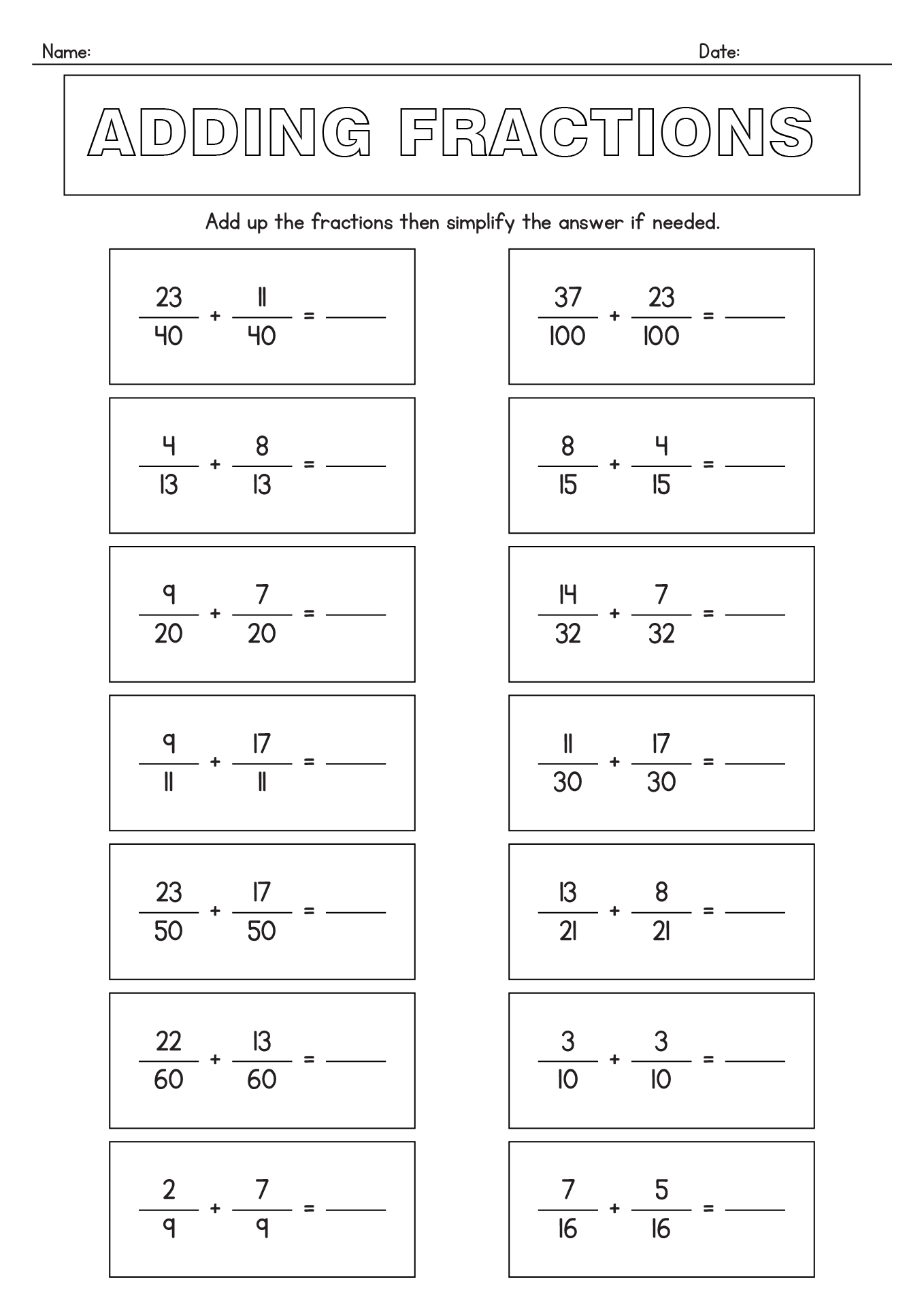
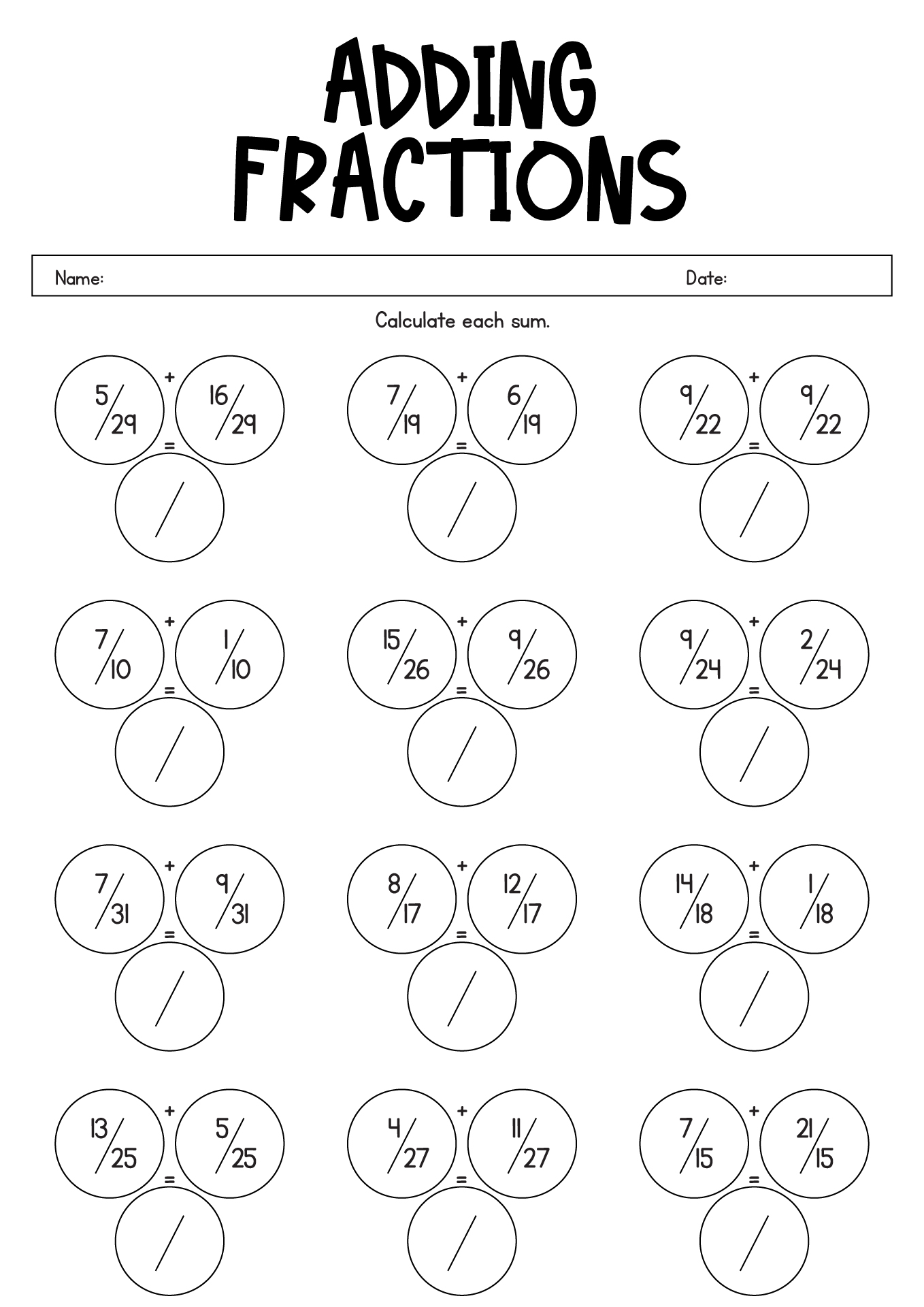
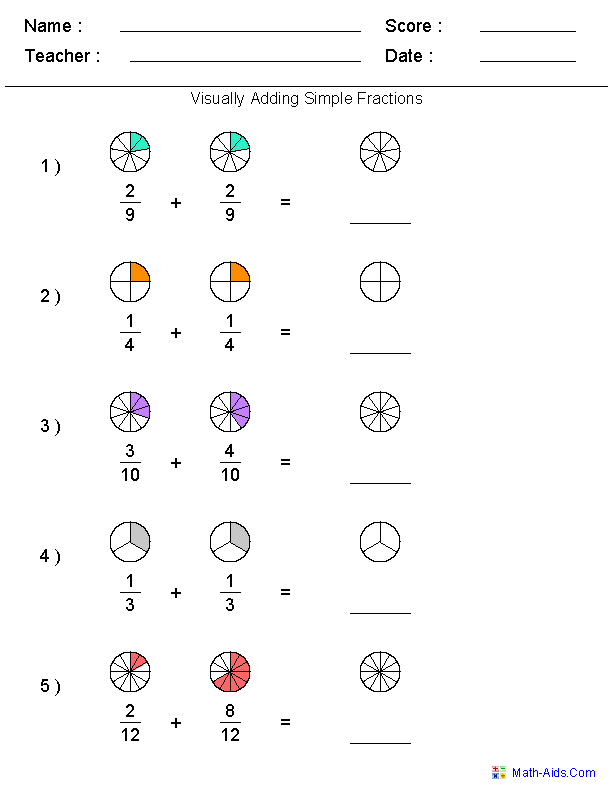
  Adding Fractions Worksheets

---

  Adding Fractions with Unlike Denominators Word Problems

---

  Adding Fractions Worksheets

---

  Practice Adding Fractions with Answer Key

---

  Adding Fractions Worksheets

---

Understanding how to add fractions can be challenging, but our Adding Fractions Worksheets with Answer Key provide the needed practice to master this essential skill.

What is the first step in adding fractions?

The first step in adding fractions is to make sure that the denominators of the fractions are the same. If the denominators are already the same, you can proceed to adding the numerators directly. If the denominators are different, you need to find a common denominator by finding the least common multiple of the two denominators and then converting the fractions to have the same denominator before adding them together.

How do you find a common denominator for fractions with different denominators?

To find a common denominator for fractions with different denominators, you need to identify the least common multiple (LCM) of the denominators. Once you have determined the LCM, you can use it as the common denominator for the fractions by rewriting each fraction with equivalent denominators. This enables you to add, subtract, or compare fractions with ease.

Can you add fractions with different numerators?

Yes, you can add fractions with different numerators by finding a common denominator. To do this, you need to find the least common multiple (LCM) of the denominators and then convert each fraction to have that common denominator. Once the fractions have the same denominator, you can add or subtract them by keeping the common denominator and adding or subtracting the numerators.

How do you add fractions with the same denominator?

To add fractions with the same denominator, simply add the numerators together and keep the denominator the same. For example, if you have 3/5 + 2/5, you would add the numerators (3 + 2 = 5) and keep the denominator the same (5), resulting in 5/5 which simplifies to 1.

What should you do if the fractions have different denominators?

When dealing with fractions that have different denominators, you should first find a common denominator by finding the least common multiple of the two denominators. Then, convert both fractions to equivalent fractions with the common denominator before performing any operations on them, such as addition, subtraction, multiplication, or division. This allows for easier and accurate calculations when working with fractions of different denominators.

How do you simplify the sum of fractions?

To simplify the sum of fractions, you first need to find a common denominator for all the fractions involved. Once you have a common denominator, you add the numerators together and keep the denominator the same. Finally, if possible, you simplify the resulting fraction by reducing it to its simplest form by dividing both the numerator and denominator by their greatest common factor.

Can you add fractions if they are in mixed number form?

Yes, you can add fractions in mixed number form by converting the mixed numbers to improper fractions and then adding them together. To do this, multiply the whole number by the denominator of the fraction, then add the numerator to get the new numerator, keeping the same denominator. Once both mixed numbers are converted to improper fractions, add them as you normally would, ensuring to simplify the final answer if needed.

How do you convert a mixed number into an improper fraction before adding?

To convert a mixed number into an improper fraction before adding, first multiply the whole number by the denominator of the fractional part, then add the numerator of the fractional part to the result. Place this sum over the original denominator to create the improper fraction. This process ensures that both numbers are in the same form, making addition easier.

What do you do if you end up with an improper fraction as the sum?

If you end up with an improper fraction as the sum, you can convert it to a mixed number by dividing the numerator by the denominator. The whole number result becomes the whole number component of the mixed number, and the remainder becomes the numerator of the new fraction with the same denominator.

Can you add fractions if they have negative values?

Yes, you can add fractions with negative values. When adding fractions with negative numbers, you simply follow the same rules as adding fractions with positive numbers. You add the numerators and keep the common denominator the same. The only difference when working with negative fractions is that you need to be mindful of the signs of the numerators.