5th Grade Division with Decimals Worksheets

Are you a 5th grade student struggling to grasp the concept of division with decimals? Worksheets can be an effective tool to help you practice and improve your skills in this area. By engaging with these specially designed resources, you will have the opportunity to strengthen your understanding of division and decimals, ultimately boosting your mathematical abilities.

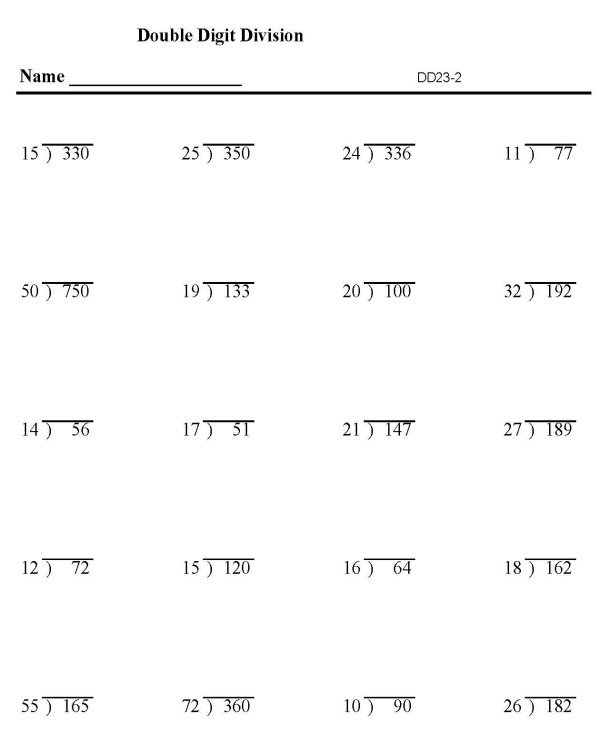
  Long Division Decimal Worksheets 5th Grade

---

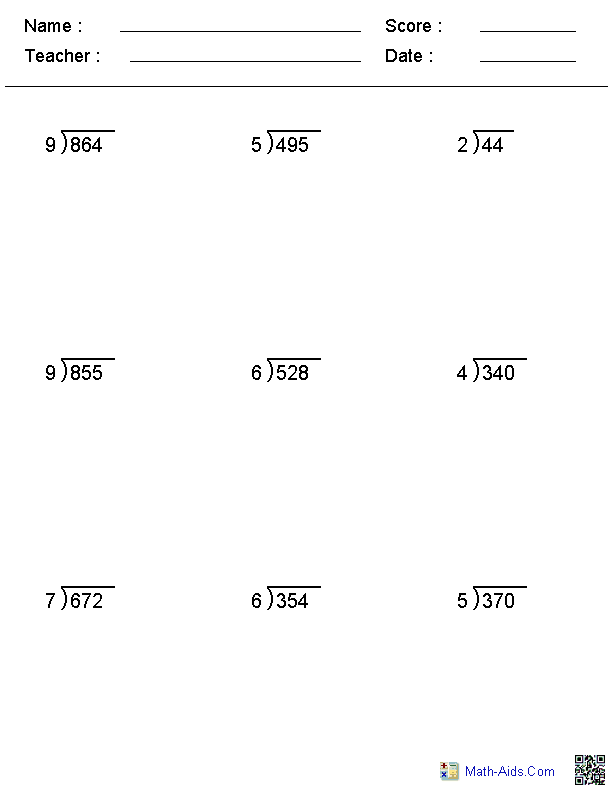
  Grade Long Division Worksheet

---

  Free Printable Decimal Division Worksheets

---

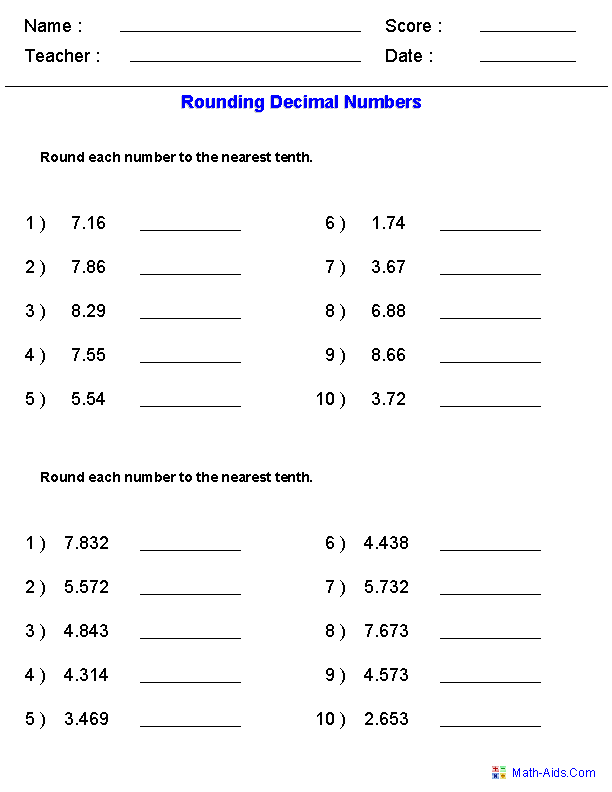
  Rounding Decimal Numbers Worksheets 5th Grade

---

  Dividing Decimals 6th-Grade Worksheets

---

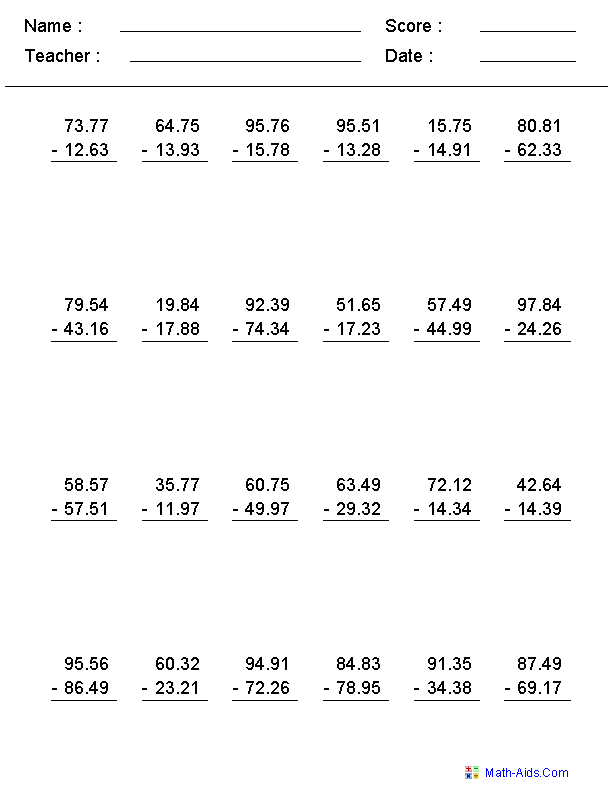
  Adding and Subtracting Decimals Worksheet 5th Grade

---

  Subtraction Worksheets with Decimals

---

  5th Grade Division Math Worksheets Printable

---

  Dividing Decimals Worksheet 5th Grade

---

  5th Grade Math Long Division Worksheets

---

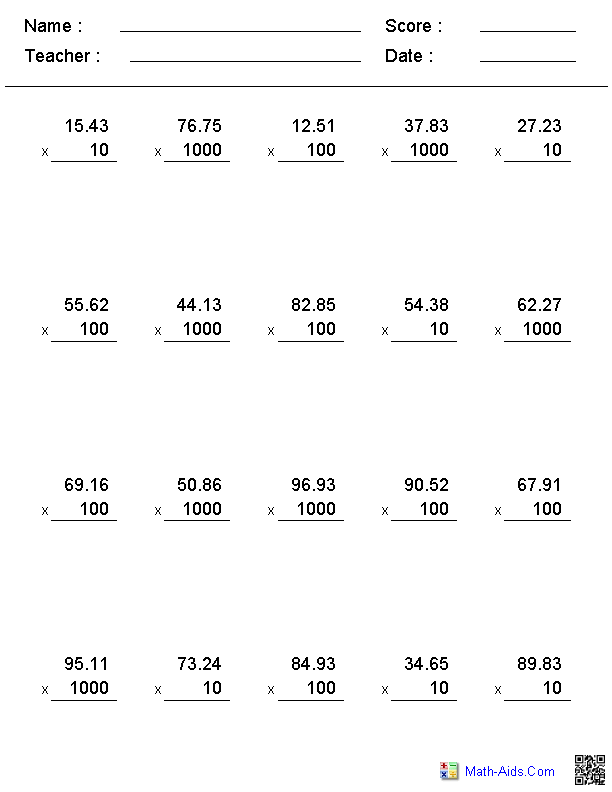
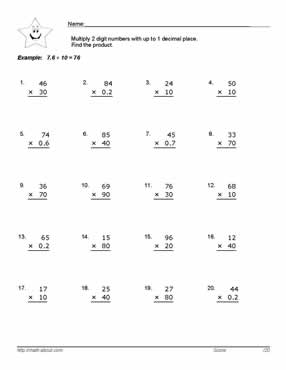
  Multiplying Decimals Worksheets 6th Grade

---

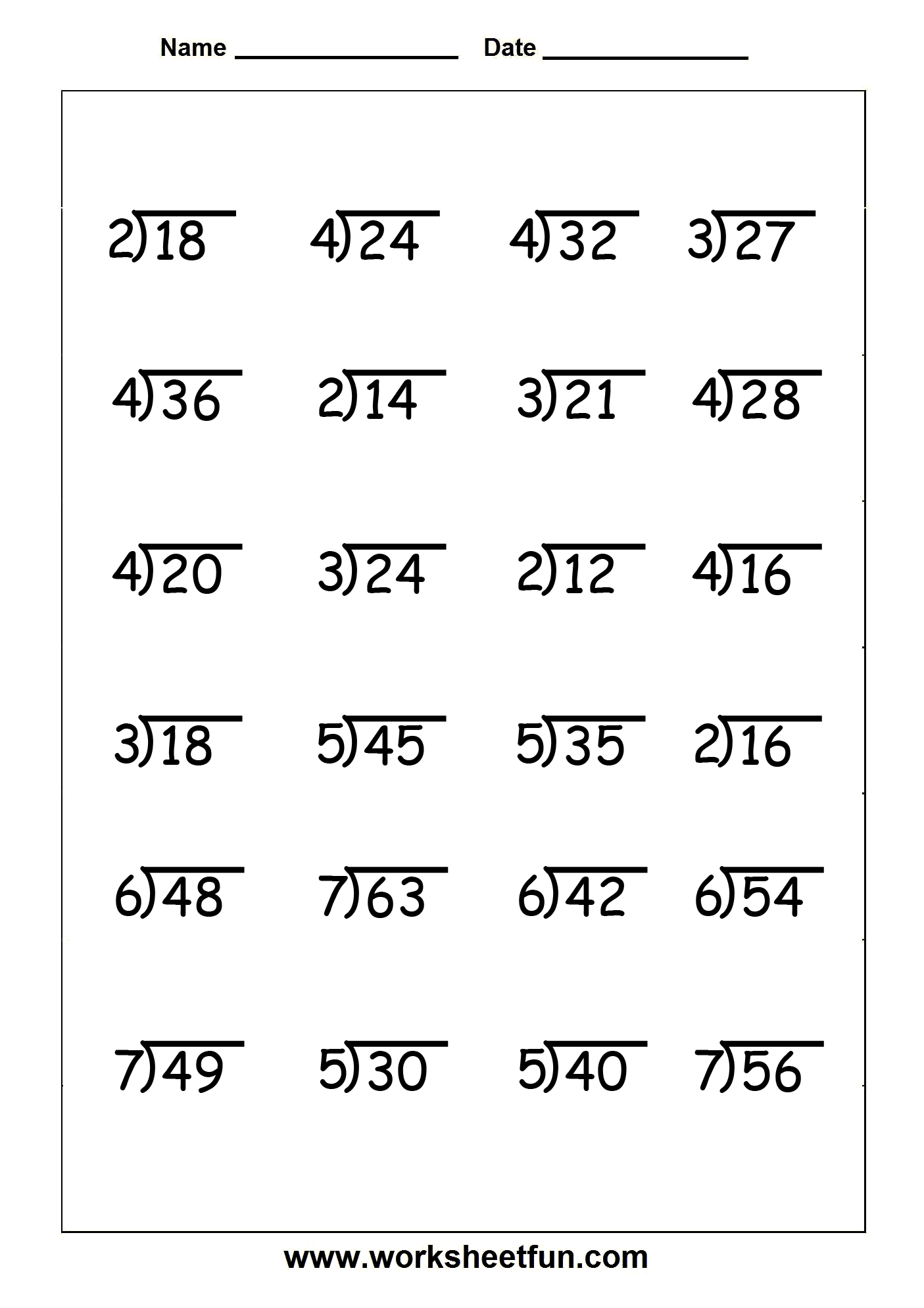
  Free Printable Division Worksheets 4th Grade

---

  Division Worksheets Grade 4

---

  Multiplication and Division Worksheets with Decimals

---

What is division with decimals?

Division with decimals is a mathematical operation where you divide a decimal number by another decimal number. The key is to align the decimal points and then perform long division as you would with whole numbers, but accounting for the decimal places in the numbers being divided. The quotient will also have the decimal point in the same position as the dividend.

How do you divide decimals by whole numbers?

To divide a decimal by a whole number, you can first ignore the decimal point and carry out the division as if you were dividing whole numbers. Once you have the quotient, you then place the decimal point in the answer directly above its position in the dividend to get the final result. It's essentially treating the decimal as a whole number before placing the decimal point correctly in the final answer.

How do you divide decimals by decimals?

To divide decimals by decimals, you can move the decimal point to the right in both numbers until the divisor is a whole number. Then, divide as you would with whole numbers. Finally, move the decimal point back to its original position in the quotient. Remember to add zeros to the dividend, if needed, to ensure the correct placement of the decimal point in the quotient.

Can you give an example of dividing a decimal by a whole number?

Sure! An example of dividing a decimal by a whole number is 3.6 ÷ 2. If we divide 3.6 by 2, we get 1.8. This means that 3.6 can be divided equally into 2 parts, with each part being 1.8.

Can you give an example of dividing a decimal by a decimal?

Sure! An example of dividing a decimal by a decimal is 2.5 divided by 0.5. This calculation would result in 5, as 2.5 divided by 0.5 equals 5.

How can you use estimation to divide decimals?

To use estimation to divide decimals, you can round the numbers to whole numbers and then perform the division. Once you have the approximate quotient, you can adjust it based on the decimal places you rounded off. For example, if you are dividing 5.2 by 1.3, you can round them to 5 and 1 to get an approximate quotient of 5. Then, adjust the quotient based on the decimals, in this case, adding 0.2 back to the result to get 4. You can use estimation as a quick way to get an approximate answer when dividing decimals.

What is the importance of the decimal point in division with decimals?

The decimal point is important in division with decimals as it helps separate whole numbers from the fractional parts of the quotient. It indicates the precise location where the division should be made between the whole number and the decimal places to ensure the correct calculation of the quotient with the appropriate level of accuracy. Without the decimal point, it would be challenging to distinguish between the whole number and the decimal part of the quotient, leading to incorrect results in division with decimals.

How can you check your answer when dividing decimals?

To check your answer when dividing decimals, you can multiply the quotient (result of the division) by the divisor (the number you divided by) and ensure that the product is equal to the dividend (the number you were trying to divide). This way, you can verify that your division was done correctly and that your answer is accurate.

How does division with decimals relate to real-life situations?

Division with decimals is commonly used in real-life situations to split things into smaller, more manageable portions. For example, dividing a total bill among a group of friends when dining out, calculating the average speed of a car during a trip, or determining the cost per unit when shopping in bulk. Division with decimals helps us distribute resources fairly, make informed decisions based on specific quantities, and manage budgets effectively in various everyday scenarios.

What are some common mistakes to avoid when dividing decimals?

When dividing decimals, it is important to remember to align the decimal points in the dividend and the divisor before performing the division. One common mistake to avoid is forgetting to move the decimal point to the right in both numbers to make the divisor a whole number. Additionally, always ensure that you are careful with the placement of the decimal point in the quotient; one misplaced decimal point can completely change the result of the division. Lastly, remember to apply the rules of decimal placement when working with the remainder to maintain accuracy in your division calculations.