Year 3 Maths Worksheets Partitioning

Are you searching for educational resources to support your child's understanding of partitioning in Year 3 maths? Look no further! With our carefully crafted worksheets, your child can develop their skills in partitioning numbers and gain confidence in this essential mathematical concept. Designed specifically for Year 3 students, these worksheets provide engaging activities that focus on the entity and subject of partitioning.

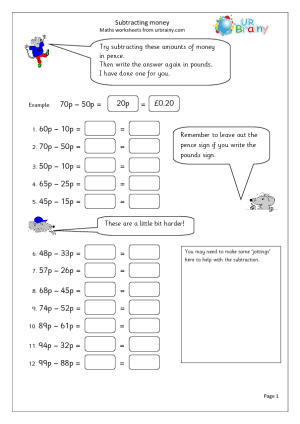
  Adding and Subtracting Money Worksheets

---

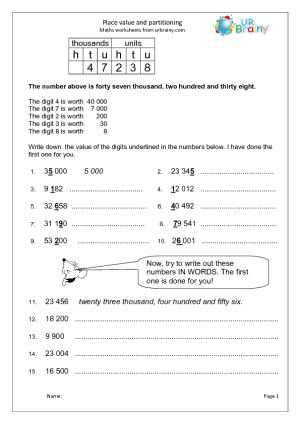
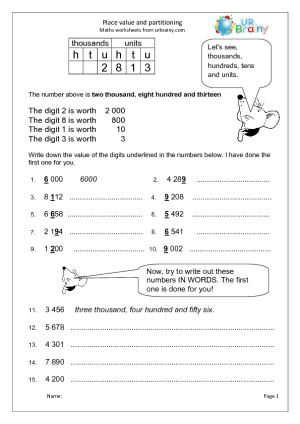
  Number Place Value Worksheets

---

  Place Value Worksheets 4 Year

---

  3 Grade Math Homework Sheets

---

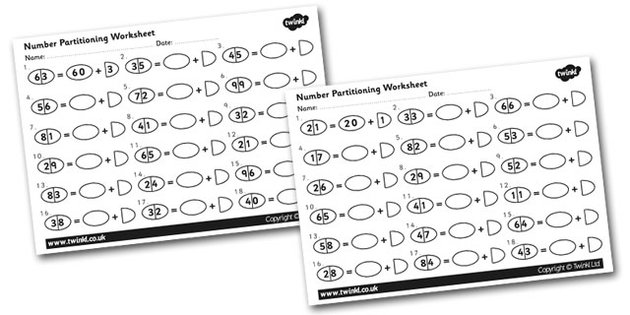
  Partitioning Numbers Worksheet

---

  4 Year Old Math Worksheets

---

  Year 3 Maths Worksheets

---

  Negative Numbers Worksheets

---

  Year 4 Maths Worksheets

---

  Year 7 Math Worksheets

---

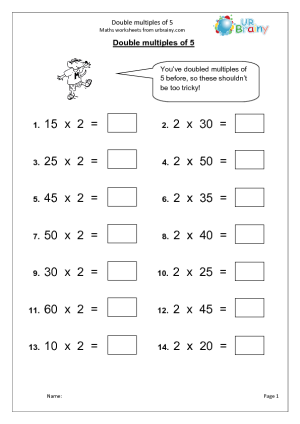
  Halving and Doubling Multiplication Worksheets

---

  Year Three

---

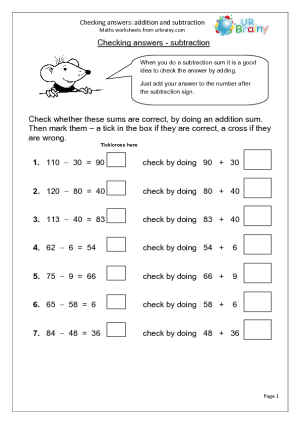
  Checking Addition Worksheets

---

What is partitioning in Year 3 Maths?

In Year 3 Maths, partitioning is the process of breaking down a number into smaller parts or components. This involves representing a number as the sum of its place value components, such as tens and ones. Partitioning helps students understand the concept of place value and how numbers are composed, making addition, subtraction, and various mathematical operations easier to grasp.

How is partitioning used to break down two-digit numbers?

Partitioning is used to break down two-digit numbers by separating the number into its tens and ones place. This involves decomposing the number into groups of ten and single units, allowing for easier manipulation and calculation. By breaking down the number into its place values, it becomes simpler to add, subtract, multiply, or divide the number and perform various mathematical operations efficiently.

What is the purpose of using partitioning to add or subtract numbers?

The purpose of using partitioning to add or subtract numbers is to break down complex calculations into smaller, more manageable parts. By breaking numbers into easier-to-work-with components, partitioning helps to simplify the arithmetic process and make it easier to perform mental calculations or work with larger numbers efficiently. This method is particularly useful for learners and individuals who are building their math skills or tackling more challenging mathematical problems.

How can partitioning be used to multiply two-digit numbers by one-digit numbers?

Partitioning can be used to multiply two-digit numbers by one-digit numbers by breaking down the multiplication process into easier steps. For example, to multiply 23 by 4, you can break down 23 into 20 and 3, multiply 20 by 4 to get 80, and then multiply 3 by 4 to get 12. Finally, add the results together, 80 + 12, to get the final product of 92. This method helps simplify the multiplication process and makes it easier to work with larger numbers.

How is partitioning helpful in understanding place value?

Partitioning is helpful in understanding place value because it allows us to break down numbers into their individual parts based on their place within the number (ones, tens, hundreds, etc.). By visually separating the digits within a number, students can better comprehend the value of each place and how they contribute to the overall value of the number. This makes it easier to perform operations, compare numbers, and manipulate digits when working with larger numbers. Overall, partitioning provides a more concrete way to grasp and work with the concept of place value.

What strategies can be used for partitioning larger numbers into thousands, hundreds, tens, and ones?

One strategy that can be used for partitioning larger numbers into thousands, hundreds, tens, and ones is to visually represent the number using a place value chart. Start by writing the number in expanded form showing the value of each digit in relation to its place value. Then, decompose the number by breaking it down into its thousands, hundreds, tens, and ones components. Another strategy is to use the traditional method of long division, where you divide the number by 1000 to find the number of thousands, then continue dividing the remainder to find the hundreds, tens, and ones. Practice with different numbers will help in mastering these strategies for partitioning larger numbers efficiently.

How can partitioning be used to compare two-digit numbers?

Partitioning can be used to compare two-digit numbers by breaking down each number into its tens and ones. By comparing the tens place first, it can be determined which number is greater or if they are equal. If the tens are the same, then the ones place is compared to determine the larger number. This strategy allows for a systematic approach to comparing two-digit numbers based on their place values.

Why is partitioning important in understanding regrouping or borrowing in subtraction?

Partitioning is crucial in understanding regrouping or borrowing in subtraction because it allows us to break down numbers into smaller, more manageable parts. By partitioning, we can see the value of each digit and easily identify where regrouping or borrowing needs to occur when subtracting. This helps in maintaining the correct place values and making the subtraction process more clear and organized.

How does partitioning help in solving word problems involving addition and subtraction?

Partitioning helps in solving word problems involving addition and subtraction by breaking down the numbers into more manageable parts. By breaking a number into smaller chunks, it becomes easier to add or subtract these parts separately before combining the results. This strategy helps in simplifying complex calculations, making it easier to solve word problems step by step.

How can partitioning be applied to solve problems involving money or measurement in Year 3 Maths?

Partitioning can be applied in Year 3 Maths to solve problems involving money or measurement by breaking down numbers into smaller, more manageable parts. For example, when adding or subtracting money amounts, students can break down the numbers into their tens and ones to simplify calculations. Similarly, when measuring lengths or distances, they can partition the units into smaller parts to make comparisons and conversions easier. This strategy helps students develop a deeper understanding of place value and improves their problem-solving skills in real-life situations involving money or measurement.