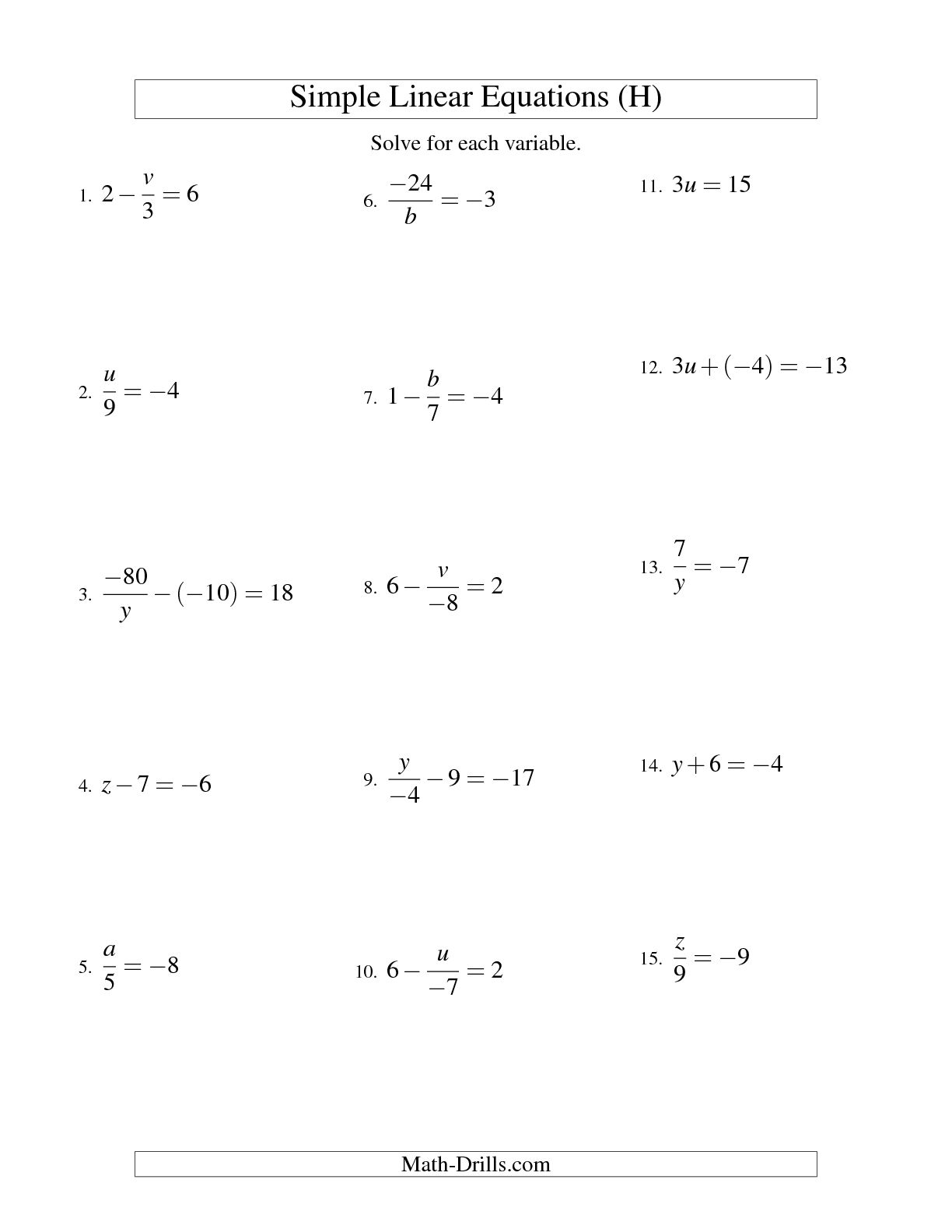
Writing Linear Equations Worksheet

Are you a math teacher searching for a useful resource to help your students practice writing linear equations? Look no further! We have created a comprehensive worksheet that focuses specifically on this essential topic. This worksheet provides various examples and exercises that will challenge and reinforce students' understanding of how to write linear equations. Whether you are teaching middle or high school students, our worksheet is designed to cater to their needs and improve their skills in this area.

  Linear Equations and Their Graphs Worksheet

---

  Equation

---

  Writing Equations From Tables Worksheets

---

  Graphing Linear Equations Worksheet Answers

---

  Kuta Software Infinite Algebra 1 Answers Key

---

  Slope-Intercept Form Worksheet

---

  Free Printable Linear Equation Worksheets

---

  Algebra 1 Worksheets

---

  How to Solve Slope-Intercept Form

---

  Multiplication Word Problems Worksheets

---

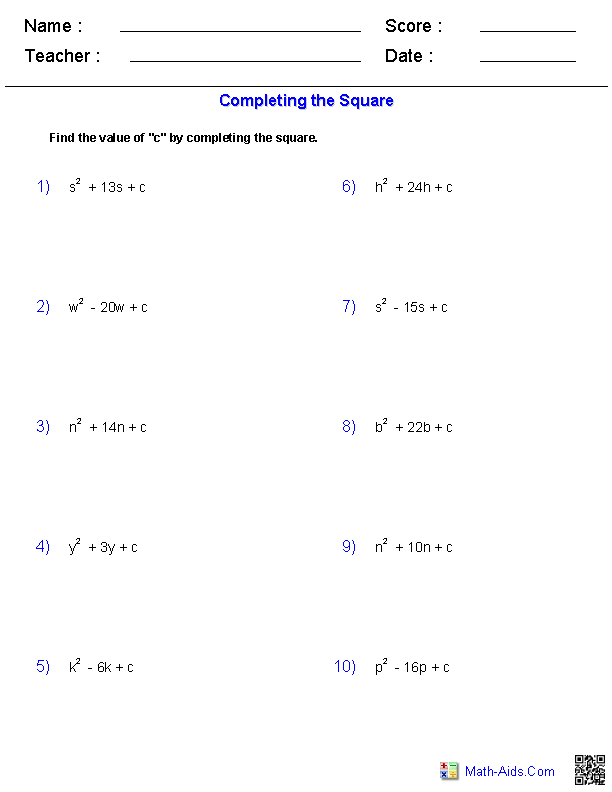
  Solving Equations Square Puzzle

---

  Writing Equations Kuta Software Infinite Algebra 1 Answers

---

  Algebraic Expressions Worksheets 6th Grade

---

  Math Word Problems for Grade 4

---

  6th Grade Math Word Problems Worksheets

---

  6th Grade Math Word Problems Worksheets

---

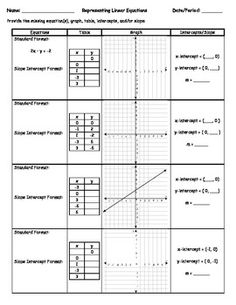
What is the general form of a linear equation?

The general form of a linear equation is written as Ax + By = C, where A, B, and C are constants, and x and y are variables with the exponent of 1.

How do you determine the slope of a linear equation?

To determine the slope of a linear equation, you can use the formula m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are any two points on the line. The slope, denoted by the letter m, represents the rate of change of the line. By calculating the change in the y-coordinates divided by the change in the x-coordinates between two points on the line, you can find the slope of the linear equation.

How do you find the y-intercept of a linear equation?

To find the y-intercept of a linear equation, you can set the x-coordinate to 0 in the equation and solve for y. The value of y that you get when x is 0 is the y-intercept of the line.

Explain how to write the equation of a line given its slope and y-intercept.

To write the equation of a line given its slope (m) and y-intercept (b), you can use the slope-intercept form of a linear equation, which is y = mx + b. Simply substitute the values of the slope and y-intercept into the equation to get the final equation of the line. For example, if the slope is 2 and the y-intercept is 3, the equation would be y = 2x + 3.

What is the point-slope form of a linear equation and how is it used?

The point-slope form of a linear equation is given by y - y1 = m(x - x1), where m is the slope of the line, and (x1, y1) is a point on the line. This form is used to represent a linear equation in terms of a specific point on the line and its slope. It is particularly useful when you have a point and the slope of a line, and you want to write the equation of the line passing through that point with that slope. By plugging in the values of the point and the slope into the equation, you can easily determine the equation of the line in a straightforward manner.

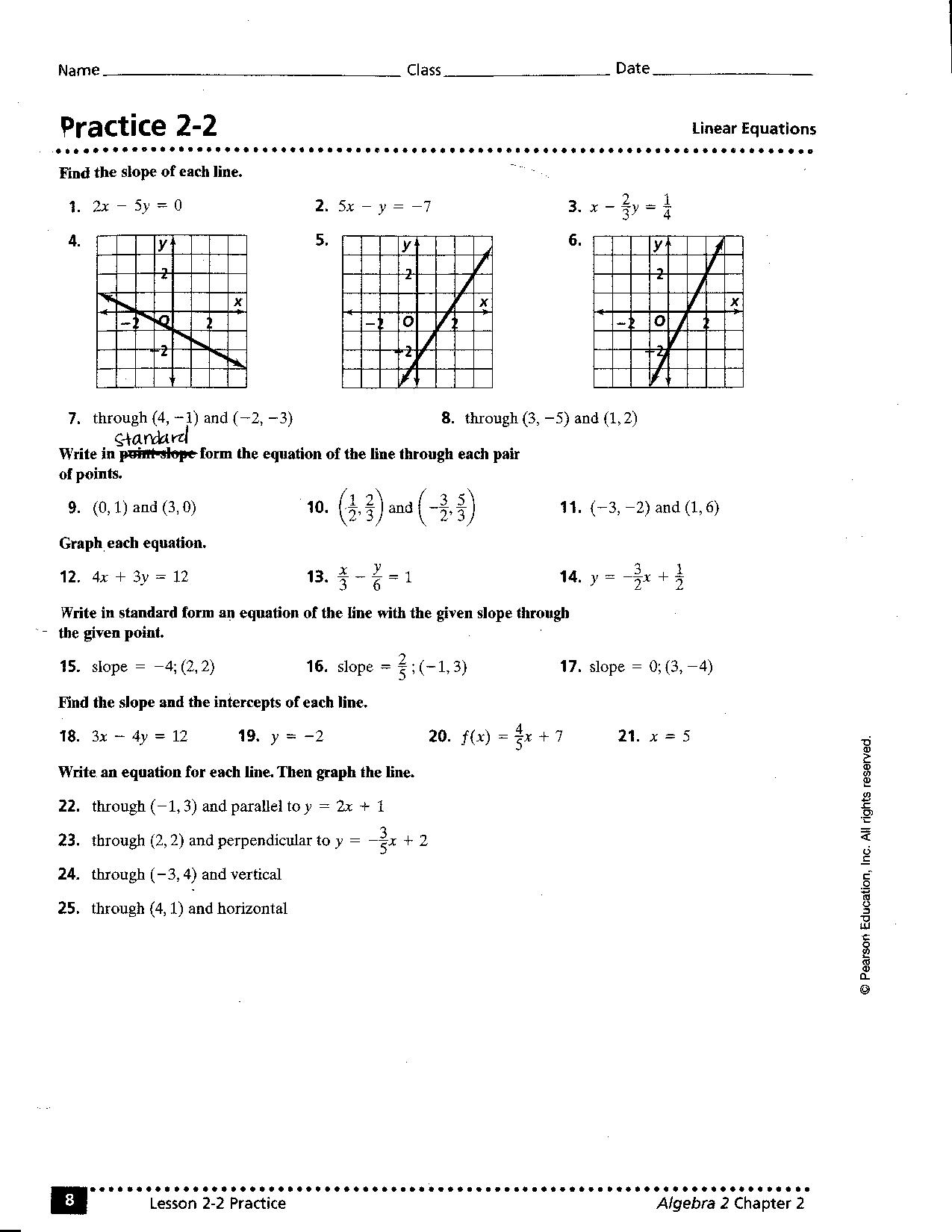
How do you convert an equation from standard form to slope-intercept form?

To convert an equation from standard form (Ax + By = C) to slope-intercept form (y = mx + b), you need to isolate y on one side of the equation. First, subtract Ax from both sides to get By = -Ax + C. Next, divide by B to get y = (-A/B)x + C/B, which can be simplified to y = mx + b, where m is the slope (-A/B) and b is the y-intercept (C/B).

Describe the process of finding the x-intercept of a linear equation.

To find the x-intercept of a linear equation, set y to zero and solve for x. This is because the x-intercept is the point where the graph crosses the x-axis, meaning at that point, the y-coordinate is zero. By setting y to zero in the equation and solving for x, you will find the x-coordinate of the x-intercept. It represents the value of x where the graph intersects the x-axis.

How do you determine if two lines are parallel or perpendicular based on their equations?

Two lines are parallel if their slopes are equal, which means that the coefficients of the variable terms in their equations are the same. On the other hand, two lines are perpendicular if the product of their slopes is -1, which means that the negative reciprocal of one slope is equal to the other slope. By comparing the coefficients of the variable terms or calculating the slopes, you can determine if two lines are parallel or perpendicular based on their equations.

Explain how to determine the equation of a line given its graph.

To determine the equation of a line given its graph, you can follow these steps: 1. Find the slope of the line by calculating the change in y-coordinates divided by the change in x-coordinates between two points on the line. 2. Use the slope-intercept form of a line, y = mx + b, where m is the slope and b is the y-intercept. 3. Determine the y-intercept by identifying the point where the line crosses the y-axis. 4. Substitute the slope and y-intercept into the slope-intercept form to get the equation of the line.

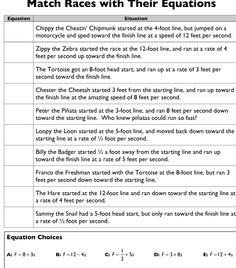
How can you use linear equations to solve real-life problems?

Linear equations can be used to solve real-life problems by representing relationships between variables in a simplified, straight-line manner. By defining the variables, setting up equations based on given information, and solving for the unknowns through algebraic manipulation, linear equations can help in areas such as budgeting, resource allocation, and predicting future outcomes in fields like economics, engineering, and science. By understanding and utilizing linear equations, one can efficiently analyze relationships and make informed decisions in various practical situations.