Simple Polynomial Factoring Worksheet
Polynomial factoring can be a tricky concept to grasp, especially for students who are new to algebra. However, with the right tools and resources, it becomes much more manageable. That's why we have created a simple polynomial factoring worksheet, designed to help students practice and strengthen their understanding of this fundamental mathematical concept. Whether you're a homeschooling parent, a teacher looking for additional resources, or a student looking for extra practice, this worksheet is the perfect tool to improve your skills in polynomial factoring.
Table of Images 👆
- Algebra 1 Factoring Polynomials Worksheet with Answers
- Algebra 1 Factoring Problems and Answers
- Factoring Quadratic Equations Worksheet
- Algebra Factoring Polynomials Worksheet
- Factoring Polynomials Worksheet
- Algebra 2 Factoring Review Worksheet Answers
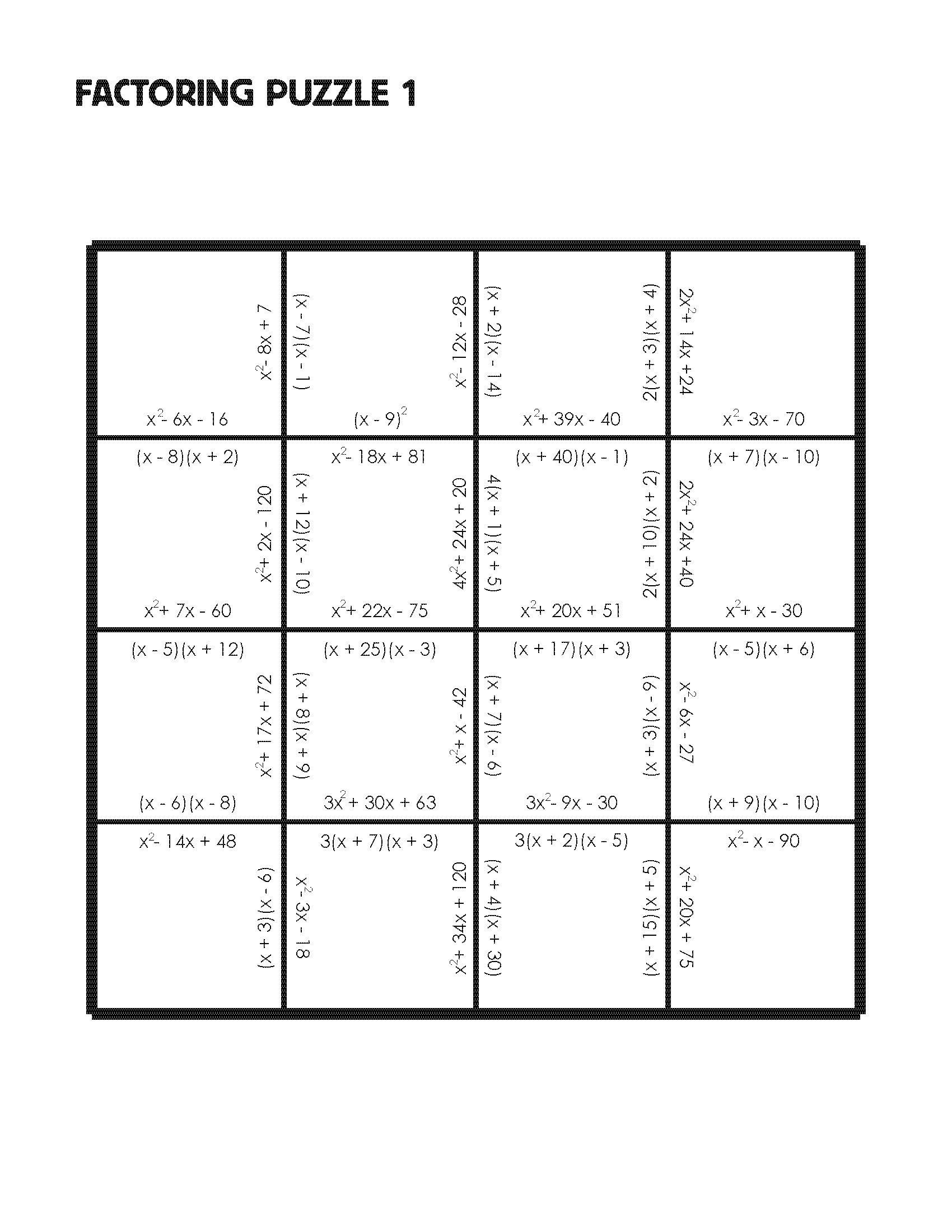
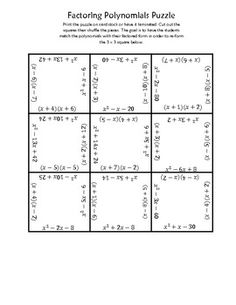
- Algebra 1 Factoring Puzzle Worksheets
- Factoring Cubic Polynomials
- Factoring Binomials Worksheet
- Factoring Polynomials by Grouping Problems
- Factoring with Coefficient Greater than 1
- Factoring Polynomials Worksheet Puzzle
- Factoring Puzzle Cut Out
- Factoring Greatest Common Factor Worksheet
- Factoring Puzzle Worksheet
- Math Algebra Equations Worksheets
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is a polynomial?
A polynomial is an algebraic expression consisting of variables, coefficients, and exponents that follow certain rules. It can have multiple terms, each involving a variable raised to a non-negative integer power, and these terms are combined using addition and subtraction. Examples of polynomials include expressions like 3x^2 + 2x - 5 or 4y^3 - y + 7.
What is factoring?
Factoring is the process of finding the factors of a given number or algebraic expression. In mathematics, factors are numbers or algebraic expressions that can be multiplied together to result in the original number or expression. Factoring is a fundamental concept in algebra and number theory, helping to simplify equations and expressions by breaking them down into their simpler components.
How can you determine if a polynomial can be factored?
You can determine if a polynomial can be factored by checking if it can be written as a product of simpler polynomials. One way to do this is by using techniques such as finding common factors, using the sum or difference of cubes formulas, or factoring by grouping. If after trying these methods the polynomial cannot be factored further, then it is considered to be fully factored.
What is the difference between factoring and expanding a polynomial?
Factoring a polynomial involves finding its equivalent form as a product of two or more simpler polynomials, while expanding a polynomial entails simplifying and multiplying out the terms of the polynomial to obtain a single expression. In other words, factoring breaks down the polynomial into simpler components, while expanding builds up the polynomial by multiplying out the terms.
How do you factor a polynomial that has a common factor?
To factor a polynomial that has a common factor, you can simply take out that common factor from each term in the polynomial. This common factor will be present in each term, so you can divide each term by this common factor to simplify the polynomial. By factoring out the common factor, you are essentially dividing each term by the factor and writing it as a product of the factor and the remaining terms.
How do you factor a polynomial that is a difference of squares?
To factor a polynomial that is a difference of squares, you need to recognize the pattern where you have two terms that are perfect squares connected by a subtraction sign. The general formula to factor a difference of squares is (a^2 - b^2) = (a + b)(a - b), where 'a' and 'b' are square roots of the terms being squared. Simply identify the squares in your polynomial expression, express them as the square of two terms, and then apply the formula to factor the polynomial into its corresponding binomial factors.
How do you factor a quadratic trinomial in the form ax^2 + bx + c?
To factor a quadratic trinomial in the form ax^2 + bx + c, where a, b, and c are constants, you need to find two numbers that multiply to a*c and add up to b. Then, you can rewrite the middle term as the sum of these two numbers, factor by grouping, and finally factor out common factors to arrive at the factored form of the trinomial.
What is a perfect square trinomial and how do you factor it?
A perfect square trinomial is a trinomial that can be factored into two identical binomial factors. To factor a perfect square trinomial, take the square root of the first and last terms, write them as the first terms of the binomials, and then write the middle term as the product of the square roots. The factored form of a perfect square trinomial will be in the form of (a ± b)².
How do you factor a polynomial using the grouping method?
To factor a polynomial using the grouping method, first, group the terms in pairs. Then, factor out the Greatest Common Factor (GCF) from each pair of terms. Next, look for a common factor between the resulting expressions in each group and factor it out. Finally, factor out the common binomial factor that remains after factoring out the terms in each group. This method helps break down the polynomial into simpler, more manageable factors.
What are some common factoring patterns to look out for when factoring polynomials?
Some common factoring patterns to look out for when factoring polynomials include factoring out a common monomial factor, recognizing the difference of squares pattern (a^2 - b^2), spotting perfect square trinomials (a^2 + 2ab + b^2 or a^2 - 2ab + b^2), and identifying the pattern for a sum or difference of cubes (a^3 + b^3 or a^3 - b^3). Additionally, recognizing quadratic trinomials that can be factored using methods such as grouping or trial and error is crucial when factoring polynomials.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.







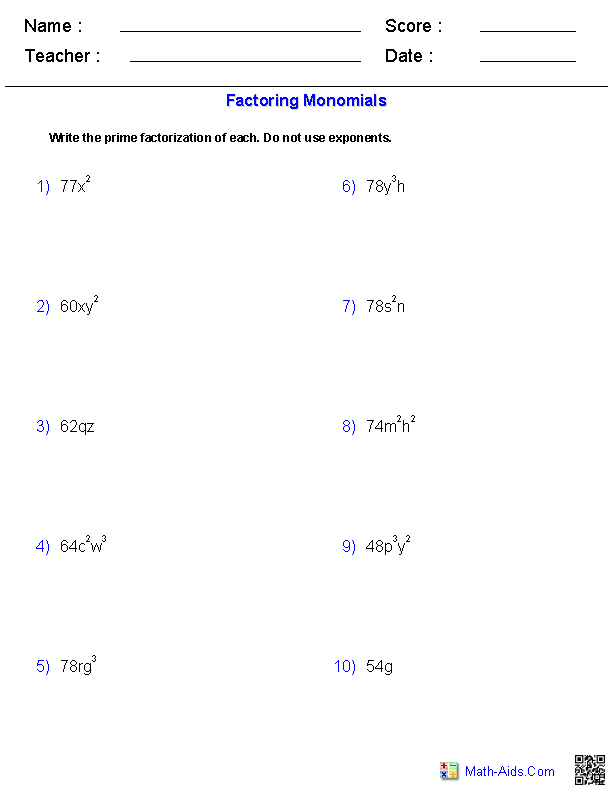


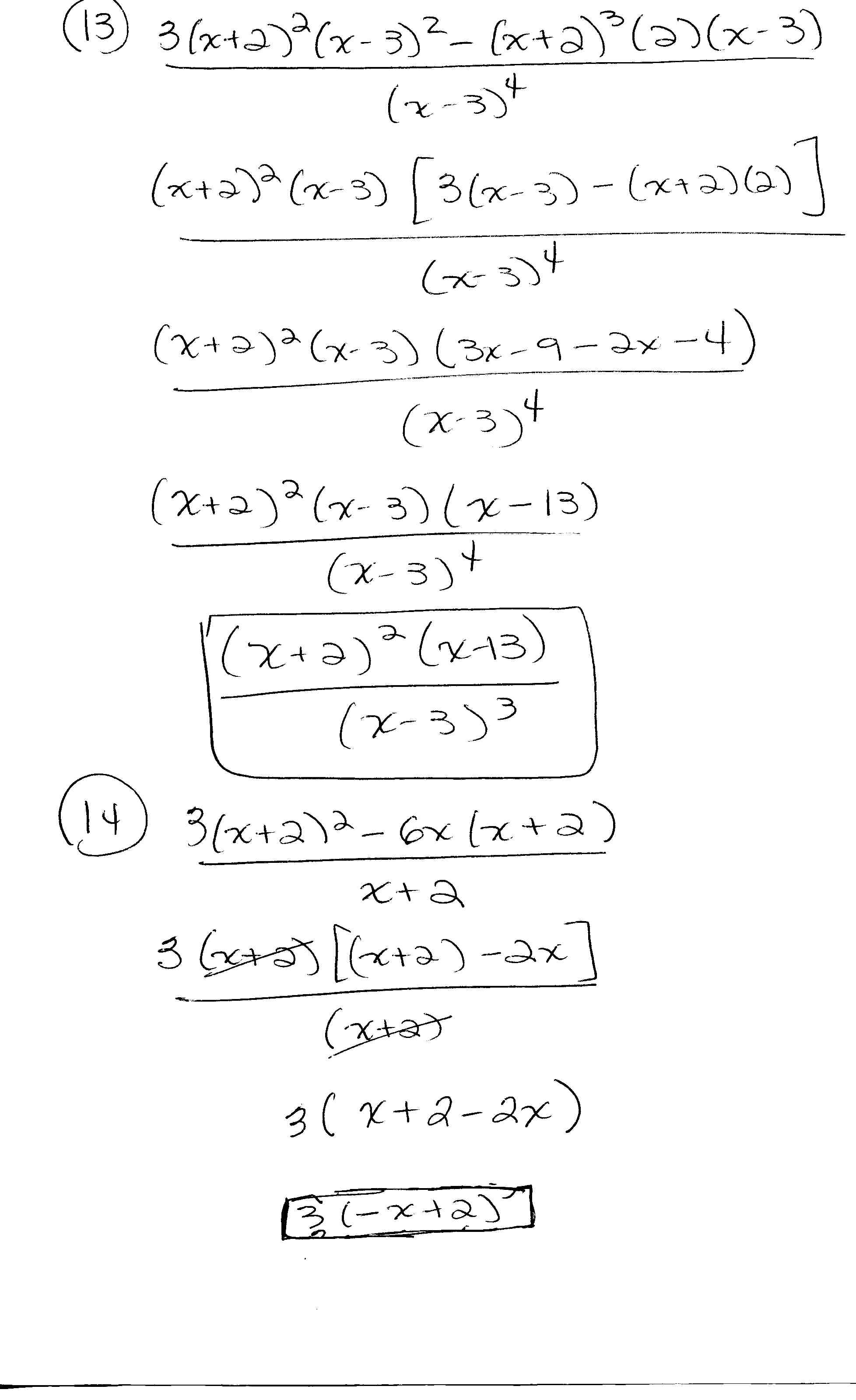

















Comments