Rational Expressions Worksheets PDF
Rational expressions worksheets are a valuable resource for students seeking to reinforce their understanding of this complex mathematical concept. Designed to provide practice and problem-solving opportunities, these worksheets are ideal for middle and high school students studying algebra or precalculus. By focusing on entity and subject, students can confidently tackle rational expressions and improve their skills in simplifying, multiplying, dividing, and adding these types of equations.
Table of Images 👆
- Simplifying Fractions Worksheet
- Subtracting Integers Worksheets 7th Grade
- Distributive Property and Factoring Worksheet
- Real Life Algebraic Expression Problems
- Glencoe Algebra 2 Answer Key Chapter 4
- This Algebra 1 Writing Variable Expressions Worksheets
- 2 Step Equation Puzzle Worksheet
- Adding and Subtracting Negative Numbers
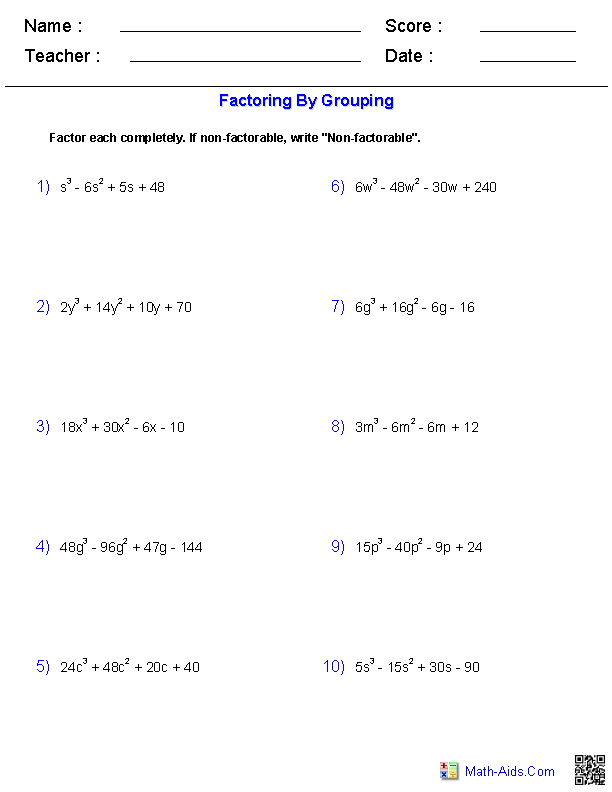
- Factoring by Grouping Worksheet
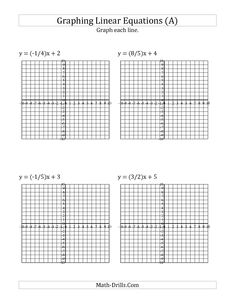
- Worksheets Slope-Intercept Equation
- Math Comic Strips
- Math Comic Strips
- Math Comic Strips
- Math Comic Strips
- Math Comic Strips
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What are rational expressions?
A rational expression is a fraction where both the numerator and the denominator are polynomials. It can be simplified by factoring the numerator and denominator and canceling out common factors. Rational expressions are widely used in algebra to represent relationships between variables and to solve equations involving fractions.
How are rational expressions simplified?
Rational expressions are simplified by factoring out common terms in the numerator and denominator, then canceling out the common factors. This process reduces the expression to its simplest form by removing any unnecessary terms or factors.
How do you add and subtract rational expressions?
To add or subtract rational expressions, first find a common denominator for the fractions. Multiply each fraction by the appropriate form of 1 to obtain equivalent fractions with the common denominator. Once all fractions have the same denominator, add or subtract the numerators, simplifying the resulting expression if needed. Keep in mind to always fully simplify the final expression to ensure it is in simplest form.
How do you multiply rational expressions?
To multiply rational expressions, simply multiply the numerators together to determine the new numerator, and multiply the denominators together to determine the new denominator. Then simplify the resulting expression by factoring and canceling out any common factors between the numerator and denominator if possible. Finally, rewrite the simplified expression in its lowest terms if necessary.
How do you divide rational expressions?
To divide rational expressions, you need to follow two steps: 1) Factor both the numerator and denominator completely to simplify the expressions, and 2) Multiply the first expression by the reciprocal of the second expression. This means you multiply the first rational expression by the second expression's denominator over its numerator. Then simplify the resulting expression by canceling out common factors if possible.
What are the restrictions on the variables in a rational expression?
In a rational expression, the variables cannot have values that make the denominator equal to zero, as division by zero is undefined. Therefore, the restrictions on the variables in a rational expression are any values that would cause the denominator to be equal to zero.
How do you solve equations involving rational expressions?
To solve equations involving rational expressions, you typically follow these steps: 1. Factor all the expressions to make the equations easier to work with. 2. Find the common denominator of the rational expressions involved. 3. Combine the fractions by adding or subtracting the numerators over the common denominator. 4. Solve the resulting equation using basic algebraic techniques like isolating the variable on one side of the equation. 5. Check your solution by ensuring it does not make any of the original expressions undefined.
How do you solve inequalities involving rational expressions?
To solve inequalities involving rational expressions, you follow the same steps as solving regular inequalities, but you must also consider the restrictions on the variables to avoid dividing by zero. First, find the critical points by setting the numerator and denominator of the rational expression equal to zero and then identifying any additional points where the inequality may change. Next, test a value in each interval determined by the critical points to determine the solution set that satisfies the inequality. Finally, express the solution set using interval notation and any other relevant restrictions on the variables.
How do you simplify complex rational expressions?
To simplify complex rational expressions, factor out common terms in the numerator and denominator, cancel out any common factors, and then simplify the expression further if possible by multiplying and dividing wherever necessary. It is important to also ensure that you simplify using the rules of exponents and algebra in order to simplify the expression as much as possible.
How do you graph rational functions?
To graph rational functions, first identify the vertical asymptotes by setting the denominator of the function equal to zero and solving for the values of x. Next, find the horizontal asymptote by comparing the degrees of the numerator and denominator functions. Then, plot any x or y-intercepts by solving the function for x = 0 or y = 0. Finally, connect the points using the behavior of the function near the asymptotes to create the graph of the rational function.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.








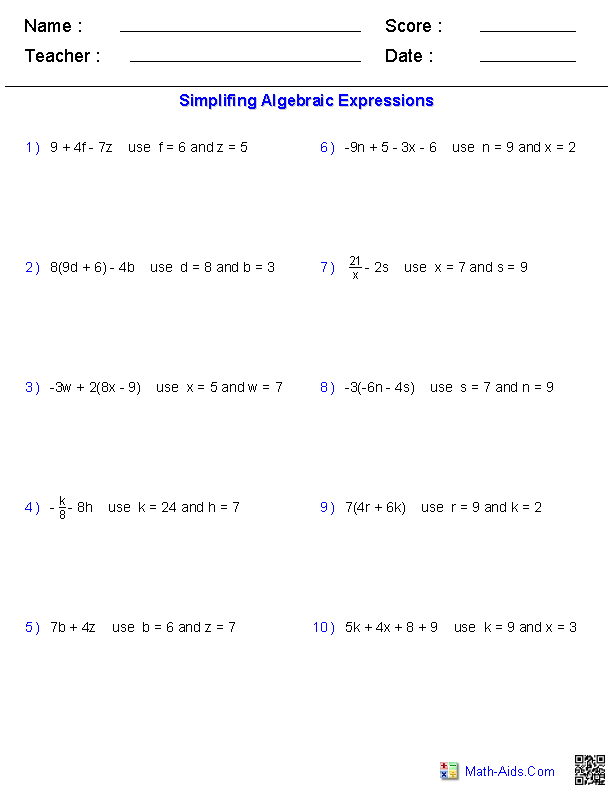















Comments