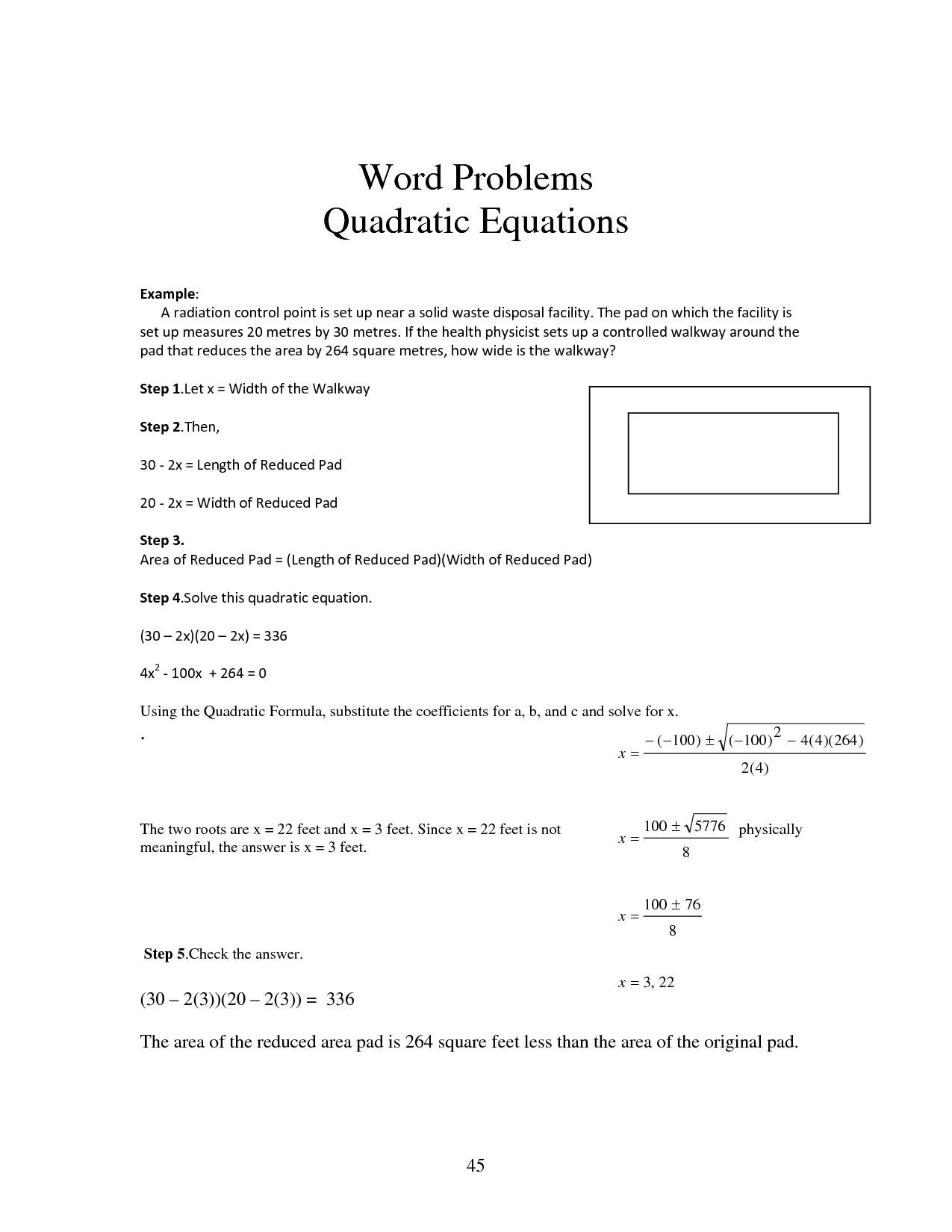
Quadratic Formula Worksheets with Answer Key

Quadratic formula worksheets provide a comprehensive way for students to practice and reinforce their understanding of solving quadratic equations. These worksheets contain a variety of problems that cover different levels of difficulty, catering to students of various abilities. With the included answer key, students can easily check their work and identify any areas for improvement. Whether you are a teacher searching for additional resources or a student looking to sharpen your quadratic equation skills, these worksheets are a valuable tool to help you excel in this subject.

  Algebra Solving Linear Equations Worksheets

---

  Empirical Formula Worksheet Answer Key

---

  Solving Quadratics Worksheet

---

  Times Tables Worksheets 3rd Grade

---

  Quadratic Word Problems Worksheet

---

  Subtracting and Adding Linear Expressions Worksheet

---

  Algebra 1 Worksheets

---

What is the quadratic formula used for?

The quadratic formula is used to solve quadratic equations of the form ax^2 + bx + c = 0, where x represents the unknown variable and a, b, and c are coefficients of the equation. By using the quadratic formula, one can find the roots or solutions of the quadratic equation, which are the values of x that satisfy the equation.

How is the quadratic formula derived?

The quadratic formula is derived using the method of completing the square on the general form of a quadratic equation ax² + bx + c = 0. By manipulating the equation and completing the square to express it in the form (x - p)² = q, where p and q are constants, the quadratic formula x = (-b ± ?(b² - 4ac)) / 2a can be derived. This formula provides the roots of a quadratic equation and is widely used in algebra and solving quadratic equations.

What are the coefficients in a quadratic equation?

The coefficients in a quadratic equation are the numerical values that are multiplied by each term in the equation. A quadratic equation is typically written in the form of \( ax^2 + bx + c = 0 \), where 'a', 'b', and 'c' are the coefficients for the quadratic term, the linear term, and the constant term respectively.

How do you identify the values of a, b, and c in a quadratic equation?

In a quadratic equation in the form of ax^2 + bx + c = 0, the values of a, b, and c can be identified directly from the equation itself. The coefficient of x^2 is 'a', the coefficient of x is 'b', and the constant term is 'c'. By comparing the given quadratic equation with the standard form, you can easily determine the values of a, b, and c to solve the equation.

What is the discriminant and how is it used in the quadratic formula?

The discriminant of a quadratic equation in the form ax^2 + bx + c = 0 is the expression b^2 - 4ac. It is used in the quadratic formula to determine the nature of the roots of the equation. If the discriminant is greater than 0, the equation has two distinct real roots; if it is equal to 0, the equation has two identical real roots; and if it is less than 0, the equation has two complex conjugate roots.

What are the two possible outcomes when using the quadratic formula?

The two possible outcomes when using the quadratic formula are: (1) two distinct real roots, which means the quadratic equation has two different solutions; or (2) two complex conjugate roots, which means the quadratic equation has no real solutions but two complex solutions.

How can the quadratic formula be used to solve real-life problems?

The quadratic formula can be used to solve real-life problems that involve situations where there are unknown values that can be represented by a quadratic equation. For example, it can be used in physics to determine the optimum angle for launching a projectile or in finance to calculate the break-even points in a business. By applying the quadratic formula, one can find the necessary solutions that provide meaningful insights and practical applications in various fields.

Can the quadratic formula be used for all types of quadratic equations?

Yes, the quadratic formula can be used for all types of quadratic equations, regardless of whether the coefficients are integers, fractions, or even complex numbers. As long as the equation is in the form of \( ax^2 + bx + c = 0 \) (where \( a \neq 0 \)), the quadratic formula can be applied to find the solutions for the quadratic equation.

Are there any limitations to using the quadratic formula?

Yes, there are some limitations to using the quadratic formula. One limitation is that it can only be used to solve quadratic equations, so if the equation is not quadratic, this formula cannot be applied. Additionally, if the equation has complex solutions, the quadratic formula may not be the most efficient method to use. Furthermore, it may not be the most practical method if the coefficients of the equation are very large or unwieldy, as it involves square roots which can lead to computational complexities.

How can the quadratic formula be graphically represented on a coordinate plane?

The quadratic formula, ax^2 + bx + c = 0, represents a parabolic curve on a coordinate plane. The graph of a quadratic equation is a U-shaped curve called a parabola. By plotting the y-values for different x-values using the formula y = ax^2 + bx + c, one can see how the parabola opens up or down, shifts left or right, and stretches or compresses. The x-intercepts of the parabola, where it crosses the x-axis, represent the solutions to the quadratic equation when y = 0, which can also be found using the quadratic formula.