Negative Exponents Worksheet with Answers
If you're in search of a comprehensive worksheet to practice negative exponents, then this blog post is just for you. This worksheet is designed to help learners grasp the concept of negative exponents and provides clear explanations and examples to reinforce understanding.
Table of Images 👆
- Exponents Worksheets
- Exponents with Negative Numbers Worksheets
- 7th Grade Math Worksheets Fractions
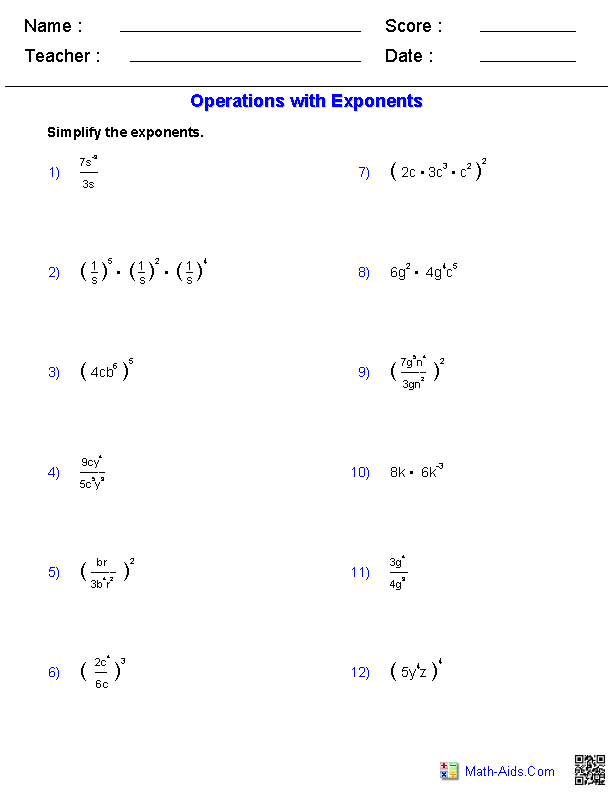
- Simplify Exponents Rules
- Powers and Exponents Worksheet
- Exponents
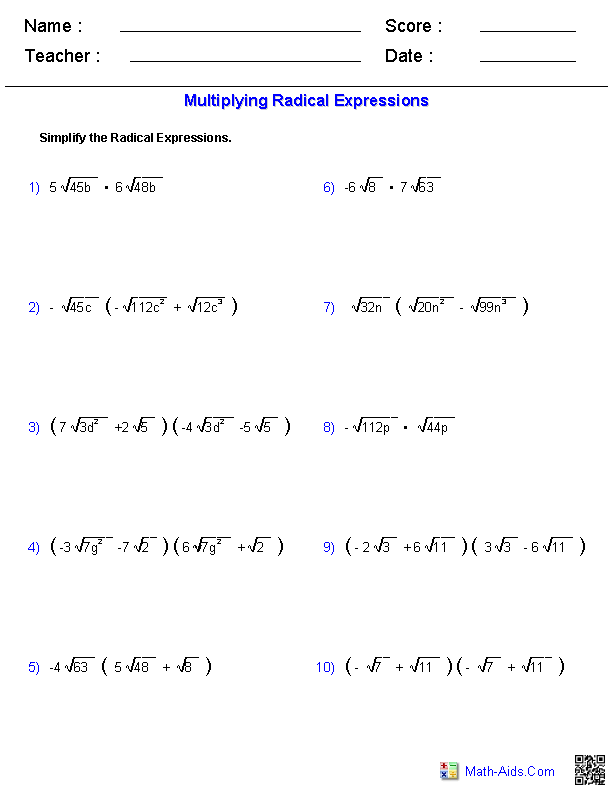
- Algebra 1 Radicals Worksheet
- Simplifying Expressions Worksheets 7th Grade
- 7th Grade Math Worksheets Algebra
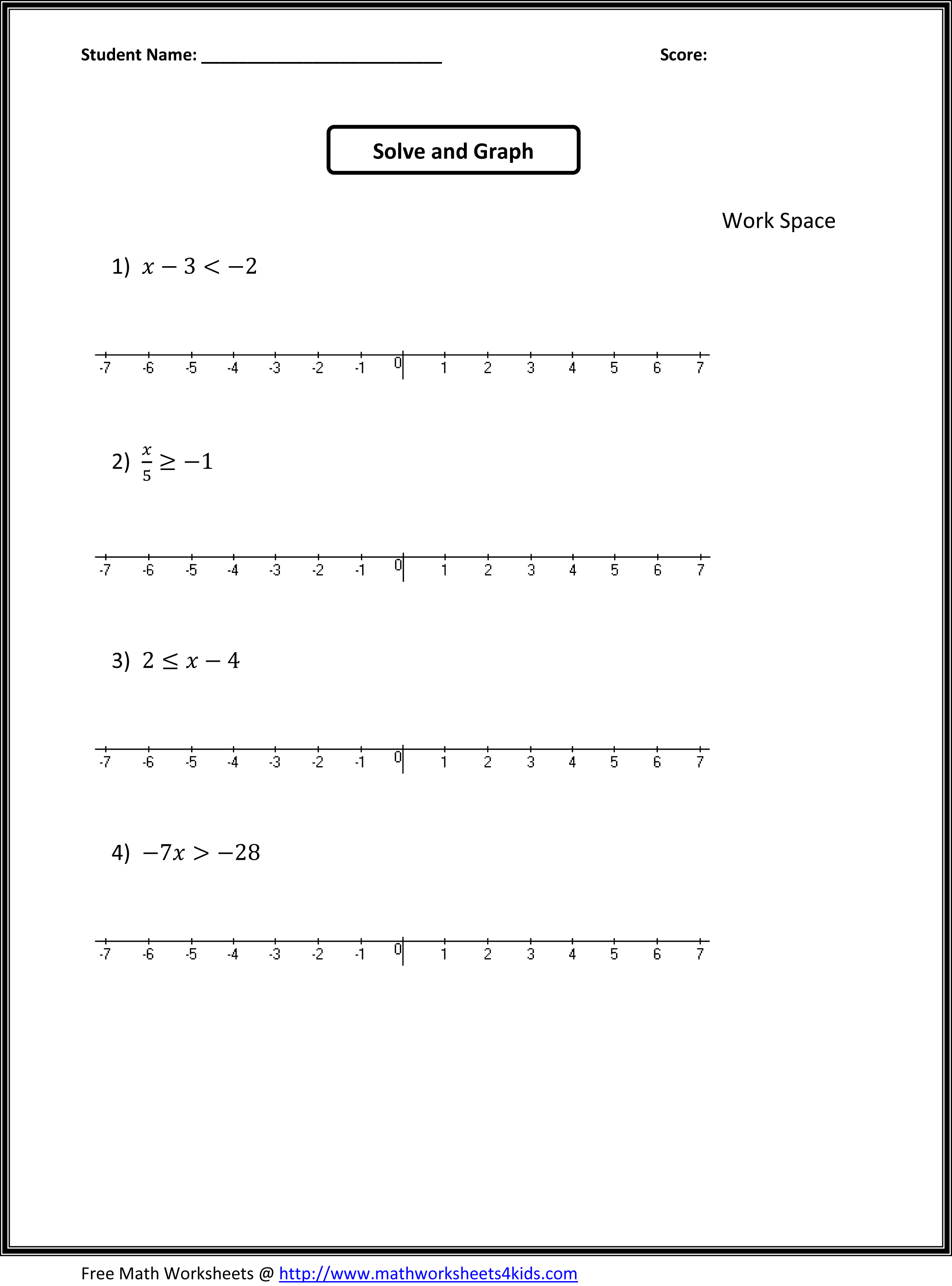
- Order of Operations Worksheets 5th
- Exponent Worksheets with Integers
- Math Word Problem Worksheets Integers
- Order of Operations Worksheets 5th Grade Math
- Multiplying Positive and Negative Integers Worksheets
- Prime Factorization with Exponents Worksheets
- 6th Grade Math Worksheets Integers
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
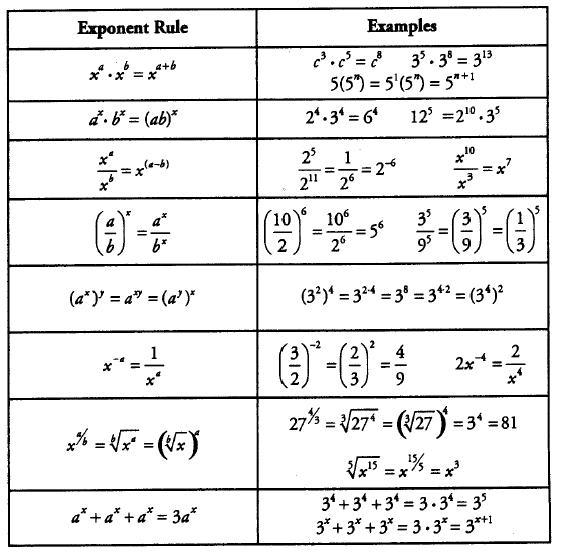
What is a negative exponent?
A negative exponent indicates that the base should be raised to the reciprocal of the exponent value. For example, if you have a number like 5^-3, it means 5 should be raised to the power of -3, which is equivalent to 1/(5^3) or 1/125. Negative exponents represent taking the reciprocal of the base raised to the positive version of the exponent.
How do you interpret a negative exponent?
A negative exponent signifies the reciprocal of the base raised to the positive equivalent of the exponent. For example, if a number is raised to the power of -n, it is equal to 1 divided by the number raised to the power of n. Negative exponents indicate fractions or decimal values that are less than one.
How do you convert a negative exponent to a positive exponent?
To convert a negative exponent to a positive exponent, you can take the reciprocal of the base raised to the positive value of the exponent. For example, if you have x^-n, you can rewrite it as 1/(x^n). This is based on the rule that x^(-n) is equivalent to 1/(x^n) in exponential notation.
What is the rule for dividing numbers with negative exponents?
When dividing numbers with negative exponents, you can rewrite them with positive exponents by moving the base to the opposite side of the fraction bar and changing the sign of the exponent. In other words, for any nonzero number 'a' and integers 'm' and 'n', a^(-m) / a^(-n) = a^(n - m).
How do you simplify a term with a negative exponent?
To simplify a term with a negative exponent, you can move the term with the negative exponent to the denominator of a fraction and change the exponent to positive. For example, if you have a term like x^-2, you can rewrite it as 1/x^2. This way, you convert the negative exponent into a positive one and simplify the term.
What is the rule for multiplying numbers with negative exponents?
When multiplying numbers with negative exponents, you can move the base with the negative exponent to the denominator and change the exponent sign to positive. This means that if you have a number with a negative exponent in the numerator, you can move it to the denominator with a positive exponent, or if the negative exponent is in the denominator, you can move it to the numerator with a positive exponent. This rule helps simplify expressions and solve calculations involving negative exponents.
How do you handle negative bases with negative exponents?
To handle negative bases with negative exponents, firstly, convert the negative exponent to a positive exponent by taking the reciprocal of the base. For example, if you have a negative base \( b \) raised to the power of a negative exponent \( -n \), rewrite it as \( \frac{1}{b^{-n}} = b^n \). This allows you to work with the positive exponent and calculate the result accordingly.
What is the result of raising a number with a negative exponent to the power of zero?
Raising a number with a negative exponent to the power of zero will result in 1. This is because any non-zero number raised to the power of zero is always equal to 1, regardless of its sign or whether it is raised with a positive or negative exponent.
What is the rule for raising a fraction with a negative exponent to a positive exponent?
To raise a fraction with a negative exponent to a positive exponent, you can rewrite the fraction as its reciprocal (flipping the numerator and denominator), then change the negative exponent to a positive one by moving the expression to the denominator (or vice versa if the exponent becomes positive). This allows you to apply the positive exponent to the fraction while maintaining the correct mathematical operations.
How do you simplify expressions with negative exponents?
To simplify expressions with negative exponents, you can move the term with the negative exponent to the denominator and change the exponent to a positive one. For example, if you have a term like x^-2, you can rewrite this as 1/x^2. This is because x^-2 is the same as 1/x^2. Then, you can combine like terms and perform any necessary operations to simplify the expression further.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.










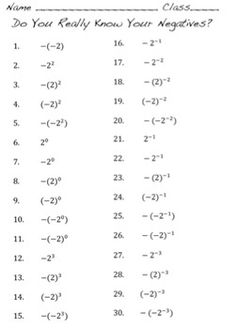
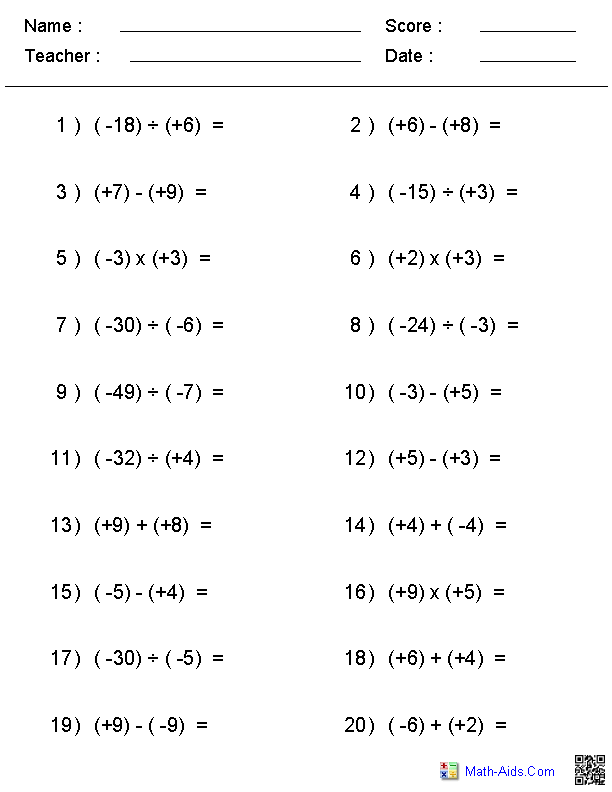

















Comments