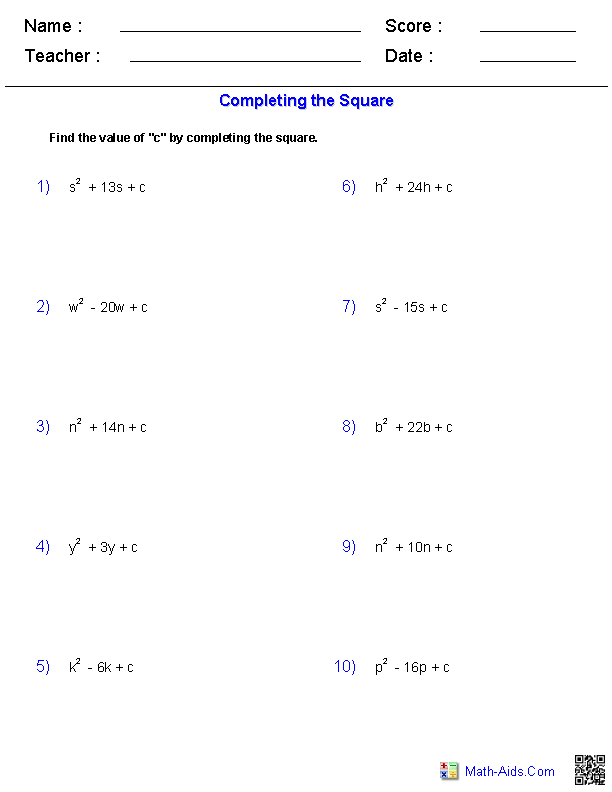
Linear-Quadratic Exponential Worksheet

If you're an educator or a student who needs additional practice with linear, quadratic, and exponential functions, you're in luck! This blog post introduces a helpful resource - the Linear-Quadratic Exponential Worksheet. Whether you're looking to strengthen your understanding of these mathematical concepts or simply want extra practice problems, this worksheet serves as the perfect tool.

  Algebra 1 Worksheets

---

  Pre-Algebra Curriculum Map

---

  Math Function Table Worksheets

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

  Regression Analysis Excel

---

What is a linear equation?

A linear equation is an algebraic equation that represents a straight line on a graph. It consists of variables raised to the first power and constant coefficients. The general form of a linear equation is y = mx + b, where y represents the dependent variable, x represents the independent variable, m is the slope of the line, and b is the y-intercept.

What is a quadratic equation?

A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where x represents an unknown variable, and a, b, and c are constants with a not equal to 0. Quadratic equations typically produce a curve called a parabola when graphed, and they have two solutions through the quadratic formula or factoring methods.

What is an exponential equation?

An exponential equation is an equation in which the variable appears in the exponent. It can be written in the form y = a^x, where a is a constant base and x is the variable raised to a power. Exponential equations arise in various fields such as mathematics, economics, and physics, and they often involve growth or decay processes that can be modeled using exponential functions.

What are the key characteristics of a linear equation?

A linear equation is an algebraic equation of the form y = mx + b, where x and y are variables, m is the slope, and b is the y-intercept. The key characteristics of a linear equation are that it represents a straight line on a graph, has a constant rate of change (slope), and the variables x and y have a power of 1. These equations can be used to model relationships between two variables that have a linear dependence on each other.

What are the key characteristics of a quadratic equation?

A quadratic equation is a polynomial equation of the second degree, having the general form of ax^2 + bx + c = 0, where a, b, and c are constants with a ? 0. The key characteristics of a quadratic equation include having a degree of 2, containing a term with a squared variable, typically graphing as a parabola, and potentially having two real or complex solutions depending on the value of the discriminant b^2 - 4ac.

What are the key characteristics of an exponential equation?

An exponential equation is characterized by having a variable as an exponent, typically involving a constant base raised to a varying power. These equations grow or decay rapidly as the input changes, exhibiting exponential growth if the base is greater than 1, and decay if the base is between 0 and 1. Exponential equations often involve compound interest, population growth, radioactive decay, or other phenomena that demonstrate rapid change over time. They can be used to model a wide range of real-world situations with exponential behavior.

How can you determine if an equation is linear, quadratic, or exponential?

To determine if an equation is linear, quadratic, or exponential, you need to look at the degree of the variables involved. A linear equation involves variables raised to the power of 1, they have a constant rate of change. A quadratic equation involves variables raised to the power of 2, they have a parabolic shape. An exponential equation involves variables as exponents, they have a constant ratio of change. By analyzing the powers of the variables in the equation, you can classify it as linear, quadratic, or exponential based on these distinctive characteristics.

What are the common applications of linear equations?

Linear equations are commonly used in various fields such as economics for cost and revenue analysis, engineering for modeling systems and designing structures, physics for solving motion and energy problems, and even everyday situations like budgeting and planning. They provide a simple and powerful way to represent relationships between variables and make predictions based on the given conditions.

What are the common applications of quadratic equations?

Quadratic equations have a wide range of applications in various fields such as physics, engineering, economics, and statistics. Some common applications include modeling the trajectory of a projectile, predicting the profit or loss of a business, determining the optimal size of a product to minimize costs, analyzing the behavior of certain physical systems, and solving optimization problems. Additionally, quadratic equations are also used in computer graphics for tasks like simulating motion, drawing curves, and creating 3D models.

What are the common applications of exponential equations?

Exponential equations have a wide range of applications in various fields such as finance for calculating compound interest, biology for modeling population growth, physics for describing radioactive decay, and economics for analyzing growth rates of variables like GDP. They are also used in engineering for modeling processes with exponential growth or decay, in computer science for analyzing algorithms with exponential complexity, and in chemistry for studying the kinetics of reactions. Overall, exponential equations are fundamental in understanding and predicting phenomena that involve exponential growth or decay.