Linear Function Tables Worksheet

Are you searching for a resource that can help your students practice linear functions? Look no further! In this blog post, we will introduce you to a useful tool for teaching and reinforcing linear functions – the Linear Function Tables worksheet. Designed with the needs of middle and high school students in mind, this worksheet focuses on the concept of linear functions and provides ample practice for students to master the subject. Whether you're a math teacher or a homeschooling parent, this worksheet is a valuable addition to your teaching arsenal.

  Input and Output Tables Graphing

---

  Representing Linear Functions Worksheets

---

  Algebra Interpreting Graphs Worksheet

---

  Linear Piecewise Functions Worksheet

---

  Equation

---

  Math Statistics Formulas Cheat Sheet

---

What is a linear function?

A linear function is a mathematical function that can be represented by a straight line graph. It has the general form of f(x) = mx + b, where "m" represents the slope of the line and "b" represents the y-intercept. Linear functions show a constant rate of change, meaning that for every unit increase in the independent variable "x," there is a constant increase or decrease in the dependent variable "y.

How can a linear function be represented in a function table?

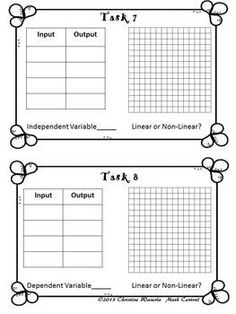
A linear function can be represented in a function table by listing input values in one column and their corresponding output values in another column. The input values are usually denoted by the variable 'x', while the output values are denoted by the variable 'y'. Each row in the table represents a pair of input and output values, where the output value can be calculated using the equation of the linear function, typically in the form of y = mx + b, where 'm' represents the slope of the line and 'b' represents the y-intercept. By inputting different x values in the table and calculating the corresponding y values using the linear function equation, you can create a complete set of data points that represent the linear relationship between the input and output values.

What is the relationship between the input and output values in a linear function?

In a linear function, the relationship between the input and output values is constant and can be represented by a straight line on a graph. The output values increase or decrease at a consistent rate as the input values change, following the formula y = mx + b, where m represents the slope of the line, which determines how much the output changes for a given change in the input, and b is the y-intercept, which is the value of y when x is zero.

How do you find the slope of a linear function from a function table?

To find the slope of a linear function from a function table, you can choose any two points on the table, (x1, y1) and (x2, y2). The slope is then calculated by dividing the difference in y-coordinates by the difference in x-coordinates, which is (y2 - y1) / (x2 - x1). This ratio represents the rate of change of the function and is the slope of the linear line connecting those two points on the table.

What does the slope represent in a linear function?

In a linear function, the slope represents the rate of change of the dependent variable in relation to the independent variable. It indicates how much the dependent variable changes for a unit change in the independent variable. A positive slope means that the dependent variable increases as the independent variable increases, while a negative slope means that the dependent variable decreases as the independent variable increases.

How do you find the y-intercept of a linear function from a function table?

To find the y-intercept of a linear function from a function table, identify the point where the input value (x) is 0. The corresponding output value (y) at x=0 is the y-intercept of the linear function. It is the point where the line crosses the y-axis on a graph, representing the value of y when x is zero in the function.

What does the y-intercept represent in a linear function?

The y-intercept in a linear function represents the value of the dependent variable (y) when the independent variable (x) is equal to zero. It is the point where the graph of the function intersects the y-axis.

How can you determine if a function table represents a linear function?

To determine if a function table represents a linear function, examine the differences in consecutive output values. If the differences between output values are constant for every pair of consecutive inputs, then the function is linear. Additionally, you can calculate the slope of the function by dividing the change in output values by the change in input values. If the slope remains constant, the function is linear.

How can you graph a linear function using a function table?

To graph a linear function using a function table, first list a set of input values in the table. For each input value, use the linear function to calculate the corresponding output value. Plot points on a coordinate plane using the input and output values as coordinates. Connect the points with a straight line to graph the linear function, showing the relationship between the input and output values visually.

How can you use a linear function table to solve real-world problems?

You can use a linear function table to solve real-world problems by inputting the relevant variables and then using the linear formula to calculate the corresponding output values. By analyzing the relationships between the variables in the table, you can make predictions, identify trends, and solve problems related to rates of change, growth, costs, revenue, and other quantitative relationships in various scenarios such as business, economics, physics, and more.