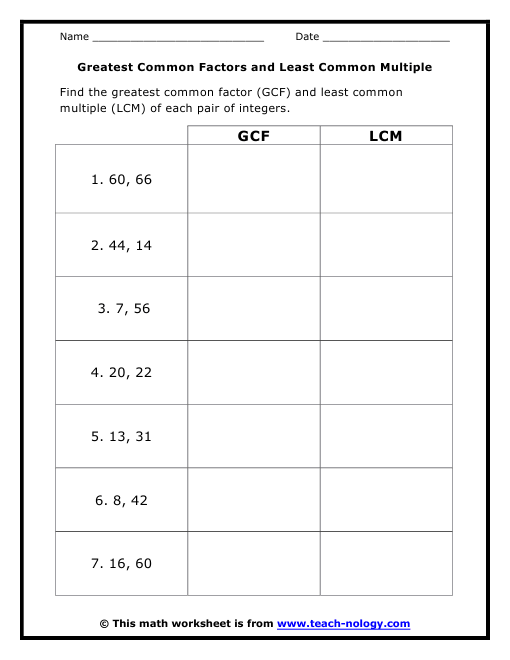
LCM Practice Worksheet

Are you a math student looking to strengthen your understanding of the concept of least common multiple (LCM)? If so, you've come to the right place. In this blog post, we will introduce you to a helpful LCM practice worksheet that will allow you to reinforce your skills in finding the LCM of different numbers. Whether you are preparing for an upcoming test or simply seeking extra practice, this worksheet is designed to provide you with the necessary practice to master the LCM concept.

  GCF and Factor Tree Worksheets

---

  GCF and LCM Worksheets

---

  Least Common Multiple Worksheets

---

  Greatest Common Factor 6th Grade Math Worksheet

---

  Greatest Common Factor Worksheets 6th Grade

---

  Least Common Multiple Worksheets

---

  Practice GCF and LCM Worksheets

---

  GCF and LCM Word Problems Worksheets

---

  Least Common Multiple Worksheets

---

  GCF and LCM Word Problems Worksheets

---

  Least Common Multiple Worksheets

---

  GCF and LCM Word Problems Worksheets

---

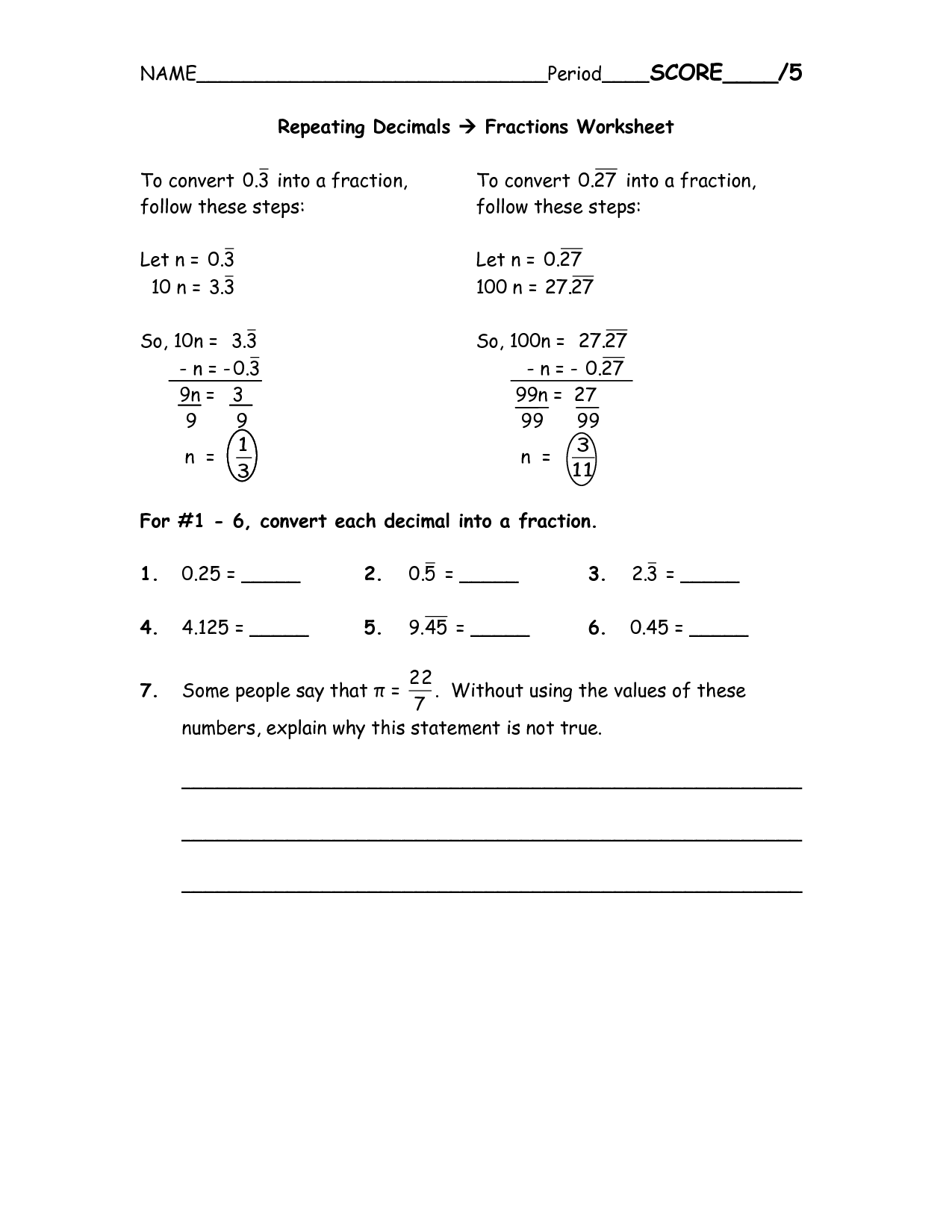
What is the definition of LCM?

The least common multiple (LCM) of two or more numbers is the smallest number that is a multiple of all the given numbers.

How do you find the LCM of two or more numbers?

To find the Least Common Multiple (LCM) of two or more numbers, you first list the prime factors of each number. Then, for each prime factor that appears in any of the numbers, take the highest power of that factor that appears in any one of the numbers. Finally, multiply these prime factors together to get the LCM of the numbers.

Can the LCM of two numbers be smaller than either of the two numbers?

No, the least common multiple (LCM) of two numbers cannot be smaller than either of the two numbers. The LCM is always a multiple of both numbers, so it will be equal to or greater than the largest of the two numbers.

Is the LCM always a multiple of both numbers?

Yes, the Least Common Multiple (LCM) of two numbers is always a multiple of both of those numbers as it is the smallest number that is divisible by both of those numbers without leaving a remainder.

Can you find the LCM of more than two numbers?

Yes, you can find the least common multiple (LCM) of more than two numbers by following a similar process of finding the LCM of two numbers. Simply continue to find the LCM of the result with the next number until all numbers are included.

Is the LCM affected by the order in which you list the numbers?

No, the least common multiple (LCM) is not affected by the order in which you list the numbers. The LCM is a property of the numbers themselves and is determined by finding the smallest number that is a multiple of all the numbers given, regardless of their order.

Can the LCM be zero?

No, the LCM (Least Common Multiple) of two or more numbers cannot be zero. The LCM is always a positive integer, even if the numbers being compared are negative.

Can you use prime factorization to find the LCM?

Yes, prime factorization can be used to find the Least Common Multiple (LCM) of numbers by multiplying the highest power of each prime factor that appears in either of the numbers. This method involves breaking down each number into its prime factors and then selecting the highest power of each prime factor to calculate the LCM. By doing this, you can efficiently determine the LCM of multiple numbers.

Is the LCM related to the concept of least common denominator?

Yes, the LCM (least common multiple) is related to the concept of least common denominator. In fractions, the least common denominator is the lowest common multiple of the denominators, which is the same as finding the LCM of the denominators. This common denominator allows fractions to be added, subtracted, or compared easily, making calculations simpler and more efficient.

How is the LCM useful in real-life situations?

The least common multiple (LCM) is useful in real-life situations to determine when two or more periodic events will align or repeat at the same time. For example, if one person paints a room every 4 days and another person paints it every 6 days, the LCM of 4 and 6 (which is 12) tells us that they will both need to paint the room on the 12th day and every 12 days after that for their schedules to align. This concept is also helpful in fields such as scheduling, manufacturing, and finance to efficiently plan and coordinate activities that occur at regular intervals.