Intersecting Lines Worksheet

If you're in need of an engaging and informative resource to help your students grasp the concept of intersecting lines, you've come to the right place. Our intersecting lines worksheet provides a focused and comprehensive set of exercises to reinforce their understanding of this fundamental geometry topic.

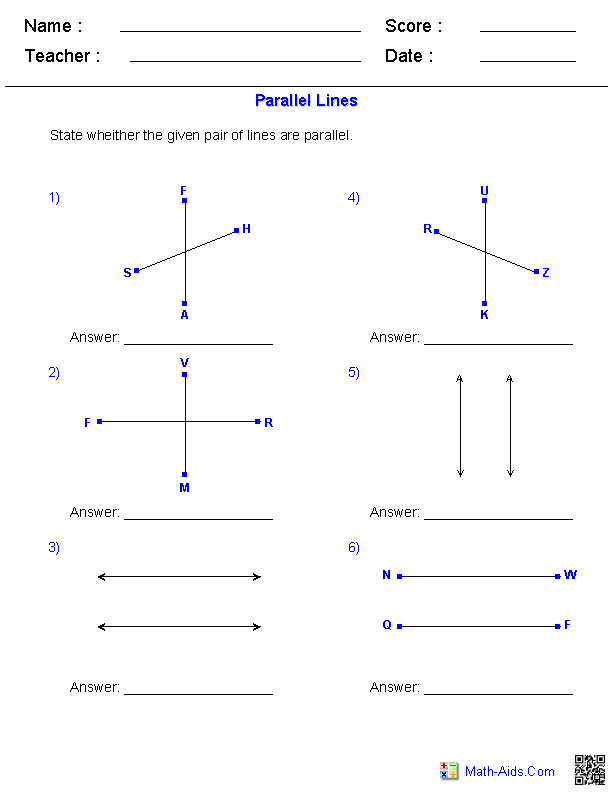
  Parallel Lines Worksheet

---

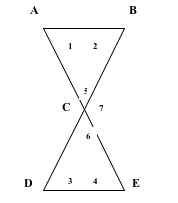
  Triangle with Vertical Angles

---

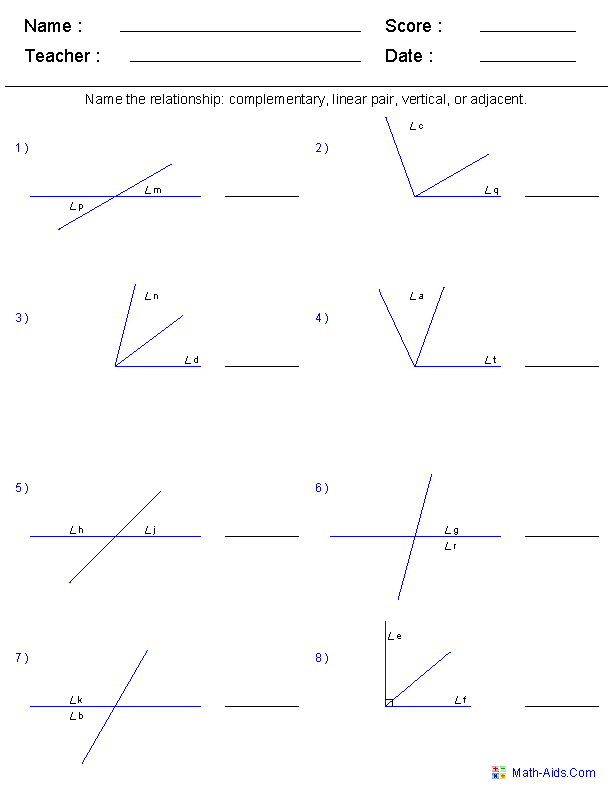
  Angle Pair Relationships Worksheet

---

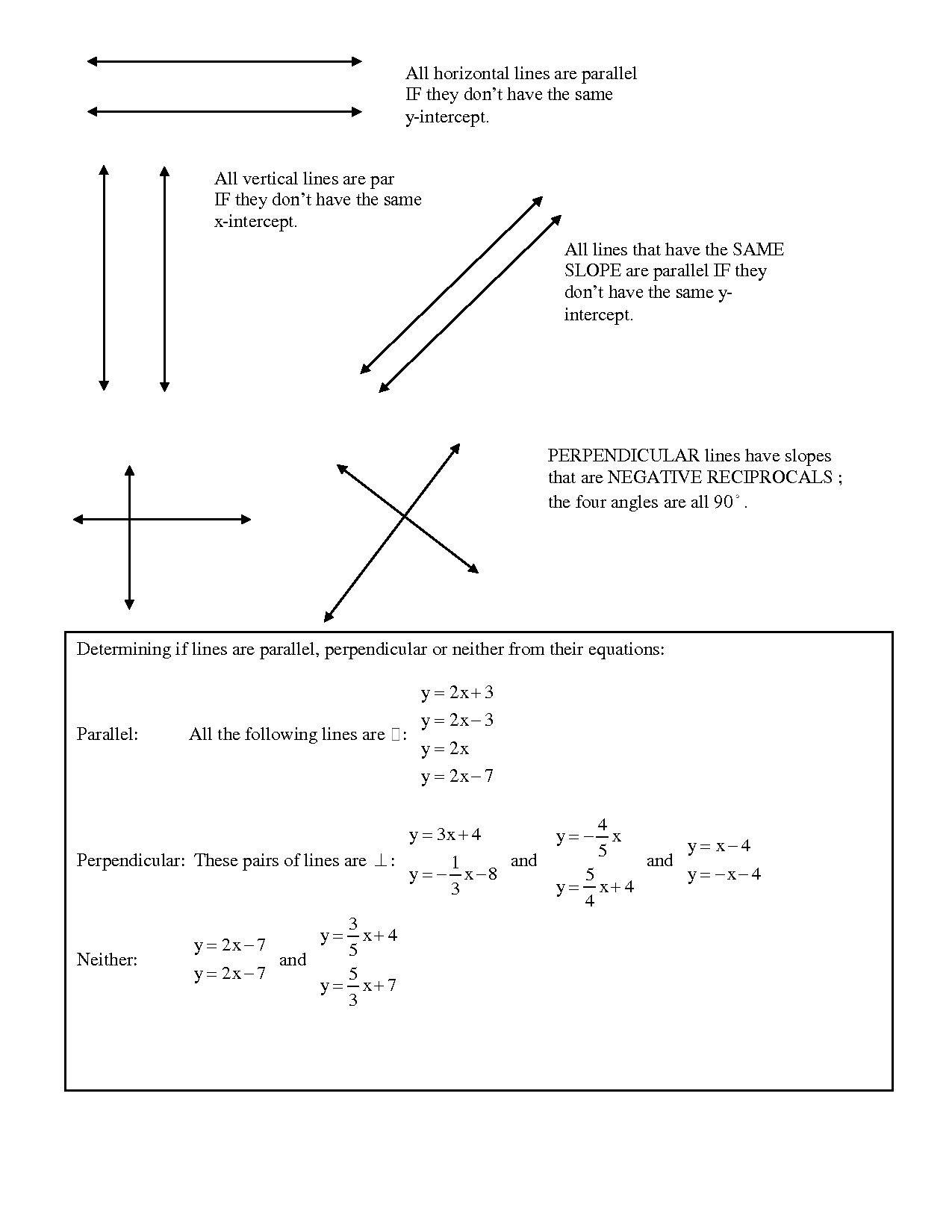
  Parallel Perpendicular Lines Worksheet

---

  Lines Angles Shapes Worksheet

---

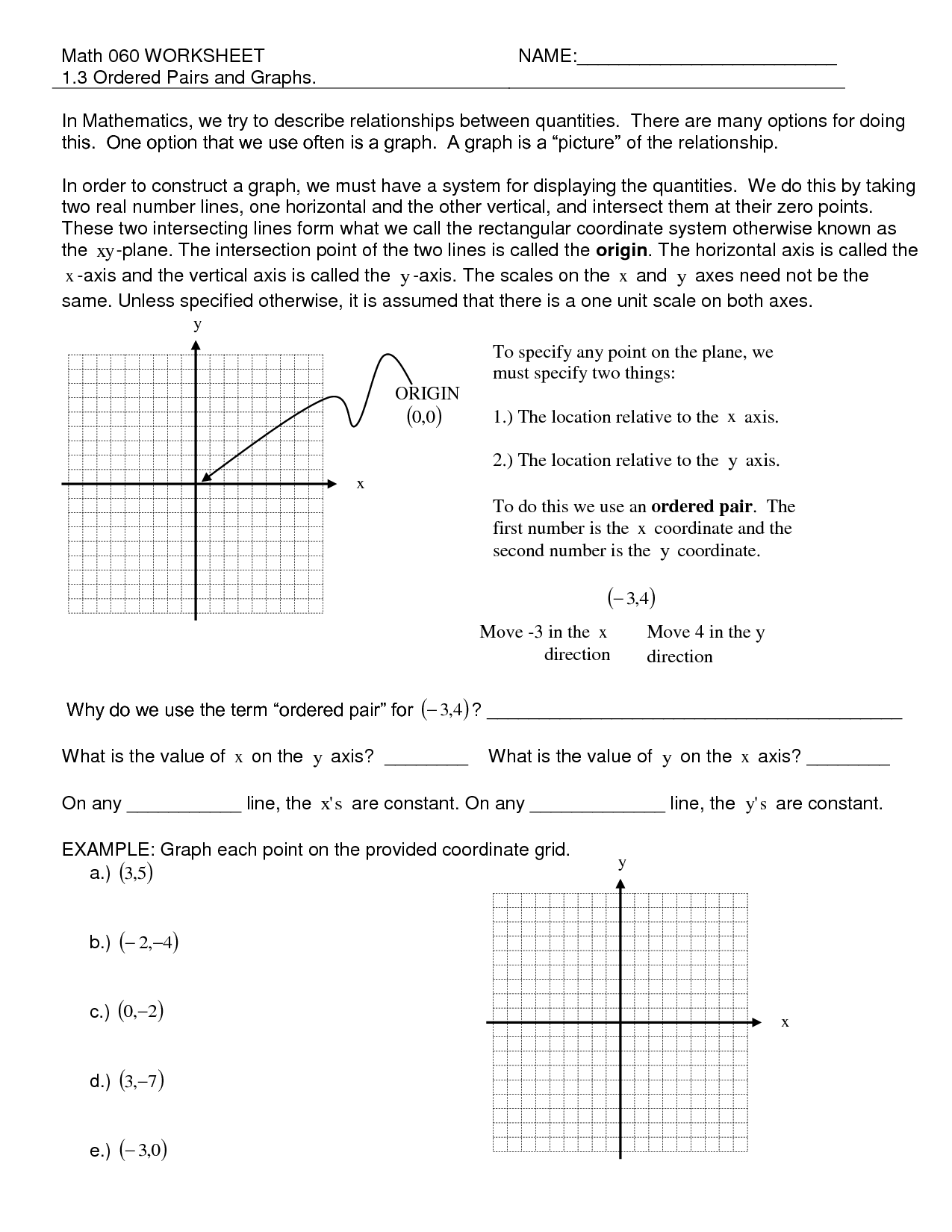
  Graphing Ordered Pairs Worksheets

---

  Venn Diagram Science Wonder Art

---

  Factoring Trinomials Worksheet

---

  Instrument Cubism Art Lesson

---

  Mathematics Formulas PDF

---

  Mathematics Formulas PDF

---

  Mathematics Formulas PDF

---

  Mathematics Formulas PDF

---

What is the intersection point of two lines that intersect each other?

The intersection point of two lines that intersect each other is a unique point where the two lines meet and cross each other. This point is the solution to the system of equations formed by the two lines and represents the coordinates where the two lines coincide in space.

How many intersection points can two lines have?

Two lines can have either 0 intersection points (if they are parallel and never meet) or 1 intersection point (if they are not parallel and intersect at one point).

If two lines are parallel, do they intersect?

No, parallel lines do not intersect. They run alongside each other in the same direction and will never cross paths.

Can two lines that lie on the same plane be non-intersecting?

Yes, two lines that lie on the same plane can be non-intersecting if they are parallel to each other. It is possible for two non-parallel lines on the same plane to intersect, but parallel lines on the same plane will never intersect.

What is the relationship between the slopes of two lines that are perpendicular to each other?

In mathematics, the relationship between the slopes of two lines that are perpendicular to each other is that their slopes are negative reciprocals of each other. This means that if the slope of one line is m, the slope of the other line will be -1/m. This is a fundamental property of perpendicular lines in geometry.

How can you find the equation of a line that passes through a given point and is perpendicular to another line?

To find the equation of a line that passes through a given point and is perpendicular to another line, first determine the slope of the given line by taking the negative reciprocal of its slope. Next, use the point-slope form of a linear equation (y - y₁ = m(x - x₁)), where (x₁, y₁) is the given point and m is the slope calculated earlier, to write the equation of the perpendicular line passing through the given point.

What is the relationship between the slopes of two lines that are parallel?

The slopes of two parallel lines are identical. This means that if two lines are parallel, they have the same steepness or incline, no matter where they are located on a graph. Parallel lines will never intersect and will run side by side in the same direction.

How do you determine if three lines intersect at a common point?

To determine if three lines intersect at a common point, you can set up a system of equations representing the equations of the three lines. Solve the system of equations to find the point of intersection. If the three lines intersect at a common point, the system of equations will have a unique solution, indicating the point where the lines intersect. If the system of equations has no solution or infinite solutions, then the lines do not intersect at a common point.

Can two lines that are not parallel or intersecting have the same slope?

Yes, two non-parallel and non-intersecting lines can have the same slope if they are coincident lines, meaning they lie on top of each other. Coincident lines have the same slope because they share all the points. In this case, even though the lines do not intersect, their slopes are equal because they are essentially the same line.

In a two-dimensional coordinate plane, how do you determine if two lines are intersecting or non-intersecting based on their equations?

To determine if two lines are intersecting or non-intersecting based on their equations on a two-dimensional coordinate plane, you can find the point of intersection by solving the system of equations. If the system has a unique solution, the lines intersect at that point. If the system has no solution, the lines are parallel and non-intersecting. And if the system has infinitely many solutions, the lines are coincident or overlapping.