Homework Polynomials Worksheet

Polynomials can be a challenging topic for students to grasp, but with the help of a well-designed worksheet, tackling homework assignments can become much easier. Whether you're a high school student struggling with factoring polynomials or a college student navigating complex polynomial functions, having access to a comprehensive and engaging worksheet is essential. With carefully crafted questions that address the key concepts and provide ample practice, these worksheets can be a valuable tool to reinforce your understanding and improve your performance in polynomials.

  Algebra 2 Factoring Polynomials Worksheet with Answers

---

  Factoring Polynomials Worksheet Puzzle

---

  Factoring Perfect Cubes Worksheet

---

  Factoring Polynomials Cheat Sheet

---

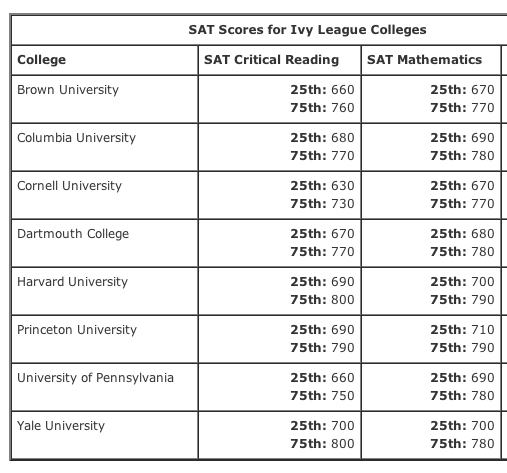
  Quadratic Functions Worksheet

---

  Kuta Software Infinite Algebra 1 Answers Key

---

  6 1 Skills Practice Operations On Functions

---

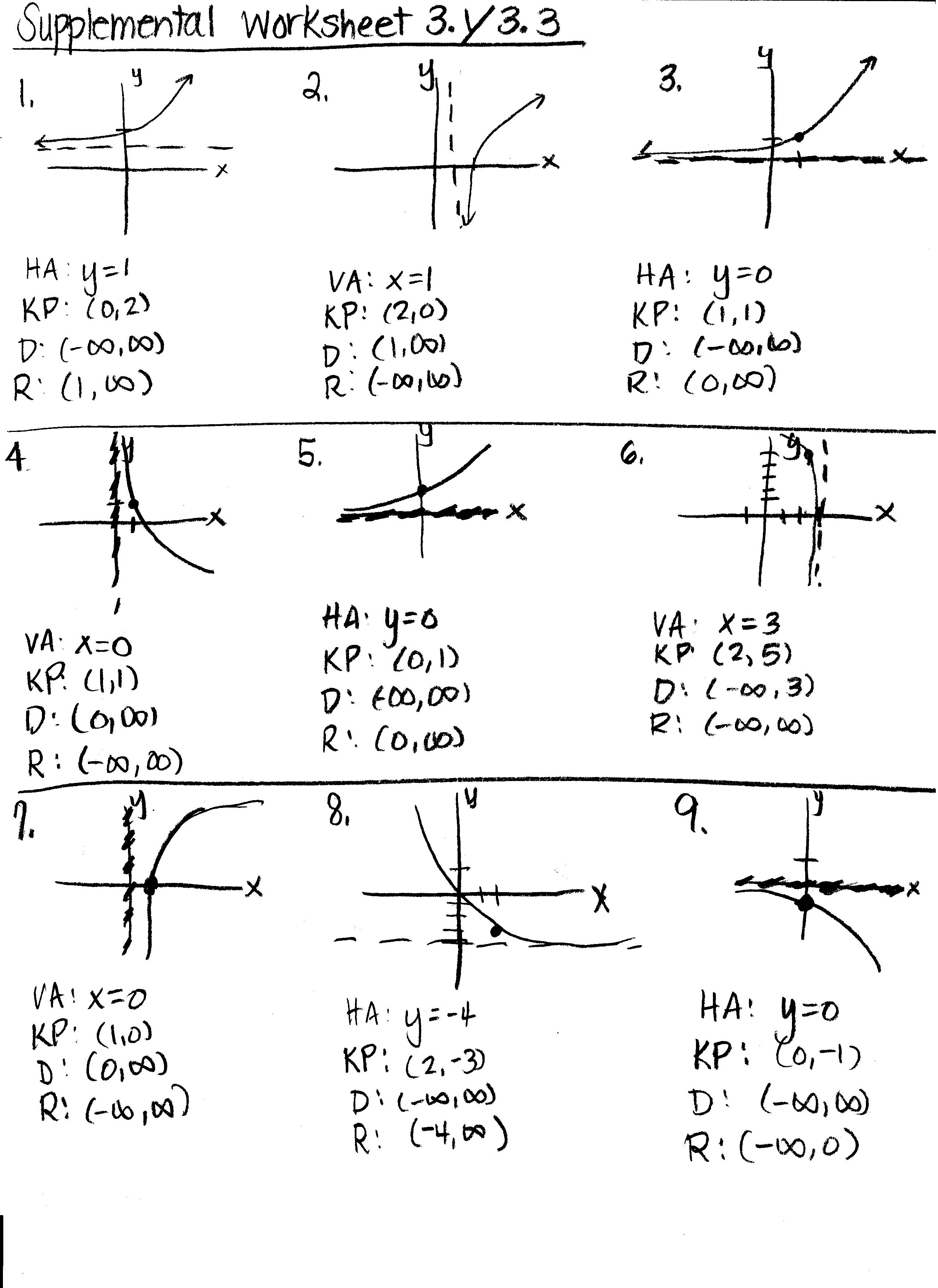
  Exponential Functions Worksheet Answer Key

---

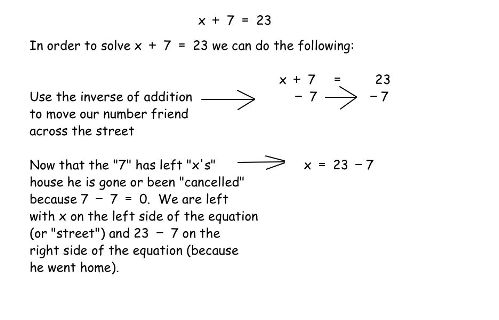
  Two-Step Equation Word Problems Worksheets

---

  Simplifying Radicals Algebra 1 Review

---

  Kuta Software Infinite Algebra 1 Answers Key

---

  Subtracting Fractions Worksheets

---

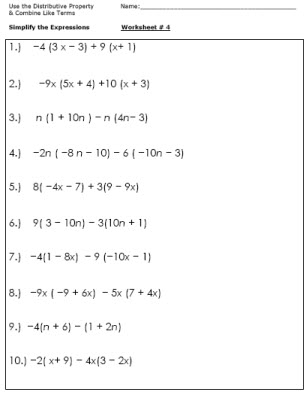
  Distributive Property and Combining Like Terms Worksheet

---

  High School Math Worksheets

---

  High School Math Worksheets

---

What is a polynomial?

A polynomial is a mathematical expression that consists of variables, constants, and coefficients combined using addition, subtraction, and multiplication operations, but not division. It can have one or more terms, where each term consists of a coefficient multiplied by one or multiple variables raised to non-negative integer exponents.

How do you identify the degree of a polynomial?

To identify the degree of a polynomial, you look at the term with the highest exponent on the variable. The degree of a polynomial is the highest exponent among all the terms. For example, if the highest exponent is 3 in a polynomial, then the degree of that polynomial is 3.

How do you add or subtract polynomials?

To add or subtract polynomials, you simply combine like terms by adding or subtracting coefficients of terms with the same variables and exponents. Start by aligning like terms, then perform the operations indicated. Remember to be careful with signs and ensure that you are combining terms with the same variables and exponents. Make sure to simplify the result by combining any like terms further if possible.

How do you multiply polynomials?

To multiply polynomials, you need to distribute each term in the first polynomial with each term in the second polynomial, and then combine like terms. This involves following the distributive property and multiplying all terms together. After multiplying each pair of terms, you then simplify the resulting expression by combining like terms and arranging them in descending order of exponents. Keep in mind the rules of multiplying different types of terms, such as monomials, binomials, and trinomials, to correctly multiply polynomials.

How do you divide polynomials using long division?

To divide polynomials using long division, first, write the dividend (numerator) inside the division symbol and the divisor (denominator) outside. Divide the first term of the dividend by the first term of the divisor to determine the first term of the quotient. Multiply the divisor by this term and subtract it from the dividend. Bring down the next term of the dividend and repeat the process until all terms in the dividend have been accounted for. The final result will be the quotient, with a remainder if the degree of the dividend is not less than the degree of the divisor.

What is the remainder when dividing polynomials using synthetic division?

When dividing polynomials using synthetic division, the remainder is the constant term that is left after fully dividing the polynomial. This remainder represents the leftover value that could not be evenly divided by the divisor polynomial.

How do you factor polynomials using the greatest common factor (GCF)?

To factor a polynomial using the greatest common factor (GCF), you first identify the largest common factor of all the terms in the polynomial. Then, you divide each term by this common factor to rewrite the polynomial as a product of the GCF and a new expression. This new expression is the factored form of the polynomial. By factoring out the GCF, you simplify the polynomial and make it easier to work with in further calculations or to find its roots.

How do you factor trinomials using the product-sum method?

To factor trinomials using the product-sum method, first identify the coefficients of the trinomial in the form ax^2 + bx + c. Then find two numbers that multiply to a*c (the product of the coefficients) and add up to b (the middle coefficient). Use these two numbers to rewrite the middle term of the trinomial. From there, factor by grouping or apply the difference of squares method if possible.

How do you solve polynomial equations using factoring?

To solve polynomial equations using factoring, you need to first factor the polynomial into its linear factors. Once you have factored the polynomial, set each factor equal to zero and solve for the variable. The solutions you obtain will be the roots of the equation. It's important to remember to check your solutions by substituting them back into the original equation to ensure they are valid.

How do you solve polynomial equations using the quadratic formula?

To solve a polynomial equation using the quadratic formula, first write the equation in standard form, Ax^2 + Bx + C = 0. Then, identify the values of A, B, and C. Next, substitute these values into the quadratic formula: x = (-B ± ?(B^2 - 4AC)) / 2A. Simplify the formula by computing the discriminant (B^2 - 4AC) to determine the number of solutions: two real solutions if the discriminant is positive, one real solution if the discriminant is zero, or two complex solutions if the discriminant is negative. Finally, calculate the solutions by plugging the values back into the formula and simplifying.