Greatest Common Factor Factor Tree Worksheets

Are you in search of effective and engaging educational resources to teach your students about the concept of greatest common factor? Look no further! Our comprehensive collection of greatest common factor factor tree worksheets is the perfect solution for you. Designed for educators and parents who want to enhance their students' understanding of this fundamental concept, these worksheets provide an interactive and hands-on approach to learning. By using factor trees, students can easily visualize and identify the greatest common factors of given numbers, making the learning process both enjoyable and effective.

  7th Grade Math Worksheets

---

  8th Grade Math Worksheets Geometry

---

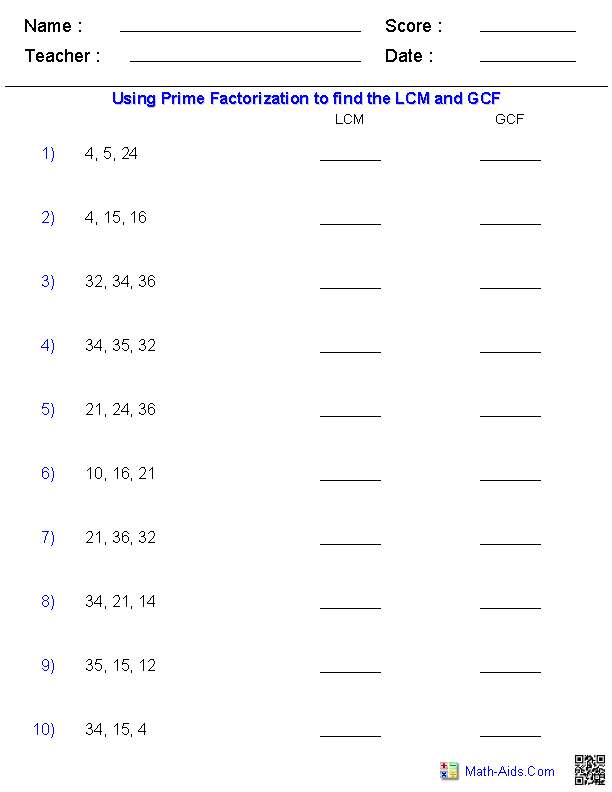
  Prime Factorization Worksheets Factor Trees

---

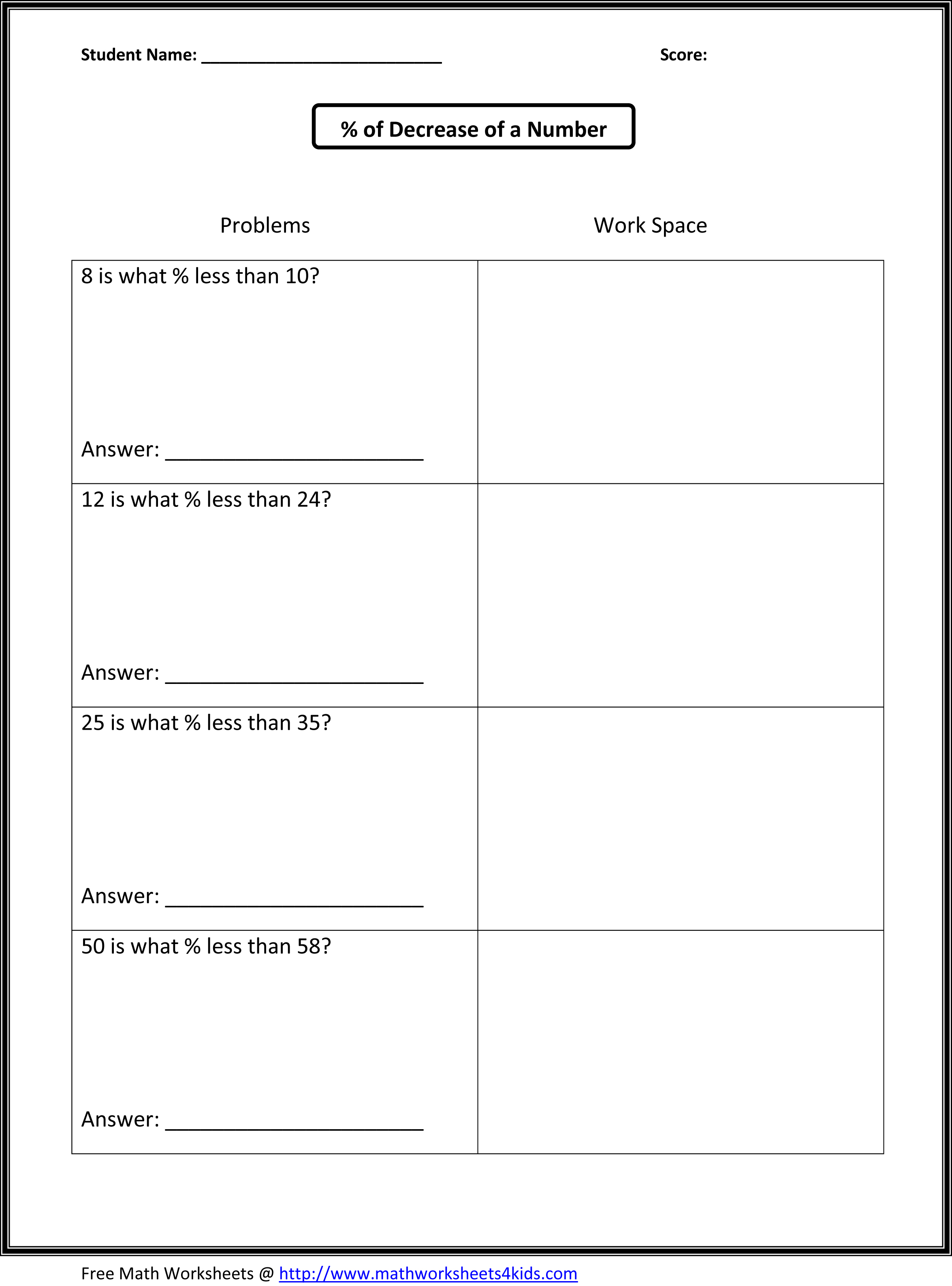
  Greatest Common Factor 6th Grade Math Worksheet

---

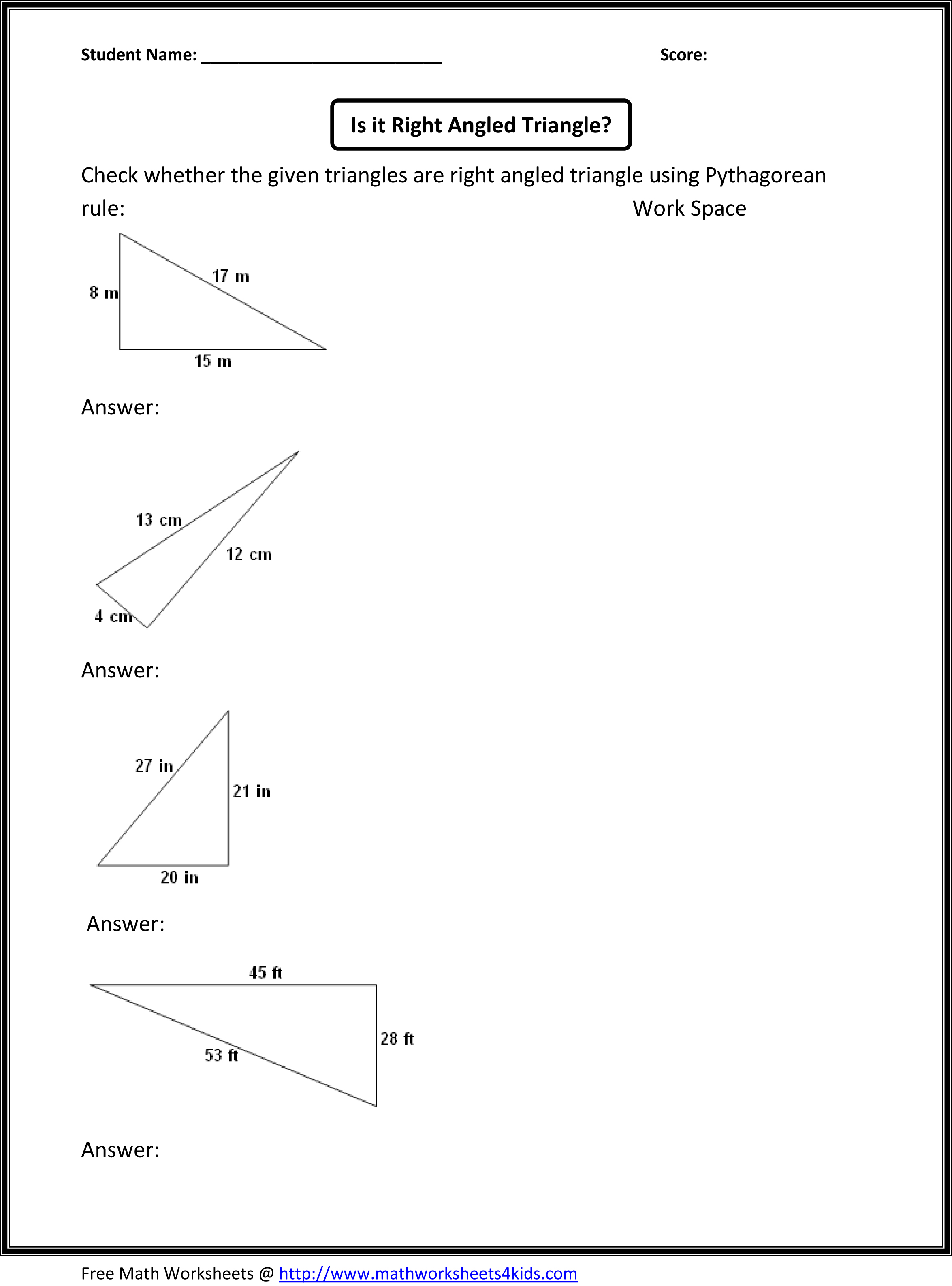
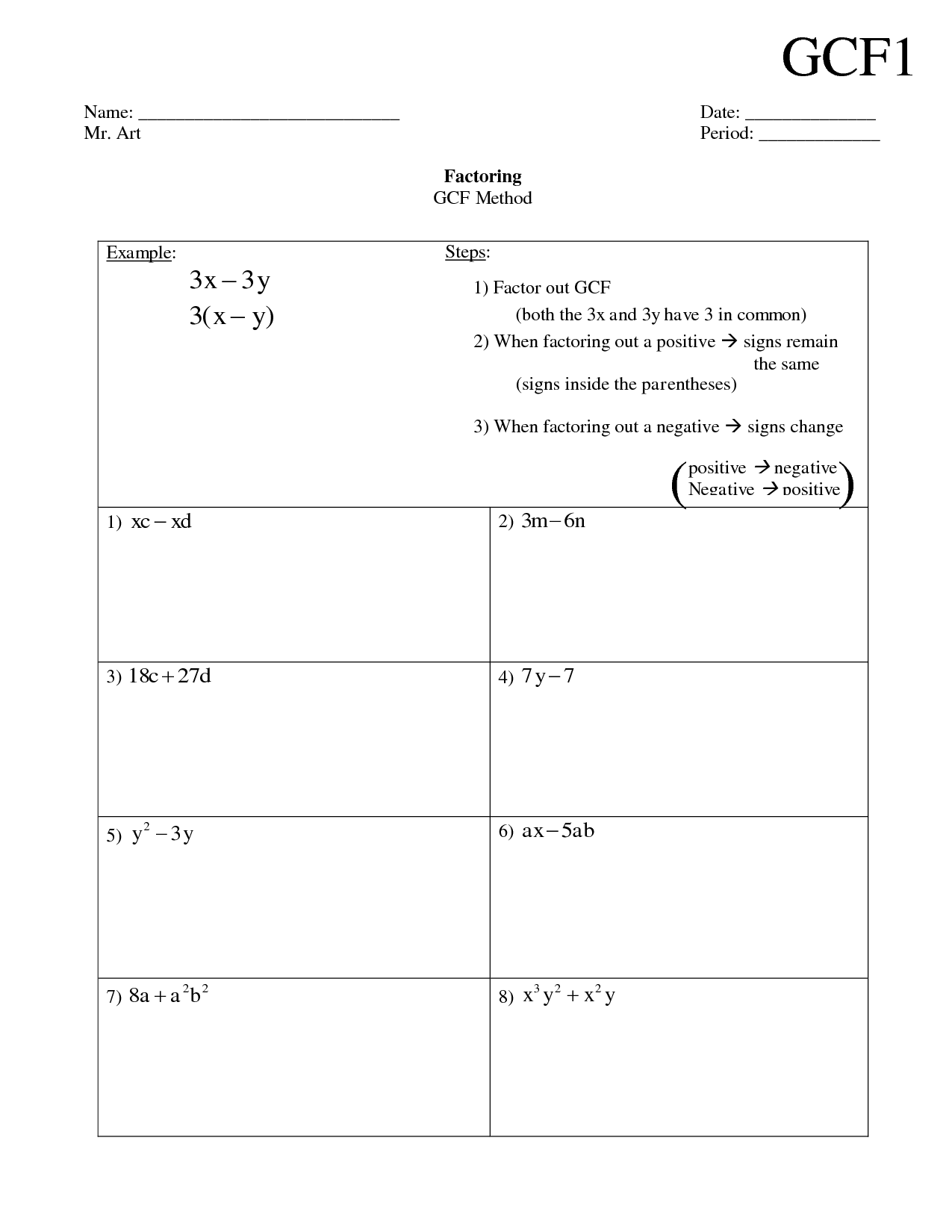
  Factoring Greatest Common Factor Worksheet

---

  Greatest Common Factor Worksheet 5th Grade Math

---

  Factor Tree Worksheets Printable

---

  Factoring Trinomials Worksheets with Answers

---

What is a factor tree?

A factor tree is a diagram used to break down a number into its prime factors by repeatedly factoring out any prime factors until only prime numbers are left. The process starts with the given number at the top of the tree, then branches out into factors that are further factored until reaching prime numbers at the bottom of the tree. This method helps in understanding the prime factorization of a number.

How does a factor tree help find the prime factors of a number?

A factor tree helps find the prime factors of a number by breaking down the number into its prime factors through a series of branches. Starting with the number at the top of the tree, it is divided into two factors. Each of those factors is then further broken down into their factors, and this process continues until only prime numbers are left at the bottom of the tree. The prime numbers at the bottom are the prime factors of the original number, making it easier to identify and understand all its prime factors.

What is the purpose of finding the prime factors of a number?

The purpose of finding the prime factors of a number is to break down the number into its simplest components, enabling us to understand its fundamental building blocks. Prime factorization helps in various mathematical calculations, such as finding the greatest common divisor, simplifying fractions, and solving equations. It is also essential in cryptography and number theory, providing insights into the properties and behavior of numbers. Additionally, prime factorization is used in computer science for data compression and encryption algorithms.

How can a factor tree be used to find the greatest common factor (GCF) of two or more numbers?

To find the greatest common factor (GCF) of two or more numbers using a factor tree, you start by breaking down each number into its prime factors using a factor tree. Then, you identify the common prime factors shared by all the numbers and multiply them together to get the GCF. The GCF will be the product of the common prime factors with the lowest exponent for each factor.

What is the definition of the greatest common factor (GCF)?

The greatest common factor (GCF) of two or more numbers is the largest number that divides each of the numbers without leaving a remainder.

Why is finding the GCF important in mathematics?

Finding the greatest common factor (GCF) is important in mathematics because it helps simplify fractions and expressions, make calculations easier, and identify common factors within numbers. It is a fundamental concept that is used in various mathematical operations such as simplifying fractions, reducing polynomials, and solving equations. Understanding the GCF allows for a deeper comprehension of mathematical relationships and simplifies complex problems by breaking them down into smaller, more manageable components.

Can a factor tree be used to find the GCF of more than two numbers?

Yes, a factor tree can be used to find the greatest common factor (GCF) of more than two numbers. By breaking down each number into its prime factors and identifying the common factors among the numbers, the GCF can be determined by finding the product of the common prime factors. This method allows for an efficient way to find the GCF of multiple numbers using a factor tree approach.

Is the GCF always a prime number?

No, the Greatest Common Factor (GCF) is not always a prime number. The GCF of two or more numbers is simply the largest number that divides each of the numbers without leaving a remainder. While the GCF can be a prime number in some cases, it can also be a composite number that is not prime, depending on the numbers being considered.

Can the GCF be larger or smaller than the given numbers?

No, the greatest common factor (GCF) of two numbers cannot be larger than the numbers themselves. The GCF is always a positive integer that divides both numbers without a remainder, so it is always smaller than or equal to the given numbers.

Are there any specific steps or rules to follow when using factor trees to find the GCF?

Yes, there are specific steps to follow when using factor trees to find the greatest common factor (GCF). First, you need to list all the factors of each number and then draw a factor tree for each number, breaking down the numbers into their prime factors. Next, identify the common prime factors in the factor trees of all the numbers and multiply them together to find the GCF. Repeat this process until no more common factors can be found. Finally, multiply the common prime factors to determine the GCF.