Graphing Systems of Equations Worksheet Answers
Are you a math enthusiast or a student looking for practice materials to enhance your understanding of graphing systems of equations? If so, you're in the right place! In this blog post, we will provide you with a variety of graphing systems of equations worksheet answers. These worksheets are designed to help you solidify your understanding of this mathematical concept and improve your problem-solving skills. Let's dive in and explore the world of graphing systems of equations together!
Table of Images 👆
- Graphing Lines Kuta Software Infinite Algebra 1 Answers Key
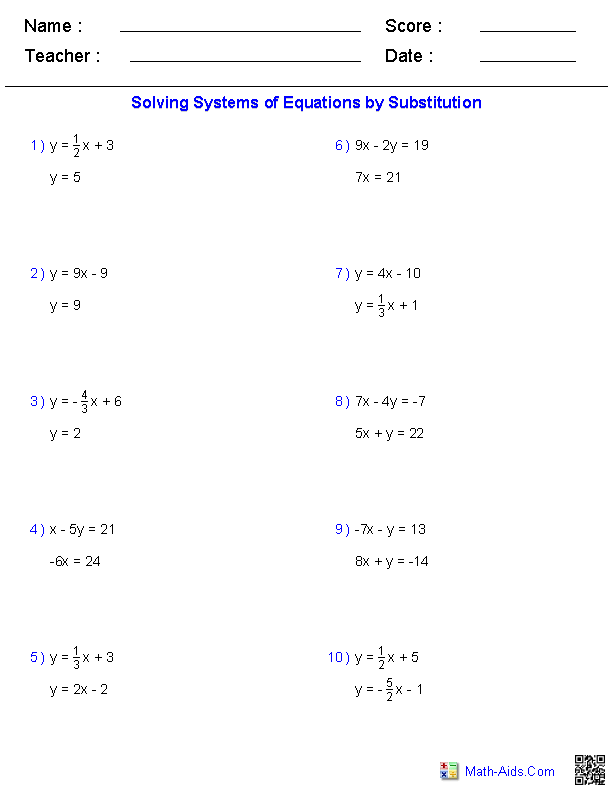
- Systems of Equations Kuta Software Infinite Algebra 1
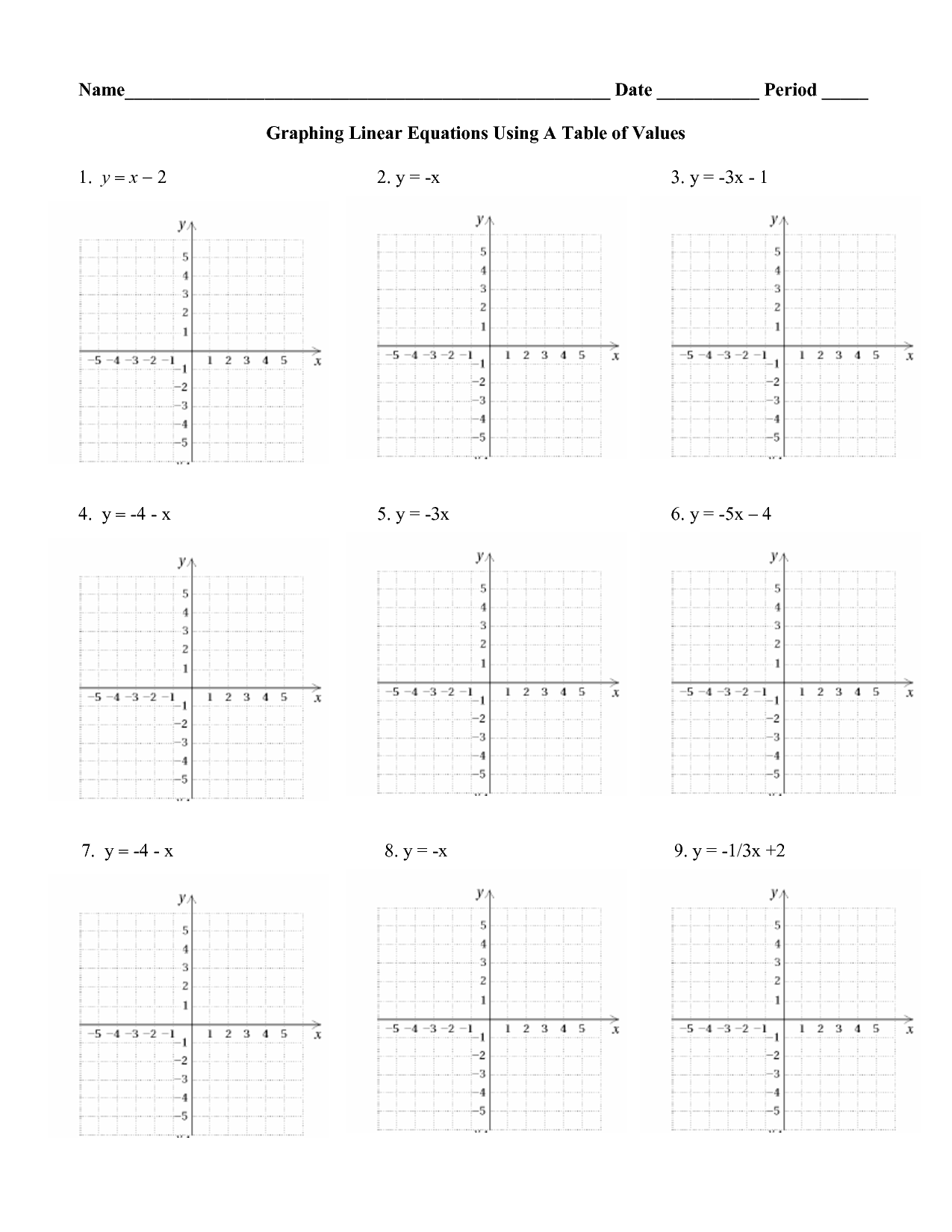
- Graphing Linear Equations Tables Worksheet
- Math Equations Pre-Algebra Worksheets
- 7th Grade Math Algebra Equations Worksheets
- Systems of Equations Point-Slope Form
- Set Up Function Notation
- Algebra Equations Word Problems Worksheets
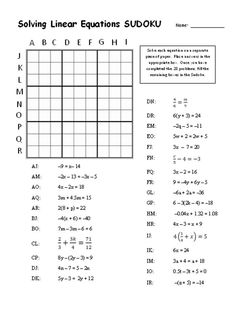
- Sudoku Solving Linear Equations
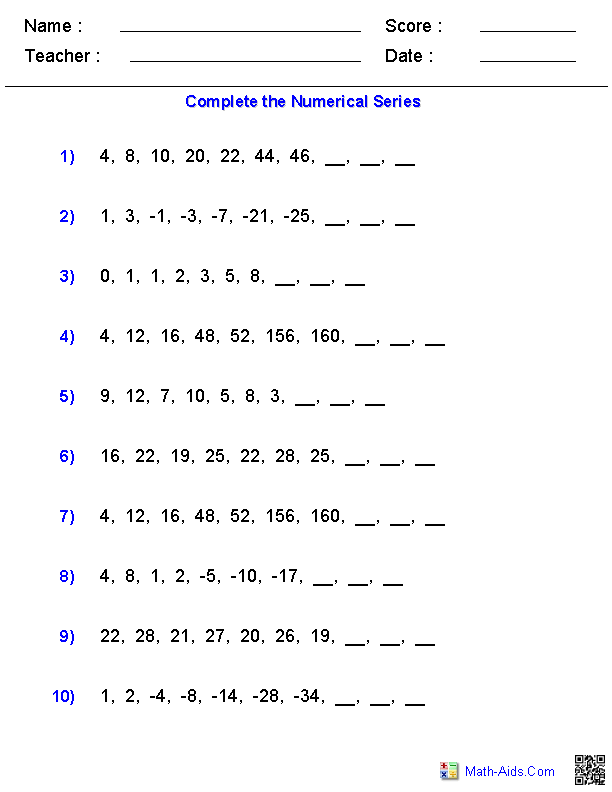
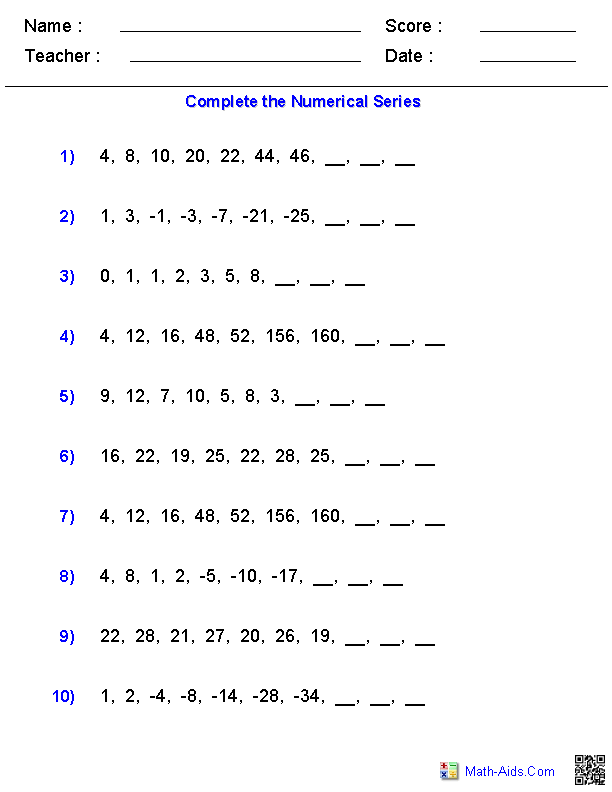
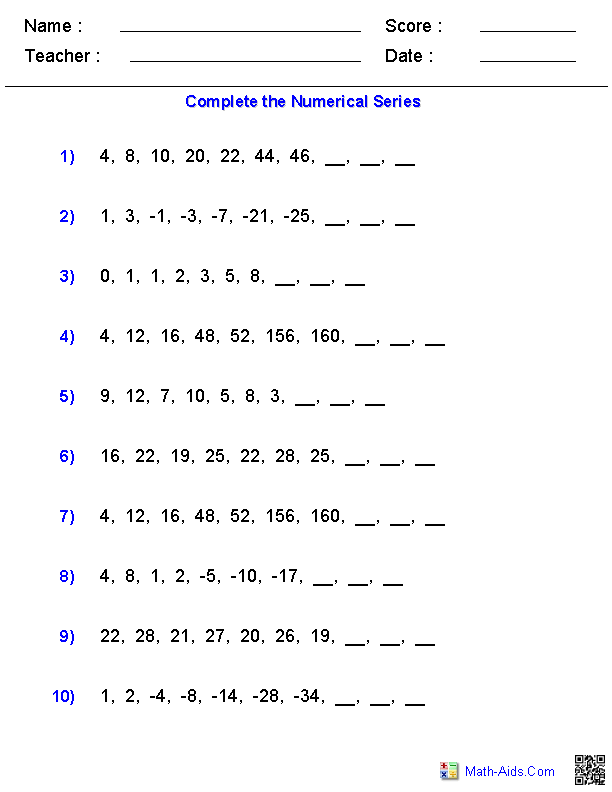
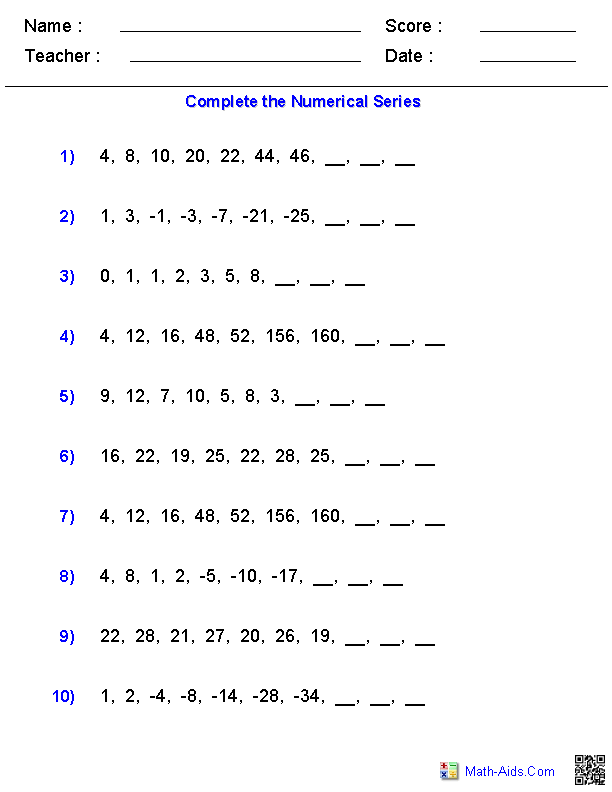
- Number Patterns Worksheets 3rd Grade Math
- Number Patterns Worksheets 3rd Grade Math
- Number Patterns Worksheets 3rd Grade Math
- Number Patterns Worksheets 3rd Grade Math
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
What is the purpose of graphing systems of equations?
The purpose of graphing systems of equations is to visually represent the relationship between two or more equations in order to find the points where they intersect, which represent the solutions to the system. Graphing provides a clear and intuitive way to understand the solutions and how the different equations relate to each other geometrically. It can also help in identifying patterns, understanding the behavior of the system, and can be used as a helpful tool for problem-solving and analysis in mathematics and other fields.
How many solutions can a system of equations have when graphed?
A system of equations can have either one unique solution, no solution (inconsistent system), or infinite solutions (dependent system) when graphed, depending on the relationship between the equations and their graphs.
What does it mean if two lines in a system of equations are parallel?
If two lines in a system of equations are parallel, it means that they have the same slope and will never intersect, no matter how far they are extended. In other words, they are always at a constant distance from each other and will continue to run parallel indefinitely.
What does it mean if two lines in a system of equations intersect at a single point?
When two lines in a system of equations intersect at a single point, it means that this point represents the unique solution to the system of equations. In other words, the values of the variables that satisfy both equations simultaneously can be found at this point of intersection.
What does it mean if two lines in a system of equations overlap each other?
If two lines in a system of equations overlap each other, it means that they represent the same line. This indicates that the system of equations has infinitely many solutions, as any point on that particular line would satisfy both equations simultaneously.
How can you determine the solution to a system of equations by graphing?
You can determine the solution to a system of equations by graphing by plotting the equations on a coordinate plane and identifying the point where the graphs intersect. This point represents the solution where the values of variables satisfy both equations simultaneously. If the graphs do not intersect, it indicates that the system has no solution.
How can you determine if a system of equations has no solution when graphed?
If a system of equations has no solution when graphed, it means the lines representing the equations are parallel and do not intersect at any point. This can be visually observed by graphing the equations and seeing that the lines are parallel and never cross each other. In such cases, the system of equations is inconsistent, and there is no common solution that satisfies all the equations simultaneously.
How can you determine if a system of equations has infinitely many solutions when graphed?
A system of equations has infinitely many solutions when the graphs of the equations coincide with each other, meaning they are essentially the same line when plotted on a coordinate plane. This indicates that the equations are dependent and represent the same relationship, leading to an infinite number of solutions as any point along the line satisfies both equations simultaneously.
What does the x-coordinate of the point of intersection represent in a system of equations?
In a system of equations, the x-coordinate of the point of intersection represents the value where the two equations intersect on the x-axis, indicating the specific point where the x-values of both equations are equal and satisfy both equations simultaneously.
What does the y-coordinate of the point of intersection represent in a system of equations?
The y-coordinate of the point of intersection in a system of equations represents the value of the common solution that both equations share for the variable y. It is the particular y-value where the two equations intersect each other on a graph, indicating the point where the solutions to both equations are equal in magnitude.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.





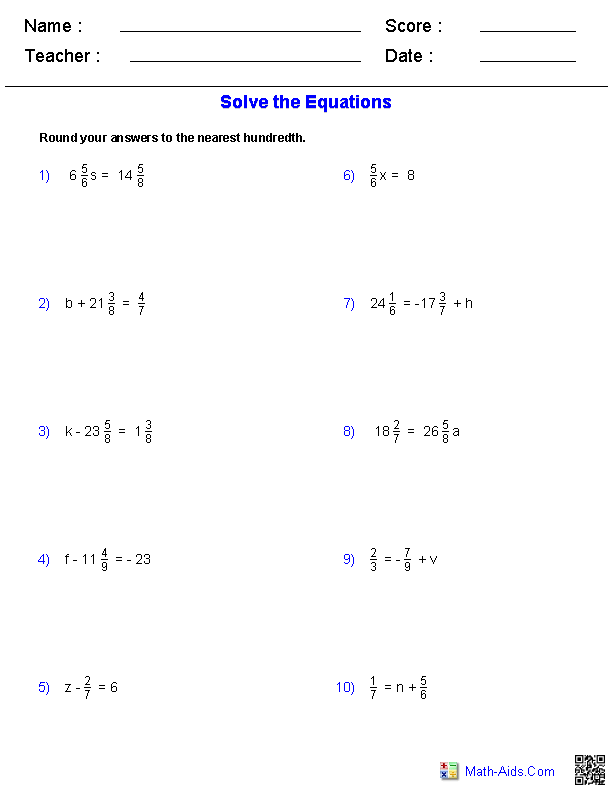
















Comments