Graphing Inequalities Worksheets
Graphing inequalities may seem challenging, but with the right resources, anyone can master this skill. If you are a student or a teacher seeking helpful worksheets to improve your understanding of graphing inequalities, you have come to the right place.
Table of Images 👆
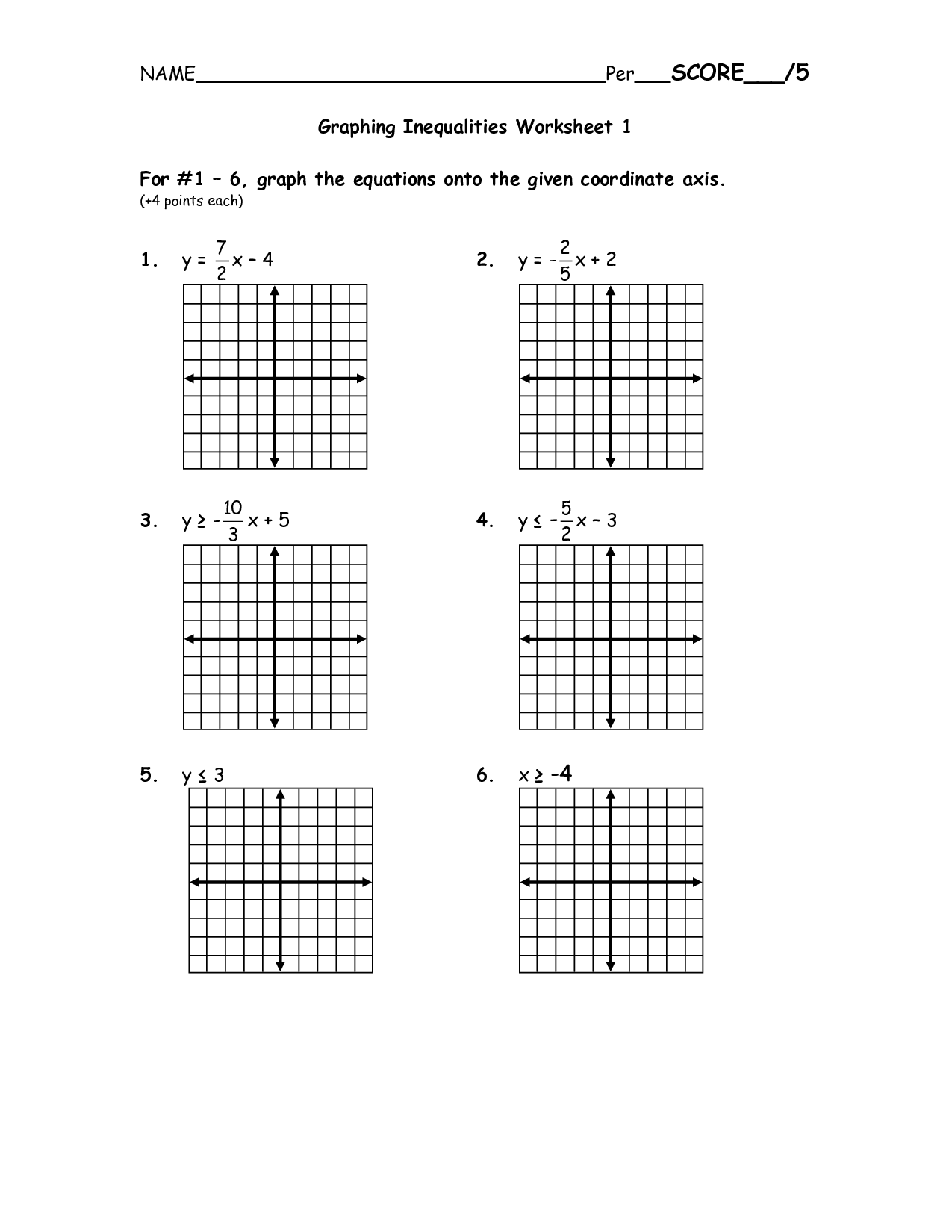
- Graphing Linear Inequalities Worksheet
- Algebra 1 Inequalities Worksheets Printable
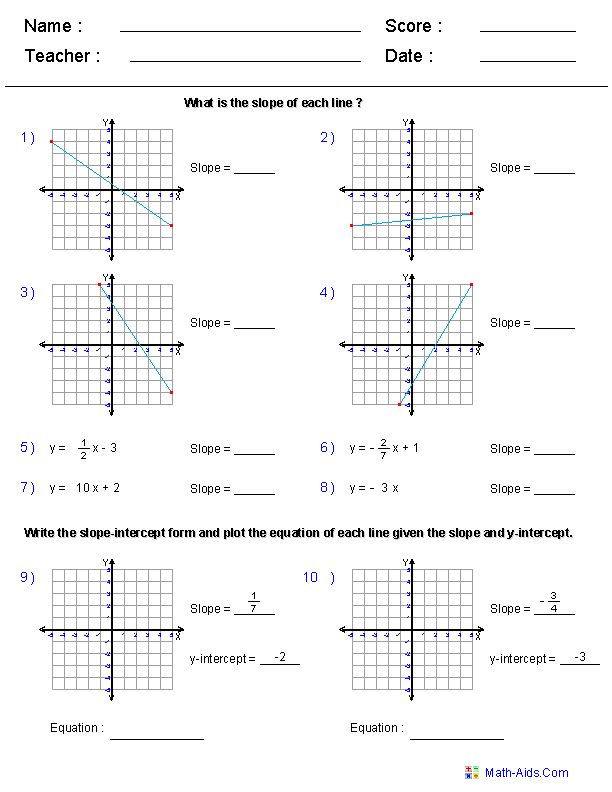
- Graphing Linear Equations Using Intercepts
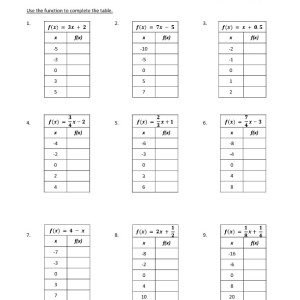
- 8th Grade Function Table Worksheet
- Two-Step Inequality Worksheets
- Number Line Inequalities Worksheet 6th Grade
- Interpreting Graphs Algebra 1 Worksheet
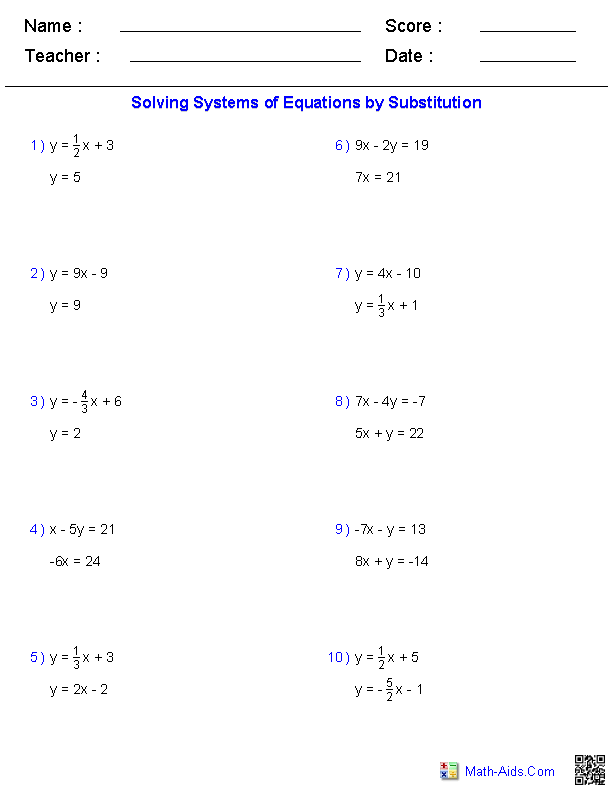
- Multi-Step Inequalities Worksheet
- 7th Grade Math Algebra Equations Worksheets
- Coordinate Plane Worksheets 6th Grade
- Algebra Equations Word Problems Worksheets
- 6th Grade Algebra Equations Worksheets
- Inequality Word Problems Worksheet
- Pre-Algebra Equations Worksheets
- Variables and Expressions Worksheets
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is a graphing inequalities worksheet?
A graphing inequalities worksheet is a document or set of problems that require students to graph solutions to inequalities on a coordinate plane. This type of worksheet typically includes various linear, quadratic, or other types of inequalities and asks students to represent the solution sets graphically, considering shading, intersection points, and boundary lines. It is a common practice in mathematics education to help students understand and visualize the relationships between variables and how they relate to each other in terms of inequalities.
How are inequalities represented on a graph?
Inequalities can be represented on a graph by shading areas of the coordinate plane that satisfy or do not satisfy the inequality. For linear inequalities, the boundary line can be drawn as a dashed or solid line and the region above or below the line can be shaded to represent the solutions that satisfy the inequality. The type of shading (solid or dotted) depends on whether the boundary line is included in the solution set or not.
What are the different symbols used in graphing inequalities?
The different symbols used in graphing inequalities include less than (<), greater than (>), less than or equal to (≤), greater than or equal to (≥), not equal to (≠), and the conjunction "and" (∧) or "or" (∨) to represent compound inequalities. These symbols are used to show the relationship between two quantities or expressions on a number line or coordinate plane, indicating where solutions lie within a range of values.
How do you determine the solution to an inequality from its graph?
To determine the solution to an inequality from its graph, you look at the shaded region on the graph. If the shaded region includes a solid line, it means the boundary is included in the solution. If the shaded region does not include the boundary line, it means the boundary is not part of the solution. Points in the shaded region are solutions to the inequality, while points outside the shaded region are not.
What is the purpose of graphing inequalities?
The purpose of graphing inequalities is to visually represent the solutions to inequality statements on a coordinate plane. By graphing inequalities, you can easily identify regions that satisfy the inequality and make it easier to understand the relationship between variables and constraints. Additionally, graphing inequalities helps in problem-solving, decision-making, and analyzing mathematical and real-world situations that involve inequalities.
How can graphing inequalities help in solving real-life problems?
Graphing inequalities can help in solving real-life problems by visually representing the possible solutions and variations in a clear and easy-to-understand manner. By graphing inequalities, one can quickly identify the feasible range of values that satisfy the given conditions, making it easier to make decisions or find solutions in scenarios such as budgeting, resource allocation, optimization problems, and decision-making processes. This visual representation helps in analyzing and interpreting data, identifying patterns, and making informed choices based on the constraints and requirements of a particular situation.
What are the key steps in graphing inequalities on a coordinate plane?
To graph inequalities on a coordinate plane, first, plot the boundary line as if it were a strict equation by replacing the inequality sign with an equal sign. Determine if the line is solid (≤ or ≥) or dashed (< or >). Next, choose a test point not on the line to determine which side of the boundary (shaded or unshaded) contains solutions. Shade the appropriate side and include the line if it is solid or omit if it is dashed. Finally, label the shaded area to represent the solution set of the inequality.
How do you shade the solution region on a graph of an inequality?
To shade the solution region on a graph of an inequality, first graph the boundary line of the inequality. Then, select a point not on the boundary line to test if it satisfies the inequality. If the point does satisfy the inequality, shade the region that contains that point. If not, shade the region that does not contain that point. This shaded region indicates the solution region for the inequality on the graph.
What happens when an inequality has a greater than or less than symbol with a line underneath?
When an inequality has a greater than or less than symbol with a line underneath, it means that the inequality includes the value of that symbol as well, creating a "greater than or equal to" or "less than or equal to" relationship. This symbol represents that the value on the line underneath is part of the solution set for the inequality.
What are some common mistakes to avoid when graphing inequalities?
Some common mistakes to avoid when graphing inequalities include not correctly identifying the inequality symbol (">" or "<"), incorrectly shading the area above or below the line representing the inequality, forgetting to include dashed or solid lines to indicate strict inequality versus non-strict inequality, and not properly labeling the axes and regions to indicate the solution set. It is essential to pay attention to these details to accurately represent the solution to the inequality on the graph.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.




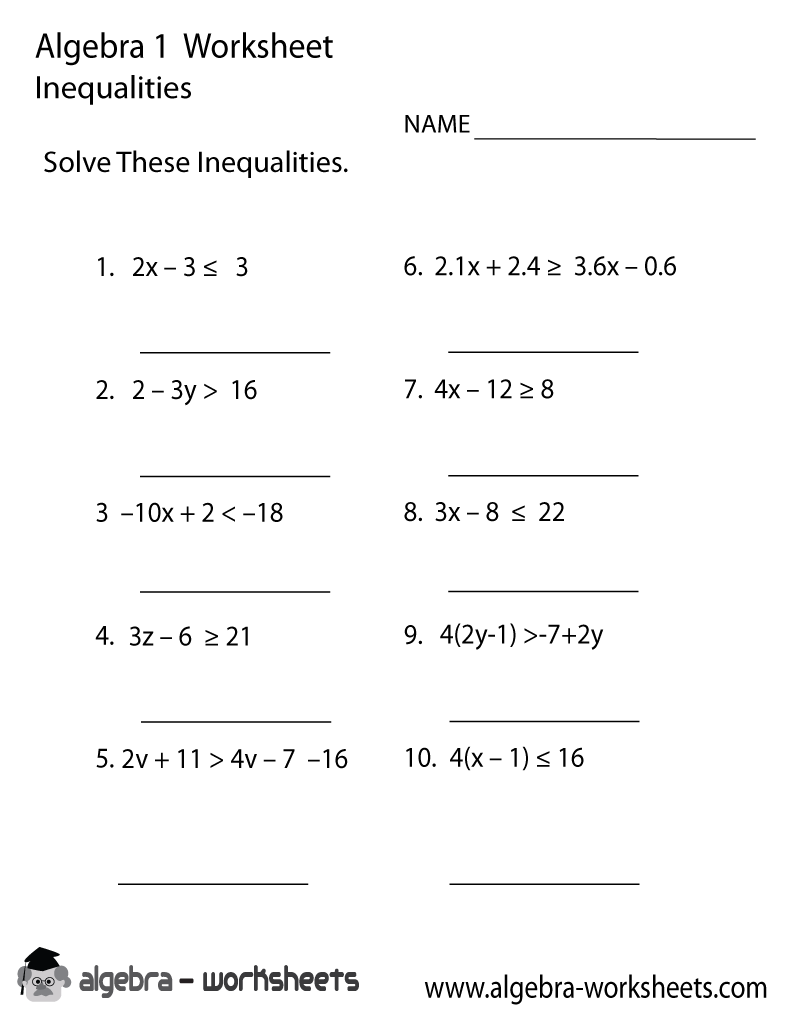






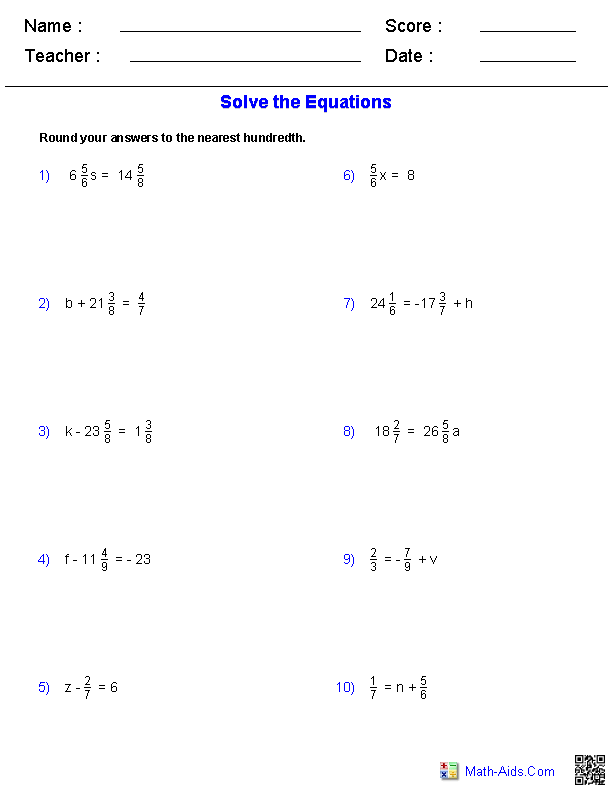















Comments