Fun Scientific Notation Worksheet

Do you want to strengthen your understanding of scientific notation? Look no further than this fun and engaging scientific notation worksheet! Designed for students in middle or high school, this worksheet provides a variety of questions aimed at reinforcing the concept of scientific notation.

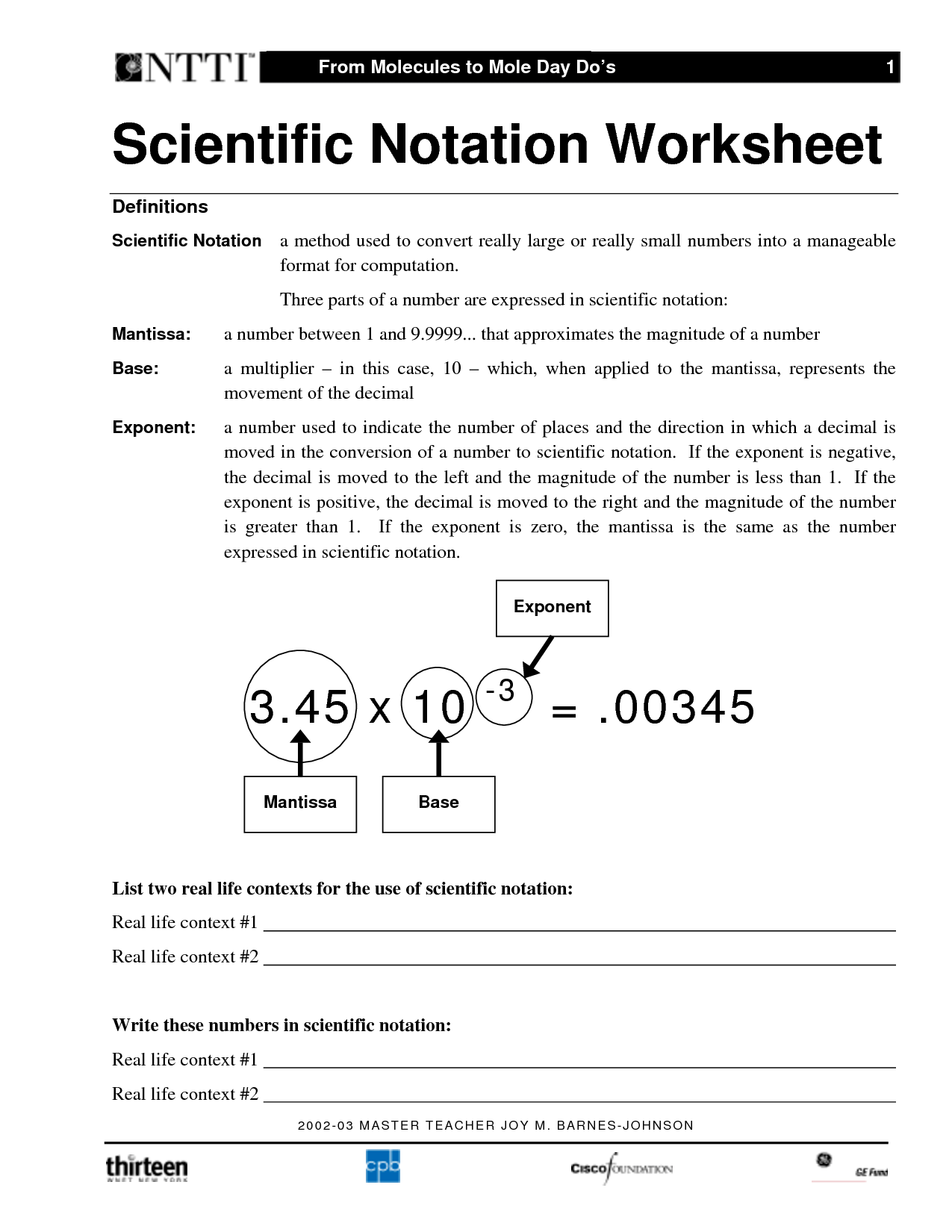
  Scientific Notation Worksheet

---

  Scientific Notation Worksheet

---

  Scientific Notation Worksheet

---

  Scientific Notation Worksheet

---

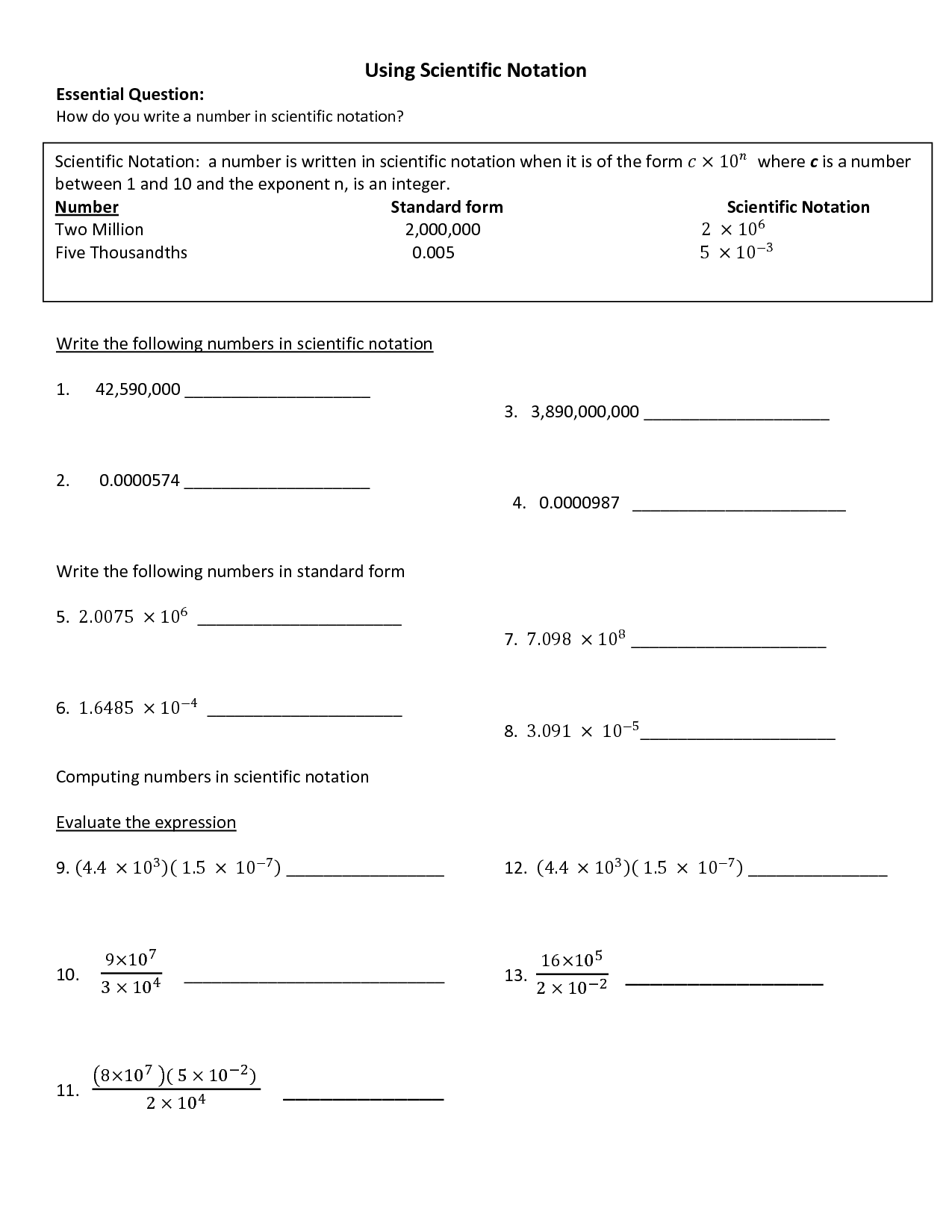
  Scientific Notation Worksheets with Answers

---

  Scientific Notation Worksheets with Answers

---

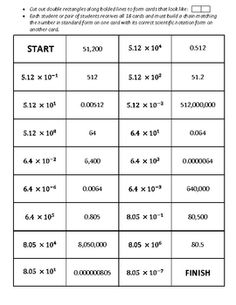
  Scientific Notation Worksheet Answers

---

  Scientific Notation Worksheet Answers

---

  Orbital Notation

---

  6th Grade Math Worksheets Mean Median Mode

---

  Multiplying and Dividing Numbers in Scientific Notation

---

What is scientific notation?

Scientific notation is a way of writing large or small numbers in a more concise and manageable format using powers of 10. It consists of two parts: a coefficient (a number typically between 1 and 10) and an exponent of 10 that indicates how many places the decimal point was moved. This notation is commonly used in scientific and mathematical calculations to represent very large or very small values efficiently.

How is scientific notation used in scientific calculations?

Scientific notation is used in scientific calculations to represent very large or very small numbers more efficiently. By expressing numbers as a coefficient multiplied by a power of 10, scientists can easily perform calculations involving extremely large or small values, reducing the likelihood of errors and making the numbers more manageable to work with. Additionally, scientific notation allows for easier comparison of different magnitudes of numbers, facilitating data analysis and interpretation in scientific research.

What are the advantages of using scientific notation?

Scientific notation allows for easy expression of very large or very small numbers by representing them as a decimal number between 1 and 10 multiplied by a power of 10. This makes it easier to perform calculations, compare numbers, and understand the scale of measurements in scientific fields. It also helps to avoid writing out many zeros, improving clarity and reducing the chance of errors.

How is a number expressed in scientific notation?

A number is expressed in scientific notation by writing it as the product of a decimal number (greater than or equal to 1, but less than 10) and a power of 10. For example, the number 6,500,000 would be written in scientific notation as 6.5 x 10^6. The number 0.00025 would be written as 2.5 x 10^-4.

How is a number converted from scientific notation to standard form?

To convert a number from scientific notation to standard form, you multiply the coefficient (the decimal part of the number) by 10 raised to the power of the exponent (the number that indicates where the decimal point should be moved). For example, if you have a number in scientific notation as 5.2 x 10^3, you would multiply 5.2 by 10^3 to get 5200 in standard form.

How is a number rounded in scientific notation?

In scientific notation, a number is rounded by adjusting the coefficient (the part before the ×10^n) to the correct number of significant figures, and then adjusting the exponent accordingly to reflect the magnitude of the number. The coefficient is rounded to the desired number of significant figures, and the exponent is adjusted accordingly so that the value remains the same.

How is multiplication or division performed on numbers in scientific notation?

To perform multiplication or division on numbers in scientific notation, you first multiply or divide the numerical parts of the numbers, then add or subtract the exponents. When multiplying, you can simply multiply the coefficients and add the exponents. When dividing, you divide the coefficients and subtract the exponents. The final result should then be expressed in scientific notation by adjusting the coefficient and exponent accordingly.

How is addition or subtraction performed on numbers in scientific notation?

To add or subtract numbers in scientific notation, the exponents of the numbers being added or subtracted must be the same. Adjust the numbers so that they have the same power of 10 by moving the decimal point to the right or left accordingly. Then, perform the addition or subtraction of the coefficients while keeping the exponent the same. Lastly, normalize the result by converting it back to scientific notation if needed.

How is scientific notation used in representing very large numbers?

Scientific notation is used in representing very large numbers by expressing them in the form of a coefficient multiplied by 10 raised to a power. This allows for easier understanding and comparison of these large values as the coefficient is between 1 and 10, while the power of 10 indicates the magnitude of the number. For example, the number 6,000,000 can be written as 6 x 10^6 in scientific notation, making it simpler to work with and manipulate such large numbers in calculations and scientific contexts.

How is scientific notation used in representing very small numbers?

Scientific notation is used in representing very small numbers by expressing them in the form of a number between 1 and 10 multiplied by a power of 10. This notation helps simplify the representation of extremely small values like 0.0000000264 by moving the decimal point to the right until there is only one non-zero digit to the left of the decimal point, which can then be expressed as 2.64 x 10^-8. This way, very small numbers can be easily written and understood in a more manageable format.