Fun High School Algebra Worksheets

High school algebra can be a challenging subject for many students. To help make the learning process more enjoyable, engaging, and effective, incorporating fun worksheets into your study routine is a great idea. These worksheets are specifically designed to cater to the needs of high school students, allowing them to practice and reinforce their algebraic skills in an entertaining way.

  High School Math Worksheets Printable

---

  Secret Code Math Page: Use

---

  High School Grade English Worksheets

---

  Algebra 2 Practice Worksheets

---

  High School Geometry Math Worksheets

---

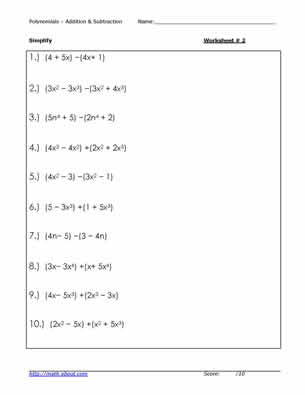
  High School Algebra Worksheets

---

  High School Algebra 2 Worksheets

---

  Fun Math Worksheets Middle School

---

  High School Math Worksheets

---

  High School Algebra 1 Math Worksheets

---

  Nutrition Worksheets for High School Students

---

What is the value of x in the equation 2x + 5 = 15?

To find the value of x in the equation 2x + 5 = 15, you need to isolate x by first subtracting 5 from both sides to get 2x = 10, and then dividing both sides by 2 to get x = 5. Therefore, the value of x in the equation is 5.

Solve the quadratic equation x^2 - 9 = 0.

To solve the quadratic equation x^2 - 9 = 0, we can factor it as (x - 3)(x + 3) = 0. Setting each factor to zero, we find that x = 3 and x = -3 are the solutions to the equation.

Simplify the expression 4x + 3y - 2x + 7y.

The simplified expression is 2x + 10y.

Find the slope of the line that passes through points (-3, 4) and (2, -1).

To find the slope of the line passing through the two points (-3, 4) and (2, -1), we use the formula: slope = (y2 - y1) / (x2 - x1). Substituting the coordinates into the formula, we get slope = (-1 - 4) / (2 - (-3)) = -5 / 5 = -1. Therefore, the slope of the line passing through the two points is -1.

Factorize the expression x^2 + 6x + 9.

The expression x^2 + 6x + 9 can be factorized into (x + 3)(x + 3) or (x + 3)^2.

Solve the system of equations: 3x + y = 10, 2x - 3y = 5.

By solving the system of equations 3x + y = 10 and 2x - 3y = 5 simultaneously, we can find that the solution is x = 3 and y = 1.

Determine the domain and range of the function f(x) = 2x + 1.

The domain of the function f(x) = 2x + 1 is all real numbers since there are no restrictions on the values x can take. The range of the function is all real numbers as well, because as x varies, the output of the function can be any real number depending on the input.

Simplify the expression (2x - 5)^2.

To simplify the expression (2x - 5)^2, you need to expand it by multiplying (2x - 5) with itself. This gives you (2x - 5)(2x - 5), which simplifies to 4x^2 - 20x + 25. So, (2x - 5)^2 simplifies to 4x^2 - 20x + 25.

Solve the inequality 3x - 8 < 5.

To solve the inequality 3x - 8 < 5, we first add 8 to both sides to isolate the variable: 3x - 8 + 8 < 5 + 8, which simplifies to 3x < 13. Then, we divide by 3 to solve for x, giving x < 13/3 or x < 4.33. Therefore, the solution to the inequality 3x - 8 < 5 is x < 4.33.

Find the y-intercept of the line given by the equation y = 2x + 3.

To find the y-intercept of the line given by the equation y = 2x + 3, you set x = 0 because the y-intercept occurs where x = 0. So, when x = 0, y = 2(0) + 3 = 3. Therefore, the y-intercept of the line is 3.