Factoring Polynomials Worksheet Generator
Are you a math teacher or a student struggling with factoring polynomials? If so, we have the perfect solution for you - a factoring polynomials worksheet generator. This tool is designed to assist educators in creating customized worksheets that cater to the specific needs of their students, providing ample practice in factoring polynomials. With just a few clicks, you can generate worksheets that focus on different types of polynomials, ensuring a comprehensive understanding of this important mathematical concept.
Table of Images 👆
- Algebra 1 Factoring Problems and Answers
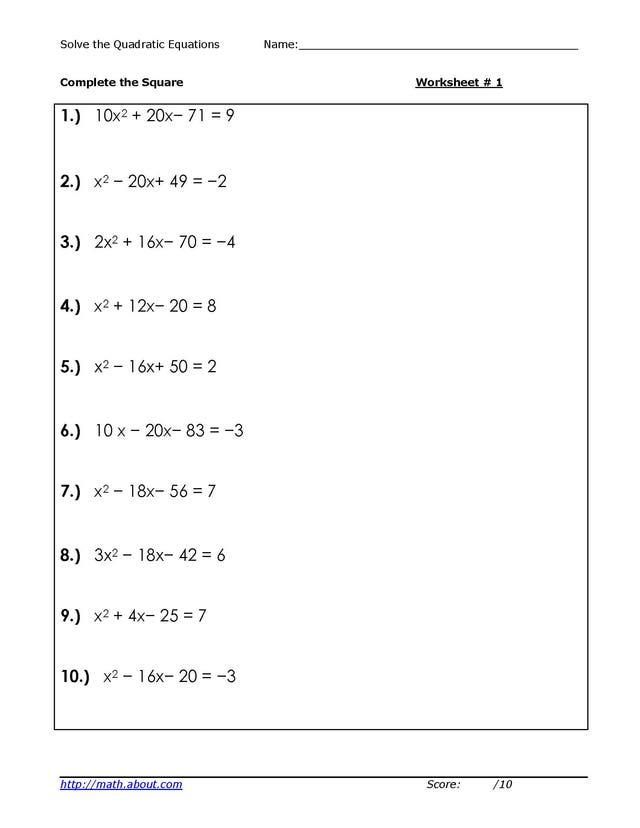
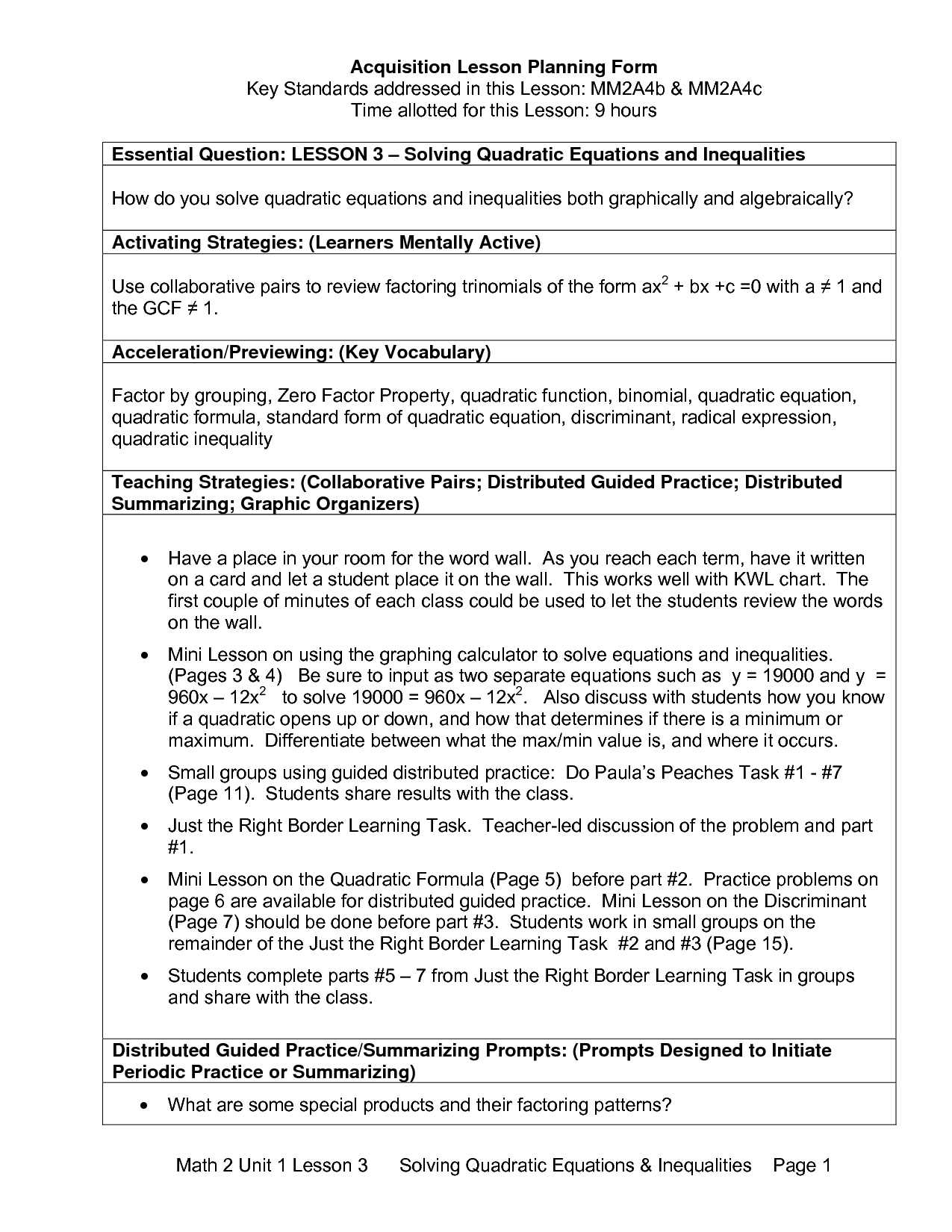
- Solve Quadratic Equations Worksheet
- Algebra Foil Method Worksheets
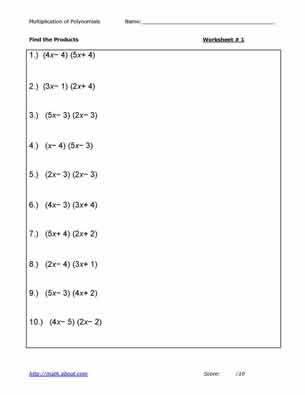
- Algebra 1 Multiplying Polynomials Worksheet
- Factoring Polynomials Worksheet.pdf
- Factoring Polynomials by Grouping Worksheets
- Foil Method Worksheet
- Polynomials in Everyday Life
- Adding and Subtracting Money Worksheets
- Graphing Quadratic Equations Worksheet PDF
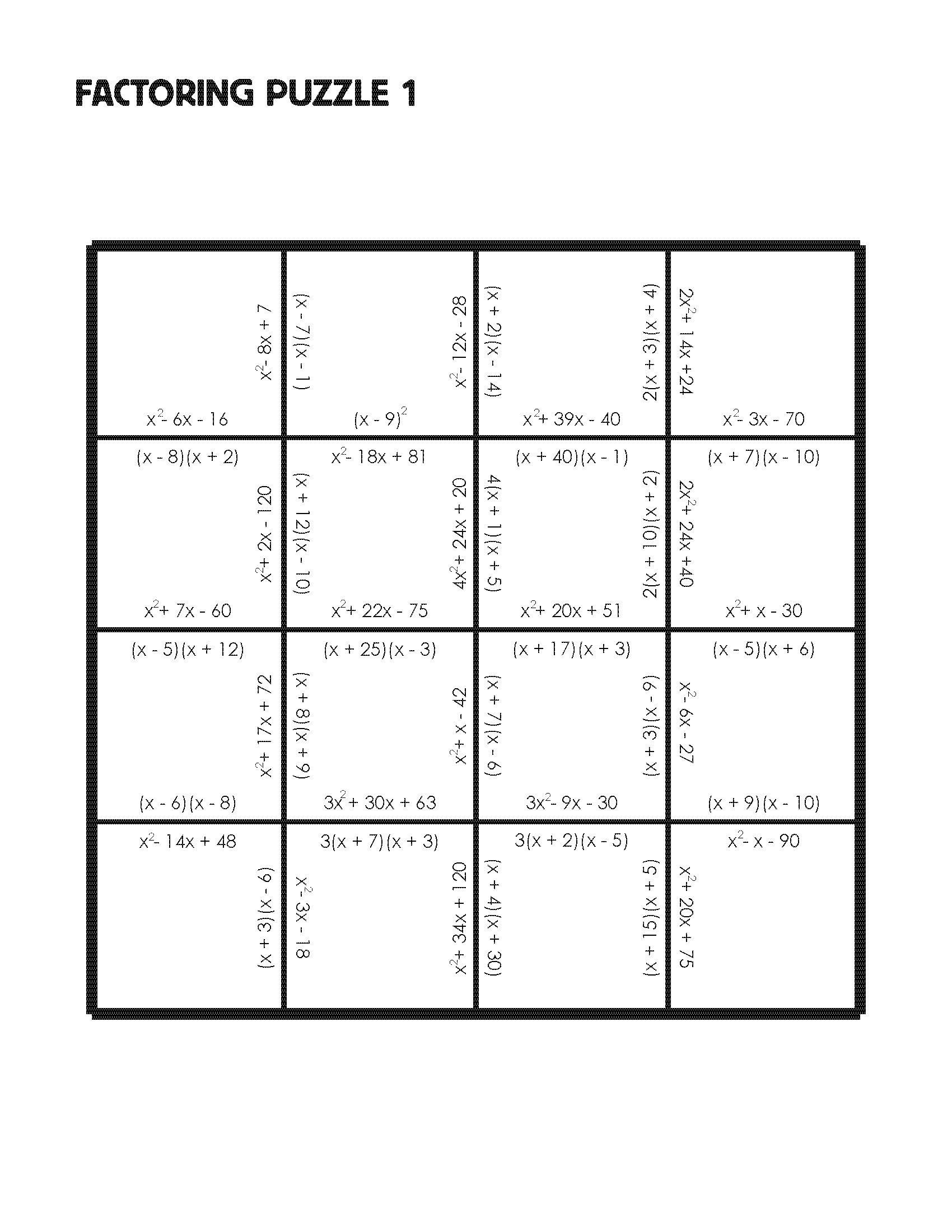
- Factoring Puzzle Cut Out
- GCF and LCM Worksheets
- Graphing Cheat Sheet
- Math Algebra Equations Worksheets
- Homophone English Worksheet Year 2
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
What is factoring?
Factoring is a financial transaction in which a company sells its accounts receivable (invoices) to a third party, known as a factor, at a discount in exchange for immediate cash. This allows the company to improve cash flow and access funds that may otherwise be tied up in unpaid invoices. Factors then collect the full amount owed from the customers of the company, allowing the company to focus on its operations without worrying about collecting payments.
What is a polynomial?
A polynomial is a mathematical expression consisting of variables, coefficients, and non-negative integer exponents that are combined using addition, subtraction, and multiplication operations. Polynomials can have one or more terms and are usually written in the form of \(a_nx^n + a_{n-1}x^{n-1} + \cdots + a_1x + a_0 \), where \(a_n, a_{n-1}, \ldots, a_1, a_0\) are constants, \(x\) is the variable, and \(n\) is a non-negative integer representing the degree of the polynomial.
How do you factor a polynomial with a common factor?
To factor a polynomial with a common factor, you simply identify the factor that each term has in common and then factor it out of each term. This common factor is then placed outside a set of parentheses, with the terms divided by the common factor placed inside the parentheses. The expression inside the parentheses now represents the remaining factors after factoring out the common factor.
How do you factor a quadratic trinomial?
To factor a quadratic trinomial, you can use the method of decomposition or the quadratic formula. First, check if you can factor out a common factor. Then, look for two numbers that multiply to the constant term and add up to the coefficient of the middle term. Use these numbers to split the middle term and factor by grouping. Finally, simplify the expression until you get two binomial factors. Remember to check the factored form to ensure it is correct.
How do you factor a difference of squares polynomial?
To factor a difference of squares polynomial, you need to identify two terms that are perfect squares and are separated by a subtraction sign. Then, you can factor the polynomial by applying the formula (a^2 - b^2) = (a + b)(a - b), where 'a' and 'b' represent the square roots of the terms in the polynomial. By using this formula, you can find the factors of the difference of squares polynomial efficiently.
How do you factor a perfect square trinomial?
To factor a perfect square trinomial, first identify if the trinomial is in the form of \(a^2 + 2ab + b^2\) or \(a^2 - 2ab + b^2\). Then, you can factor it as \((a + b)^2\) or \((a - b)^2\), respectively. This means you take the square root of the first term, the square root of the last term, and use the middle term to determine the sign in the binomial factor.
How do you factor a polynomial with four terms?
To factor a polynomial with four terms, you can try grouping the terms in pairs and factoring out a common factor from each pair. Then, look for common factors between the two resulting expressions and factor them out further if possible. Keep simplifying until you have factored the polynomial completely. Another method you can use is the method of trial and error by attempting to find two binomials that multiply together to give the original polynomial. Be sure to check your work by multiplying your factored form to ensure that you have factored the polynomial correctly.
How do you factor a polynomial by grouping?
To factor a polynomial by grouping, first group the terms in pairs. Then, factor out the greatest common factor from each pair separately. Next, look for a common binomial factor between the two resulting terms and factor that out. Finally, combine the remaining terms to get the fully factored form of the polynomial. This method is especially useful for polynomials with four terms.
How do you factor a polynomial with a leading coefficient greater than 1?
To factor a polynomial with a leading coefficient greater than 1, you can use various methods such as factoring by grouping, factor by substitution, or other techniques like trial and error or the rational root theorem. It involves finding the roots or factors of the polynomial by looking for common factors, grouping terms, or using algebraic manipulation until you can simplify the expression. By factoring the polynomial, you are essentially breaking it down into its component factors, which can help you understand its behavior and solve equations involving the polynomial more easily.
How do you check if a factoring is correct?
To check if a factoring is correct, you can multiply the factors together. If the result matches the original number that was factored, then the factoring is correct. It's important to be diligent in your calculations to ensure accuracy.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.












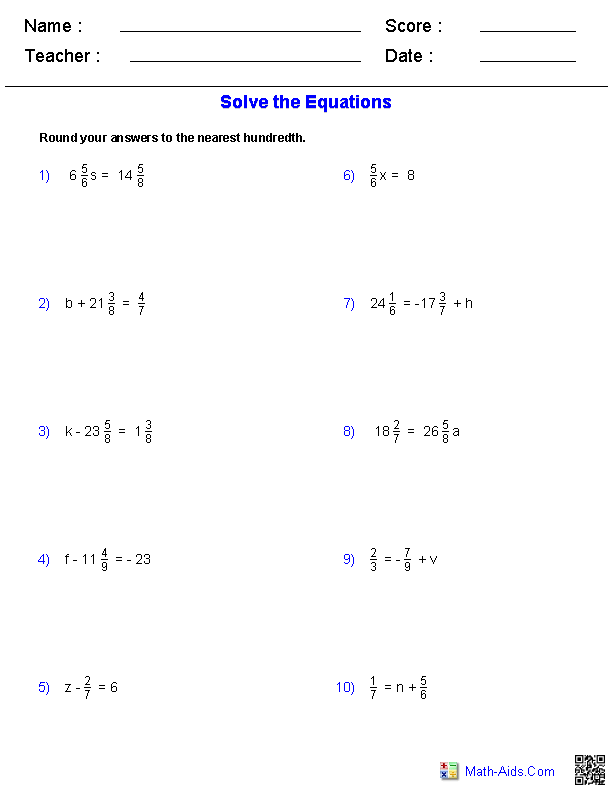















Comments