Factoring Polynomials Completely Worksheet
Factoring polynomials can be a challenging concept to grasp for many students. However, with the right resources and practice, it can become much easier. That's why we have created a comprehensive worksheet that focuses on factoring polynomials completely. Designed specifically for high school students who are currently studying algebra, this worksheet will help you strengthen your understanding of factoring and improve your problem-solving skills.
Table of Images 👆
- Algebra 1 Factoring Polynomials Worksheet with Answers
- Polynomials and Factoring Practice Worksheet Answers
- Algebra 1 Factoring Problems and Answers
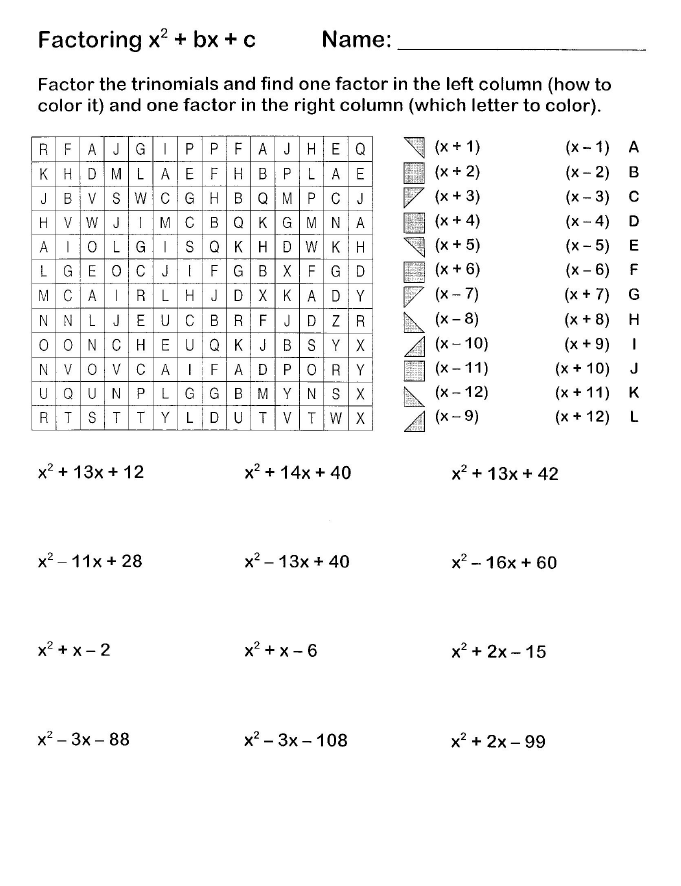
- Factoring Trinomials Worksheet Coloring
- Factoring by Grouping Worksheet
- Factoring Trinomials Worksheets Free Printable
- Factoring Polynomials Worksheet
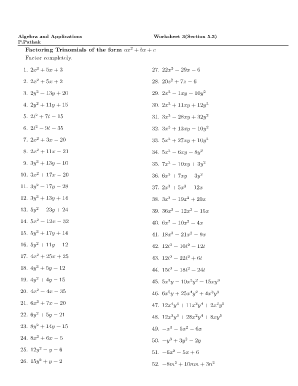
- Factoring Polynomials Completely
- Factoring Trinomials Practice Worksheet
- Factoring Polynomials by Grouping Worksheets
- Factoring Trinomials Worksheet
- Algebra 2 Factoring Review Worksheet Answers
- Algebra 1 Factoring Polynomials Worksheet
- Factoring by Grouping Worksheet Answers
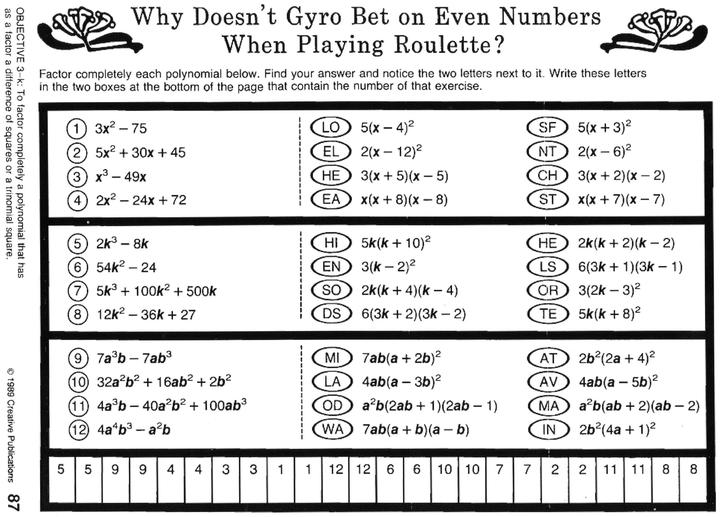
- Factoring Polynomials Worksheet Puzzle
- Ax2 Bx C Factoring Trinomials Worksheet with Answers
- Factoring Polynomials by Grouping Problems
- Algebra 1 Factoring Worksheets
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
What is the term "factoring" referring to when we talk about factoring polynomials completely?
When we talk about factoring polynomials completely, the term "factoring" refers to the process of breaking down a polynomial into its irreducible factors, which are expressions that cannot be factored further. This involves finding all the factors of the polynomial, including any common factors and factors that are quadratic or higher degree. By factoring a polynomial completely, we can express it as a product of its irreducible factors, which helps in simplifying and understanding the polynomial expression.
What is a polynomial, and why do we need to factor it?
A polynomial is an expression consisting of variables and coefficients that involve operations of addition, subtraction, and multiplication. Factoring a polynomial involves breaking it down into simpler components and finding its roots, which helps us to solve equations, identify patterns, and understand the behavior of the polynomial function. It is important to factor polynomials as it allows us to simplify complex expressions, find solutions to equations, and analyze their properties, making it easier to work with them in various mathematical contexts.
How do you determine the greatest common factor (GCF) of a polynomial?
To determine the greatest common factor (GCF) of a polynomial, you need to factorize each term in the polynomial completely and then identify the highest power of each common factor that appears in all terms. The GCF is then the product of those common factors raised to the highest power they share. This process helps in simplifying the polynomial and finding the largest factor that all terms have in common.
How can you factor a simple trinomial like x^2 + 5x + 6?
To factor the trinomial x^2 + 5x + 6, you would look for two numbers that multiply to 6 (the constant term) and add up to 5 (the coefficient of the middle term). In this case, the numbers are 2 and 3. Then, rewrite the middle term using these numbers to split it into two terms: x^2 + 2x + 3x + 6. Finally, factor by grouping: (x^2 + 2x) + (3x + 6) = x(x + 2) + 3(x + 2) = (x + 2)(x + 3). So, x^2 + 5x + 6 factors to (x + 2)(x + 3).
What is a quadratic polynomial, and how can you factor it completely?
A quadratic polynomial is a polynomial of degree 2, which is in the form of ax^2 + bx + c, where a, b, and c are constants with a not equal to 0. To factor a quadratic polynomial completely, you can use methods like factoring by grouping, the AC method (product-sum method), or the quadratic formula. By factoring a quadratic polynomial completely, you break it down into simpler factors or expressions that represent the original polynomial accurately.
How do you apply the difference of squares method to factor a polynomial?
To apply the difference of squares method to factor a polynomial, you need to identify if the polynomial can be written as the difference of two perfect squares (e.g., a² - b²). Then you factor it by using the formula (a + b)(a - b), where 'a' and 'b' represent the square roots of the terms. By expanding the product of (a + b)(a - b), you can simplify the polynomial into its factored form.
What is a perfect square trinomial, and how can you factor it completely?
A perfect square trinomial is a trinomial that can be written as the square of a binomial. It is in the form of \( a^2 + 2ab + b^2 \), where \( a \) and \( b \) are constants. To factor a perfect square trinomial completely, you can use the formula \( (a + b)^2 = a^2 + 2ab + b^2 \). This means that a perfect square trinomial can be factored as \( (a + b)^2 \), where \( a \) and \( b \) are the square roots of the first and last terms of the trinomial, respectively.
What does it mean to factor a polynomial completely in terms of irreducible factors?
Factoring a polynomial completely in terms of irreducible factors means breaking down the polynomial into a product of irreducible polynomials, where irreducible polynomials cannot be factored further over the given field. This process involves finding all possible factors of the polynomial, including linear and quadratic factors, until the polynomial can no longer be factored any further. The goal is to express the polynomial as a product of irreducible factors that cannot be simplified any more.
How can you factor a polynomial if it has a common binomial factor?
To factor a polynomial that has a common binomial factor, you can first factor out the common binomial factor using the distributive property. This involves dividing each term of the polynomial by the binomial factor, which leaves you with the remaining terms. You then write the binomial factor outside the parentheses and the simplified terms inside the parentheses to express the factored form of the polynomial. This process helps simplify the polynomial expression by breaking it down into more manageable factors.
What is the connection between factoring polynomials and solving equations with these factors?
The connection between factoring polynomials and solving equations with these factors lies in the Fundamental Theorem of Algebra, which states that every polynomial equation has as many solutions as its degree. By factoring a polynomial, we can break it down into simpler expressions that represent its factors, each of which corresponds to a potential solution to the equation. Solving the equation then involves setting each factor equal to zero and finding the roots, or solutions, of the equation. This process allows us to efficiently find all the possible solutions to a polynomial equation by leveraging the relationships between the factors and the solutions.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.













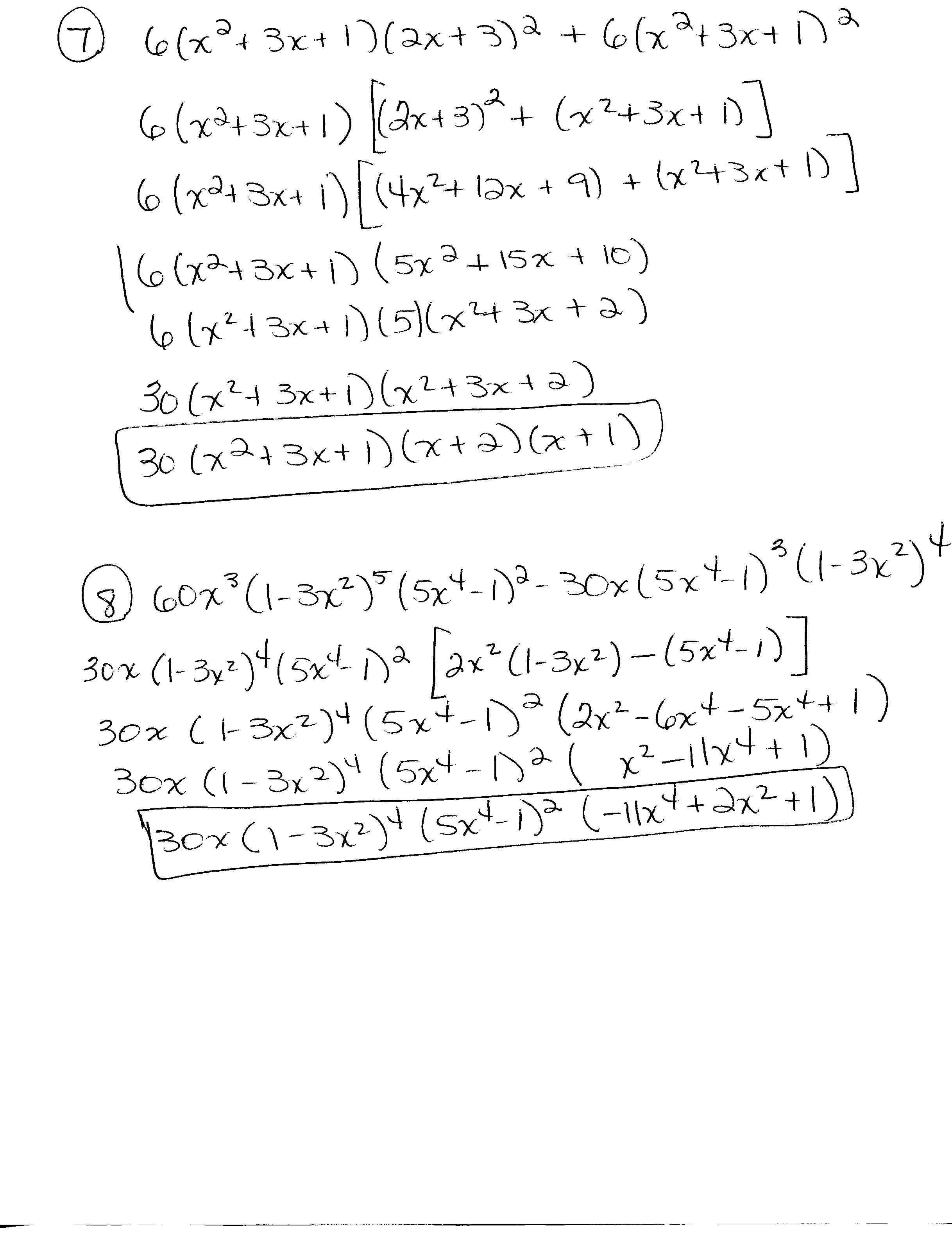

















Comments