Equation of Line Worksheets

The equation of line worksheets are designed to help students master the concept of finding the equation of a line given different information. These worksheets provide practice problems that focus on identifying the entities involved, such as slope, y-intercept, and point(s) on the line, and using these entities to form the equation of the line. Whether you are a math teacher in search of additional resources or a student who wants to improve their skills in finding line equations, these worksheets offer a valuable tool for learning and practicing this fundamental concept.

  Point-Slope Formula Worksheet

---

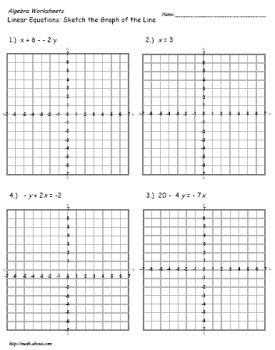
  Linear Equations Worksheets

---

  These Linear Equations Worksheets

---

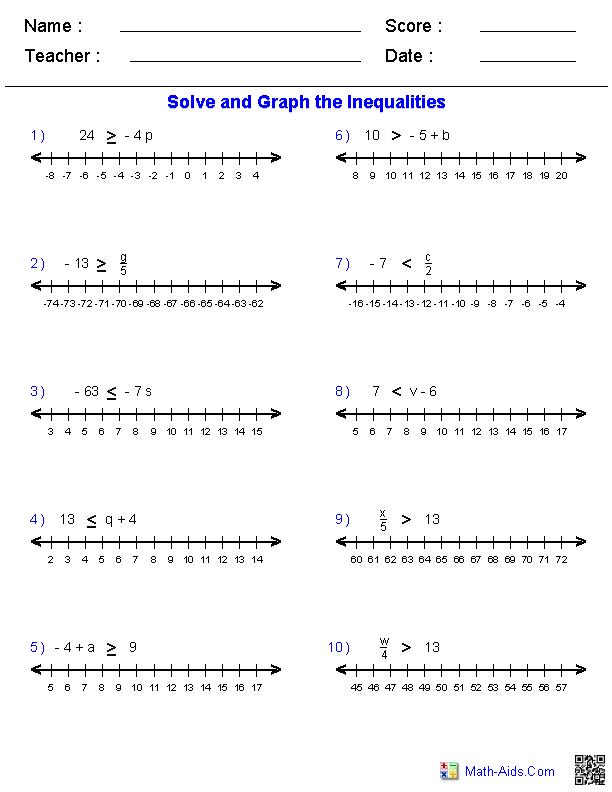
  One Step Inequalities Worksheet

---

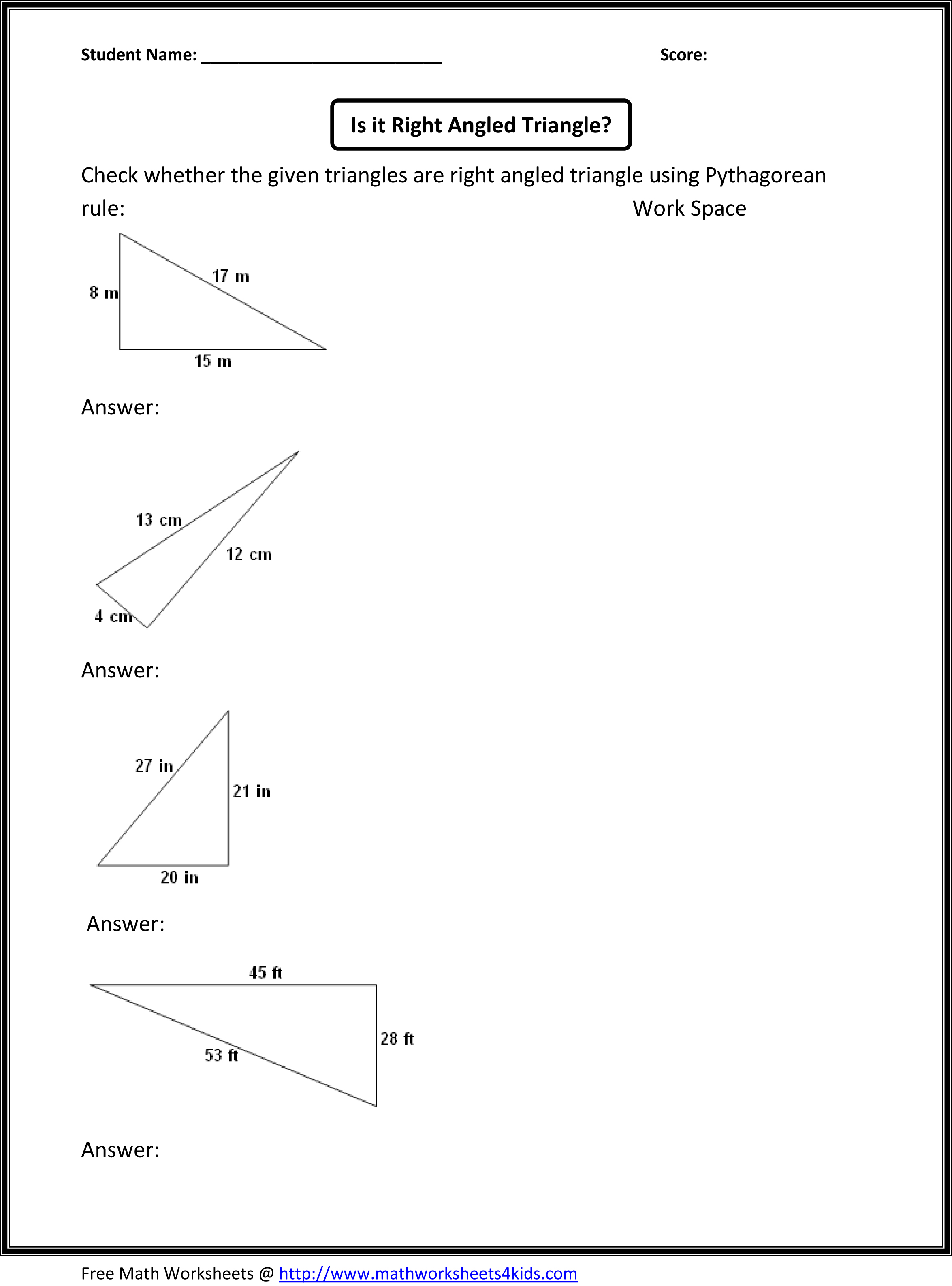
  7th Grade Math Worksheets

---

  8th Grade Math Worksheets Geometry

---

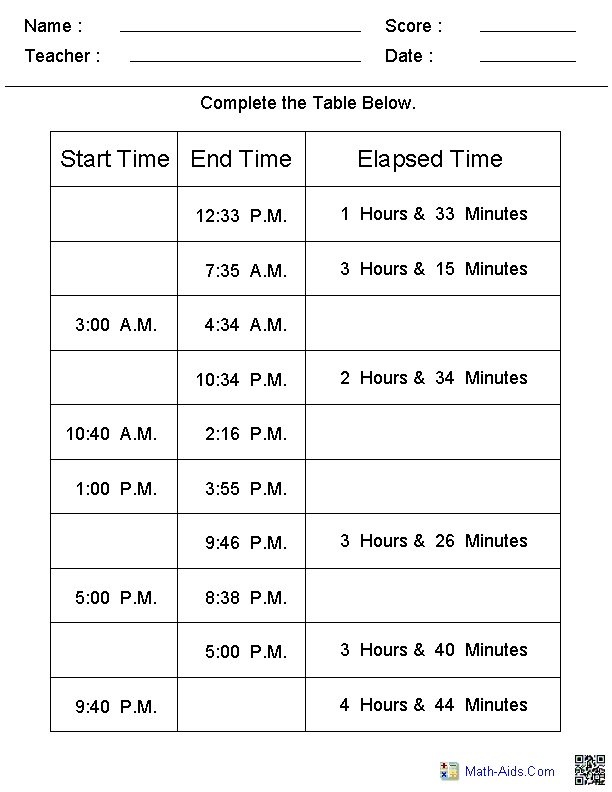
  Table Elapsed Time Worksheets

---

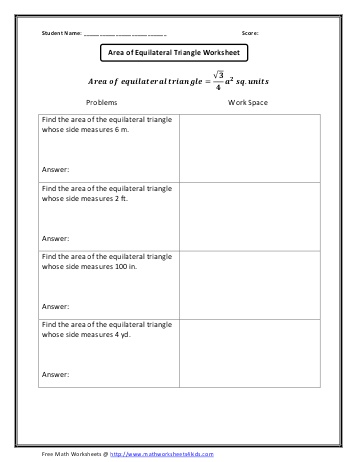
  Equilateral Triangle Worksheet

---

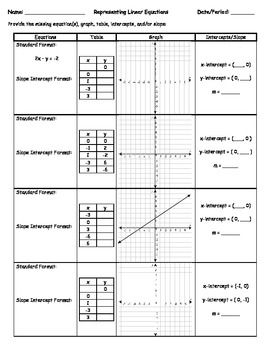
  Linear Equations and Their Graphs Worksheet

---

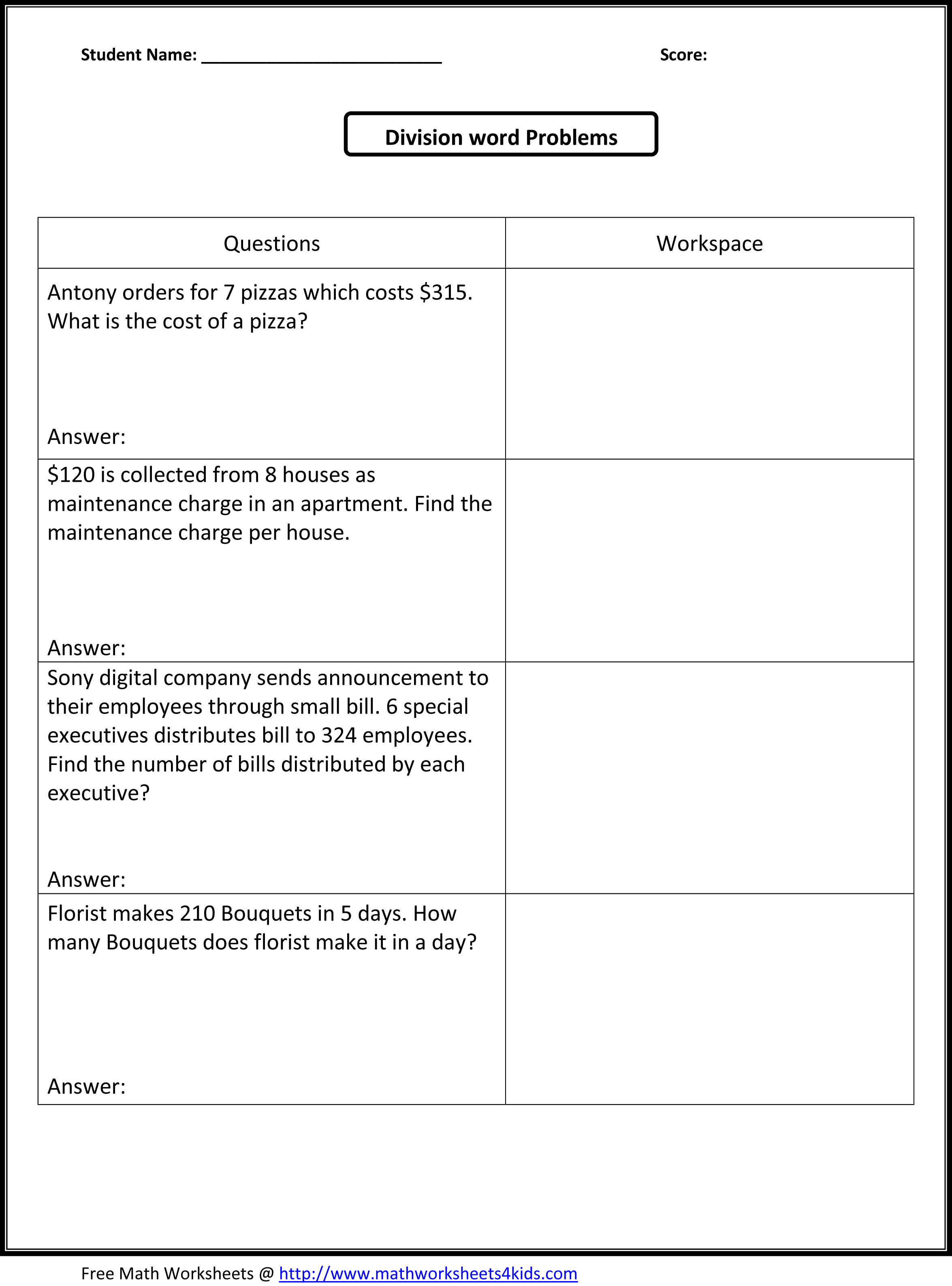
  5th Grade Math Word Problems Worksheets

---

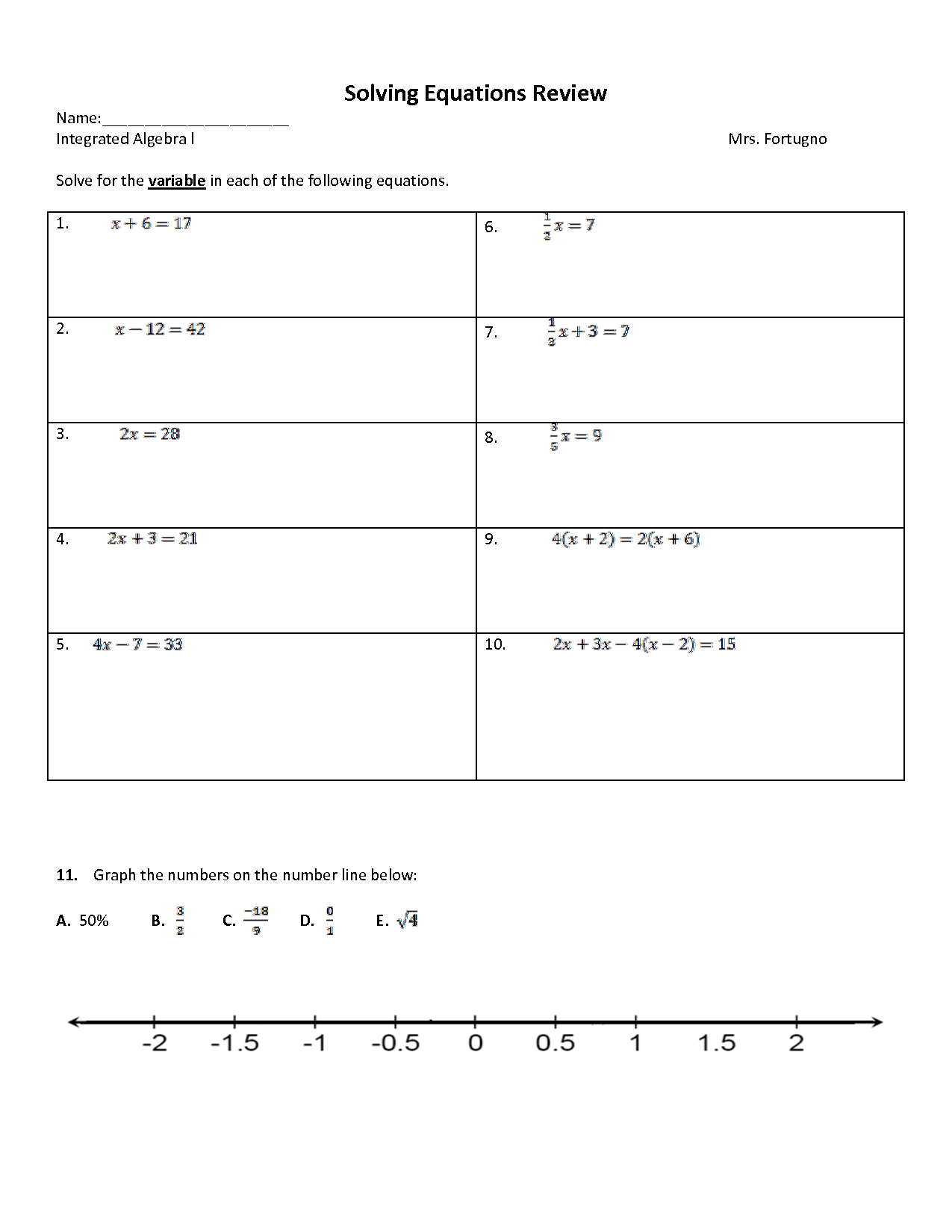
  Solving Equations with Variables On Both Sides Worksheet

---

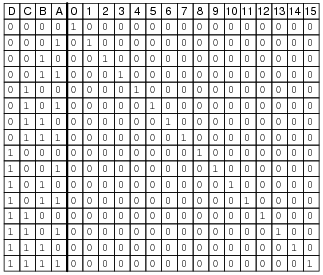
  Binary to Decimal Decoder Truth Table Examples

---

  Multiplication Word Problems Worksheets

---

  8th Grade Math Word Problems

---

  Kuta Software Infinite Algebra 1 Answers Key

---

What is the equation of a line in slope-intercept form?

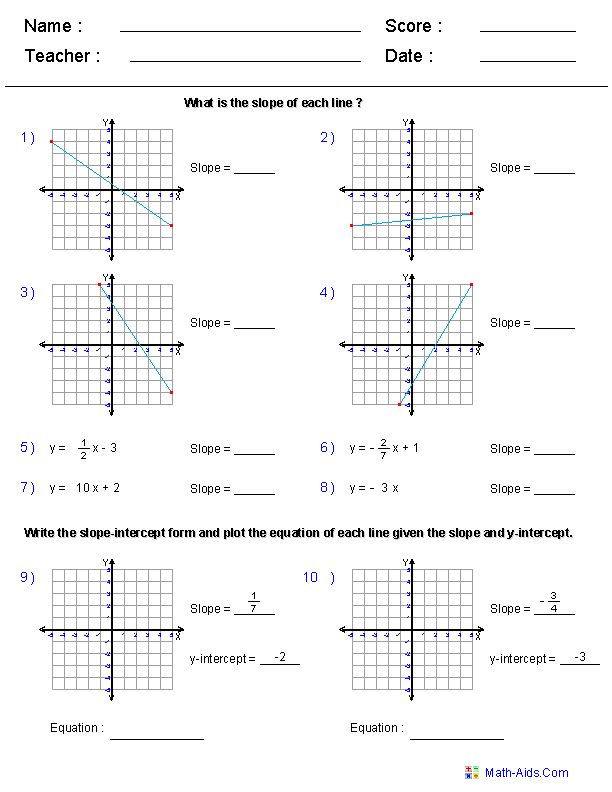
The equation of a line in slope-intercept form is y = mx + b, where m represents the slope of the line and b represents the y-intercept, which is the point where the line intersects the y-axis. The slope (m) indicates the rate at which the line increases or decreases vertically as it moves horizontally, while the y-intercept (b) indicates the point where the line crosses the y-axis.

How can you determine the slope of a line?

To determine the slope of a line, you can use the formula "rise over run," which is calculated by dividing the change in the y-coordinate by the change in the x-coordinate between two points on the line. Alternatively, you can also use the equation of the line in the form y = mx + b, where "m" represents the slope of the line.

What is the equation of a line in point-slope form?

The equation of a line in point-slope form is y - y? = m(x - x?), where (x?, y?) is a point on the line and m is the slope of the line.

How can you find the equation of a line given two points on the line?

To find the equation of a line given two points on the line, first calculate the slope of the line using the formula (y2 - y1)/(x2 - x1) where (x1, y1) and (x2, y2) are the coordinates of the two points. Once you have the slope, choose one of the points to substitute into the point-slope form equation y - y1 = m(x - x1) where m is the slope. Simplify the equation to convert it into slope-intercept form y = mx + b, where b represents the y-intercept. This will give you the equation of the line passing through the two points.

How do you convert an equation of a line from standard form to slope-intercept form?

To convert an equation of a line from standard form (Ax + By = C) to slope-intercept form (y = mx + b), you can solve for y by isolating it on one side of the equation. First, subtract Ax from both sides to isolate the y-term, giving you By = -Ax + C. Then, divide by B to get y alone, resulting in y = (-A/B)x + C/B. This equation is now in slope-intercept form, where the coefficient of x (-A/B) gives you the slope (m) of the line and the constant term (C/B) is the y-intercept (b) of the line.

How do you find the x-intercept of a line given its equation?

To find the x-intercept of a line given its equation, you set y equal to zero and solve for x. The x-coordinate where the line crosses the x-axis represents the x-intercept of the line.

How can you determine if two lines are parallel or perpendicular?

Two lines are parallel if they have the same slope, meaning they are always at the same angle to each other and will never intersect. To determine if two lines are perpendicular, calculate the slopes of both lines and if the product of the slopes is -1, then the lines are perpendicular as they intersect at a right angle.

How do you find the equation of a line parallel to a given line passing through a specific point?

To find the equation of a line parallel to a given line passing through a specific point, you need to know that parallel lines have the same slope. First, determine the slope of the given line. Then, use the given point to create the equation of the line using the point-slope form: y - y? = m(x - x?), where m is the slope of the given line and (x?, y?) is the specific point the new line passes through.

What is a linear equation and how is it related to the equation of a line?

A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and a single variable raised to the first power. It represents a straight line when graphed on a coordinate plane, with the variable typically on the vertical axis and the constant on the horizontal axis. The equation of a line in slope-intercept form (y = mx + b) is a specific type of linear equation, where "m" is the slope of the line and "b" is the y-intercept. Thus, the equation of a line is a specific example of a linear equation that describes a straight line's relationship between its variables.

How can you graph the equation of a line given its equation?

To graph the equation of a line, first rewrite the equation in slope-intercept form, y = mx + b, where m is the slope and b is the y-intercept. Plot the y-intercept on the y-axis, then use the slope to find at least one more point on the line by moving vertically (rise) and horizontally (run) according to the slope. Once you have two points, draw a straight line passing through them to represent the graph of the line.