Distributive Property Worksheets 6th Grade

Are you a teacher or parent looking for supplemental resources to reinforce the concept of the distributive property? Look no further, as we have a collection of worksheets specifically designed for 6th-grade students. These worksheets will provide ample practice opportunities for students to understand and apply the distributive property in various mathematical expressions.

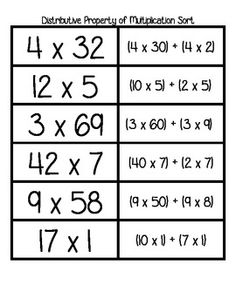
  Algebra Worksheet Distributive Property

---

  Math Worksheets Distributive Property

---

  Distributive Property 4th Grade

---

  6th Grade Math Word Problems Worksheets

---

  Simplifying Expressions Worksheets 7th Grade

---

  Basic Math Skills Practice Worksheets

---

  Commutative Property of Addition Examples

---

  Math Addition Worksheets 2nd Grade

---

  Math Fraction Manipulatives

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

  High Contrast Black and White Lion

---

What is the distributive property in math?

The distributive property in math states that the product of a number with the sum or difference of two or more numbers is equal to the sum or difference of the products of the number with each of the individual numbers. In other words, a(b + c) = ab + ac and a(b - c) = ab - ac, where a, b, and c are any real numbers.

How does the distributive property work with addition?

The distributive property with addition states that when you multiply a number by the sum of two other numbers, you can first multiply the number by each of the two numbers and then add the results together. In symbols, it can be expressed as: a(b + c) = ab + ac. This property helps simplify expressions and is a fundamental concept in algebra.

How does the distributive property work with subtraction?

When using the distributive property with subtraction, you distribute the negative sign to each term inside the parentheses. This means that when you have a subtraction outside the parentheses, the subtraction sign is applied to each term inside the parentheses. For example, if you have \( a - (b + c) \), you distribute the subtraction sign to the terms inside the parentheses to get \( a - b - c \).

How does the distributive property work with multiplication?

The distributive property states that when you multiply a number by a sum of two numbers, you can multiply each addend separately and then add the results. For example, if you have a(b + c), you can distribute the a to both b and c individually, resulting in a*b + a*c. This property allows for simplifying expressions and solving equations more efficiently in mathematics.

How does the distributive property work with division?

The distributive property does not directly apply with division the same way it does with multiplication. However, when dividing by a number, you can think of it as breaking up the dividend into smaller parts, similar to how you would use the distributive property with multiplication. For example, when dividing a sum or difference by a number, you can divide each individual term by that number separately. Ultimately, division breaks down a quantity into equal parts, which is a different operation than the distributive property at its core.

How do you simplify expressions using the distributive property?

To simplify expressions using the distributive property, you need to distribute (multiply) each term outside the parentheses to every term inside the parentheses. This means multiplying the term outside the parentheses by each term inside the parentheses and then combining like terms to simplify the expression. You should always follow the order of operations - parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right) - to ensure the correct simplification of the expression.

How can the distributive property be used to solve equations?

The distributive property can be used to solve equations by simplifying expressions on each side of the equation. By expanding and distributing terms within parentheses, you can manipulate the equation to isolate variables and determine their values. This property allows you to combine like terms and ultimately solve for the unknown variable in the equation.

How does the distributive property relate to factoring in algebra?

The distributive property states that when multiplying a sum by a number, you can distribute the number to each term in the sum. This property is essential in factoring algebraic expressions because factoring involves reversing the process of distribution. By factoring, we break down an expression into its factors, often common terms or binomial factors, by identifying how the distributive property was applied in the original expression. So, understanding the distributive property is crucial for efficiently factoring algebraic expressions.

How can the distributive property be used to solve real-world problems?

The distributive property can be used to simplify real-world problems involving multiplication and addition. By breaking down multiplication into smaller parts and distributing the factors across the different terms in an equation, the distributive property can help to solve problems involving calculations such as finding the total cost of multiple items at different prices, or distributing resources evenly among a group of people. This property allows for a more efficient and systematic approach to solving complex problems by making computations easier to manage and understand.

What are some common mistakes students make when applying the distributive property?

Common mistakes students make when applying the distributive property include forgetting to distribute each term inside the parentheses, incorrectly distributing a negative sign, mixing up the order of operations, and not distributing to all terms in a sum or difference. It is important for students to carefully work through each step and practice applying the distributive property to avoid these errors.