Distributive Property Printable Worksheets

Are you a teacher or parent in search of effective and engaging worksheets to help teach the concept of distributive property to your students or children? Look no further! We have a wide range of printable worksheets that focus on the entity and subject of distributive property, ensuring that your target audience gains a solid understanding of this mathematical concept.

  Distributive Property Math Algebra Worksheets

---

  Math Properties Worksheets 6th Grade

---

  Kuta Software Infinite Algebra 1 Answers

---

  Math-Drills.com Distributive Worksheets

---

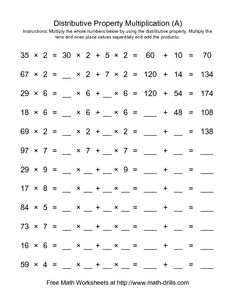
  Distributive Property Multiplication Worksheets

---

  Distributive Property and Combining Like Terms Worksheet

---

  Combining Like Terms Worksheets

---

  6th Grade Math Worksheets Fractions

---

  Solving Equations with Parentheses Worksheet

---

  Solving Algebra Equations Worksheets

---

  Math Properties Worksheets 6th Grade

---

  Simple Equations with Variables Worksheets

---

  6th Grade Math Worksheets Fractions

---

  Multiplication by 5 Worksheets

---

  Common Core Adding and Subtracting Fractions Worksheets

---

  Common Core Adding and Subtracting Fractions Worksheets

---

What is the main concept or principle behind the distributive property?

The main concept behind the distributive property is that it allows us to distribute a factor that is outside of a parenthesis to each term within the parenthesis. This property demonstrates that when we multiply a sum by a number, we can simply multiply each addend within the sum by that number individually and then perform the operation. In other words, it helps to break down a complex multiplication expression into simpler parts, making calculations easier and more organized.

How does the distributive property affect operations such as multiplication and addition?

The distributive property states that when multiplying a number by a sum, you can distribute the multiplication across each term in the sum. This means that in multiplication, you can break down the operation and distribute it over the numbers being multiplied. For example, 3 x (2 + 4) can be solved as 3 x 2 + 3 x 4. Similarly, the distributive property also affects addition as it allows you to simplify expressions involving addition by breaking them down into smaller parts.

Can you provide an example of using the distributive property with multiplication?

Sure! An example of using the distributive property with multiplication is: 2 x (3 + 4) = (2 x 3) + (2 x 4). Using the distributive property, you distribute the multiplier (2) to each term inside the parentheses, then carry out the multiplication to simplify the expression on both sides of the equation.

In what situations is it helpful to use the distributive property?

The distributive property is helpful in situations where you need to simplify expressions by distributing a factor to every term inside parentheses. This property is especially useful when working with algebraic equations, factoring polynomials, or performing calculations involving multiplication and addition or subtraction. It helps break down complex expressions into simpler terms, making it easier to solve problems and manipulate equations effectively.

How does the distributive property help simplify mathematical expressions and equations?

The distributive property helps simplify expressions and equations by allowing us to distribute a number or variable to each term inside parentheses. This simplifies the calculation process by breaking down the expression into smaller, more manageable parts. By distributing the number or variable, we can combine like terms and perform operations more efficiently, ultimately leading to a simpler and more concise mathematical expression or equation.

What are some common mistakes or misconceptions when using the distributive property?

One common mistake when using the distributive property is forgetting to multiply all terms inside the parentheses by the term outside the parentheses. Another misconception is incorrectly applying the distributive property when the operation inside the parentheses is division or subtraction, as it is only applicable for multiplication or addition. It is important to carefully follow the rules of the distributive property to avoid errors in mathematical calculations.

Can you give an example of using the distributive property with subtraction?

Certainly! An example of using the distributive property with subtraction is: 2(7 - 4) = 2*7 - 2*4. By applying the distributive property, we can simplify the expression to get 2(3) = 14 - 8, which further simplifies to 6 = 6.

How does the distributive property relate to factoring in algebra?

In algebra, the distributive property is used to expand or simplify expressions by distributing each term in one factor to each term in the other factor. Factoring, on the other hand, involves finding the common factors within an expression to simplify it. The distributive property can be helpful in factoring because it allows us to break down an expression into its factors by reversing the process of distribution. By identifying common factors using the distributive property, we can factor out these terms to simplify the expression.

What are some real-life scenarios where understanding and applying the distributive property is useful?

Understanding and applying the distributive property is useful in various real-life scenarios such as calculating discounts on purchases, distributing resources or funds among different parties, simplifying complex mathematical expressions in finance or accounting, and breaking down complicated algebraic equations in engineering or physics. Additionally, the distributive property can be applied in scaling recipes, organizing data in spreadsheets, or optimizing operations in business and manufacturing processes. Overall, mastering the distributive property can enhance problem-solving skills and efficiency in many practical situations.

Can you explain why the distributive property is a fundamental concept in mathematics?

The distributive property is a fundamental concept in mathematics because it allows us to simplify and manipulate algebraic expressions by breaking them down into simpler parts. By distributing a factor across terms inside parentheses, we can make calculations more efficient and solve complex equations more easily. This property is foundational for understanding how numbers and variables interact in arithmetic and algebra, serving as a crucial tool in various mathematical applications and problem-solving techniques.