Cylinder Printable Worksheets

Cylinder printable worksheets provide an excellent tool for students to enhance their understanding and grasp of this specific geometrical shape. Whether you're a teacher looking for supplementary resources or a parent seeking additional practice for your child, these worksheets offer an engaging way to reinforce key concepts and develop problem-solving skills related to cylinders. With a focus on entity and subject, these worksheets facilitate the exploration and examination of cylinder properties, volume, surface area, and real-life applications – catering to learners at varying levels of proficiency.

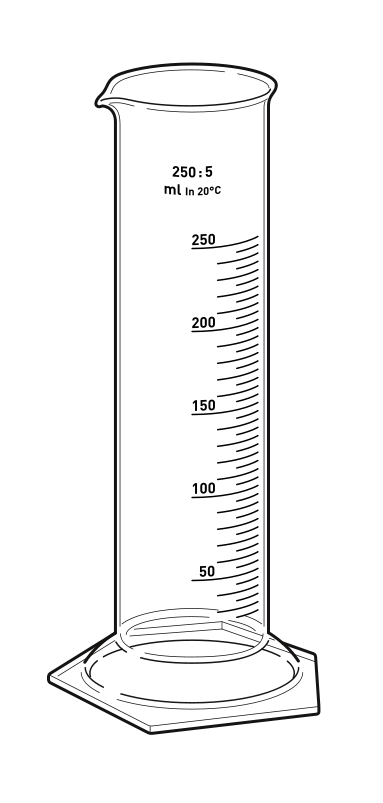
  250 Ml Graduated Cylinder

---

  Make Your Own 3D Shapes

---

  Geometry Properties of Parallelograms Worksheet

---

  Liquid Volume Graduated Cylinder Worksheet

---

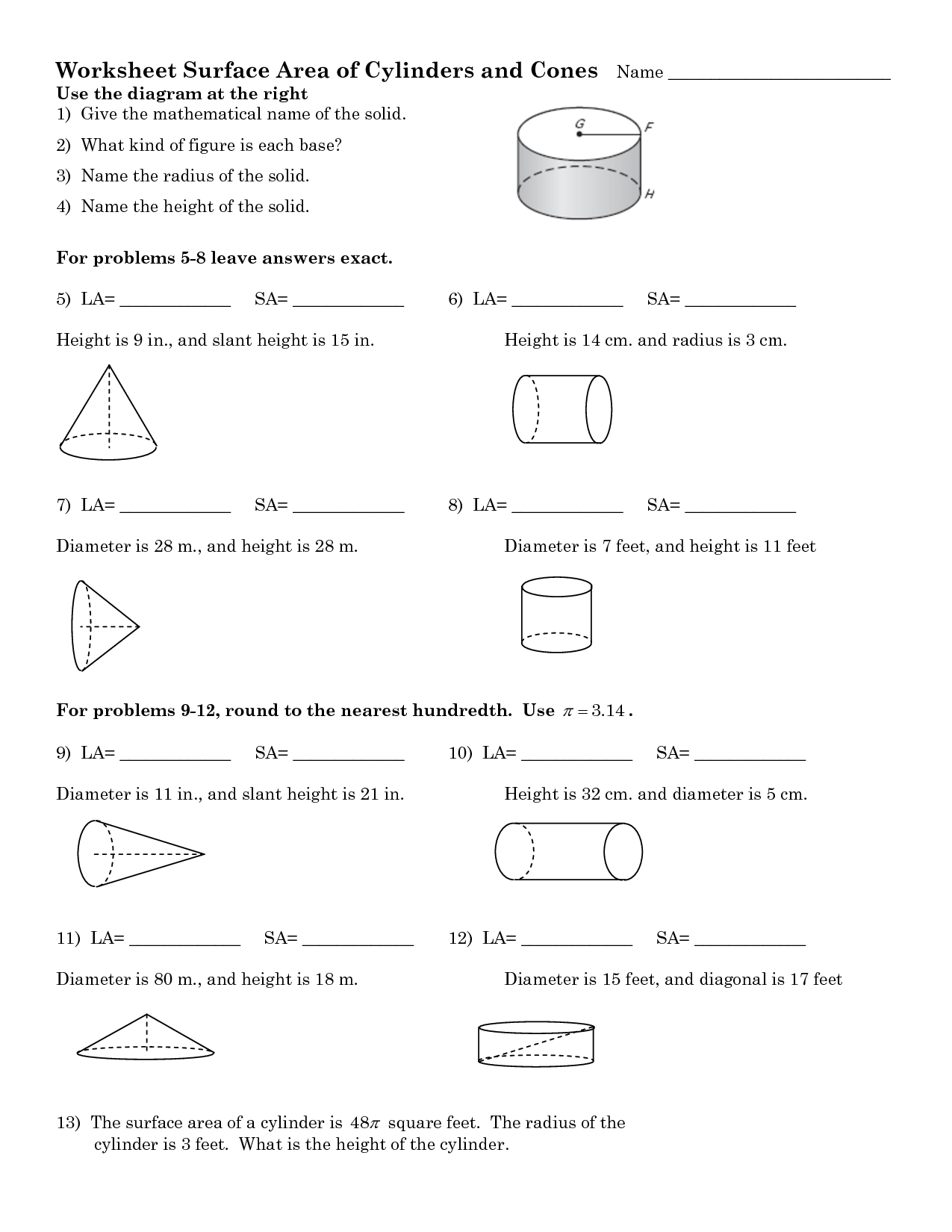
  Surface Area Cylinder Worksheet

---

  Write Cursive Letters

---

  Gruffalo Colouring Pages

---

  2nd Grade Math Coloring Worksheets

---

  Shape Word Search Puzzles

---

  3-Dimensional Shape Worksheets Printable

---

  Drawing 2D Shapes Worksheet

---

What is a cylinder?

A cylinder is a three-dimensional geometric shape that has two parallel circular bases connected by a curved surface. It is a type of prism, with its cross-section being a circle. A cylinder is commonly used in everyday objects such as cans, tubes, and barrels.

How many faces does a cylinder have?

A cylinder has two faces - one is the circular base, and the other is the circular top.

What is the relationship between the height and the radius of a cylinder?

The height and radius of a cylinder are independent of each other; changing one does not necessarily affect the other. The height and radius determine the volume and surface area of the cylinder but do not have a direct proportional relationship to each other.

How do you calculate the surface area of a cylinder?

To calculate the surface area of a cylinder, you need to add the areas of the two circular bases and the lateral surface area. The formula for the surface area of a cylinder is 2?r² + 2?rh, where r is the radius of the base and h is the height of the cylinder. Calculate the areas of the two circular bases by using the formula ?r² and then find the lateral surface area by multiplying the circumference of the base (2?r) by the height of the cylinder (h). Finally, add these three components together to find the total surface area of the cylinder.

What is the formula for finding the volume of a cylinder?

The formula for finding the volume of a cylinder is V = ?r^2h, where V is the volume, r is the radius of the base of the cylinder, and h is the height of the cylinder.

How can you find the lateral surface area of a cylinder?

To find the lateral surface area of a cylinder, you can use the formula 2?r*h, where r is the radius of the cylinder and h is the height of the cylinder. Multiply the circumference of the base (2?r) by the height of the cylinder (h) to calculate the lateral surface area. This formula calculates the surface area of just the curved part of the cylinder, excluding the top and bottom circle.

Can the base of a cylinder be any shape other than a circle?

No, the base of a cylinder must always be in the shape of a circle. This is a defining characteristic of a cylinder, where the base is circular and the top surface is also circular, parallel to the base. Changing the base shape to any other geometric shape would alter the fundamental properties of a cylinder.

How would you find the total surface area of a cylinder?

To find the total surface area of a cylinder, you would add the areas of the two bases (top and bottom) and the lateral surface area. The formula for the lateral surface area of a cylinder is given by 2?rh, where r is the radius of the base and h is the height of the cylinder. The formula for the area of each base is ?r^2, where r is the radius of the base. So, the total surface area of a cylinder is calculated as 2?r(r + h), where r is the radius of the base and h is the height of the cylinder.

How can you determine if a given shape is a cylinder or not?

To determine if a given shape is a cylinder or not, you would need to assess its characteristics. A cylinder is a three-dimensional shape with two parallel and congruent circular bases connected by a curved surface. So, if the shape has these defining features of two circular bases and a connecting curved surface, then it is a cylinder. If it lacks any of these features, then it is not a cylinder.

What are some real-life examples of cylinders?

Some real-life examples of cylinders are soda cans, paper towel rolls, coffee mugs, and hydraulic cylinders in heavy machinery. These objects all have a cylindrical shape, with curved sides and circular bases.