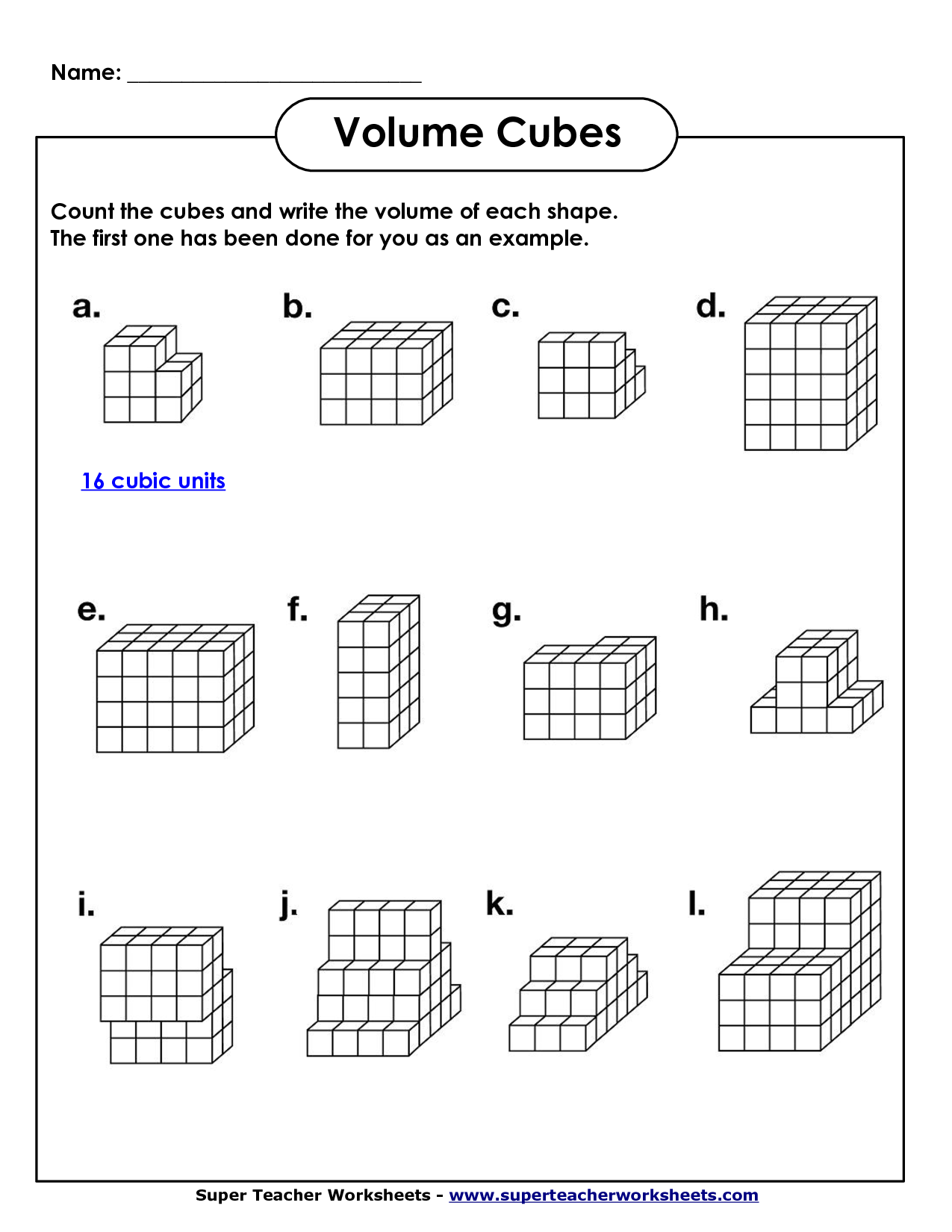
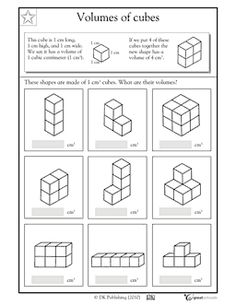
Cubic Volume Worksheets

If you're a math teacher or a parent looking for engaging and educational resources to help your students or children understand the concept of cubic volume, then these worksheets are perfect for you.

  Cube Volume Worksheets 5th Grade Math

---

  Cubic Volume Worksheets for 5th Grade

---

  Volume Worksheets 5th Grade

---

  Volume Unit Cubes Worksheets

---

  Cube Volume Worksheets 5th Grade Math

---

  Cube Root as an Exponent

---

  Cube Root Worksheet

---

  Science Lab Safety Worksheets

---

What is cubic volume?

Cubic volume refers to the amount of space occupied by a three-dimensional object, measured in cubic units. It is calculated by multiplying the length, width, and height of an object. The cubic volume is expressed in cubic units such as cubic inches, cubic feet, or cubic meters, depending on the units used for the measurements.

How is cubic volume measured?

Cubic volume is measured by multiplying the length, width, and height of an object or space to determine the total amount of space it occupies in three dimensions. This formula, cubic volume = length x width x height, is used to calculate the amount of cubic units (such as cubic inches, cubic feet, or cubic meters) that an object or space encompasses.

What are the units used to express cubic volume?

Cubic volume is typically expressed in cubic units, such as cubic centimeters (cm³), cubic meters (m³), or cubic inches (in³), depending on the system of measurement being used.

How do you calculate the cubic volume of a rectangular prism?

To calculate the cubic volume of a rectangular prism, multiply the length, width, and height of the prism together. The formula for the volume of a rectangular prism is Volume = Length x Width x Height. By multiplying these three dimensions, you will find the total cubic units inside the prism, representing its volume.

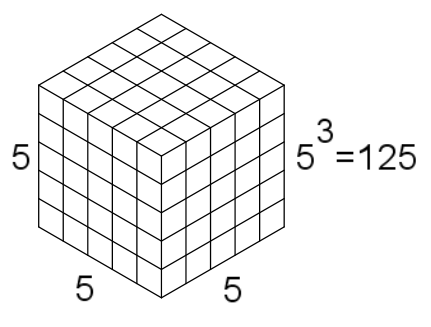
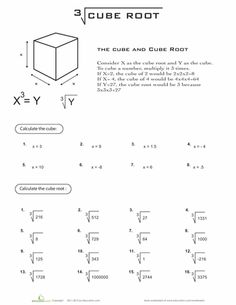
How is the cubic volume of a cube different from a rectangular prism?

The cubic volume of a cube is calculated by cubing the length of one side, while the volume of a rectangular prism is calculated by multiplying its length, width, and height. In a cube, all sides are equal in length, whereas in a rectangular prism, the lengths of the sides can vary. This means that the dimensions are different for each shape, leading to a different calculation method for their volumes.

What is the formula for calculating the cubic volume of a sphere?

The formula for calculating the cubic volume of a sphere is (4/3) x ? x r^3, where r is the radius of the sphere.

How do you find the cubic volume of a triangular prism?

To find the cubic volume of a triangular prism, you need to first calculate the area of the triangular base by multiplying the base length by the height of the triangle and dividing the result by 2. Then, multiply the area of the base by the height of the prism to find the volume. The formula is V = (1/2) * b * h * H, where V is the volume, b is the base length of the triangle, h is the height of the triangle, and H is the height of the prism.

Is it possible to have negative cubic volume?

No, it is not possible to have negative cubic volume. Volume is a measure of the amount of space occupied by an object and it is a scalar quantity that is always non-negative. Negative volume would imply that the space occupied by an object has a negative magnitude, which is not physically possible or mathematically meaningful.

How is cubic volume used in real-life applications?

Cubic volume is commonly used in real-life applications such as determining the amount of space needed for packaging and storing items, calculating the capacity of containers like boxes and tanks, and designing structures like buildings and rooms. It is essential for industries such as construction, shipping, manufacturing, and interior design to accurately measure and manage the volume of objects and spaces for optimal utilization and efficiency. Additionally, cubic volume calculations are vital in fields like engineering and architecture for creating designs and blueprints that adhere to specific spatial requirements and constraints.

Can the cubic volume of an object change if its dimensions change?

Yes, the cubic volume of an object can change if its dimensions change. The volume of an object is directly proportional to the cube of its dimensions. So, if the dimensions change, the volume will also change accordingly.