Combinations Worksheets 7th Grade
In the world of 7th grade math, combinations can often be a tricky concept to grasp. Understanding how to calculate the number of possible combinations is essential for solving a variety of math problems. Luckily, there are worksheets available that can help students practice and master this concept. With a focus on the entity and subject of combinations, these worksheets can provide the necessary practice for 7th graders to confidently tackle combination problems in their math class.
Table of Images 👆
- 7th Grade Math Worksheets
- Free Printable Combination Worksheets
- 7th Grade Writing Math Equations
- 8th Grade Math Worksheets Ratios
- 7th Grade Math PSSA Formula Sheet
- Irregular Verbs Worksheets
- Permutations and Combinations Worksheet 7th Grade
- 6th Grade Probability Worksheets
- 8th Grade Math Worksheets Printable
- 7th Grade Math PSSA Formula Sheet
- 7th Grade Texas History Lesson Plans
- Probability Graphic Organizer
- Basic Probability Worksheet
- Mean Median Mode Worksheets 7th Grade
- Simplifying Expressions Worksheets 7th Grade
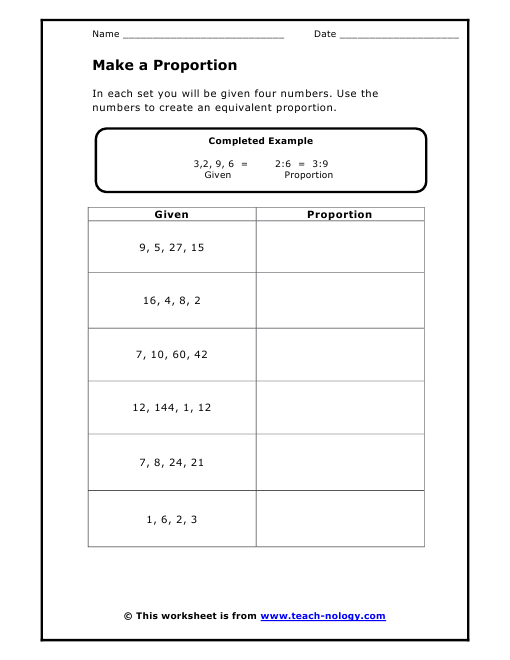
- Ratio and Proportion Worksheets
- 7th Grade Math Formula Chart
- Math Expressions Worksheets 7th Grade
- 2nd Grade Math Practice Worksheets
More 7th Grade Worksheets
7th Grade Math Worksheets with Answer Key7th Grade Math Coloring Worksheets
7th Grade Vocabulary Worksheets
Pre-Algebra 7th Grade Math Worksheets
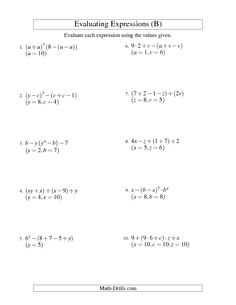
Algebraic Expressions Worksheets 7th Grade
Reading Comprehension Worksheets 7th Grade
7th Grade Math Worksheets Proportions
Complex Sentence Worksheets 7th Grade
Geometry Angles Worksheet 7th Grade Math
What are combinations?
Combinations are selections of items from a group without considering the order in which the items are arranged. In other words, a combination is a way of choosing a subset of items from a larger set, where the order of the selected items does not matter.
How do you calculate the number of combinations?
To calculate the number of combinations, use the formula nCr = n! / (r!(n-r)!), where n represents the total number of items and r represents the number of items being chosen. Simply substitute the values into the formula and compute the result to determine the total number of combinations.
What is the difference between combinations and permutations?
The main difference between combinations and permutations is that combinations are the selection of items without considering the order, while permutations are the arrangement of items where the order matters. In other words, combinations focus on the selection of elements, while permutations focus on both the selection and arrangement of elements.
How are combinations used in real-life situations?
Combinations are used in real-life situations when dealing with selection or arrangement of items where the order does not matter. For example, in a lottery or raffle draw, combinations are used to calculate the number of possible ways a winning ticket can be chosen. In the field of genetics, combinations are used to determine the different possible genotypes that can occur when alleles are mixed. Additionally, combinations are used in forming unique teams or groups, such as selecting a committee from a pool of candidates or picking a group of people to work on a project together.
What is the formula for calculating combinations?
The formula for calculating combinations is C(n, k) = n! / (k!(n-k)!) where n is the total number of items and k is the number of items to choose.
Can you have repetitions in combinations?
No, in combinations, each combination is unique and does not contain any repetitions of elements. Each element can only appear once in a combination.
What is the significance of order in combinations?
Order in combinations refers to the arrangement or sequence of selecting items. In the context of combinations, order is not important, meaning that the selection of items is considered the same regardless of the order in which they are chosen. This is because combinations focus on the selection of a group of items without considering the specific order in which those items are chosen. By disregarding order, combinations help simplify calculations and focus solely on the selection process, making them useful in various mathematical and real-world scenarios.
How do you determine the number of possible combinations when given a certain set of options?
To determine the number of possible combinations when given a certain set of options, you can use the formula for combinations, which is "nCr = n! / r!(n-r)!" where n is the total number of options and r is the number of options you want to choose. By calculating this formula, you can find the total number of unique combinations possible from the given set of options.
How do you approach solving combination problems step by step?
To solve combination problems, start by identifying the total number of options available for each position in the combination (e.g., number of choices for each item). Then, determine the total number of possible combinations by applying the formula for calculating combinations, which is nCr = n! / r!(n-r)!. Substitute the values into the formula and simplify to find the number of possible combinations. Remember to follow the rule of combinations – the order of items does not matter.
What strategies can you use to simplify combination problems and make them easier to solve?
One strategy to simplify combination problems is to break them down into smaller, manageable parts. This can involve grouping items together, identifying patterns, or categorizing elements to make the problem more structured and easier to tackle. Additionally, using visualization techniques, such as diagrams or charts, can help in organizing the information and visualizing the relationships between different elements in the problem. Lastly, leveraging shortcuts or formulas specific to combination problems, like the formula for calculating combinations, can also streamline the problem-solving process and make it more efficient.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.






























Comments