Arithmetic Recursive and Explicit Worksheet

Are you a math teacher or a homeschooling parent searching for an effective way to help your students practice arithmetic skills? Look no further! This blog post is here to introduce you to the concept of arithmetic worksheets, which are a valuable tool for reinforcing key mathematical concepts. By utilizing both recursive and explicit methods, these worksheets provide an engaging and interactive way for students to master arithmetic operations.

  Arithmetic and Geometric Sequences Worksheet Answers

---

  Arithmetic and Geometric Sequence Formula Sheet

---

  Arithmetic and Geometric Sequences Worksheet Answers

---

  Glencoe Algebra 2 Worksheet Answers

---

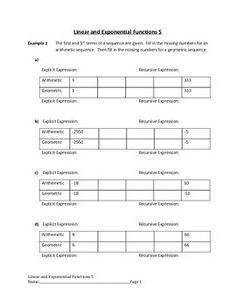
  Exponential Linear and Sequences Worksheets

---

  Exponential Functions and Geometric Sequences Worksheet

---

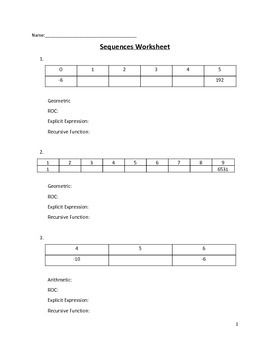
  Recursive and Explicit Formula Worksheet

---

  Arithmetic and Geometric Sequences Worksheets

---

  Recursive Patterns Worksheet

---

What is the difference between a recursive formula and an explicit formula?

A recursive formula defines a sequence by relating each term to the ones preceding it, requiring previous terms to calculate subsequent ones, while an explicit formula directly expresses a term in relation to its position in the sequence without reference to previous terms, allowing for a straightforward calculation of any term without needing to know preceding terms.

How do you find the next term in a sequence using a recursive formula?

To find the next term in a sequence using a recursive formula, you first determine the relationship between consecutive terms in the sequence. Then, you use the recursive formula to calculate the next term by applying the defined relationship to the last known term in the sequence. This involves repeatedly applying the formula to obtain successive terms in the sequence until you reach the desired term.

How do you find the nth term in a sequence using an explicit formula?

To find the nth term in a sequence using an explicit formula, first identify the pattern or rule that the sequence follows. Once you have determined the pattern, you can then create a formula that directly calculates the nth term based on the value of n. For example, in an arithmetic sequence, the explicit formula would take the form of \(a_n = a_1 + (n-1) \cdot d\), where \(a_n\) represents the nth term, \(a_1\) is the first term, and d is the common difference between terms. Simply substitute the value of n into this formula to find the nth term in the sequence.

How do you convert a recursive formula to an explicit formula?

To convert a recursive formula to an explicit formula, you need to find a pattern in the recursive formula that can be directly expressed in terms of the variable without reference to previous terms. This often involves solving for the general term in the recurrence relation by eliminating the dependence on prior terms using techniques like substitution, algebraic manipulations, or induction. Once you've obtained a formula that directly calculates the nth term in the sequence without recursion, you have successfully transformed the recursive formula into an explicit formula.

How do you convert an explicit formula to a recursive formula?

To convert an explicit formula to a recursive formula, you need to break down the explicit formula to express the nth term in terms of previous terms. This involves finding a pattern where each term relies on the values of one or more preceding terms. Once you identify this pattern, you can create a recursive formula that defines each term as a function of prior terms in the sequence, switching from directly calculating each term to using the values of previous terms to determine the next term iteratively.

What is the advantage of using a recursive formula in arithmetic sequences?

One advantage of using a recursive formula in arithmetic sequences is that it allows for easier calculation of subsequent terms without having to know all the terms before. By knowing just the initial term and the common difference, the recursive formula can generate the entire sequence by repeatedly applying the formula. This can be more efficient and practical compared to calculating each term individually, especially for longer sequences or when only specific terms are needed.

What is the advantage of using an explicit formula in arithmetic sequences?

The advantage of using an explicit formula in arithmetic sequences is that it allows for direct calculation of any term in the sequence without the need to calculate each term individually. This can save time and effort, especially when dealing with longer sequences or when trying to find specific terms quickly. Additionally, the explicit formula provides a clear and concise way of understanding the pattern and behavior of the arithmetic sequence.

How do you determine if a sequence is arithmetic?

To determine if a sequence is arithmetic, you need to check if there is a constant difference between consecutive terms. If the difference between each term is the same, then the sequence is arithmetic. This can be done by calculating the common difference between terms or by observing a consistent pattern in the differences.

How do you find the common difference in an arithmetic sequence?

To find the common difference in an arithmetic sequence, subtract any term in the sequence from the term that comes after it. This will give you the constant value by which each term in the sequence is increasing or decreasing. By consistently subtracting consecutive terms, you can identify the common difference in an arithmetic sequence.

How do you find the sum of an arithmetic sequence?

To find the sum of an arithmetic sequence, you use the formula: \( S_n = \dfrac{n}{2} (a_1 + a_n) \), where \( S_n \) is the sum of the sequence, \( n \) is the number of terms, \( a_1 \) is the first term, and \( a_n \) is the last term. Simply plug in the values of these variables to calculate the sum of the arithmetic sequence.