Area of a Trapezoid Worksheet

Are you a math teacher or a student who wants to practice calculating the area of a trapezoid? Look no further, because this blog post will provide you with a worksheet specifically designed to help you master this mathematical concept. The worksheet includes a variety of exercises that will challenge your understanding of the subject and allow you to hone your skills in finding the area of a trapezoid.

  Trapezoid Area and Perimeter Worksheet

---

  Measurement Worksheet

---

  Trapezoid Area and Perimeter Worksheet

---

  Area of Trapezoids and Triangles Worksheets

---

  Trapezoid Area and Perimeter Worksheet

---

  Trapezoid Shape Worksheet

---

  Geometry Area of Regular Polygons Worksheet

---

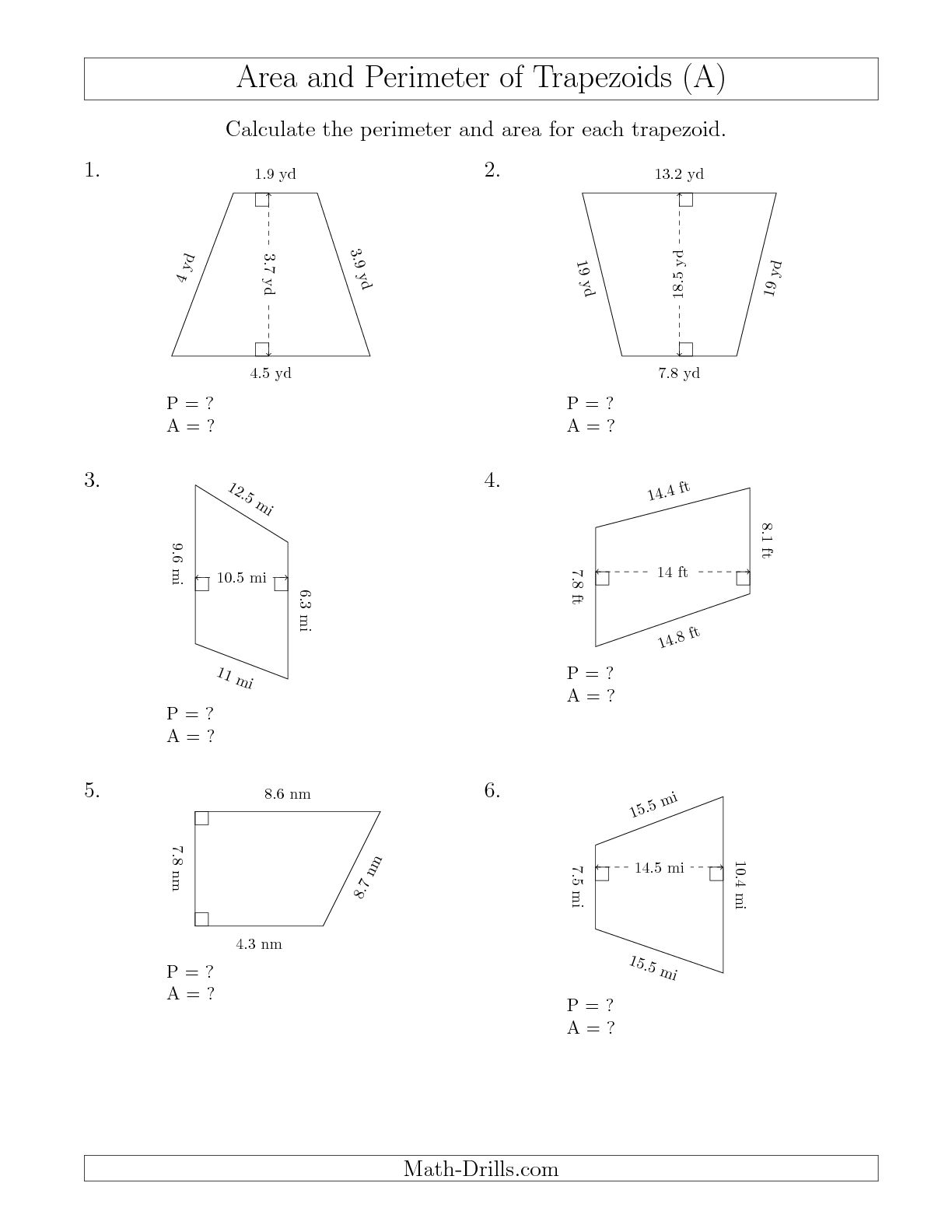
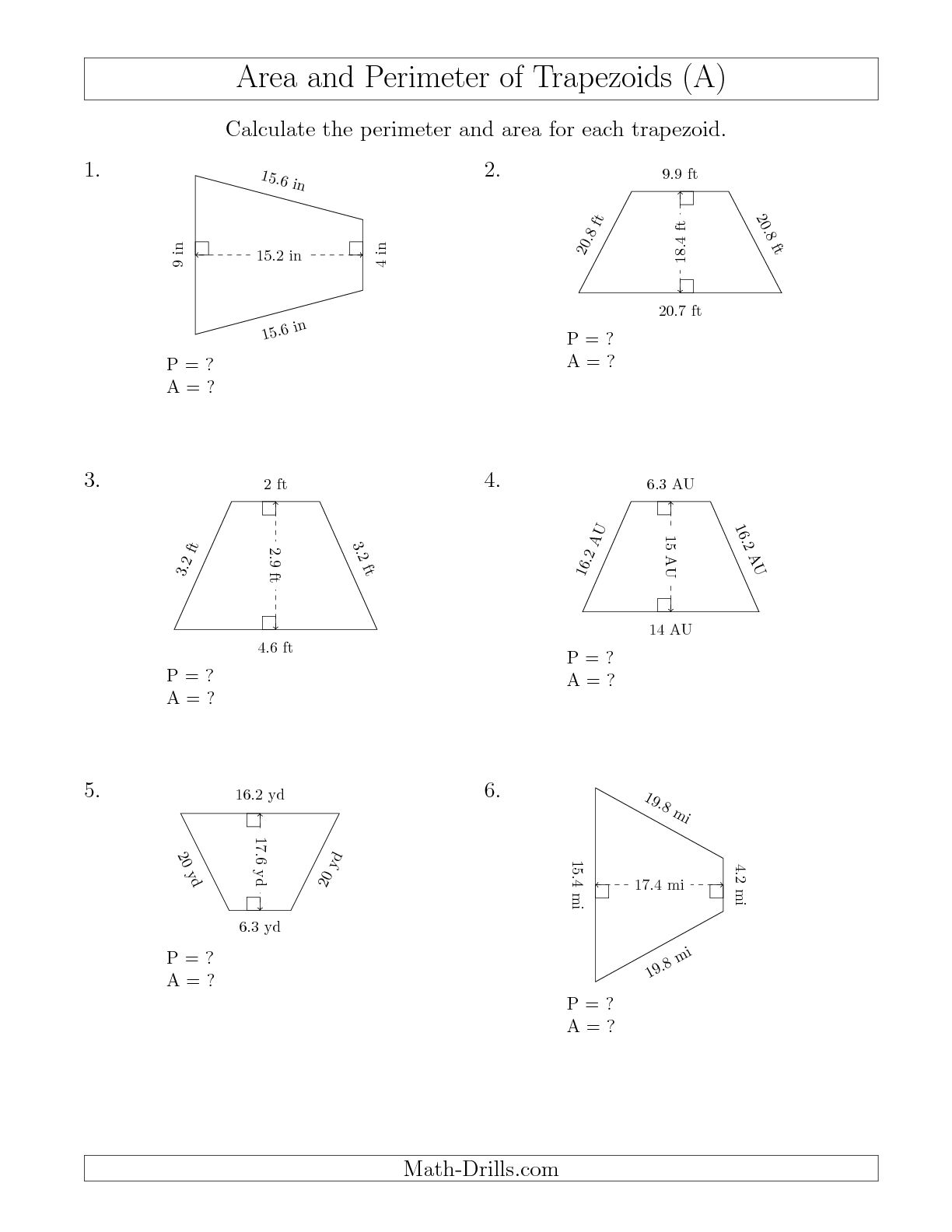
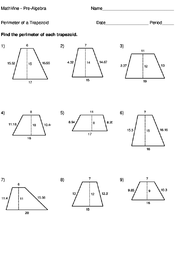
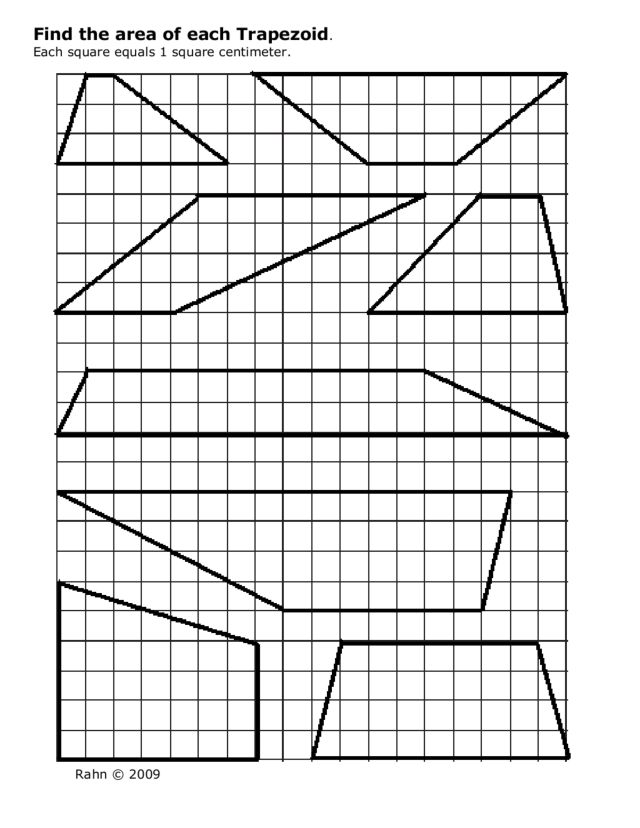
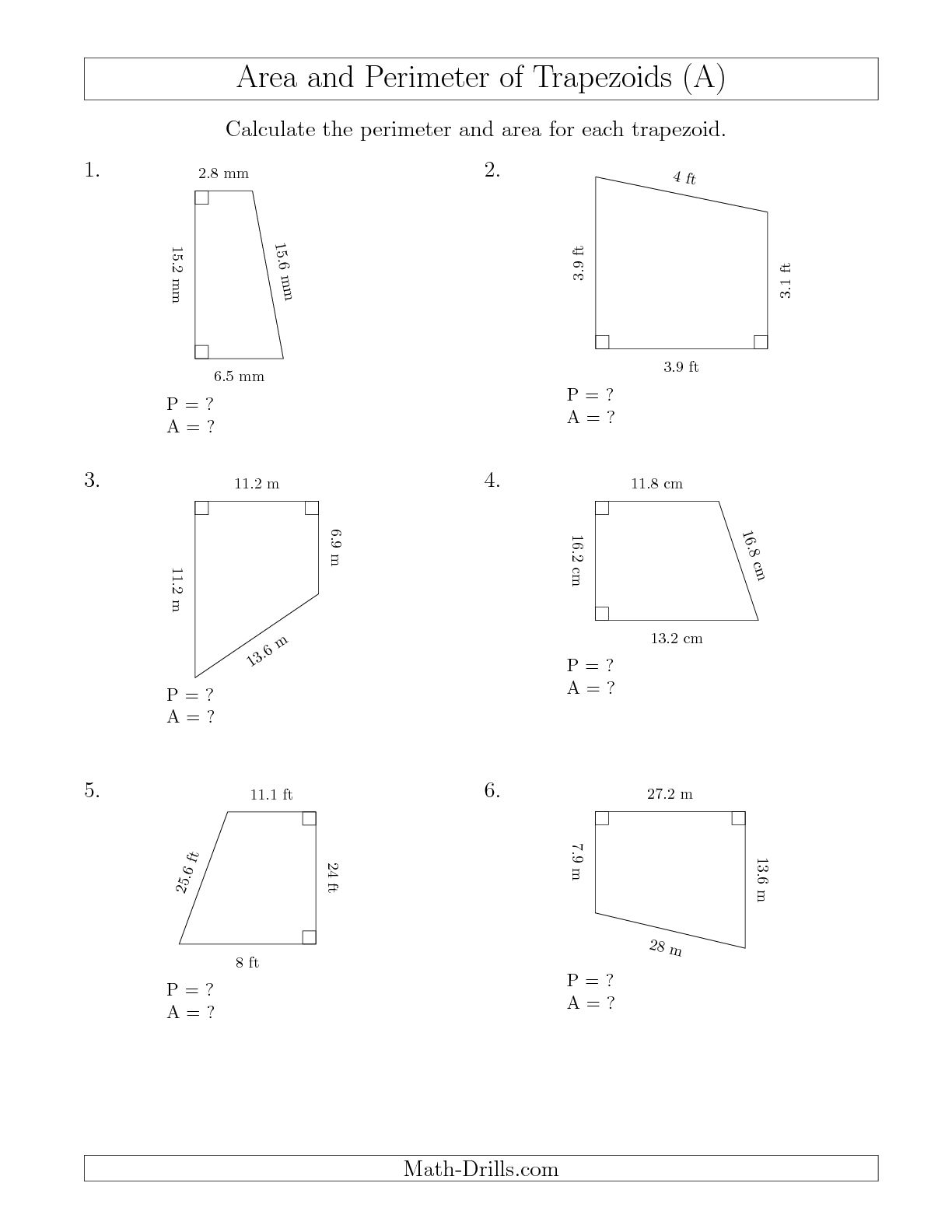
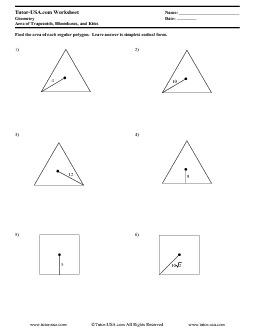
What is the formula for finding the area of a trapezoid?

The formula for finding the area of a trapezoid is A = 1/2 * (b1 + b2) * h, where A represents the area, b1 and b2 are the lengths of the two parallel bases of the trapezoid, and h is the height of the trapezoid perpendicular to the bases.

Define base 1 and base 2 of a trapezoid.

The base 1 of a trapezoid is the longer of the two parallel sides, while the base 2 is the shorter of the two parallel sides. Each base serves as a foundation for the trapezoid, and the height of the trapezoid is the perpendicular distance between these two bases.

How do you calculate the height of a trapezoid?

To calculate the height of a trapezoid, you use the formula: height = (2 * area) / (base1 + base2), where the area of the trapezoid is calculated by multiplying the average of the length of the parallel sides (base1 and base2) by the height. By rearranging the formula, you can find the height by plugging in the values for the area, base1, and base2 of the trapezoid.

Give an example of a trapezoid where the bases are of equal length.

An example of a trapezoid where the bases are of equal length is an isosceles trapezoid. In an isosceles trapezoid, the non-parallel sides are of equal length, making the bases equal as well. This type of trapezoid has two opposite angles that are equal in measure, making it symmetric and balanced.

Can a trapezoid have a right angle? Explain why or why not.

No, a trapezoid cannot have a right angle. By definition, a trapezoid is a quadrilateral with only one pair of parallel sides. A right angle implies that two sides are perpendicular, which would result in a rectangle or a square, not a trapezoid.

How does the length of the bases affect the area of a trapezoid?

The area of a trapezoid is calculated by multiplying the average of the lengths of the two bases by the height. As the length of the bases increases, the area of the trapezoid will also increase because the average of the bases will be larger, resulting in a larger area when multiplied by the height. Conversely, if the length of the bases decreases, the area of the trapezoid will also decrease.

Find the area of a trapezoid with base 1 measuring 6 cm, base 2 measuring 10 cm, and height of 4 cm.

The area of a trapezoid can be calculated using the formula A = 1/2*(base1 + base2)*height. Plugging in the values given into the formula, where base1 is 6 cm, base2 is 10 cm, and height is 4 cm, we get A = 1/2*(6 + 10)*4 = 1/2*16*4 = 32 cm². Therefore, the area of the trapezoid is 32 square centimeters.

If the area of a trapezoid is 24 square units and the height is 3 units, what could the length of the bases be?

The lengths of the bases of the trapezoid could be 8 units and 4 units, or 6 units and 6 units, as long as their product equals 24 square units when multiplied by the height of 3 units.

What is the relationship between the area of a trapezoid and the length of its diagonals?

The area of a trapezoid is not directly related to the length of its diagonals. The area of a trapezoid is calculated using its bases and height, while the length of its diagonals can vary depending on the dimensions and angles of the trapezoid. The diagonals of a trapezoid can help in determining other properties of the shape, such as its angles and side lengths, but they do not have a direct impact on the area of the trapezoid.

Can the bases of a trapezoid be horizontal? Explain why or why not.

Yes, the bases of a trapezoid can be horizontal. In a trapezoid, the pair of parallel sides are called the bases. These bases can be either horizontal or slanted, as long as they are parallel to each other. The key defining characteristic of a trapezoid is not the orientation of its bases, but rather the presence of only one pair of parallel sides.