Algebraic Expressions Worksheets 9th Grade
Algebraic expressions worksheets are essential tools for 9th-grade students who are studying algebra. These worksheets provide a structured and comprehensive approach to learning and practicing algebraic concepts, allowing students to deepen their understanding of variables, coefficients, and equations. By working through these worksheets, students can enhance their problem-solving skills and strengthen their foundation in algebra, enabling them to tackle more complex mathematical challenges with confidence.
Table of Images 👆
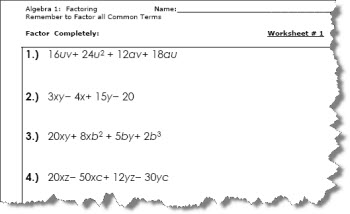
- Algebra 1 Worksheets
- Math Expressions Worksheets 7th Grade
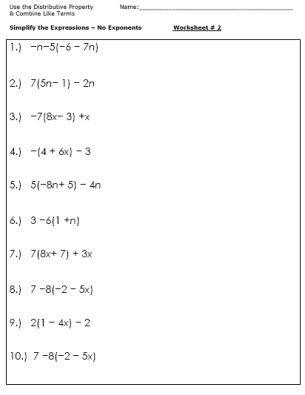
- Simplifying Algebraic Expressions Worksheet
- Math Variables and Expressions Worksheets
- Algebra 1 Worksheets 9th Grade Math
- Variable Expressions Worksheets 6th Grade
- Simplifying Expressions Worksheets 7th Grade
- Algebra 1 Worksheets
- Simplify Expressions Worksheet
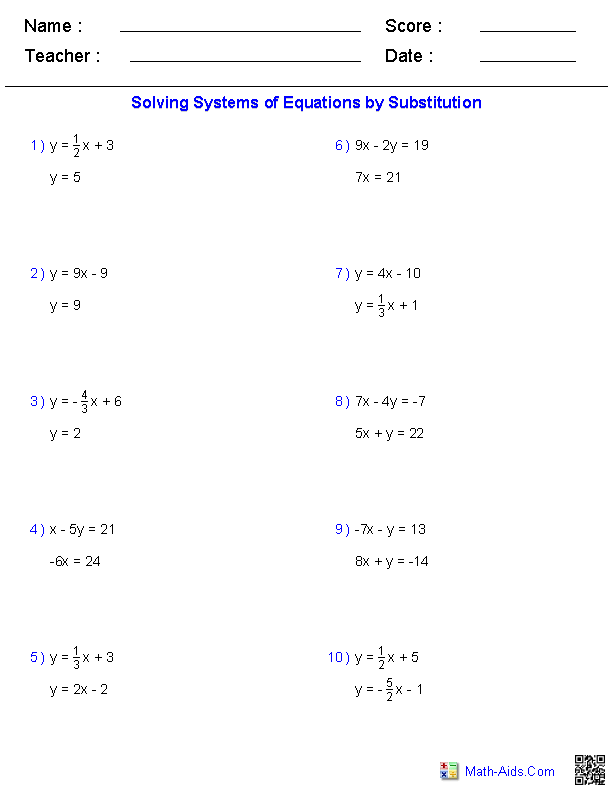
- 7th Grade Math Algebra Equations Worksheets
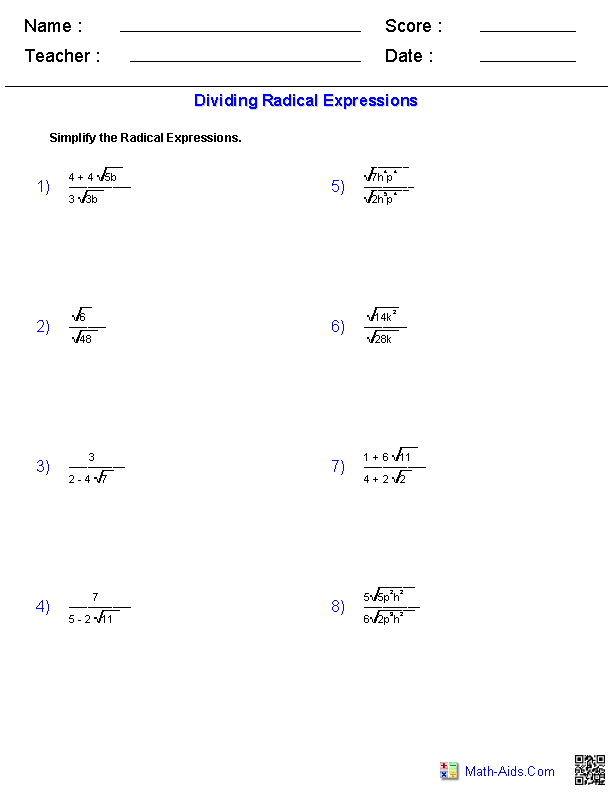
- Dividing Radical Expressions Worksheets
- Algebra 1 Worksheets
- 9th Grade Algebra Math Worksheets
- Pre-Algebra Math Worksheets
- 9th Grade Algebra Math Worksheets Printable
More 9th Grade Worksheets
9th Grade Vocabulary WorksheetsPrintable 9th Grade Reading Comprehension Worksheets
English 9th Grade Vocabulary Worksheets
What is an algebraic expression?
An algebraic expression is a combination of variables, constants, and mathematical operations such as addition, subtraction, multiplication, and division. It does not have an equal sign and represents a mathematical relationship or formula. It can be simplified or evaluated by substituting specific values for the variables.
How do you simplify algebraic expressions?
To simplify algebraic expressions, you need to combine like terms by adding or subtracting coefficients of the same variables. Next, you can perform any operations inside parentheses and simplify any exponents by applying the appropriate rules. Finally, you can factor out any common factors to further simplify the expression. Remember to follow the order of operations (PEMDAS) to ensure the correct simplification of the algebraic expression.
What are the different types of algebraic expressions?
Algebraic expressions can be categorized into different types based on the number of terms they contain. The most common types include monomials (one term), binomials (two terms), trinomials (three terms), and polynomials (more than three terms). Each type of algebraic expression follows specific rules and properties that define their behavior and operations in mathematical computations.
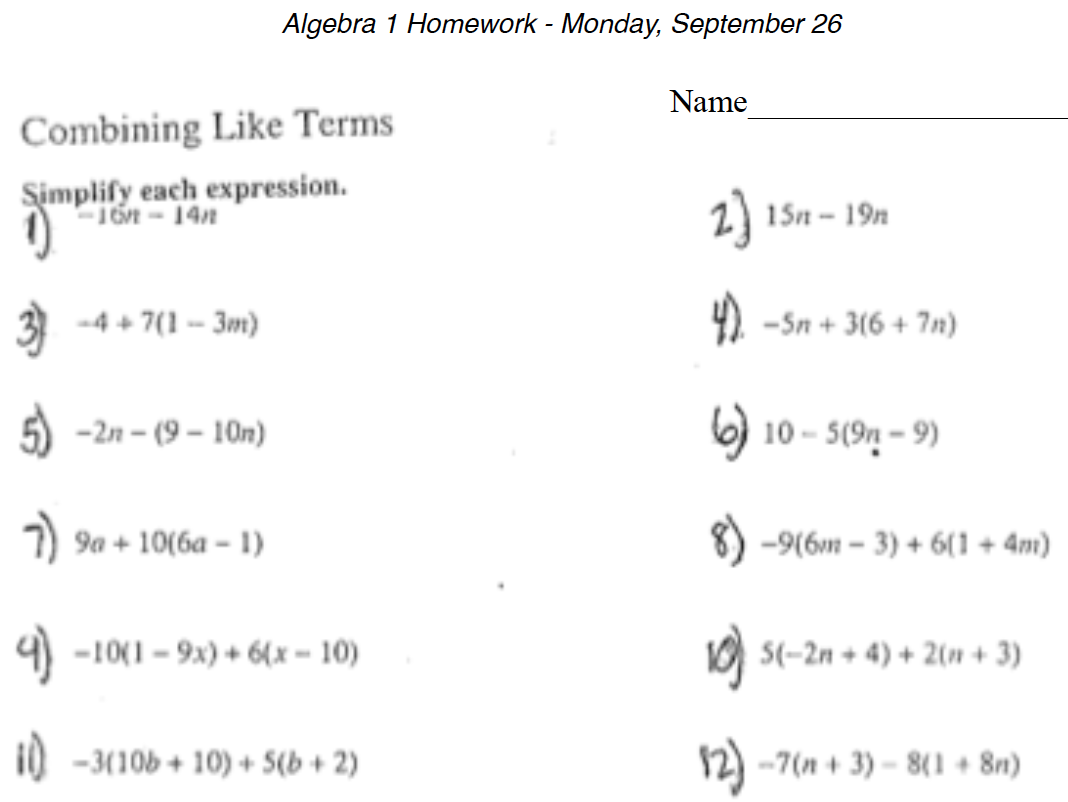
How do you combine like terms in an expression?
To combine like terms in an expression, you need to identify terms that have the same variable(s) raised to the same power(s) and then add or subtract them based on their coefficients. Simply add or subtract the coefficients of these like terms while keeping the variable(s) the same. Keep in mind that the variable part of the term must be exactly the same in order to be considered a like term. By simplifying and combining like terms, you can make the expression more compact and easier to work with.
What are the rules for adding and subtracting algebraic expressions?
When adding or subtracting algebraic expressions, you need to combine like terms. Like terms are terms that have the same variables raised to the same power. You can add or subtract coefficients of like terms while keeping the variables the same. It's important to pay attention to the sign of each term and apply the rules of addition and subtraction accordingly. You can only add or subtract terms that are similar, and you cannot combine terms that are not like terms.
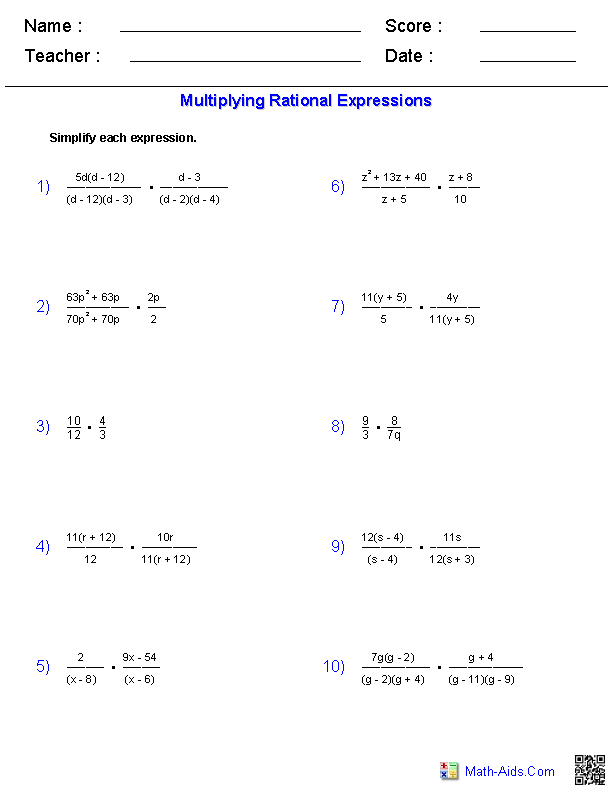
How do you multiply and divide algebraic expressions?
To multiply algebraic expressions, you simply multiply the coefficients together and then multiply the variables together by adding their exponents if they are the same. For division, you divide the coefficients and then divide the variables by subtracting their exponents if they are the same. Remember to simplify the resulting expression by combining like terms and following the rules of exponents.
What is the difference between an equation and an expression?
An equation contains an equal sign and shows a relationship between two expressions, with the goal of finding the value of the variable that makes the equation true. On the other hand, an expression does not have an equal sign and can consist of variables, constants, and operators, serving as a mathematical phrase without asserting equality.
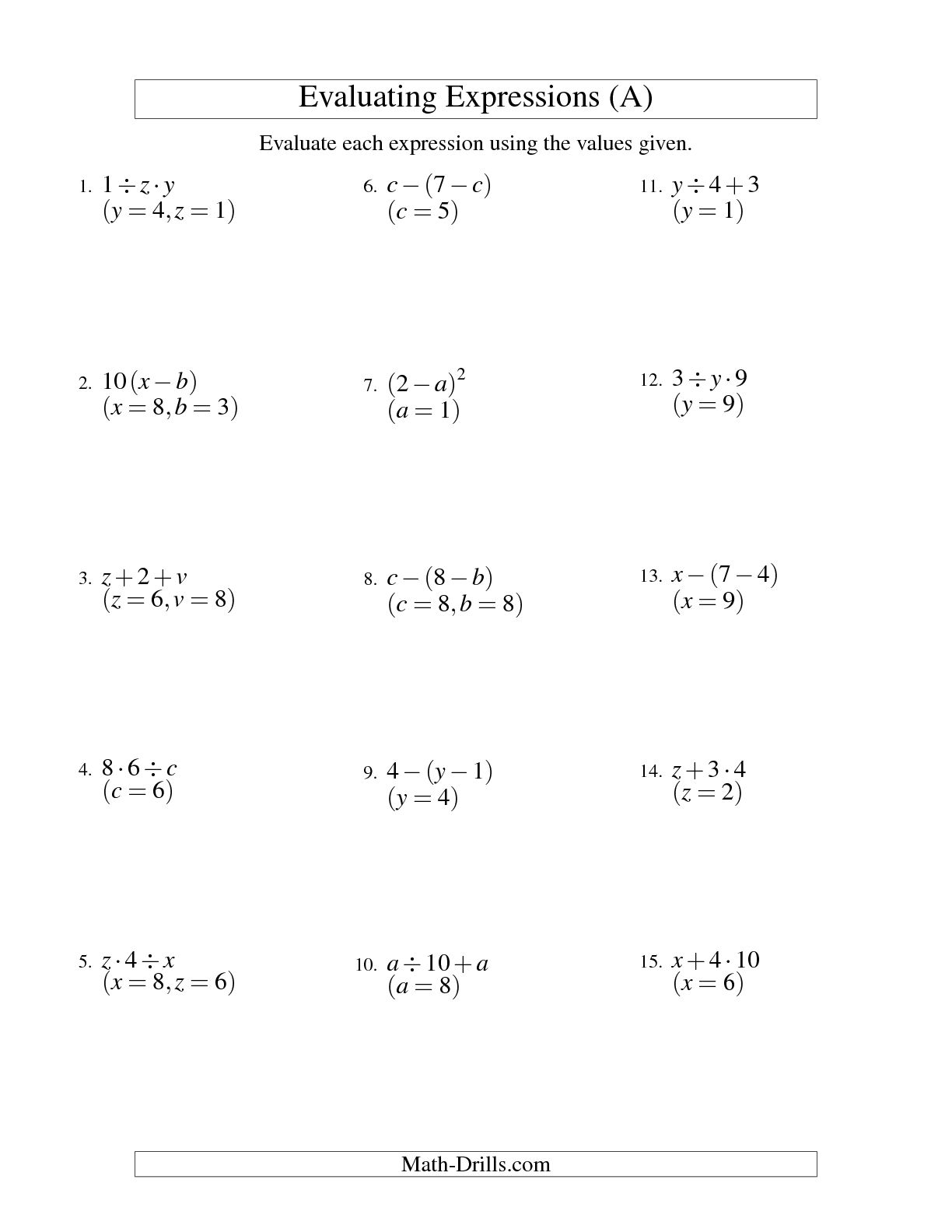
How do you evaluate an algebraic expression for a given value?
To evaluate an algebraic expression for a given value, you simply substitute the given value for the variables in the expression and then simplify. For example, if the expression is 2x + 5 and you are asked to evaluate it for x = 3, you would substitute 3 in place of x to get 2(3) + 5, which simplifies to 6 + 5, resulting in a final answer of 11.
What are the properties of algebraic expressions?
Algebraic expressions have several important properties, including the ability to combine like terms by adding or subtracting them, the use of variables to abstract unknown quantities, the ability to simplify expressions by applying the laws of operations such as the distributive property, and the flexibility to represent relationships and patterns in mathematics through equations and inequalities. Additionally, algebraic expressions can be evaluated by substituting values for variables, allowing for the solution of equations and the exploration of mathematical concepts in a structured and systematic way.
What are some real-life applications of algebraic expressions?
Real-life applications of algebraic expressions include calculating costs and savings in finance and economics, determining distances and speeds in physics and engineering, analyzing trends and patterns in data science, and designing models and formulas in chemistry and biology. Algebraic expressions play a crucial role in a wide range of fields and professions, helping to solve various practical problems and make informed decisions based on mathematical relationships and equations.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.






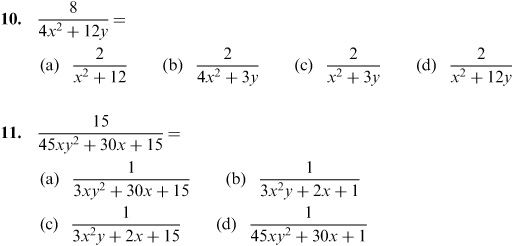
















Comments