Algebraic Expressions Worksheets 7th Grade
Algebraic Expressions Worksheets are designed to provide 7th grade students with a comprehensive practice in understanding and simplifying algebraic expressions. These worksheets focus on developing an understanding of variables, coefficients, and constants, and teach students how to evaluate and simplify expressions using order of operations. With a variety of engaging exercises and real-world examples, these worksheets provide ample opportunities for students to strengthen their algebraic skills and build a solid foundation in this important mathematical subject.
Table of Images 👆
- Math Expressions Worksheets 7th Grade
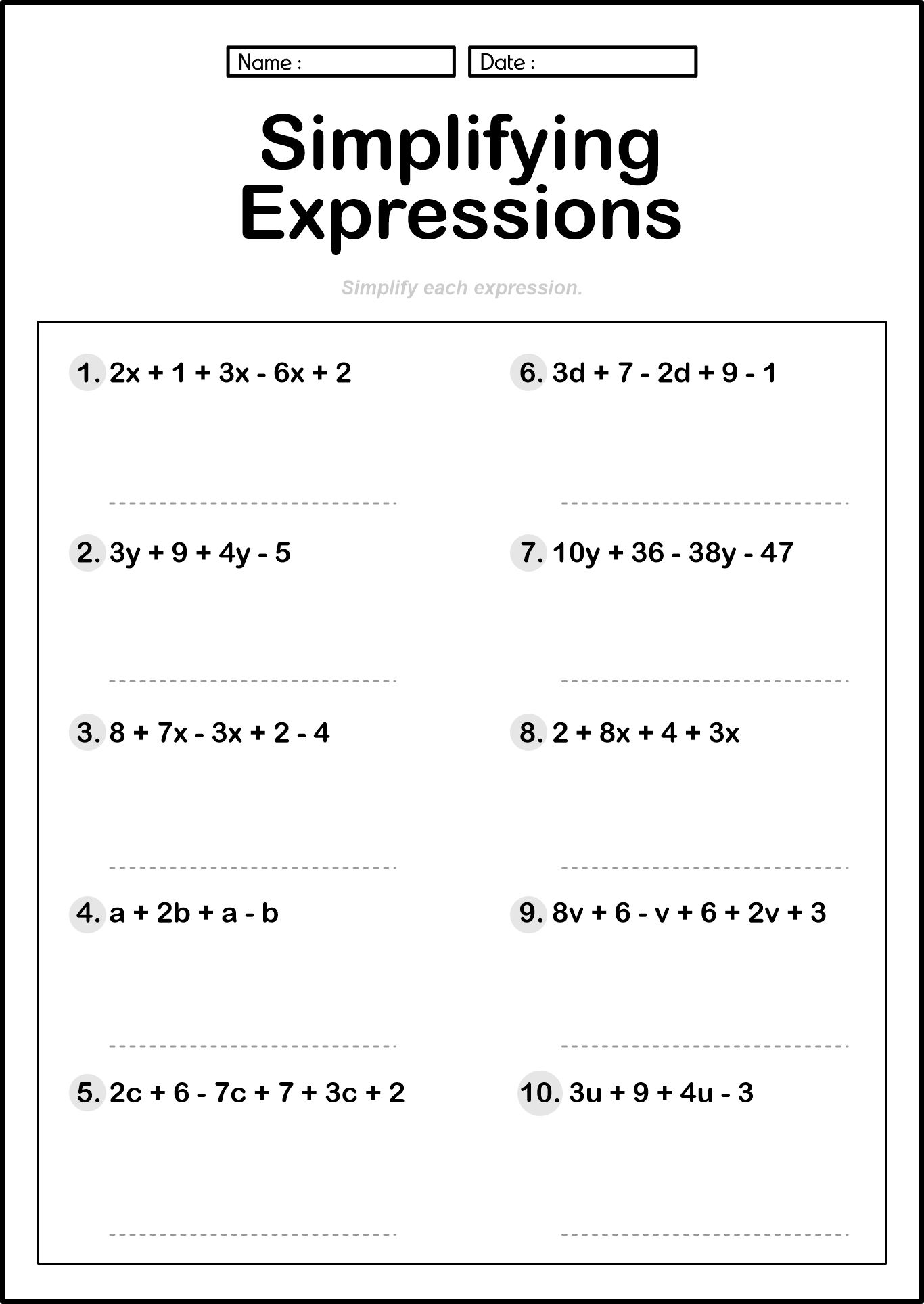
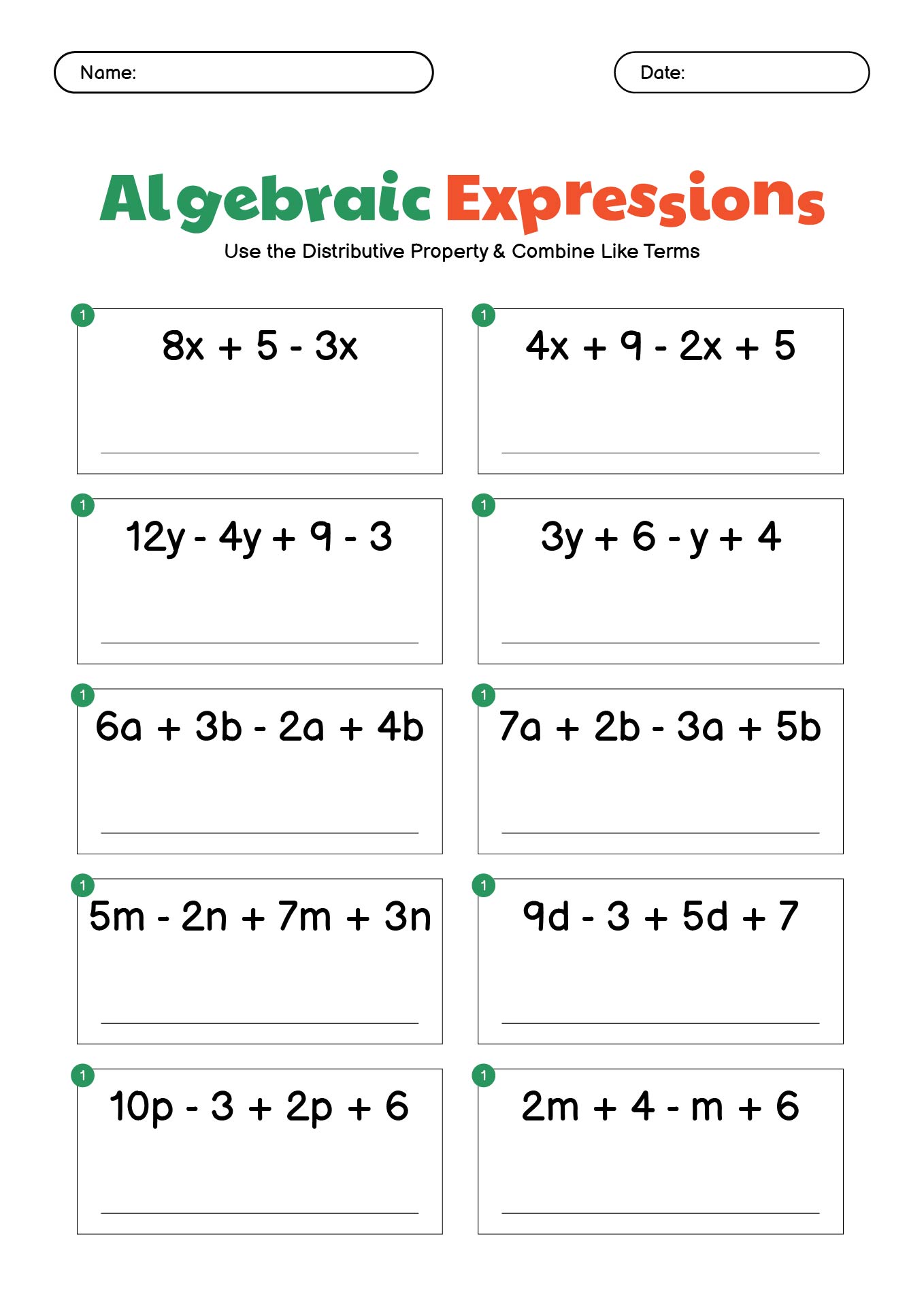
- Simplifying Expressions Worksheets 7th Grade
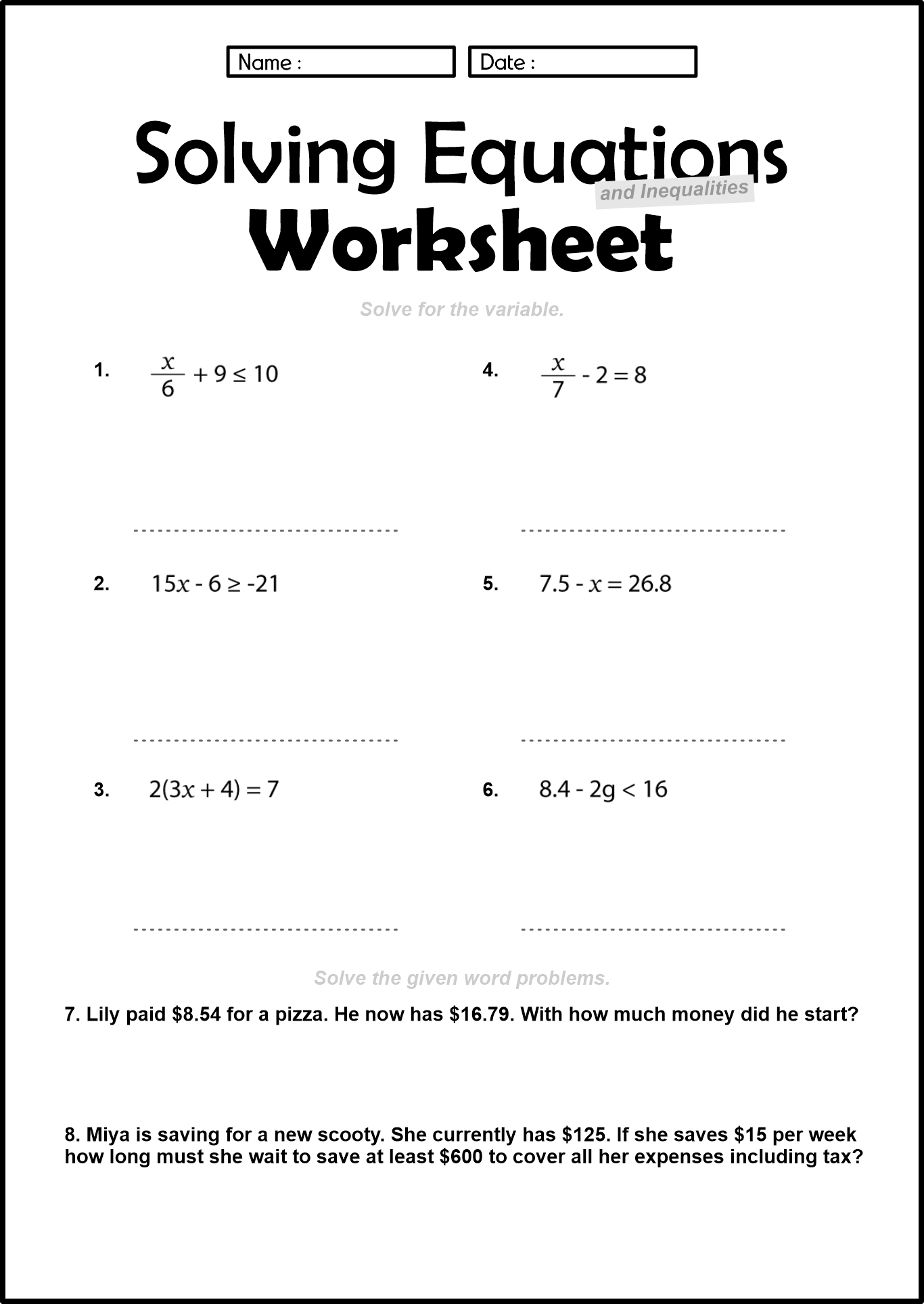
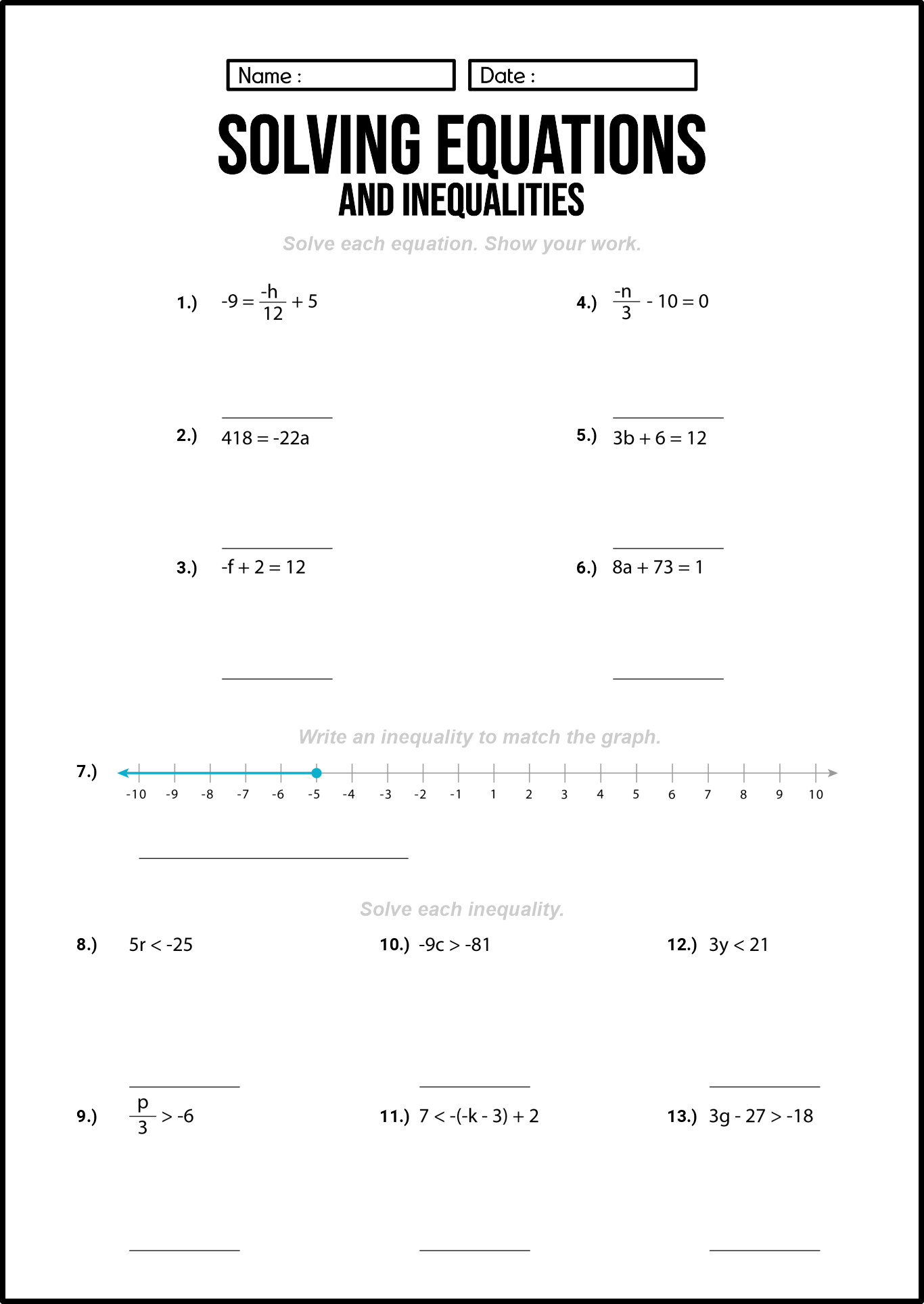
- Solving Equations Worksheets 7th Grade Math
- 7th Grade Algebra Worksheets
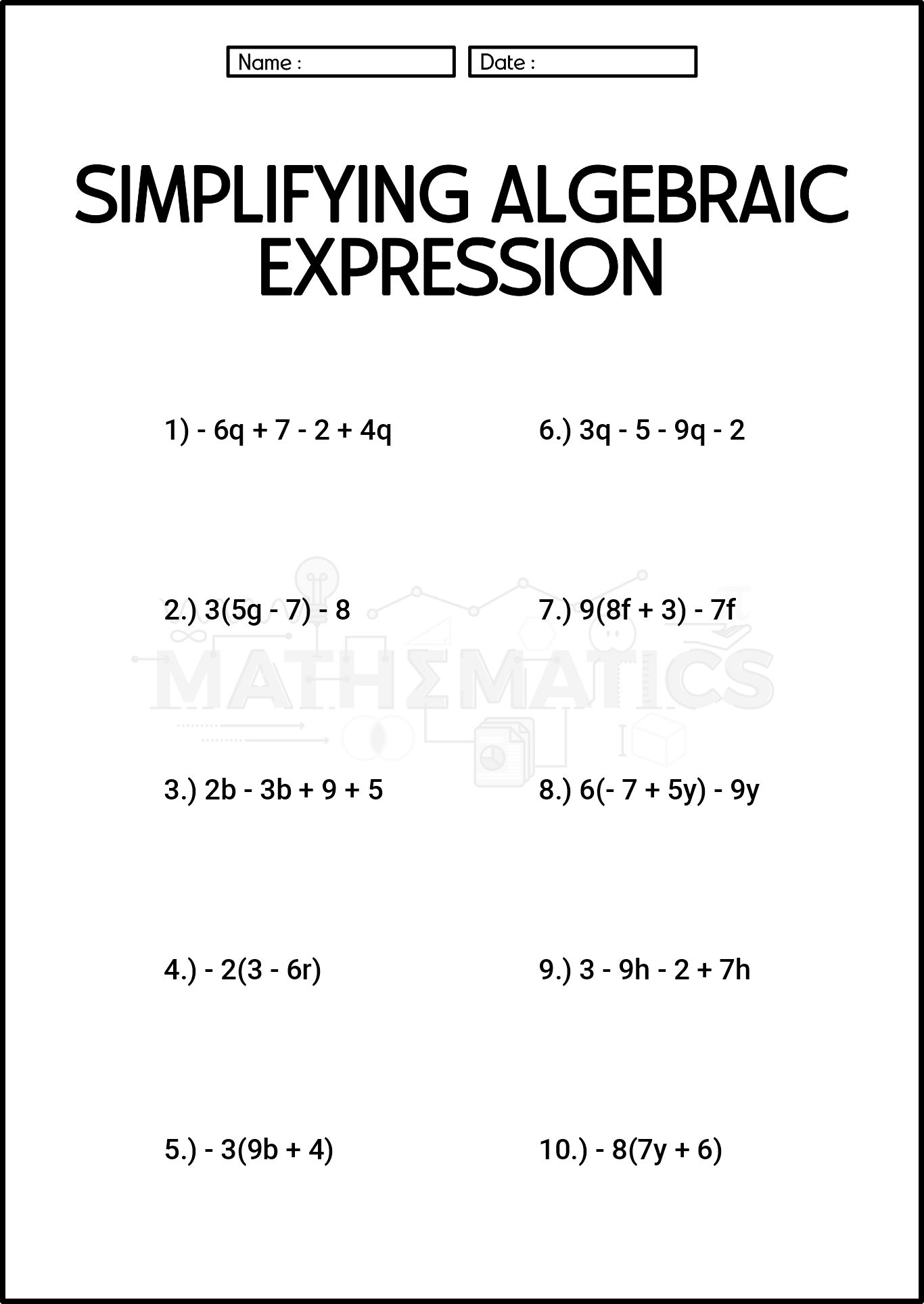
- Simplify Expressions Worksheet
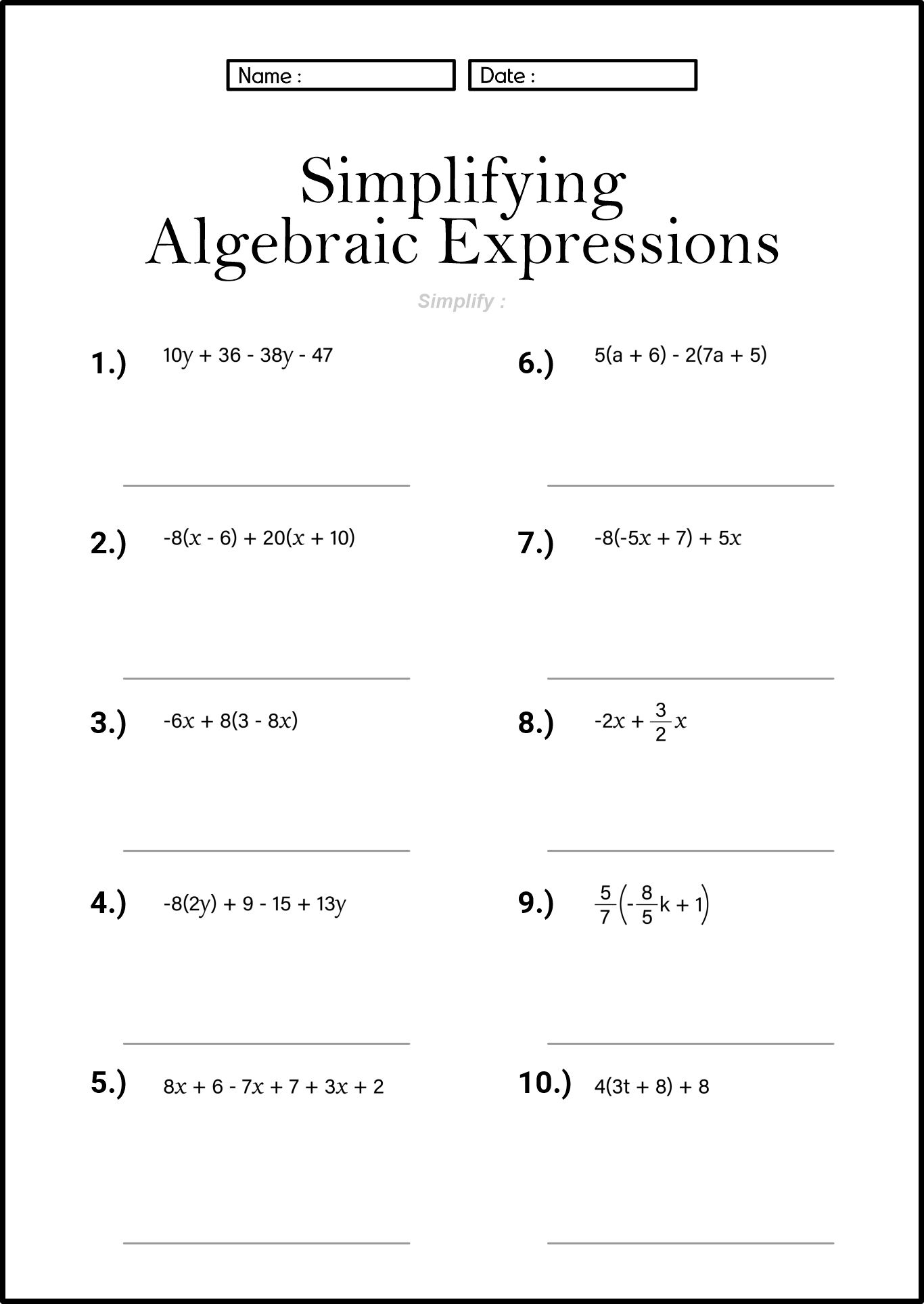
- Simplifying Algebraic Expressions Worksheet
- 7th Grade Math Algebra Equations Worksheets
- Simplifying Expressions Worksheets 7th Grade
- 7th Grade Math Worksheet Algebraic Expression
- 7th Grade Combining Like Terms Worksheet
- Simplifying Algebraic Expressions Worksheets for 7th Graders
- Algebraic Expressions and Variables Worksheets for Grade 7
- Seventh Grade Algebra Equations Activity Worksheets
- Grade 7 Algebraic Expressions Problem Sets
- 7th Grade Algebraic Equations and Inequalities Worksheets

More 7th Grade Worksheets
7th Grade Math Worksheets with Answer Key7th Grade Math Coloring Worksheets
7th Grade Vocabulary Worksheets
Pre-Algebra 7th Grade Math Worksheets
Algebraic Expressions Worksheets 7th Grade
7th Grade Math Worksheets Proportions
Complex Sentence Worksheets 7th Grade
Geometry Angles Worksheet 7th Grade Math
What are algebraic expressions?
Algebraic expressions are mathematical expressions that consist of variables, constants, and mathematical operations (such as addition, subtraction, multiplication, and division). These expressions can represent quantities and can be simplified, evaluated, or manipulated using algebraic rules. They are used to represent relationships and solve problems in algebra.
How do you simplify algebraic expressions?
To simplify algebraic expressions, you need to combine like terms by adding or subtracting coefficients of the same variables. You should also apply the distributive property when necessary. Additionally, you can use the rules of exponents and follow the order of operations to simplify expressions involving multiple operations. Finally, remember to eliminate any parentheses by distributing terms inside them.
What are like terms in algebraic expressions?
Like terms in algebraic expressions are terms that have the same variables raised to the same powers. These terms can be combined through addition or subtraction because they represent the same type of quantity. For example, in the expression 3x² + 5x² - 2x², the terms 3x², 5x², and -2x² are like terms because they all have the variable x raised to the power of 2.
How do you combine like terms in algebraic expressions?
To combine like terms in algebraic expressions, you simply add or subtract the coefficients of the terms that have the same variable(s) raised to the same power. For example, in the expression 3x + 4x - 2x, you would add the coefficients of x (3 + 4 - 2) to get 5x. Remember to keep the variable and its exponent unchanged while combining the coefficients.
How do you substitute values into algebraic expressions?
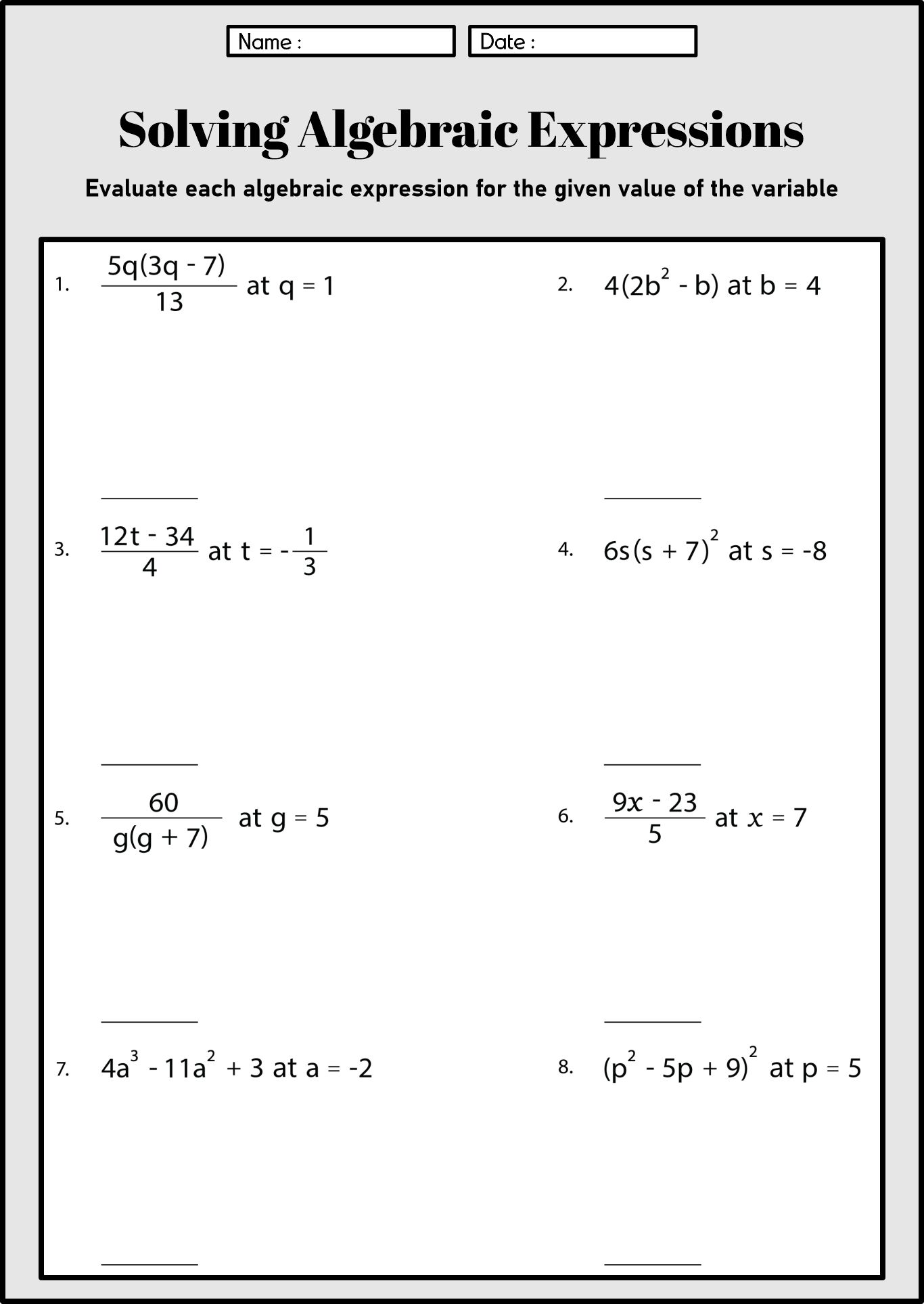
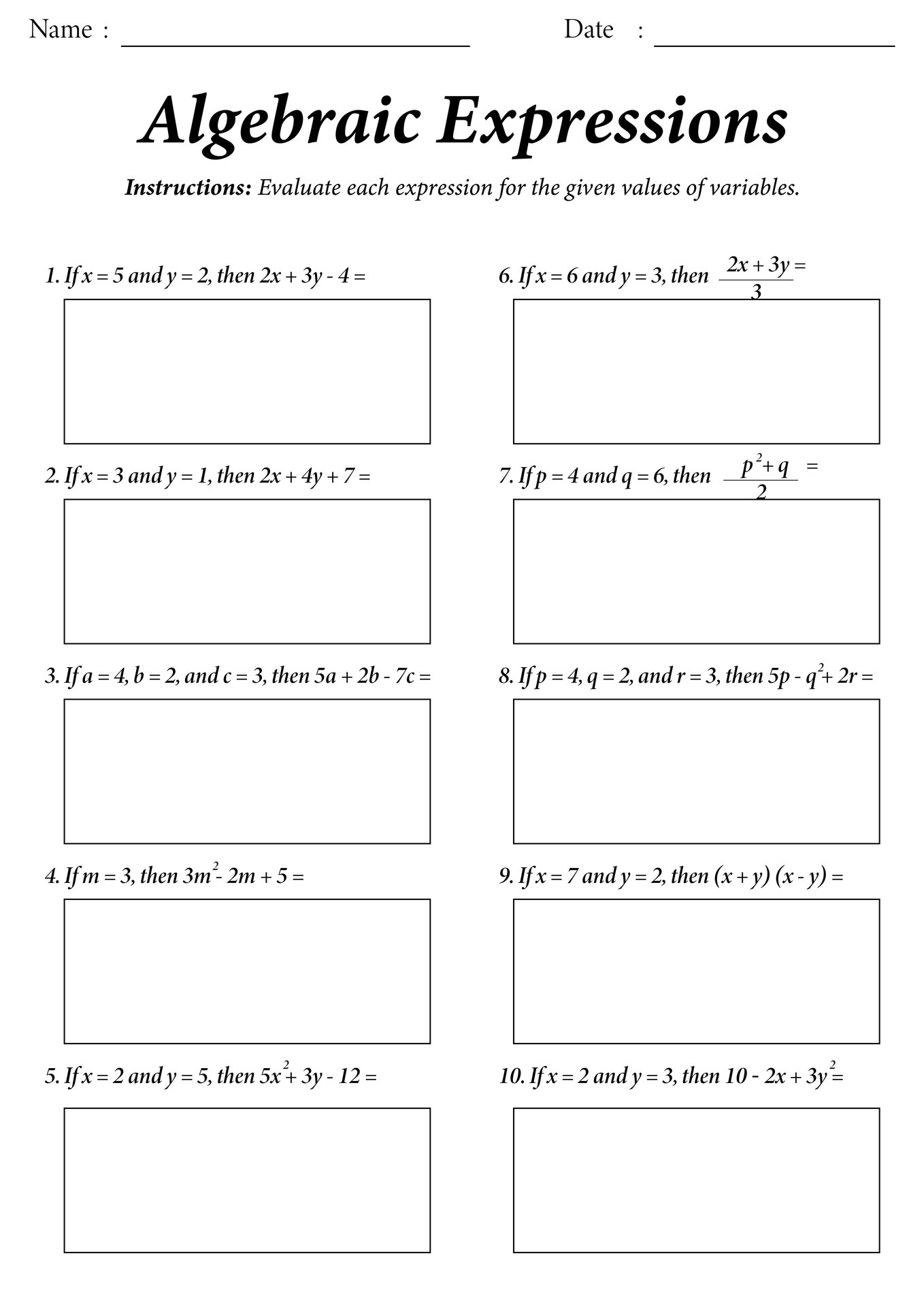
To substitute values into algebraic expressions, you simply replace any variables in the expression with the given values. For example, if the expression is 4x + 3 and x = 2, you would substitute 2 in place of x to get 4(2) + 3 = 8 + 3 = 11. Similarly, if the expression is 2y^2 - y and y = 3, you would substitute 3 in place of y to get 2(3)^2 - 3 = 2(9) - 3 = 18 - 3 = 15.
What are the different operations (addition, subtraction, multiplication, division) you can perform on algebraic expressions?
You can perform addition, subtraction, multiplication, and division operations on algebraic expressions. Addition involves combining like terms with the same variables, subtraction is similar but with subtracting terms, multiplication includes distributing and combining terms, and division is the process of simplifying and reducing expressions by dividing terms or coefficients.
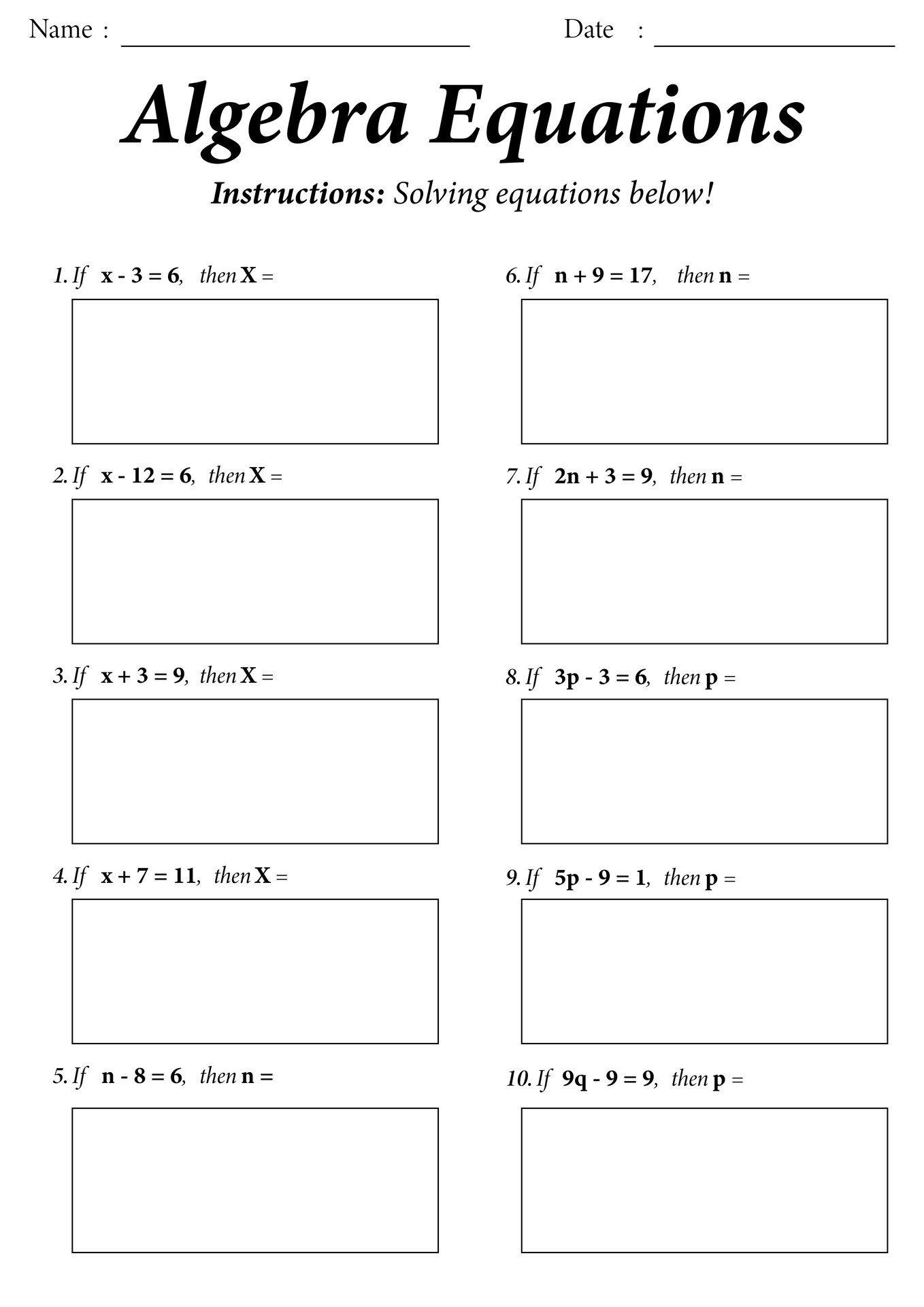
How do you solve equations involving algebraic expressions?
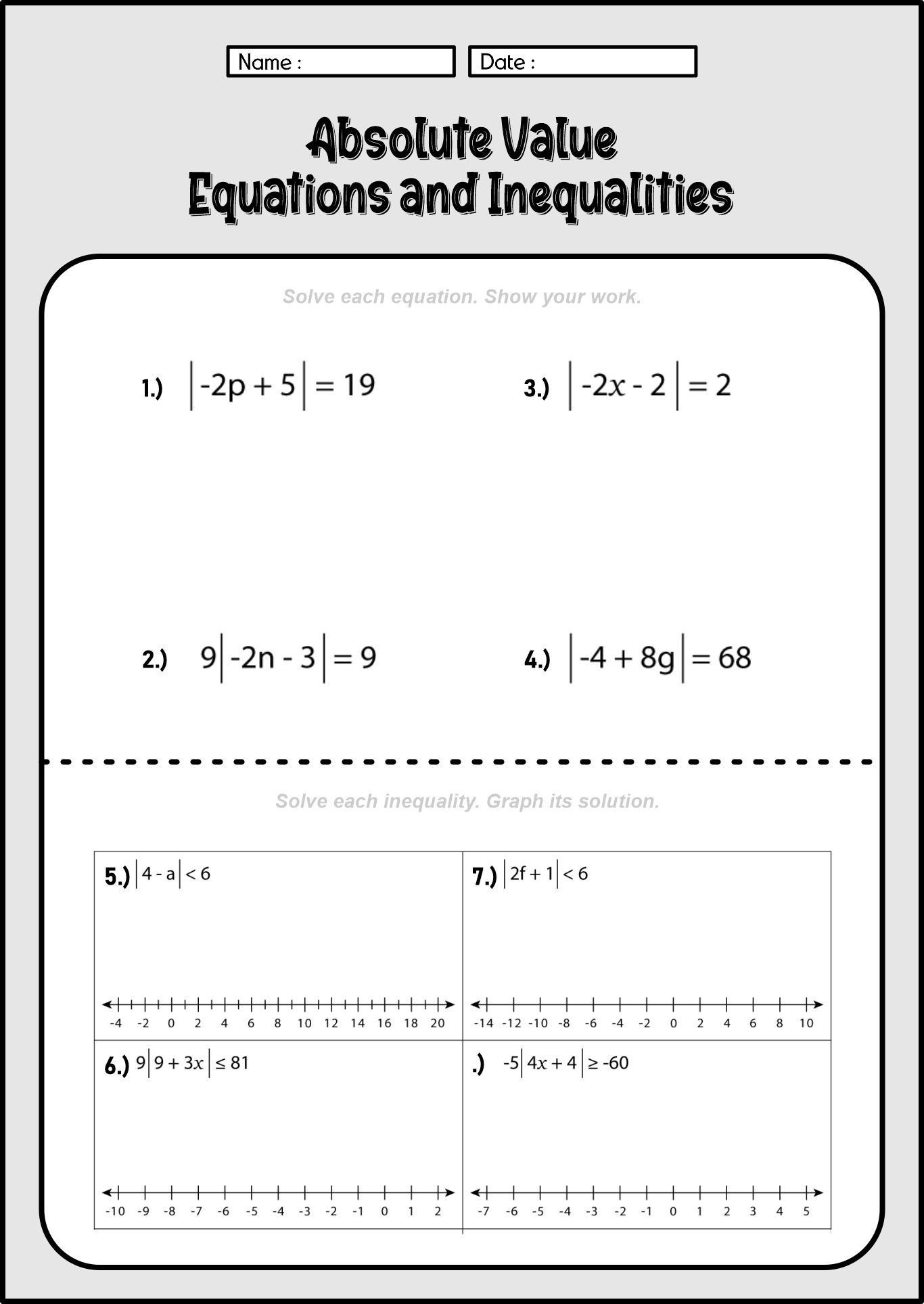
To solve equations involving algebraic expressions, you need to isolate the variable by performing a series of inverse operations. Begin by simplifying both sides of the equation through addition, subtraction, multiplication, and division to isolate the variable on one side. Ensure to perform the same operation on both sides to maintain the equality of the equation. Continue simplifying until you have the variable isolated and can solve for its value. Remember to check your solution by substituting it back into the original equation to ensure it satisfies the equality.
What are the properties of algebraic expressions?
Algebraic expressions have the properties of addition, subtraction, multiplication, and division, where terms can be combined using these operations. They can include variables, constants, and coefficients, and are written using mathematical symbols and mathematical operations. Furthermore, algebraic expressions follow the rules of arithmetic, such as the commutative, associative, and distributive properties, allowing for simplification and manipulation of the expression.
How can you determine if two algebraic expressions are equivalent?
Two algebraic expressions are considered equivalent if they yield the same value for any given set of variables. You can determine if two algebraic expressions are equivalent by simplifying and manipulating both expressions using properties such as the distributive property, commutative property, and associative property. If after simplifying the expressions, they are identical in form and value, then they are considered equivalent. Additionally, you can also substitute numerical values for the variables in the expressions and compare the results to see if they are equal, which would also indicate their equivalence.
How can you translate word problems into algebraic expressions?
To translate word problems into algebraic expressions, you should carefully read the problem and identify the unknown quantities or variables. Assign a letter, usually a single letter or variable, to represent each unknown quantity. Then use mathematical operators (+, -, x, ÷) and appropriate symbols (such as parentheses) to represent the relationships between the quantities. Remember that "is" usually means equals (=), "more than" means addition (+), "less than" means subtraction (-), "times" means multiplication (x), and "divided by" means division (÷). Finally, simplify and solve the algebraic expression to find the answer.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.





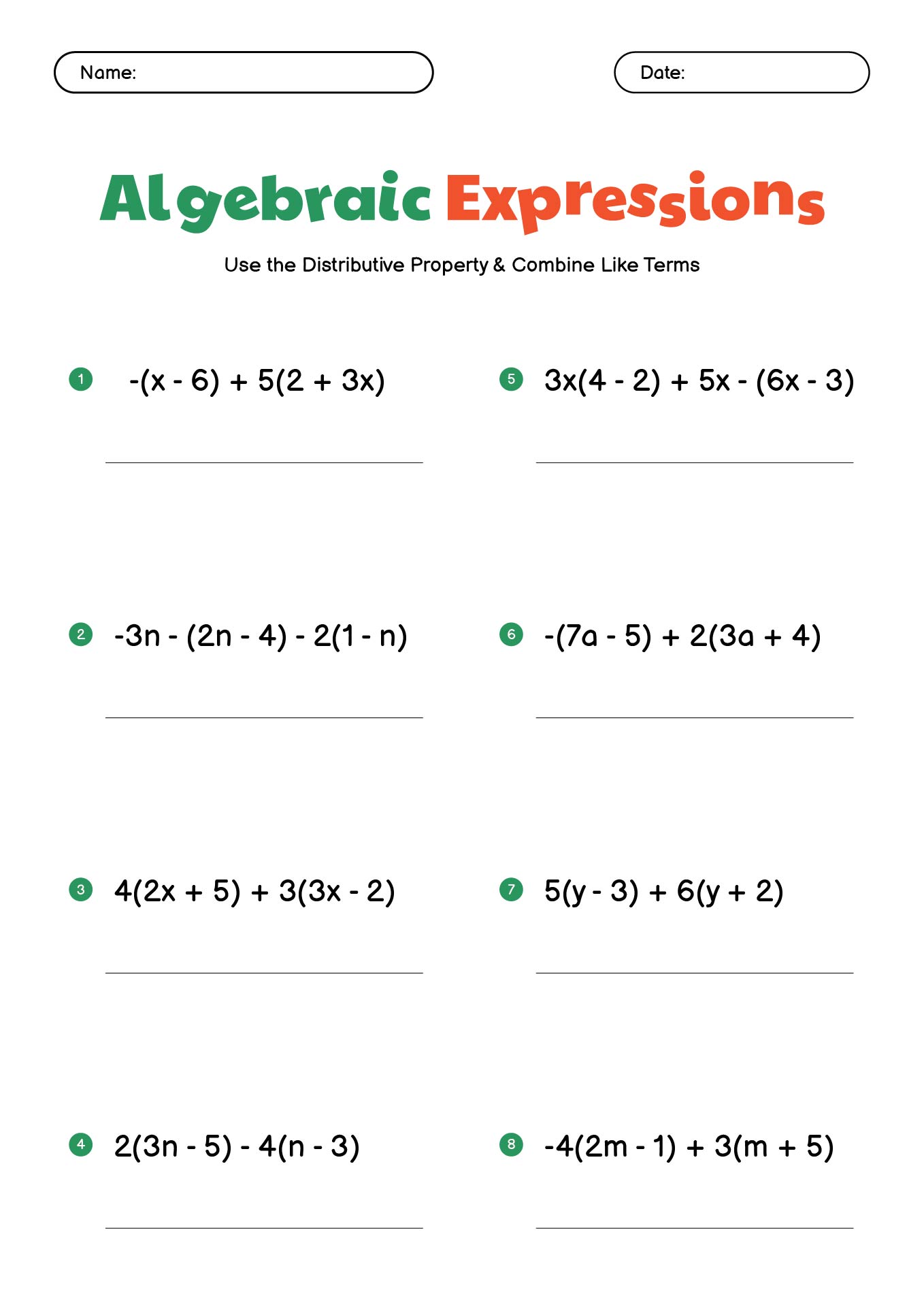














Comments